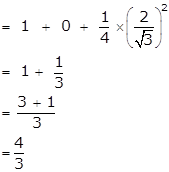Class 10 SELINA Solutions Maths Chapter 21 - Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables)
Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Exercise Ex. 21(A)
Solution 1
Solution 2
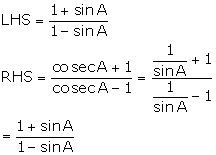
Solution 3
Solution 4
Solution 5
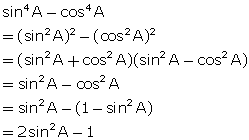
Solution 6
Solution 7
Solution 8
Solution 9
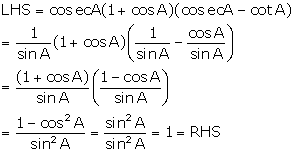
Solution 10
Solution 11
Solution 12
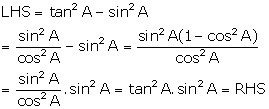
Solution 13
Solution 14
Solution 15
Solution 16
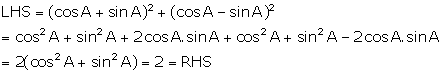
Solution 17
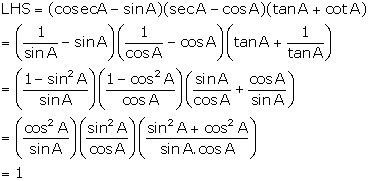
Solution 18
Solution 19
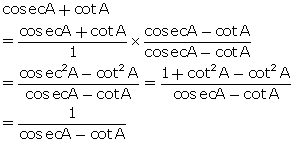
Solution 20
Solution 21
Solution 22
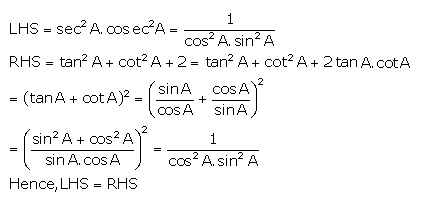
Solution 23
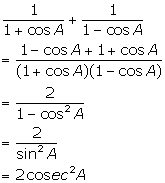
Solution 24
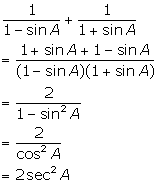
Solution 25
Solution 26
Solution 27
Solution 28
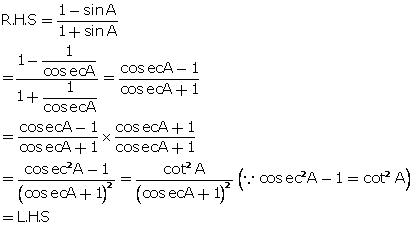
Solution 29
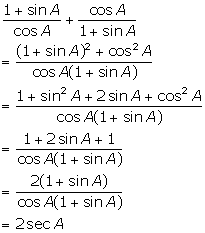
Solution 30
Solution 31
Solution 32
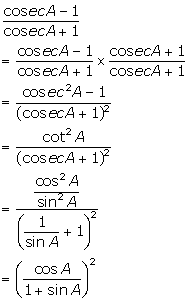
Solution 33
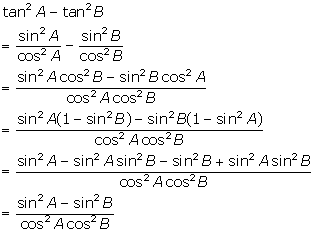
Solution 34
To prove: 
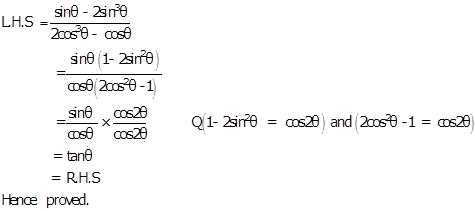
Solution 35
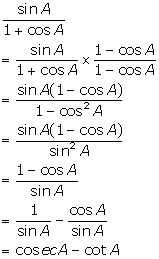
Solution 36
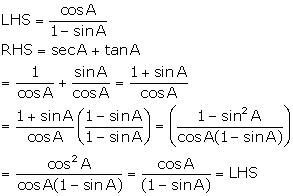
Solution 37
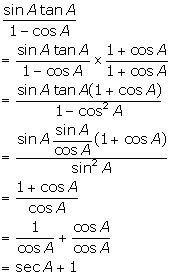
Solution 38
Solution 39
Solution 40
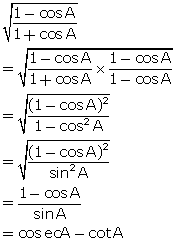
Solution 41
Solution 42
Solution 43
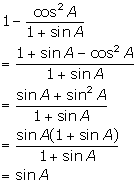
Solution 44
Solution 45
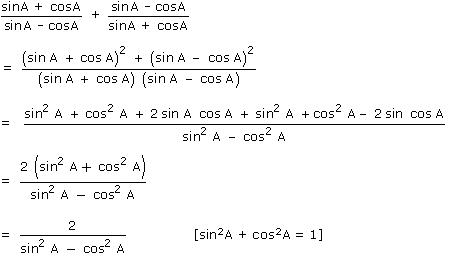
Solution 46
Solution 47
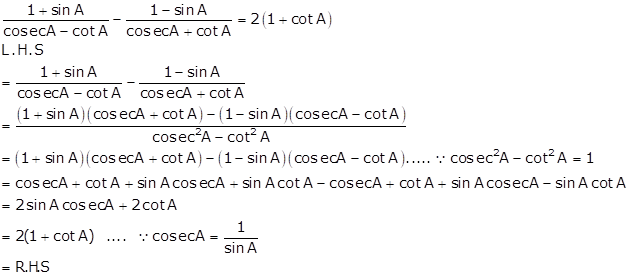
Solution 48
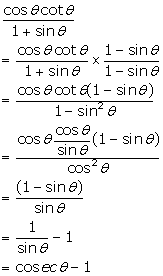
Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Exercise Ex. 21(B)
Solution 1
(i)
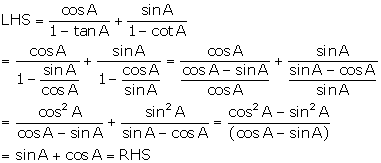
(ii)
(iii)
(iv)
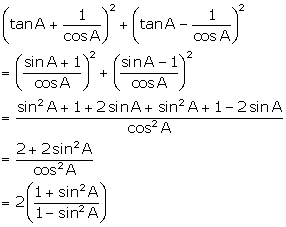
(v)
(vi)
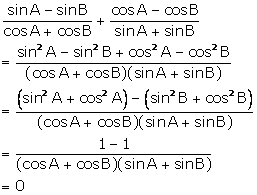
(vii)
(viii)
(ix)
Solution 2
Solution 3
Solution 4
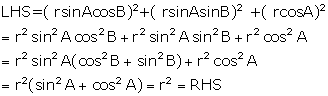
Solution 5
Given:
and
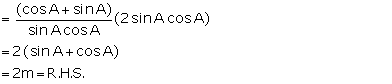
Solution 6
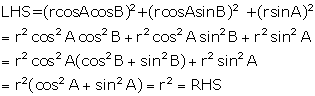
Solution 7
LHS = (m2 + n2) cos2B
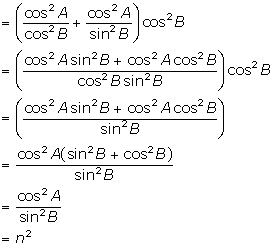
Hence, (m2 + n2) cos2B = n2.
Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Exercise Ex. 21(C)
Solution 1
(i)
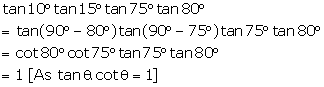
(ii)
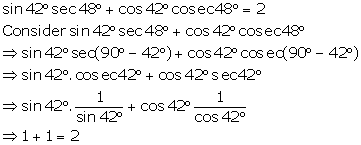
(iii)
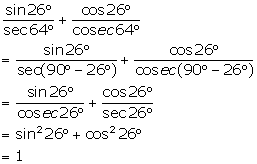
Solution 2
Solution 3
(i) 
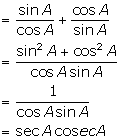
(ii) 
Solution 4
(i) We know that for a triangle 
ABC
A + B + C = 180°
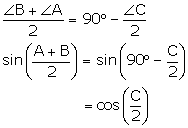
(ii) We know that for a triangle ABC
A + B + C = 180°
B + C = 180° - A
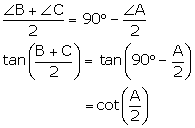
Solution 5
(i)
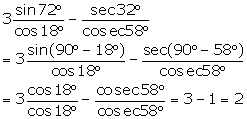
(ii) 
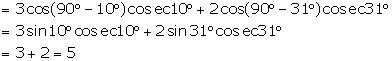
(iii) 
(iv) 
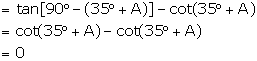
(v) 
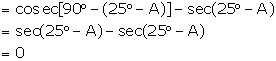
(vi) 
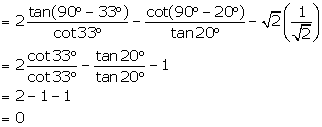
(vii) 
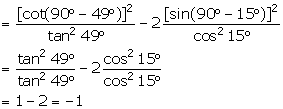
(viii) 
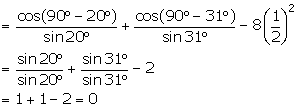
(ix) 
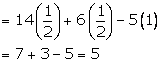
Solution 6
Since, ABC is a right angled triangle, right angled at B.
So, A + C = 90
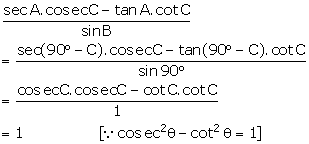
Solution 7
(i) 
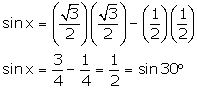
Hence, x = 
(ii) 
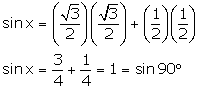
Hence, x = 
(iii) 
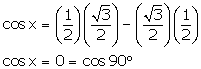
Hence, x =
(iv) 
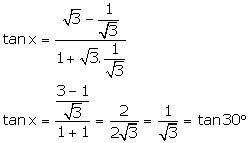
Hence, x =
(v) 
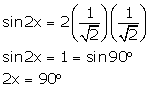
Hence, x = 
(vi) 
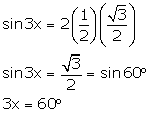
Hence, x = 
(vii) 
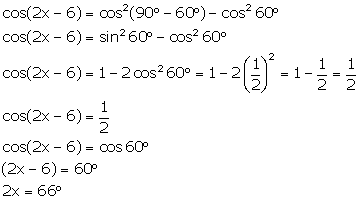
Hence, 
Solution 8
(i) 
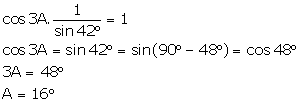
(ii) 
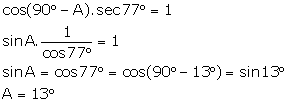
Solution 9
(i)
(ii)
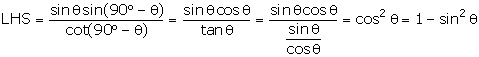
Solution 10
Solution 11
Solution 12
Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Exercise Ex. 21(D)
Solution 1
(i) sin 21o = 0.3584
(ii) sin 34o 42'= 0.5693
(iii) sin 47o 32'= sin (47o 30' + 2') =0.7373 + 0.0004 = 0.7377
(iv) sin 62o 57' = sin (62o 54' + 3') = 0.8902 + 0.0004 = 0.8906
(v) sin (10o 20' + 20o 45') = sin 30o65' = sin 31o5' = 0.5150 + 0.0012 = 0.5162
Solution 2
(i) cos 2° 4’ = 0.9994 - 0.0001 = 0.9993
(ii) cos 8° 12’ = cos 0.9898
(iii) cos 26° 32’ = cos (26° 30’ + 2’) = 0.8949 - 0.0003 = 0.8946
(iv) cos 65° 41’ = cos (65° 36’ + 5’) = 0.4131 -0.0013 = 0.4118
(v) cos (9° 23’ + 15° 54’) = cos 24° 77’ = cos 25° 17’ = cos (25° 12’ + 5’) = 0.9048 - 0.0006 = 0.9042
Solution 3
(i) tan 37o = 0.7536
(ii) tan 42o 18' = 0.9099
(iii) tan 17o 27' = tan (17o 24' + 3') = 0.3134 + 0.0010 = 0.3144
Solution 4
(i) From the tables, it is clear that sin 29o = 0.4848
Hence, 
= 29o
(ii) From the tables, it is clear that sin 22o 30' = 0.3827
Hence, = 22o 30'
(iii) From the tables, it is clear that sin 40o 42' = 0.6521
sin - sin 40o 42' = 0.6525 -; 0.6521 = 0.0004
From the tables, diff of 2' = 0.0004
Hence, = 40o 42' + 2' = 40o 44'
Solution 5
(i) From the tables, it is clear that cos 10° = 0.9848
Hence, 
= 10°
(ii) From the tables, it is clear that cos 16° 48’ = 0.9573
cos - cos 16° 48’ = 0.9574 - 0.9573 = 0.0001
From the tables, diff of 1’ = 0.0001
Hence, = 16° 48’ - 1’ = 16° 47’
(iii) From the tables, it is clear that cos 46° 30’ = 0.6884
cos q - cos 46° 30’ = 0.6885 - 0.6884 = 0.0001
From the tables, diff of 1’ = 0.0002
Hence, = 46° 30’ - 1’ = 46° 29’
Solution 6
(i) From the tables, it is clear that tan 13° 36’ = 0.2419
Hence, 
= 13° 36’
(ii) From the tables, it is clear that tan 25° 18’ = 0.4727
tan - tan 25° 18’ = 0.4741 - 0.4727 = 0.0014
From the tables, diff of 4’ = 0.0014
Hence, = 25° 18’ + 4’ = 25° 22’
(iii) From the tables, it is clear that tan 36° 24’ = 0.7373
tan - tan 36° 24’ = 0.7391 - 0.7373 = 0.0018
From the tables, diff of 4’ = 0.0018
Hence, = 36° 24’ + 4’ = 36° 28’
Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Exercise Ex. 21(E)
Solution 1
(i) 
(ii)
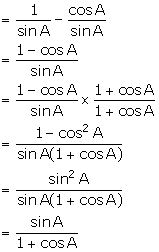
(iii) 
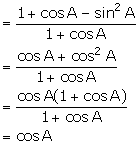
(iv) 
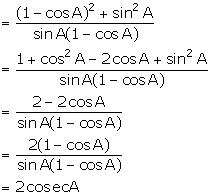
(v) 
(vi) 
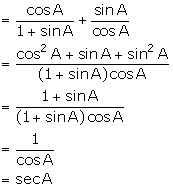
(vii) 
(viii) 
(ix) 
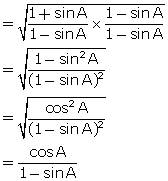
(x) 
(xi)
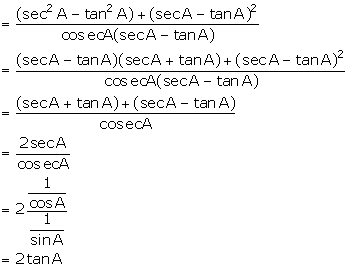
(xii) 
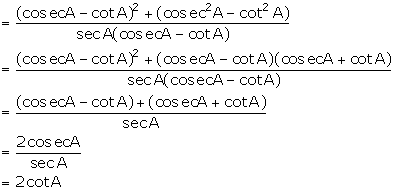
(xiii) 
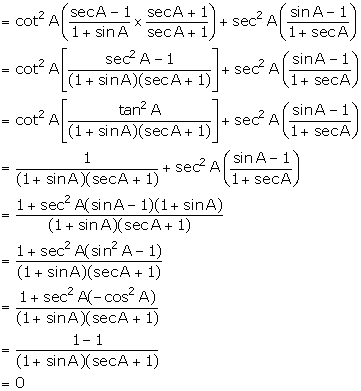
(xiv) 
(xv) 
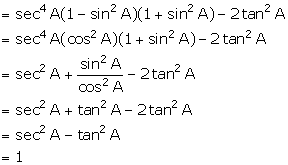
(xvi) 
(xvii) 
Solution 2
Solution 3
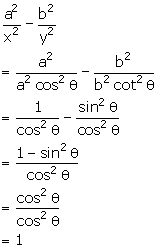
Solution 4
Solution 5
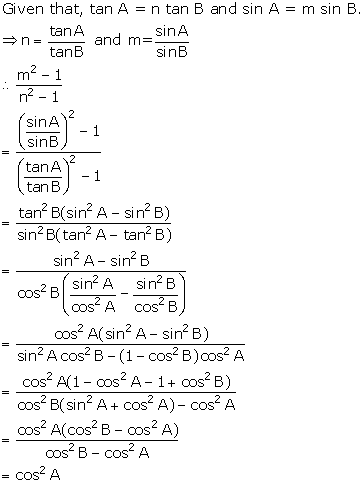
Solution 6
(i) 2 sinA - 1 = 0
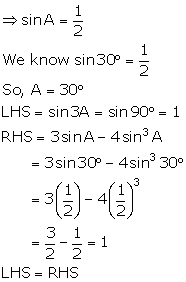
(ii)
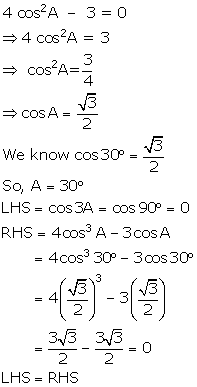
Solution 7
(i) 
(ii) 
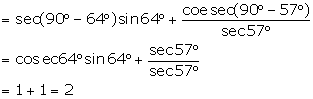
(iii) 
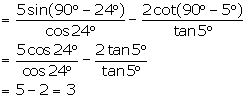
(iv) 
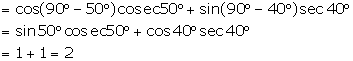
(v) 
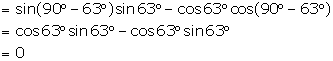
(vi) 
(vii) 
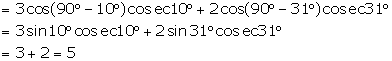
(viii) 
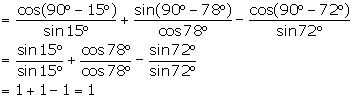
Solution 8
(i) 
(ii) 
(iii) 
(iv) 
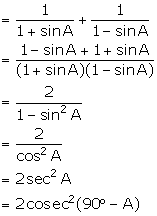
(v) 
Solution 9
Since, A and B are complementary angles, A + B = 90°
(i)
(ii)
(iii)
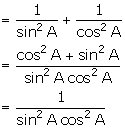
= cosec2A [sec(90 - B)]2
= cosec2A cosec2B
(iv) 
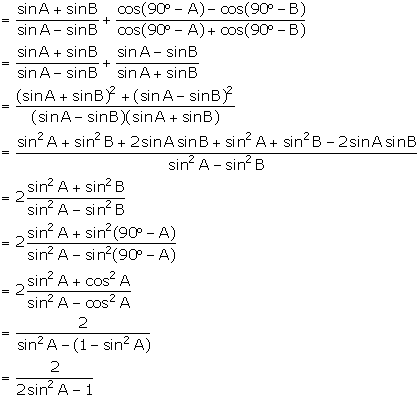
Solution 10
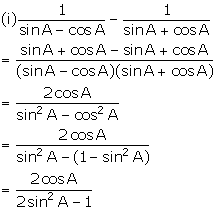
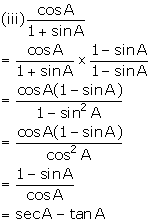
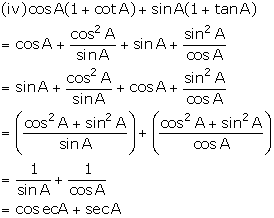
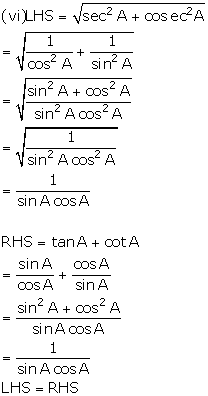
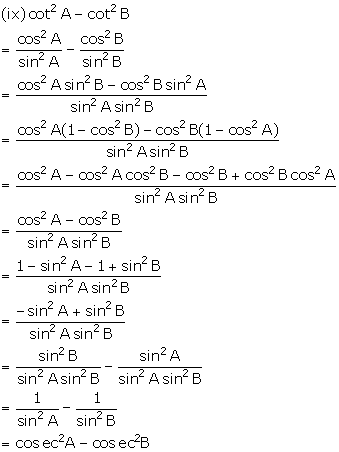
Solution 11
4 cos2A - 3 = 0
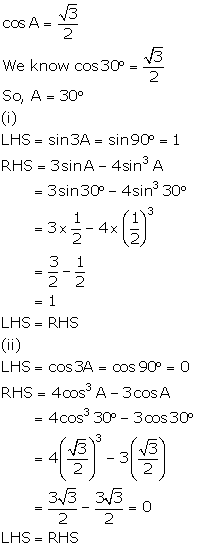
Solution 12
(i) 
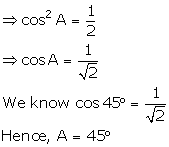
(ii) sin 3A - 1 = 0
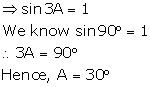
(iii) 
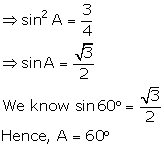
(iv) 
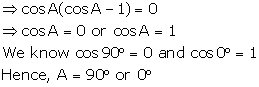
(v) 
Solution 13
(i) 
(ii) 
Solution 14
_ASH_files/20141209110714_image002.gif)
Solution 15
Solution 16
sin2
28° + sin2
62° + tan2
38° - cot2
52° + 
sec2 30°
= sin2
28° + [sin (90 -
28)°]2 +
tan2 38° - [cot(90 - 38)°]2 +
sec2 30°
= sin2
28° + cos2 28° + tan2
38° - tan2
38° + sec2 30°
