Class 10 SELINA Solutions Maths Chapter 18 - Tangents and Intersecting Chords
Tangents and Intersecting Chords Exercise Ex. 18(A)
Solution 1
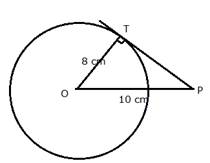
OP = 10 cm; radius OT = 8 cm

Length of tangent = 6 cm.
Solution 2
AB = 15 cm, AC = 7.5 cm
Let 'r' be the radius of the circle.
![]() OC = OB = r
OC = OB = r
AO = AC + OC = 7.5 + r
In ∆AOB,
AO2 = AB2 + OB2
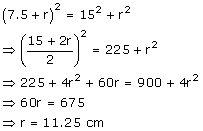
Therefore, the length of radius of a circle is 11.25 cm.
Solution 3
From Q, QA and QP are two tangents to the circle with centre O.
Therefore, QA = QP .....(i)
Similarly, from Q, QB and QP are two tangents to the circle with centre O'.
Therefore, QB = QP ......(ii)
From (i) and (ii)
QA = QB
Therefore, tangents QA and QB are equal.
Solution 4
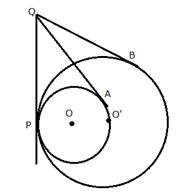
From Q, QA and QP are two tangents to the circle with centre O.
Therefore, QA = QP .......(i)
Similarly, from Q, QB and QP are two tangents to the circle with centre O'.
Therefore, QB = QP .......(ii)
From (i) and (ii)
QA = QB
Therefore, tangents QA and QB are equal.
Solution 5
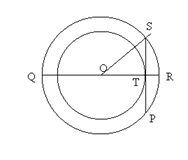
Radius of outer circle, OS = 5 cm.
Radius of inner circle, OT = 3 cm.
 (Angle between the radius and the tangent)
(Angle between the radius and the tangent)
In Rt. triangle OTS, by Pythagoras Theorem,

Since OT is perpendicular to SP and OT bisects chord SP,
SP = 8 cm
Solution 6
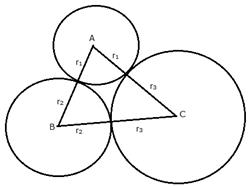
AB = 6 cm, AC = 8 cm and BC = 9 cm
Let radii of the circles having centers A, B and C be r1, r2 and r3 respectively.
r1 + r3 = 8 ....(1)
r3 + r2 = 9 ....(2)
r2 + r1 = 6 ....(3)
Adding (1), (2) and (3),
r1 + r3 + r3 + r2 + r2 + r1 = 8 + 9 + 6
2(r1 + r2 + r3) = 23
r1 + r2 + r3 = 11.5 cm
r1 + 9 = 11.5 (Since r2 + r3 = 9)
r1 = 2.5 cm
r2 + 8 = 11.5 (Since r1 + r3 = 8)
r2 = 3.5 cm
r3 + 6 = 11.5 (Since r1 + r2 = 6)
r3 = 5.5 cm
Hence, r1 = 2.5 cm, r2 = 3.5 cm and r3 = 5.5 cm
Solution 7
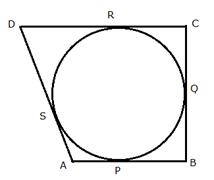
Let the circle touch the sides AB, BC, CD and DA of quadrilateral ABCD at P, Q, R and S respectively.
Since AP and AS are tangents to the circle from external point A,
AP = AS ....(i)
Similarly, we can prove that:
BP = BQ ....(ii)
CR = CQ ....(iii)
DR = DS ....(iv)
Adding (i), (ii), (iii) and (iv),
AP + BP + CR + DR = AS + DS + BQ + CQ
AB + CD = AD + BC
Hence, AB + CD = AD + BC
Solution 8
From A, AP and AS are tangents to the circle.
Therefore, AP = AS ....(i)
Similarly, we can prove that
BP = BQ ....(ii)
CR = CQ ....(iii)
DR = DS ....(iv)
Adding,
AP + BP + CR + DR = AS + DS + BQ + CQ
AB + CD = AD + BC
But AB = CD and BC = AD ....(v) [Opposite sides of a parallelogram]
Therefore, AB + AB = BC + BC
2AB = 2BC
AB = BC ....(vi)
From (v) and (vi)
AB = BC = CD = DA
Hence, ABCD is a rhombus.
Solution 9
From B, BQ and BP are the tangents to the circle.
Therefore, BQ = BP ....(i)
Similarly, we can prove that
AP = AR ....(ii)
and CR = CQ ....(iii)
Adding,
AP + BQ + CR = BP + CQ + AR ....(iv) [Proved]
Adding (AP + BQ + CR) to both sides,
2(AP + BQ + CR) = AP + BP + CQ + BQ + AR + CR
2(AP + BQ + CR) = AB + BC + CA
Therefore, AP + BQ + CR = ![]() x (AB + BC + CA)
x (AB + BC + CA)
AP + BQ + CR = ![]() x Perimeter of triangle ABC
x Perimeter of triangle ABC
Solution 10
From A, AP and AR are the tangents to the circle.
Therefore, AP = AR.
Similarly, we can prove that
BP = BQ and CR = CQ
Adding,
AP + BP + CQ = AR + BQ + CR
(AP + BP) + CQ = (AR + CR) + BQ
AB + CQ = AC + BQ
But AB = AC
Therefore, BQ = CQ
Solution 11
Radius of bigger circle = 6.3 cm
Radius of smaller circle = 3.6 cm
i)
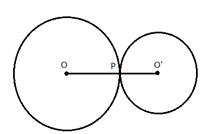
Two circles are touching each other at P externally.
O and O’ are the centers of the circles.
Join OP and O’P
OP = 6.3 cm, O’P = 3.6 cm
Distance between the centres = OO'
= OP + O’P
= 6.3 + 3.6
= 9.9 cm
ii)
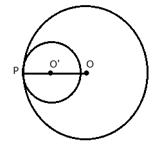
Two circles are touching each other at P internally.
O and O’ are the centers of the circles.
Join OP and O’P.
OP = 6.3 cm, O’P = 3.6 cm
Distance between the centres = OO’
= OP - O’P
= 6.3 - 3.6
= 2.7 cm
Solution 12
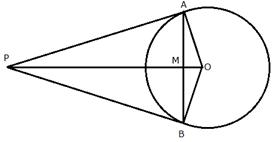
i) In ![]()
AP = BP (Tangents from P to the circle)
OP = OP (Common)
OA = OB (Radii of the same circle)
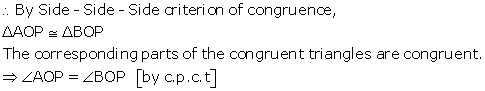
ii) In ![]()
OA = OB (Radii of the same circle)
![]() (Proved
(Proved ![]() )
)
OM = OM (Common)

Hence, OM or OP is the perpendicular bisector of chord AB.
Solution 13
Draw TPT' as common tangent to the circles.
i) TA and TP are the tangents to the circle with centre O.
Therefore, TA = TP ....(i)
Similarly, TP = TB ....(ii)
From (i) and (ii)
TA = TB
Therefore, TPT' is the bisector of AB.
ii) Now in ![]()
![]()
Similarly in ![]()
![]()
Adding,

Solution 14
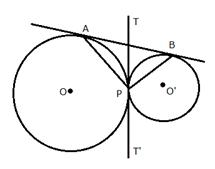
In quadrilateral OPAQ,

Now,
In triangle OPQ,
OP = OQ (Radii of the same circle)
From (i) and (ii)
![]()
Solution 15
AP and BQ are two parallel tangents of a circle with centre O metting the third tangent at points P and Q respectively.
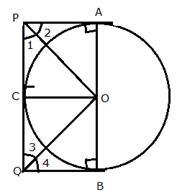
Join OP, OQ, OA, OB and OC.
In ![]()
OA = OC (Radii of the same circle)
OP = OP (Common)
PA = PC (Tangents from P)
Similarly, we can prove that
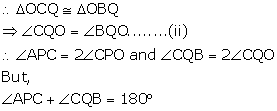
(Sum of interior angles of a transversal)
![]()
Now in ![]()
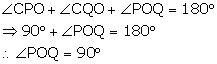
That is, PQ subtends a right angle at the centre.
Solution 16
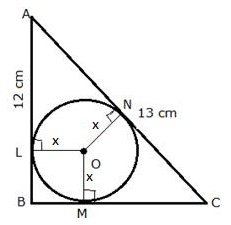
In ![]()

Therefore, LBMO is a square.
LB = BM = OM = OL = x
![]()
Since ABC is a right triangle
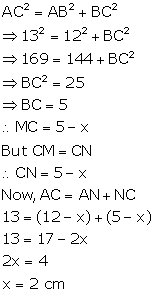
Solution 17
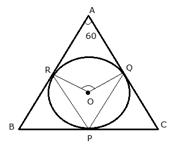
The incircle touches the sides of the triangle ABC and
![]()
i) In quadrilateral AROQ,
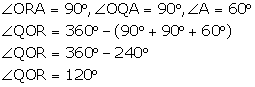
ii) Now arc RQ subtends ![]() at the centre and
at the centre and ![]() at the remaining part of the circle.
at the remaining part of the circle.

Solution 18
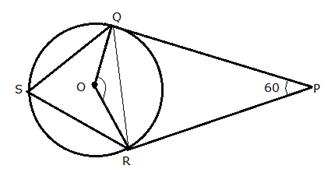
Join QR.
i) In quadrilateral ORPQ,
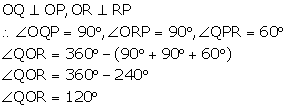
![]()
ii) In ![]()
OQ = QR (Radii of the same circle)
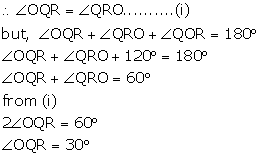
iii) Now arc RQ subtends ![]() at the centre and
at the centre and ![]() at the remaining part of the circle.
at the remaining part of the circle.

Solution 19
In ![]()
OB = OC (Radii of the same circle)

Now in ![]()
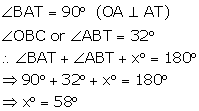
Solution 20

BQ and BR are tangents from point B to the circle.
Therefore, BR = BQ = 27 cm.
Also CR = (38 - 27) = 11 cm
Since CR and CS are tangents from point C to the circle,
CS = CR = 11 cm
So, DS = (25 - 11) = 14 cm
Now DS and DP are tangents from point D to the circle.
Therefore, DS = DP.
Now, ![]() (given)
(given)
and ![]()

Hence, OSDP is a square.
Then, OP = OS = DP = DS = 14 cm
Therefore, radius = OS = 14 cm.
Solution 21
 (angles in alternate segment)
(angles in alternate segment)
But OS = OR (Radii of the same circle)

Now,
OQ = OR (radii of same circle)

But in ![]()

From (i) and (ii),

Solution 22

Join AT and BT.
i) TC is the diameter of the circle
![]() (Angle in a semi-circle)
(Angle in a semi-circle)
ii) ![]()
![]() (Angles in the same segment of the circle)
(Angles in the same segment of the circle)
![]()
![]() (Angles in the same segment of the circle)
(Angles in the same segment of the circle)
![]()
iii) ![]() (angle between a tangent and a chord through the point of contact is equal to an angle in the alternate segment)
(angle between a tangent and a chord through the point of contact is equal to an angle in the alternate segment)
![]()
Now in ![]()

Solution 23
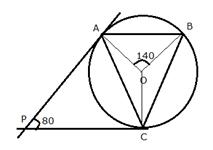
Join OC.
Now, PA and PC are the tangents to the circle with centre O.
![]()
In quadrilateral APCO,


Now, arc BC subtends ![]() at the centre and
at the centre and ![]() at the remaining part of the circle
at the remaining part of the circle
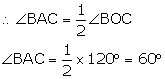
Solution 24
∠CAB = ∠BAQ = 30° (AB is angle bisector of ∠CAQ)
Then, ∠CAQ = 2∠BAQ = 60°
Now, ∠CAQ + ∠PAC = 180° (angles in linear pair)
∴ ∠PAC = 180° - 60° = 120°
∠PAC = 2∠CAD (AD is angle bisector of ∠PAC)
∠CAD = 60°
Now,
∠DAB = ∠CAD + ∠CAB = 60° + 30° = 90°
Thus, BD subtends a right angle on the circle.
So, BD is the diameter of the circle.
Tangents and Intersecting Chords Exercise Ex. 18(B)
Solution 1
i) Since two chords AB and CD intersect each other at P.

ii) Since two chords AB and CD intersect each other at P.
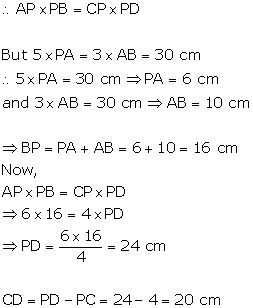
iii) Chord BA and tangent PT of a circle intersect each other at point P outside the circle.

Solution 2

Solution 3
i) PAQ is a tangent and AB is the chord.
![]() (angles in the alternate segment)
(angles in the alternate segment)
ii) OA = OD (radii of the same circle)
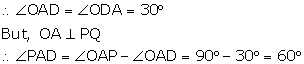
iii) BD is the diameter.
![]() (angle in a semi-circle)
(angle in a semi-circle)
Now in ![]()

Solution 4
PQ is a tangent and OR is the radius.

But in ![]()
OT = OR (Radii of the same circle)

In ![]()
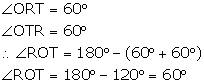
Solution 5
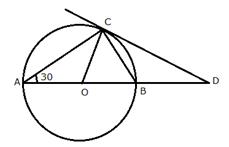
Join OC.
![]() (angles in alternate segment)
(angles in alternate segment)
Arc BC subtends  at the centre of the circle and
at the centre of the circle and ![]() at the remaining part of the circle.
at the remaining part of the circle.
![]()
Now in ![]()
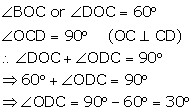
Now in ![]()
![]()
Solution 6
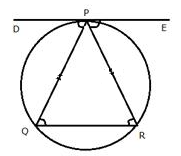
DE is the tangent to the circle at P.
DE||QR (Given)

Since the angle between a tangent and a chord through the point of contact is equal to the angle in the alternate segment
![]() (DE is tangent and PQ is chord)
(DE is tangent and PQ is chord)
from (i) and (ii)
![]()
Hence, triangle PQR is an isosceles triangle.
Solution 7

Join O'A and O'B.
CD is the tangent and AO is the chord.
![]() (angles in alternate segment)
(angles in alternate segment)
In ![]()
OA = OB (Radii of the same circle)
![]()
From (i) and (ii)
![]()
Therefore, OA is bisector of ![]() BAC.
BAC.
Solution 8
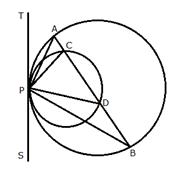
Draw a tangent TS at P to the circles given.
Since TPS is the tangent, PD is the chord.

Subtracting (i) from (ii)
![]()
But in ![]()
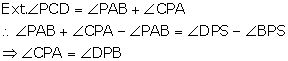
Solution 9
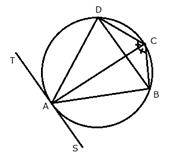

TAS is a tangent and AB is a chord

But these are alternate angles
Therefore, TS||BD.
Solution 10
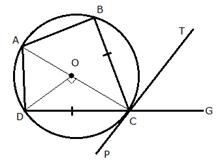
Join OC, OD and OA.
i)

ii)
PCT is a tangent and CA is a chord.
![]()
But arc DC subtends ![]() at the centre and
at the centre and ![]() at the
at the
remaining part of the circle.
![]()
Solution 11
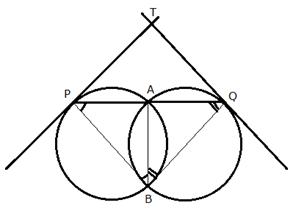
Join AB, PB and BQ
TP is the tangent and PA is a chord
![]() (angles in alternate segment)
(angles in alternate segment)
Similarly,
![]()
Adding (i) and (ii)

But they are the opposite angles of the quadrilateral PBQT.
Therefore, PBQT are cyclic.
Hence, P, B, Q and T are concyclic.
Solution 12
i) PA is the tangent and AB is a chord
![]() ( angles in the alternate
segment)
( angles in the alternate
segment)
AD is the bisector of ![]()
![]()
In ![]()
![]()
Therefore, ![]() is an isosceles
triangle.
is an isosceles
triangle.
ii) In ![]()

Solution 13
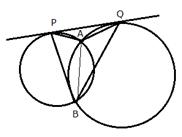
Join AB.
 (angles in alternate segment)
(angles in alternate segment)
Similarly,

Adding (i) and (ii),
From (iii) and (iv)
Hence, ![]() and
and ![]() are supplementary.
are supplementary.
Solution 14
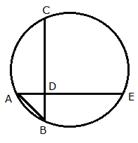
Join AB.
i) In Rt. ![]()

Chords AE and CB intersect each other at D inside the circle.
Therefore,
AD x DE = BD x DC
3 x DE = 4 x 9
DE = 12 cm
ii) Given, AD = BD ....(i)
We know that:
AD x DE = BD x DC
But AD = BD
Therefore, DE = DC ....(ii)
Adding (i) and (ii)
AD + DE = BD + DC
Therefore, AE = BC
Solution 15
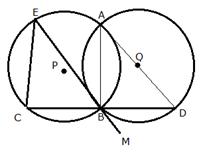
Join AB and AD
EBM is a tangent and BD is a chord.
![]() (angles in alternate segments)
(angles in alternate segments)
![]() (Vertically opposite angles)
(Vertically opposite angles)
![]()
Since in the same circle or congruent circles, if angles are equal, then chords opposite to them are also equal.
Therefore, CE = BD
Solution 16
AB is a tangent and hence, is a straight line.
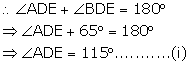
AB i.e. DB is tangent to the circle at point B and BC is the diameter.
Now, OE = OC (radii of the same circle)

(vertically opposite angles)
In ![]()
Tangents and Intersecting Chords Exercise Ex. 18(C)
Solution 1
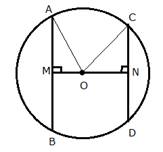
Given: A circle with centre O and
radius r. ![]() . Also AB > CD
. Also AB > CD
To prove: OM < ON
Proof: Join OA and OC.
In Rt.![]()
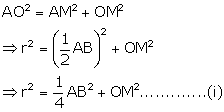
Again in Rt. ![]()
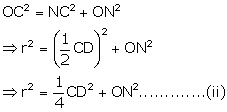
From (i) and (ii)
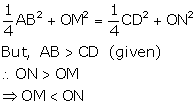
Hence, AB is nearer to the centre than CD.
Solution 2
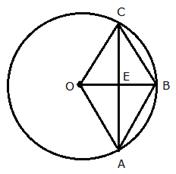
i) Radius = 10 cm
In rhombus OABC,
OC = 10 cm
![]()
In Rt.![]()
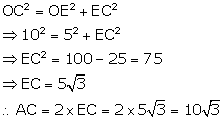
Area of rhombus = ![]()

ii) Area of rhombus = ![]()
But area of rhombus OABC = 2 x area of ![]()
![]()
Where r is the side of the equilateral triangle OAB.
Therefore, radius of the circle = 8 cm
Solution 3

If two circles touch internally, then distance between their centres is equal to the difference of their radii. So, AB = (5 - 3) cm = 2 cm.
Also, the common chord PQ is the perpendicular bisector of AB. Therefore, AC = CB = ![]() AB = 1 cm
AB = 1 cm
In right ![]() ACP, we have AP2 = AC2 + CP2
ACP, we have AP2 = AC2 + CP2
![]() 52 = 12 + CP2
52 = 12 + CP2
![]() CP2 = 25 - 1 = 24
CP2 = 25 - 1 = 24
![]() CP =
CP = ![]()
Now, PQ = 2 CP
= 2 x ![]() cm
cm
![]()
Solution 4
Given: AB and AC are two equal chords of C (O, r).
To prove: Centre, O lies on the bisector of ![]() BAC.
BAC.
Construction: Join BC. Let the bisector of ![]() BAC intersects BC in P.
BAC intersects BC in P.
Proof:
In ![]() APB and
APB and ![]() APC,
APC,
AB = AC (Given)
![]() BAP =
BAP = ![]() CAP (Given)
CAP (Given)
AP = AP (Common)
![]() (SAS congruence criterion)
(SAS congruence criterion)
![]() BP = CP and
BP = CP and ![]() APB =
APB = ![]() APC (CPCT)
APC (CPCT)
![]() APB +
APB + ![]() APC = 180
APC = 180![]() (Linear pair)
(Linear pair)
![]() 2
2![]() APB = 180
APB = 180![]() (
(![]() APB =
APB = ![]() APC)
APC)
![]()
![]() APB = 90
APB = 90![]()
Now, BP = CP and ![]() APB = 90
APB = 90![]()
![]() AP is the perpendicular bisector of chord BC.
AP is the perpendicular bisector of chord BC.
![]() AP passes through the centre, O of the circle.
AP passes through the centre, O of the circle.
Solution 5
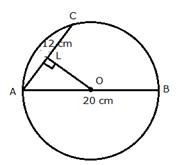
AB is the diameter and AC is the chord.
Draw ![]()
Since ![]() and hence it bisects AC, O is the centre of the circle.
and hence it bisects AC, O is the centre of the circle.
Therefore, OA = 10 cm and AL = 6 cm
Now, in Rt.![]()
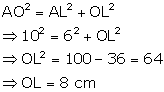
Therefore, chord is at a distance of 8 cm from the centre of the circle.
Solution 6
ABCD is a cyclic quadrilateral in which AD||BC
![]()
(Sum of opposite angles of a quadrilateral)
![]()
Now in ![]()

Now in ![]()
Solution 7
Since ABCD is a cyclic quadrilateral, therefore, ![]() BCD +
BCD + ![]() BAD = 180
BAD = 180![]()
(since opposite angles of a cyclic quadrilateral are supplementary)
![]()
![]() BCD + 70
BCD + 70![]() = 180
= 180![]()
![]()
![]() BCD = 180
BCD = 180![]() - 70
- 70![]() = 110
= 110![]()
In ![]() BCD, we have,
BCD, we have,
![]() CBD +
CBD + ![]() BCD +
BCD + ![]() BDC = 180
BDC = 180![]()
![]() 30
30![]() + 110
+ 110![]() +
+ ![]() BDC = 180
BDC = 180![]()
![]()
![]() BDC = 180
BDC = 180![]() - 140
- 140![]()
![]()
![]() BDC = 40
BDC = 40![]()
Solution 8

ABCD is a cyclic quadrilateral.
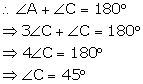
Similarly,

Hence, ![]()
Solution 9

Join AD.
AB is the diameter.
![]() ADB = 90º (Angle in a semi-circle)
ADB = 90º (Angle in a semi-circle)
But, ![]() ADB +
ADB + ![]() ADC = 180º (linear pair)
ADC = 180º (linear pair)
![]()
![]() ADC = 90º
ADC = 90º
In ![]() ABD and
ABD and ![]() ACD,
ACD,
![]() ADB =
ADB = ![]() ADC (each 90º)
ADC (each 90º)
AB = AC (Given)
AD = AD (Common)
![]()
![]() ABD
ABD ![]()
![]() ACD (RHS congruence criterion)
ACD (RHS congruence criterion)
![]() BD = DC (C.P.C.T)
BD = DC (C.P.C.T)
Hence, the circle bisects base BC at D.
Solution 10
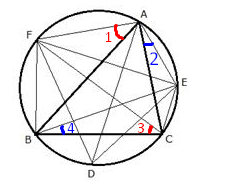
Join ED, EF and DF. Also join BF, FA, AE and EC.
![]()
![]()
In cyclic quadrilateral AFBE,
![]() (Sum of opposite angles)
(Sum of opposite angles)
Similarly in cyclic quadrilateral CEAF,
![]()
Adding (ii) and (iii)

![]()


Solution 11
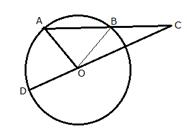
Join OB.
BC = OD ... (given)
OD = OB ... (radii of the same circle)
Hence,
BC = OB
In ![]()

In ![]()

Since DOC is a straight-line
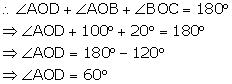
Solution 12
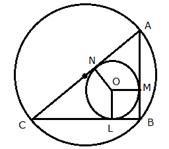
Join OL, OM and ON.
Let D and d be the diameter of the circumcircle and incircle.
and let R and r be the radius of the circumcircle and incircle.
In circumcircle of ![]()
![]()
Therefore, AC is the diameter of the circumcircle i.e. AC = D
Let radius of the incircle = r
![]()
Now, from B, BL, BM are the tangents to the incircle.

(Tangents from the point outside the circle)
Now,
AB+BC+CA = AM+BM+BL+CL+CA
= AN+r+r+CN+CA
= AN+CN+2r+CA
= AC+AC+2r
= 2AC+2r
= 2D+d
Solution 13
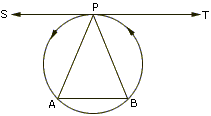
Join AP and BP.
Since TPS is a tangent and PA is the chord of the circle.
![]() (angles in alternate segments)
(angles in alternate segments)
But
arc PA = arc PB,
hence PA = PB
![]()
But these are alternate angles
![]()
Solution 14
From P, AP is the tangent and PMN is the secant for first circle.
![]()
Again from P, PB is the tangent and PMN is the secant for second circle.
![]()
From (i) and (ii)
![]()
Therefore, P is the midpoint of AB.
Solution 15
i) PQ is tangent and CD is a chord
![]() (angles in the alternate segment)
(angles in the alternate segment)
![]()
ii)
iii) In ![]()

Solution 16
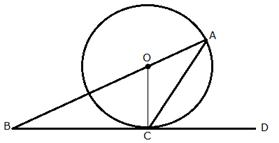
Join OC.
BCD is the tangent and OC is the radius.
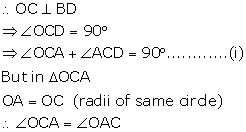
Substituting in (i)
![]()
Solution 17
i) In ![]()
![]() and BC is the diameter of the circle.
and BC is the diameter of the circle.
Therefore, AB is the tangent to the circle at B.
Now, AB is tangent and ADC is the secant
![]()
Now,
∠BDC = ∠BDA = 90o ... angle subtended by a diameter
ii) In ![]()
From (i) and (ii)
![]()
Now in ![]()


Solution 18
In ![]()
![]() (angles in the same segment)
(angles in the same segment)
AC = AE (Given)
![]() (Common)
(Common)
![]() (ASA Postulate)
(ASA Postulate)
![]() AB = AD
AB = AD
but AC = AE
![]()
In ![]()
![]() (angles in the same segment)
(angles in the same segment)
BC = DE
![]() (angles in the same segment)
(angles in the same segment)
![]() (ASA Postulate)
(ASA Postulate)
![]() BP = DP and CP = PE (cpct)
BP = DP and CP = PE (cpct)
Solution 19
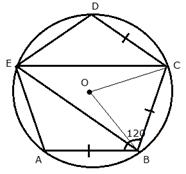
i) Join OC and OB.
AB = BC = CD and ![]()
OA = OD = OC = OB all are radii of the circle
Hence,
All the 3 triangles are congruent
So,
∠AOB = ∠DOC = ∠COB [By C.P.C.T.C]
OB and OC are the bisectors of ![]() and
and ![]() respectively.
respectively.
![]()
In ![]()
Hence,
∠AOB = ∠DOC = ∠COB = 60o
Arc BC subtends ![]() at the centre and
at the centre and ![]() at the remaining part of the circle.
at the remaining part of the circle.
![]()
ii)
Similarly,
∠BED = 0.5 ∠BOD = 60o
Solution 20
In the given fig, O is the centre
of the circle and CA and CB are the tangents to the circle from C. Also, ![]() ACO = 30
ACO = 30![]()
P is any point on the circle. P and PB are joined.
To find: (i) ![]()
(ii) ![]()
(iii)![]()
Proof:

Solution 21

Given: ABC is a triangle with AB = 10 cm, BC= 8 cm, AC = 6 cm. Three circles are drawn with centre A, B and C touch each other at P, Q and R respectively.
We need to find the radii of the three circles.

Solution 22
ABCD is a square whose diagonals AC and BD intersect each other at right angles at O.
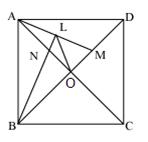
i) ![]()
In ![]()
But, ![]() (vertically opposite angles)
(vertically opposite angles)
![]()
Now in ![]()
Adding (i) and (ii)
ii)

and
![]()
In quadrilateral ALOB,
![]()
Therefore, ALOB is a cyclic quadrilateral.
iii)

In triangle ALB
∠ALB = 180 - ∠ABL - ∠BAL
=180 - 22.5 - 67.5
∠ALB = 90°
∠ALB = ∠AOB ...(Each 90°)
If a line segment joining two points subtends equal angles at two other points lying on the same side of the line containing the line segment, then the four points lie on a circle
(i.e. they are concyclic).
Hence, ALOB is a cyclic quadrilateral.
Solution 23
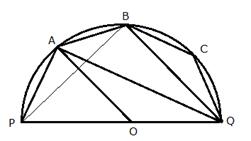
Join PB.
i) In cyclic quadrilateral PBCQ,
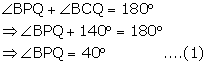
Now in ![]()
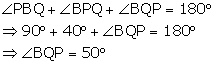
In cyclic quadrilateral PQBA,
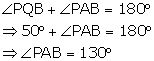
ii) Now in ![]()

![]()
iii) Arc AQ subtends ![]() at the centre and
at the centre and ![]() APQ at the remaining
part of the circle.
APQ at the remaining
part of the circle.
We have,
![]()
From (1), (2) and (3), we have
![]()
![]()
Now in ![]()
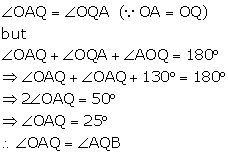
But these are alternate angles.
Hence, AO is parallel to BQ.
Solution 24
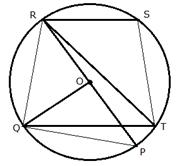
Join PQ, RQ and ST.
i)
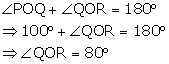
Arc RQ subtends ![]() at the centre and
at the centre and ![]() QTR at the remaining part of the circle.
QTR at the remaining part of the circle.
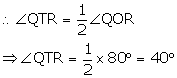
ii) Arc QP subtends ![]() at the centre and
at the centre and ![]() QRP at the remaining part of the circle.
QRP at the remaining part of the circle.
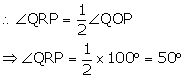
iii) RS || QT
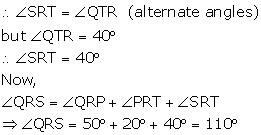
iv) Since RSTQ is a cyclic quadrilateral
![]() (sum of opposite angles)
(sum of opposite angles)
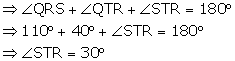
Solution 25
i) Since PAT||BC
![]() (alternate angles) .........(i)
(alternate angles) .........(i)
In cyclic quadrilateral ABCD,
![]()
from (i) and (ii)
![]()
ii) Arc AB subtends ![]() at the centre and
at the centre and ![]() at the remaining part of the circle.
at the remaining part of the circle.

iii)
Solution 26
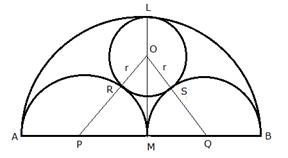
Let O, P and Q be the centers of the circle and semicircles.
Join OP and OQ.
OR = OS = r
and AP = PM = MQ = QB = ![]()
Now, OP = OR + RP = r + ![]() (since PM=RP=radii of same circle)
(since PM=RP=radii of same circle)
Similarly, OQ = OS + SQ = r + ![]()
OM =  - r ; OL =
- r ; OL = ![]() - r
- r
Now in Rt. ![]()
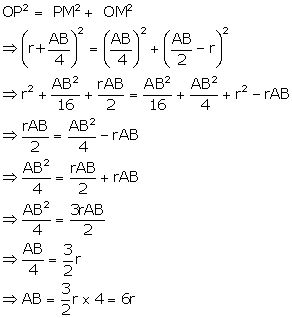
Hence AB = 6 x r
Solution 27
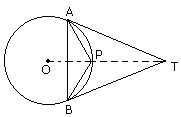
Join PB.
In ![]() TAP and
TAP and ![]() TBP,
TBP,
TA = TB (tangents segments from an external points are equal in length)
Also, ![]() ATP =
ATP = ![]() BTP. (since OT is equally inclined with TA and TB) TP = TP (common)
BTP. (since OT is equally inclined with TA and TB) TP = TP (common)
![]()
![]() TAP
TAP ![]()
![]() TBP (by SAS criterion of congruency)
TBP (by SAS criterion of congruency)
![]()
![]() TAP =
TAP = ![]() TBP (corresponding parts of congruent triangles are equal)
TBP (corresponding parts of congruent triangles are equal)
But ![]() TBP =
TBP = ![]() BAP (angles in alternate segments)
BAP (angles in alternate segments)
Therefore, ![]() TAP =
TAP = ![]() BAP.
BAP.
Hence, AP bisects ![]() TAB.
TAB.
Solution 28
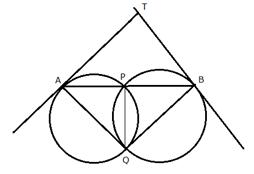
Join PQ.
AT is tangent and AP is a chord.
![]() (angles in alternate segments) ........(i)
(angles in alternate segments) ........(i)
Similarly, ![]() .......(ii)
.......(ii)
Adding (i) and (ii)
![]()
Now in ![]()
![]()
Therefore, AQBT is a cyclic quadrilateral.
Hence, A, Q, B and T lie on a circle.
Solution 29
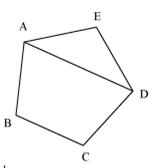
ABCDE is a regular pentagon.
![]()
In ![]() AED,
AED,
AE = ED (Sides of regular pentagon ABCDE)
![]()
![]() EAD =
EAD = ![]() EDA
EDA
In ![]() AED,
AED,
![]() AED +
AED + ![]() EAD +
EAD + ![]() EDA = 180º
EDA = 180º
![]() 108º +
108º + ![]() EAD +
EAD + ![]() EAD = 180º
EAD = 180º
![]() 2
2![]() EAD = 180º - 108º = 72º
EAD = 180º - 108º = 72º
![]()
![]() EAD = 36º
EAD = 36º
![]()
![]() EDA = 36º
EDA = 36º
![]() BAD =
BAD = ![]() BAE -
BAE - ![]() EAD = 108º - 36º = 72º
EAD = 108º - 36º = 72º
In quadrilateral ABCD,
![]() BAD +
BAD + ![]() BCD = 108º + 72º = 180º
BCD = 108º + 72º = 180º
![]() ABCD is a cyclic quadrilateral
ABCD is a cyclic quadrilateral
Solution 30
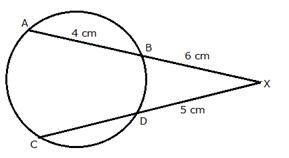
We know that XB.XA = XD.XC
Or, XB.(XB + BA) = XD.(XD + CD)
Or, 6(6 + 4) = 5(5 + CD)
Or, 60 = 5(5 + CD)
Or, 5 + CD = ![]() = 12
= 12
Or, CD = 12 - 5 = 7 cm.
Solution 31
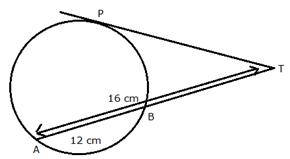
PT is the tangent and TBA is the secant of the circle.
Therefore, TP2 = TA x TB
TP2 = 16 x (16-12) = 16 x 4 = 64 = (8)2
Therefore, TP = 8 cm
Solution 32
From the figure we see that BQ = BR = 27 cm (since length of the tangent segments from an
external point are equal)
As BC = 38 cm
![]() CR = CB - BR = 38 - 27
CR = CB - BR = 38 - 27
= 11 cm
Again,
CR = CS = 11cm (length of tangent segments from an external point are equal)
Now, as DC = 25 cm
![]() DS = DC - SC
DS = DC - SC
= 25 -11
= 14 cm
Now, in quadrilateral DSOP,
![]() PDS = 90
PDS = 90![]() (given)
(given)
![]() OSD = 90
OSD = 90![]() ,
, ![]() OPD = 90
OPD = 90![]() (since tangent is perpendicular to the
(since tangent is perpendicular to the
radius through the point of contact)
![]() DSOP is a parallelogram
DSOP is a parallelogram
![]() OP||SD and
OP||SD and ![]() PD||OS
PD||OS
Now, as OP = OS (radii of the same circle)
![]() OPDS is a square.
OPDS is a square. ![]() DS = OP = 14cm
DS = OP = 14cm
![]() radius of the circle = 14 cm
radius of the circle = 14 cm
Solution 33

In ![]() AXB,
AXB,
![]() XAB +
XAB + ![]() AXB +
AXB + ![]() ABX=180
ABX=180![]() [Triangle property]
[Triangle property]
![]()
![]() XAB + 50
XAB + 50![]() + 70
+ 70![]() = 180
= 180![]()
![]()
![]() XAB=180
XAB=180![]() - 120
- 120![]() = 60
= 60![]()
![]()
![]() XAY=90
XAY=90![]() [Angle of semi-circle]
[Angle of semi-circle]
![]()
![]() BAY=
BAY=![]() XAY -
XAY -![]() XAB = 90
XAB = 90![]() - 60
- 60![]() = 30
= 30![]()
and ![]() BXY =
BXY = ![]() BAY = 30
BAY = 30![]() [Angle of same segment]
[Angle of same segment]
![]()
![]() ACX =
ACX = ![]() BXY +
BXY + ![]() ABX [External angle = Sum of two interior angles]
ABX [External angle = Sum of two interior angles]
= 30![]() + 70
+ 70![]()
= 100![]()
also,
![]() XYP=90
XYP=90![]() [Diameter ⊥ tangent]
[Diameter ⊥ tangent]
![]() APY =
APY = ![]() ACX -
ACX -![]() CYP
CYP
![]() APY=100
APY=100![]() - 90
- 90![]()
![]() APY=10
APY=10![]()
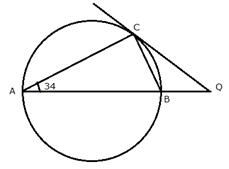
Solution 34
PAQ is a tangent and AB is a chord of the circle.
i) ![]() (angles in alternate segment)
(angles in alternate segment)
ii) In ![]()
![]()
iii) ![]() (angles in the same
segment)
(angles in the same
segment)
Now in ![]()
![]()
iv) PAQ is the tangent and AD is chord
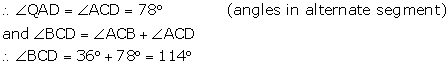
Solution 35
i) AB is the diameter of the circle.
![]()
In ![]()

ii) QC is tangent to the circle
![]()
The angle between tangent and chord = angle in the alternate segment
![]()
ABQ is a straight-line
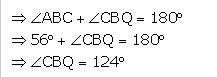
In ΔCBQ
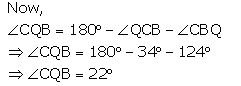
Solution 36
i)

![]()
ii) Since, BPDO is a quadrilateral,

Solution 37
i) PQ = RQ
![]() (opposite angles of equal sides
of a triangle)
(opposite angles of equal sides
of a triangle)
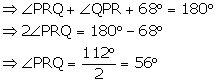
Now, ![]() QOP = 2
QOP = 2![]() PRQ (angle at the
centre is double)
PRQ (angle at the
centre is double)
![]()
ii) ![]() PQC =
PQC = ![]() PRQ (angles in
alternate segments are equal)
PRQ (angles in
alternate segments are equal)
![]() QPC =
QPC = ![]() PRQ (angles in
alternate segments)
PRQ (angles in
alternate segments)
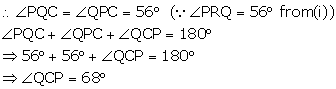
Solution 38
Consider two concentric circles with centres at O. Let AB and CD be two chords of the outer circle which touch the inner circle at the points M and N respectively.
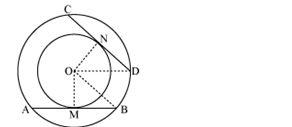
To prove the given question, it is sufficient to prove AB = CD.
For this join OM, ON, OB and OD.
Let the radius of outer and inner circles be R and r respectively.
AB touches the inner circle at M.
![]() AB is a tangent to the inner circle
AB is a tangent to the inner circle
![]() OM
OM![]() AB
AB
![]() BM =
BM = ![]() AB
AB
![]() AB = 2BM
AB = 2BM
Similarly ON![]() CD, and CD = 2DN
CD, and CD = 2DN
Using Pythagoras' theorem in ![]() OMB and
OMB and ![]() OND
OND
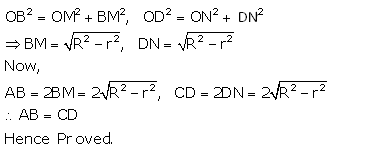
Solution 39
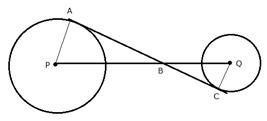
Since AC is tangent to the circle with center P at point A.
![]()
In ![]()
Also in Rt.![]()
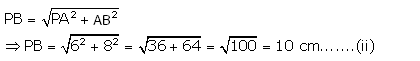
From (i) and (ii),
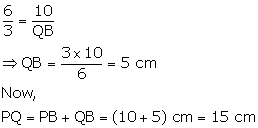
Solution 40
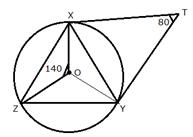
In the figure, a circle with centre O, is the circumcircle of triangle XYZ.
![]()
Tangents at X and Y intersect at point T, such that ![]() XTY = 80o
XTY = 80o
Since a tangent at any point of a circle is perpendicular to the radius at the point of contact,
we have ∠OXT = ∠OYT = 90o
So in quadrilateral XOYT
Sum of angles of a quadrilateral is 360 degrees,
∠XOY = 360 − (90 + 90 + 80)
Solution 41
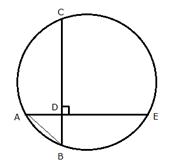
From Rt. ![]()
![]()
Now, since the two chords AE and BC intersect at D,
AD x DE = CD x DB
3 x DE = 9 x 4
![]()
Hence, AE = AD + DE = (3 + 12) = 15 cm
Solution 42

Solution 43


