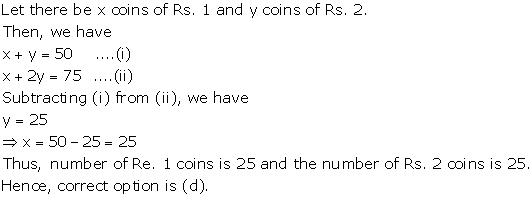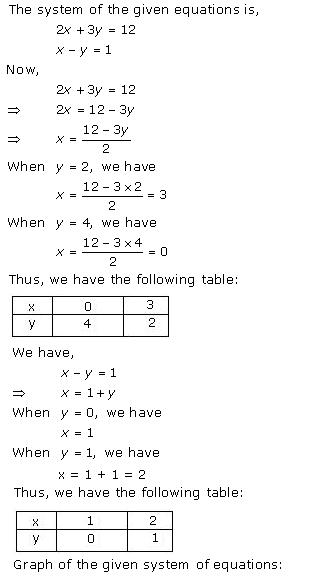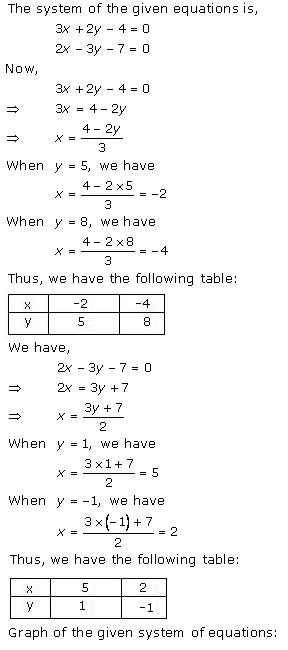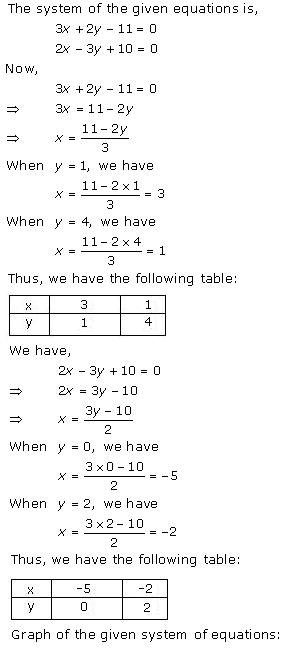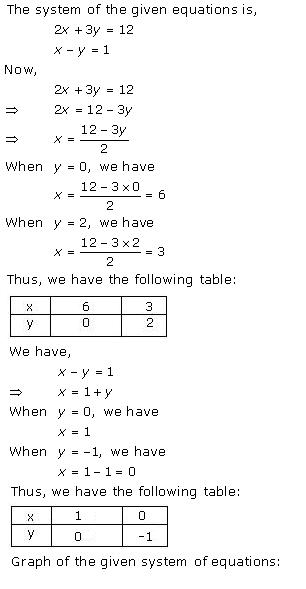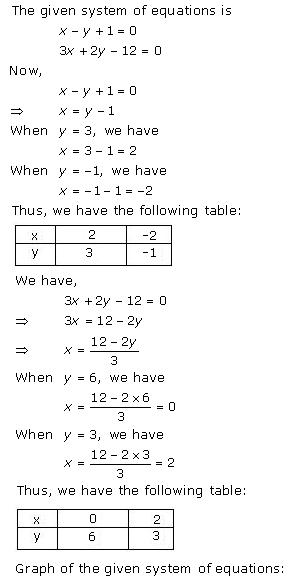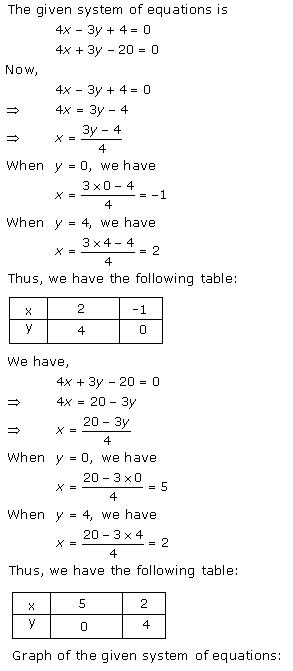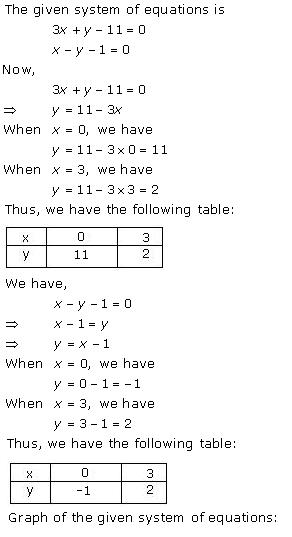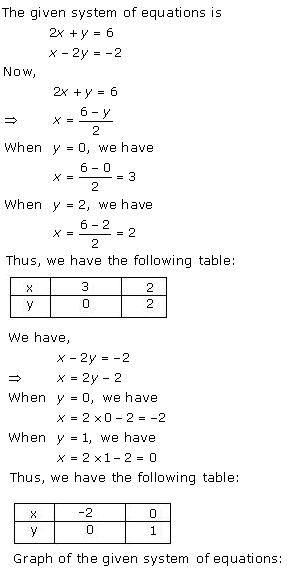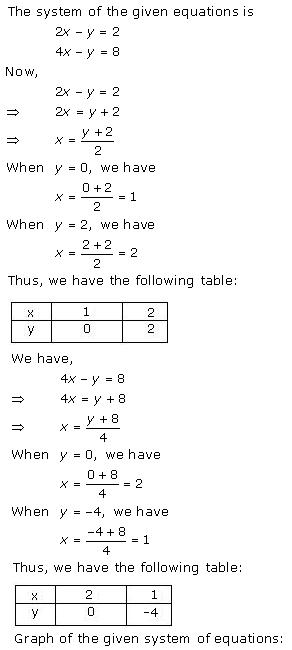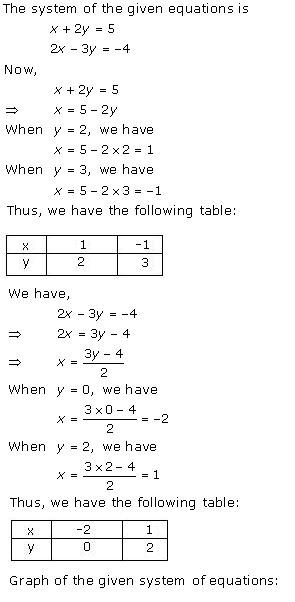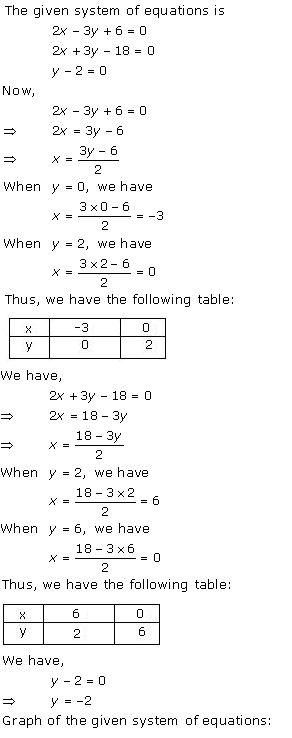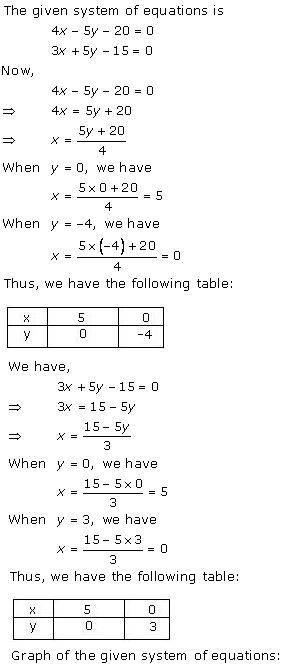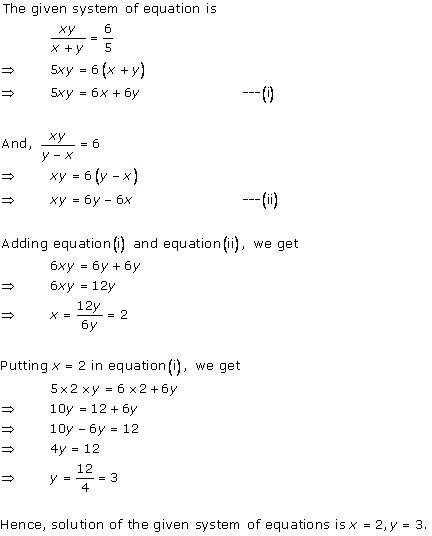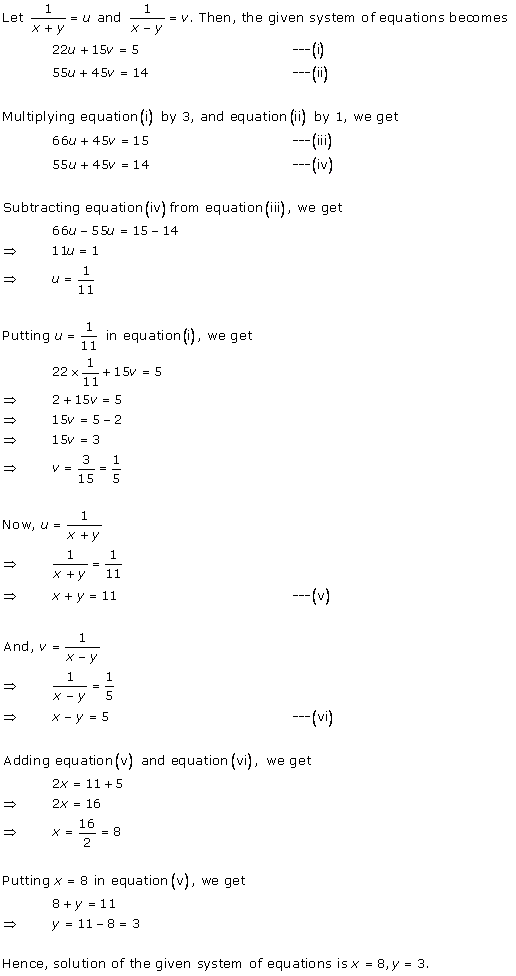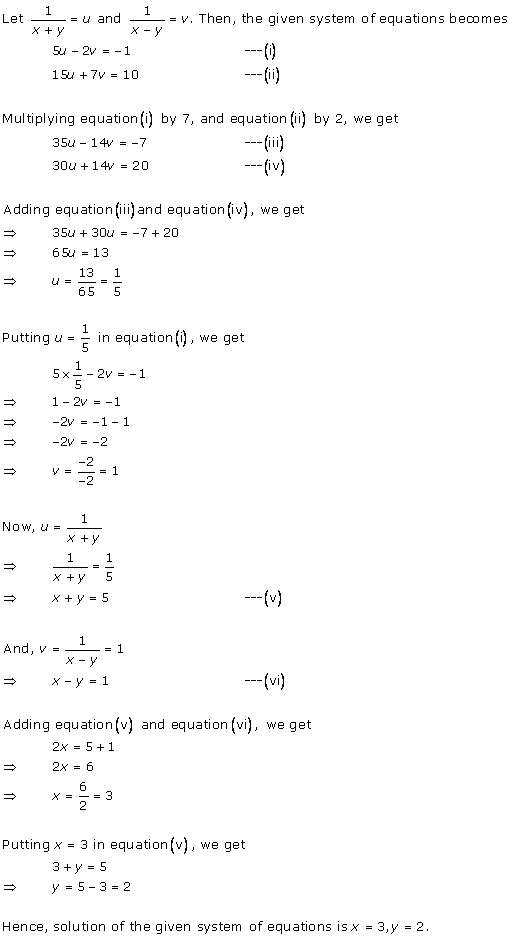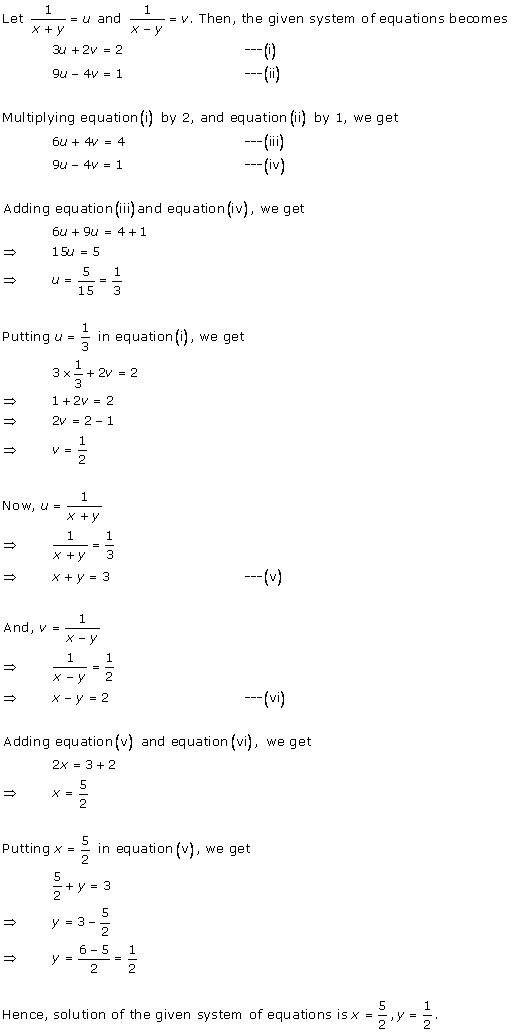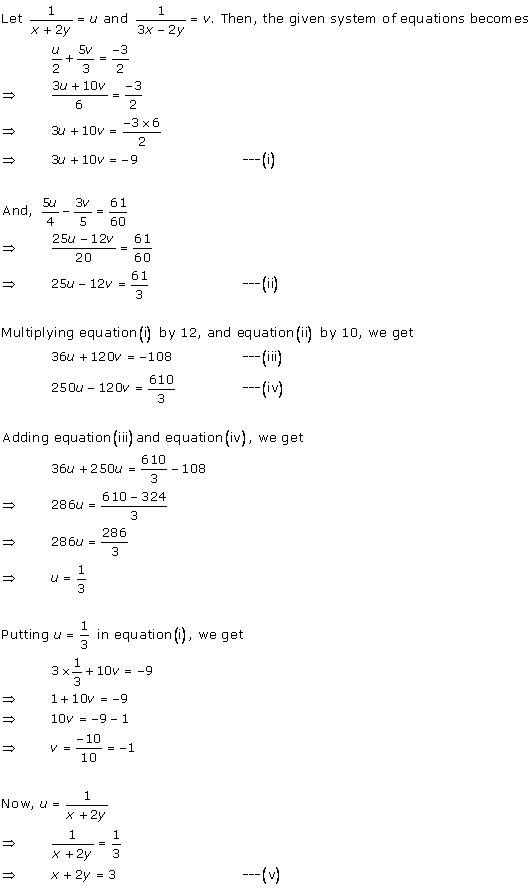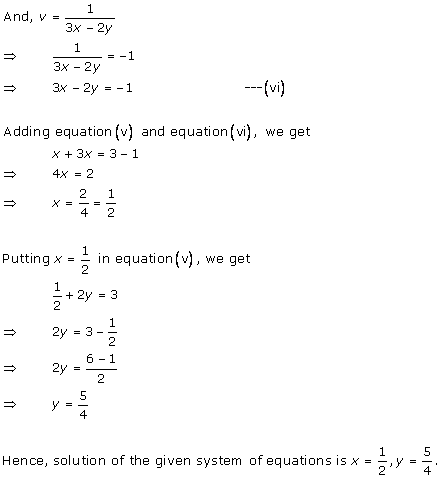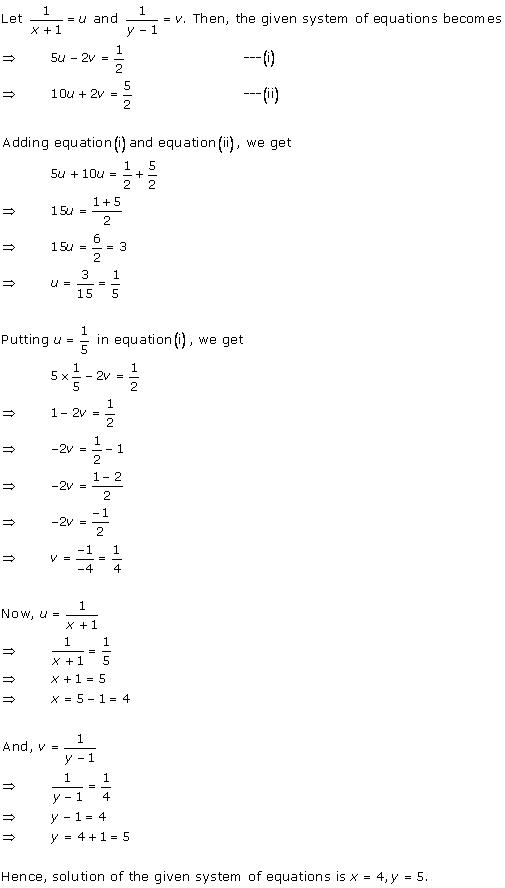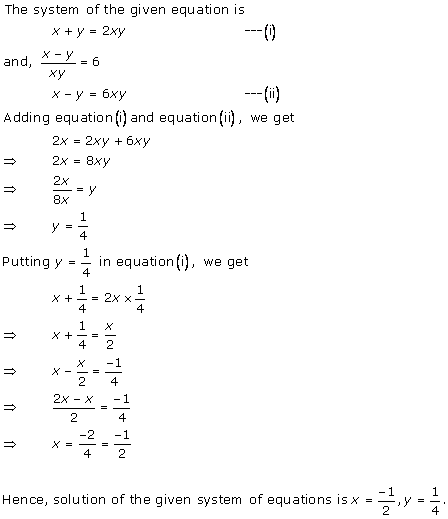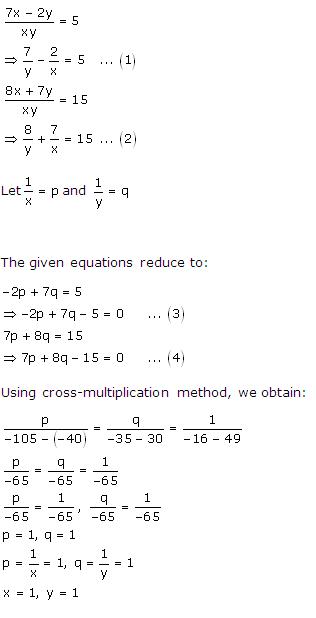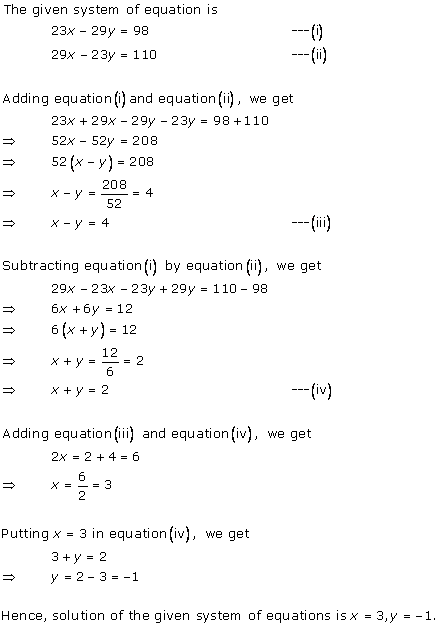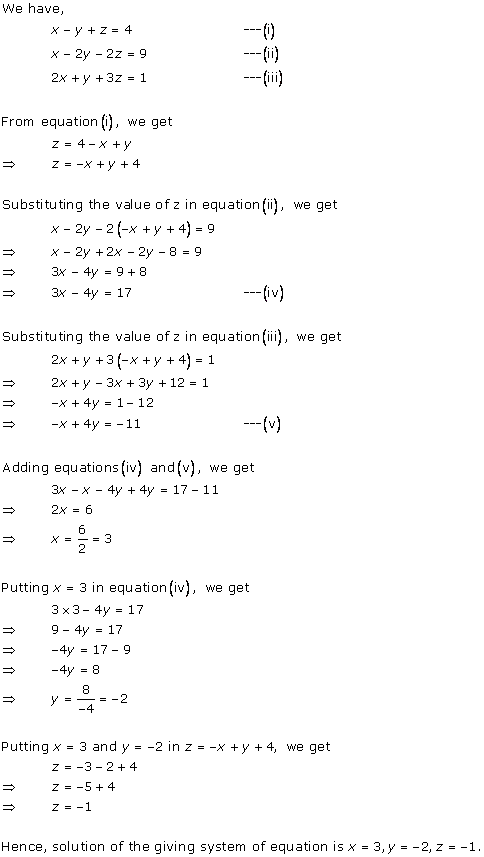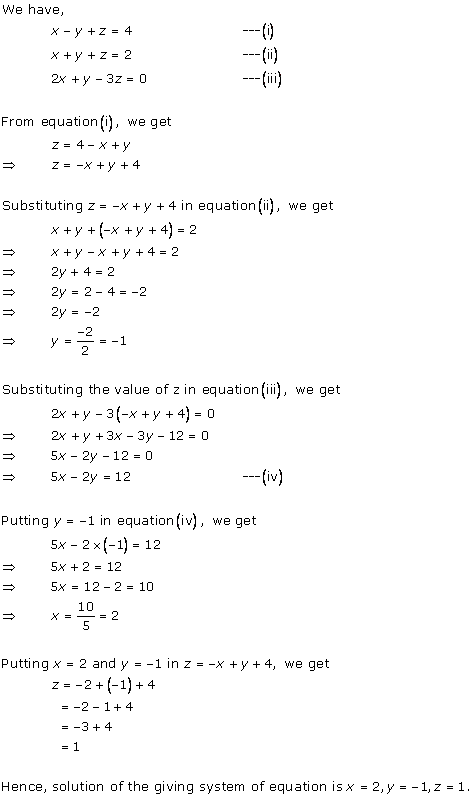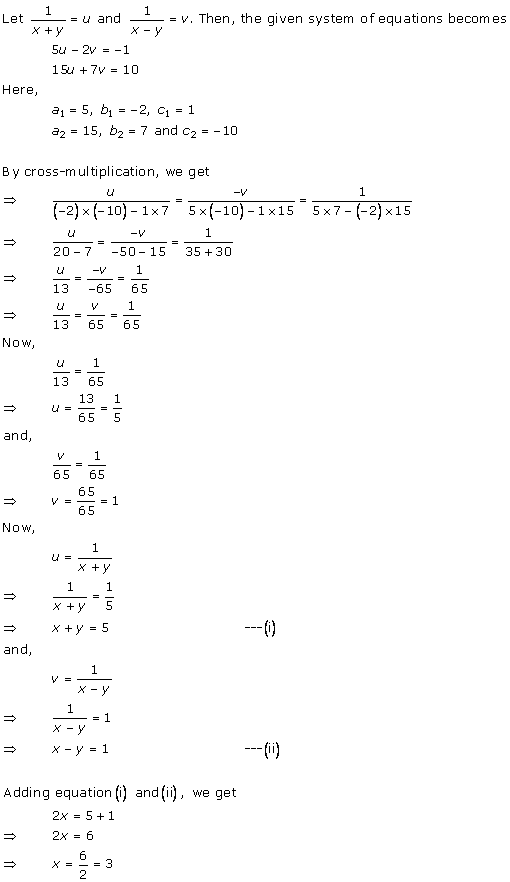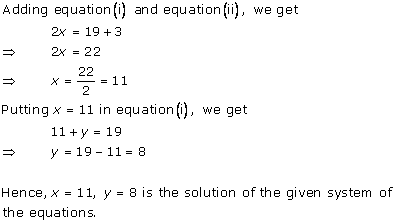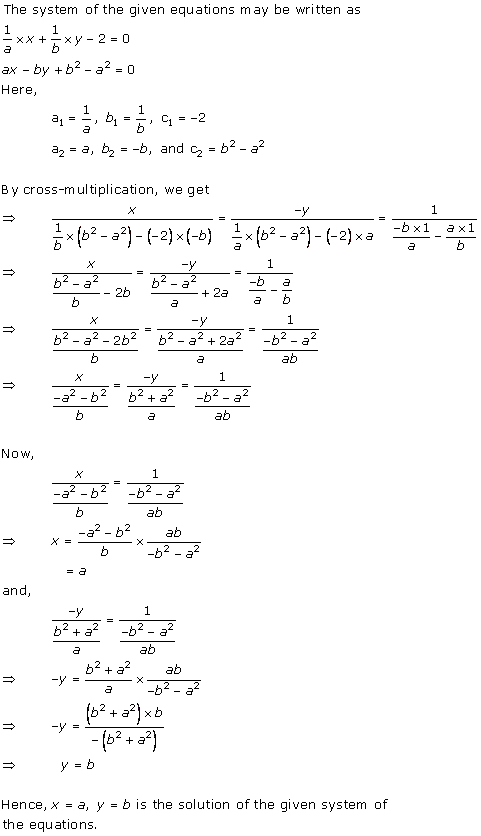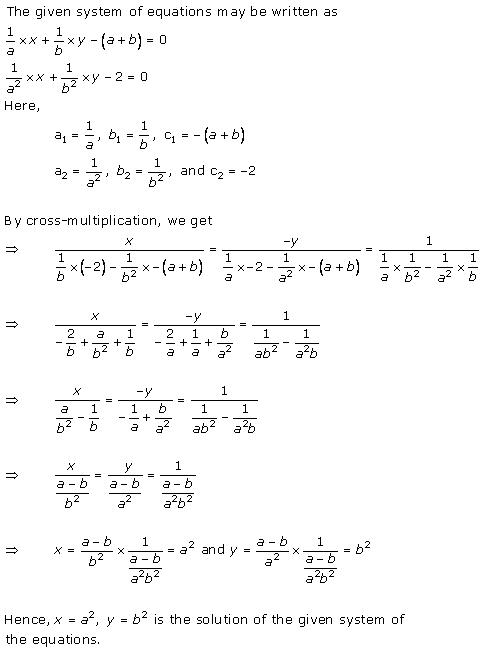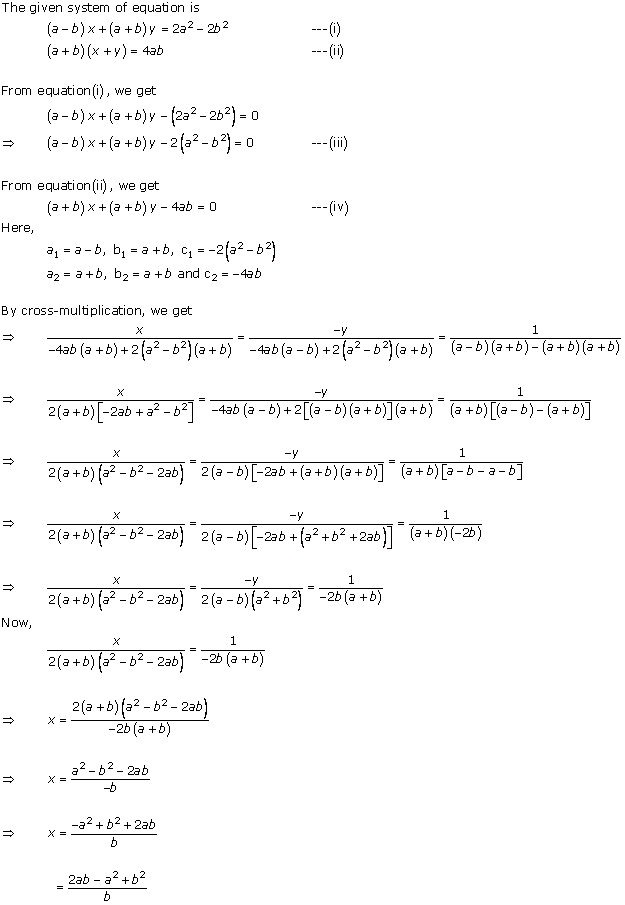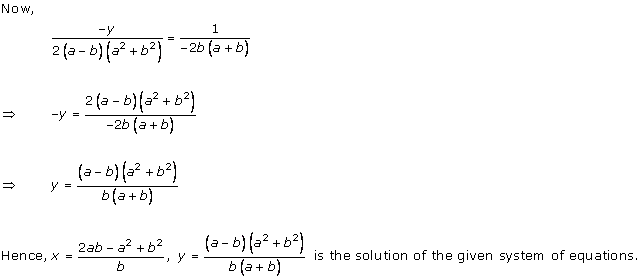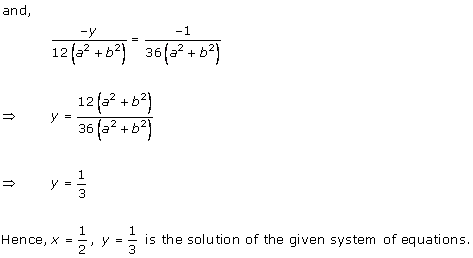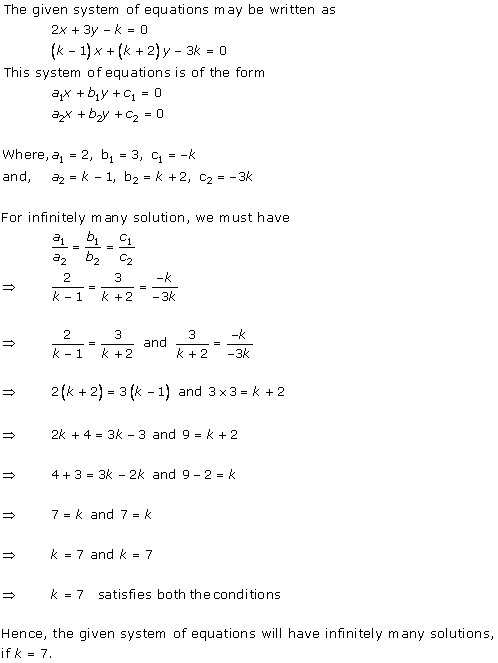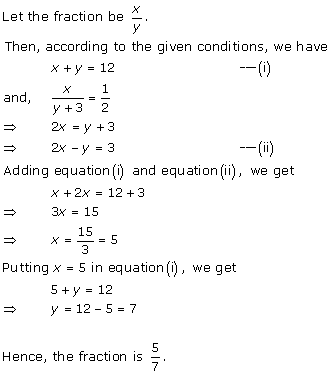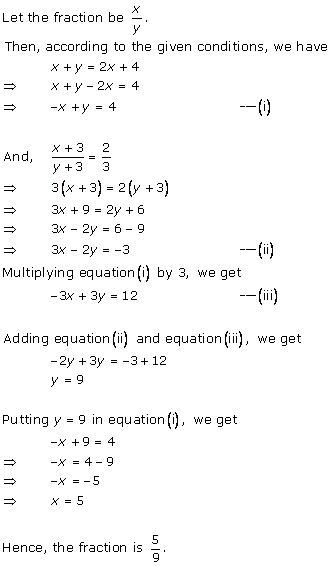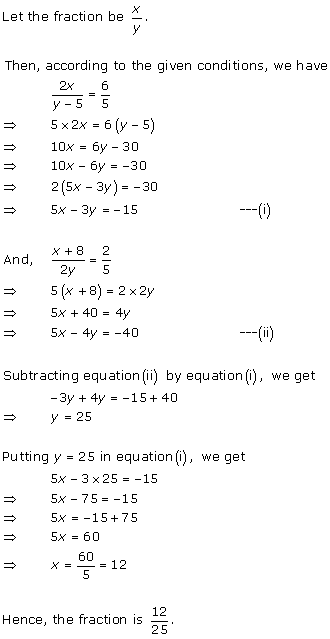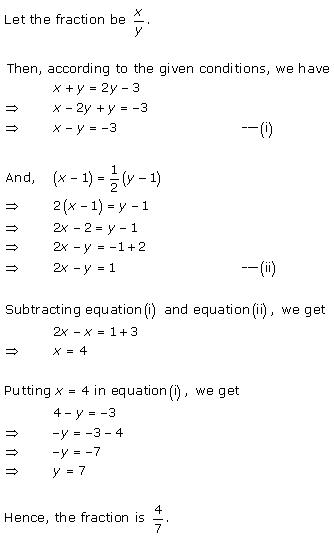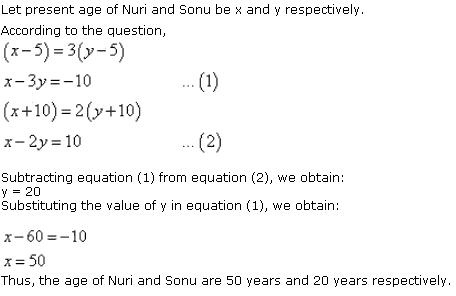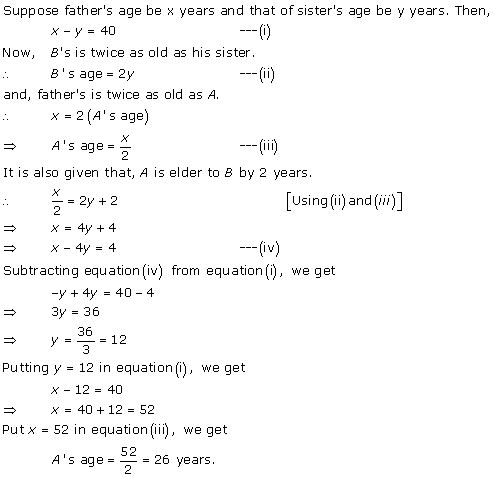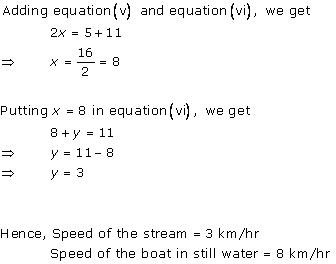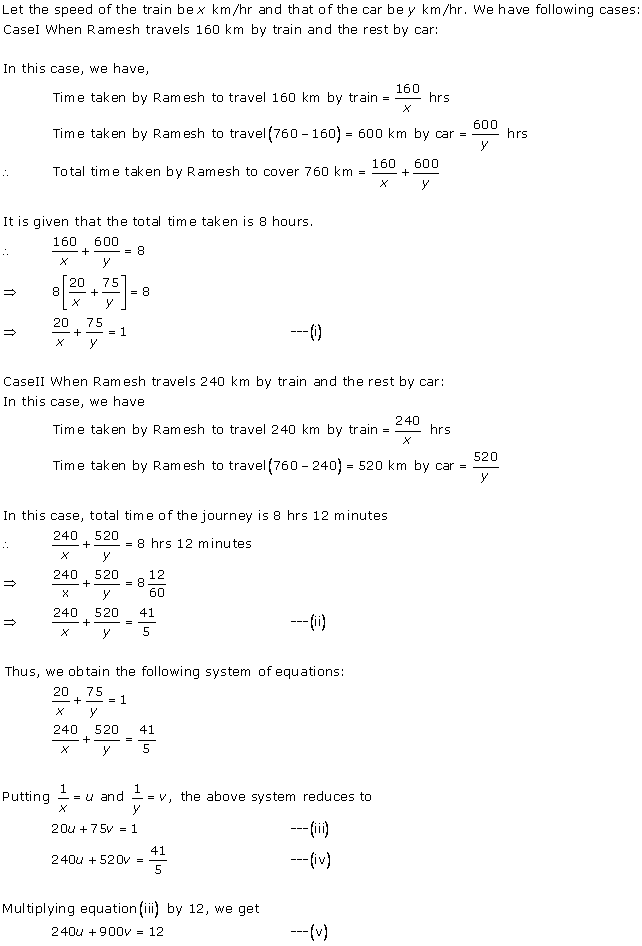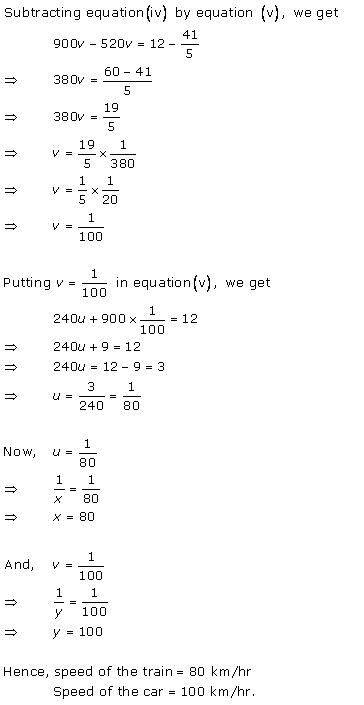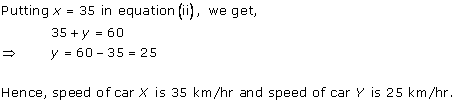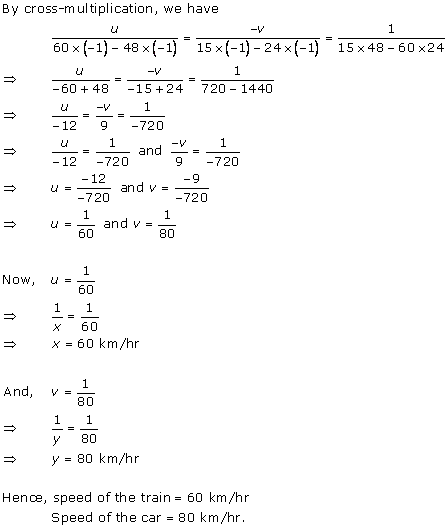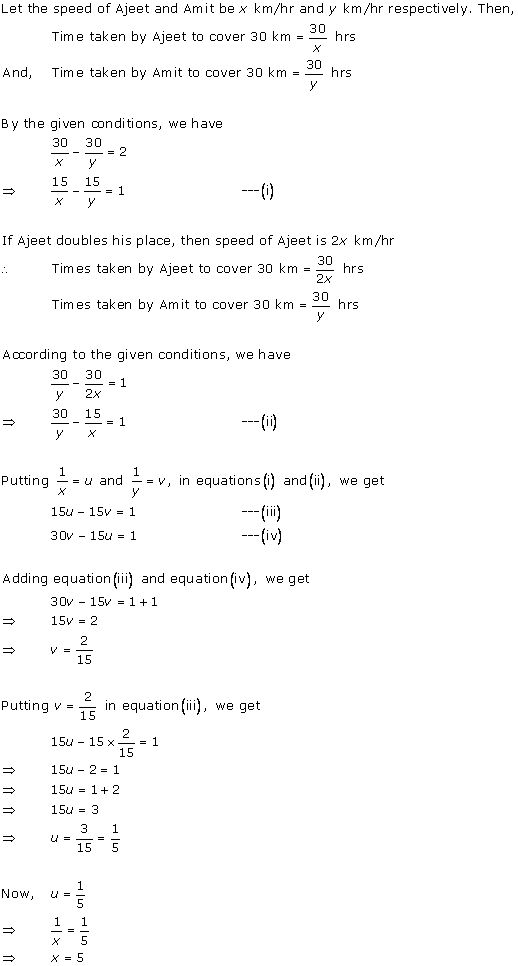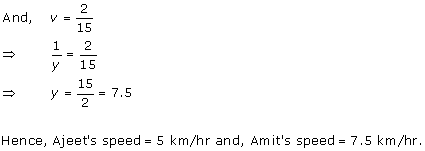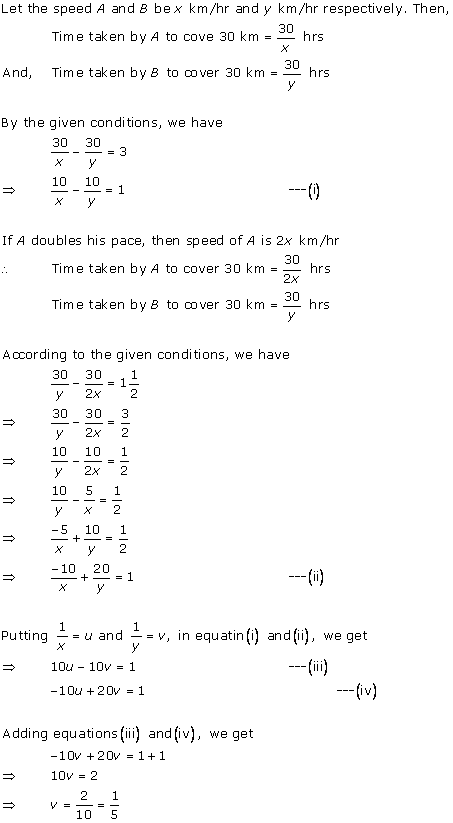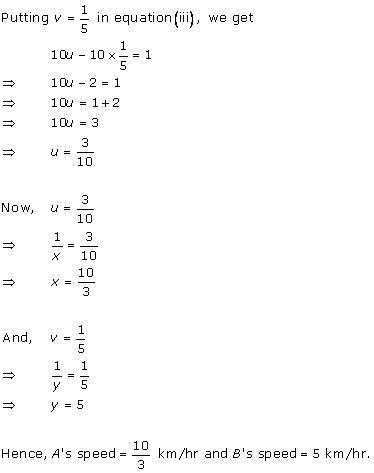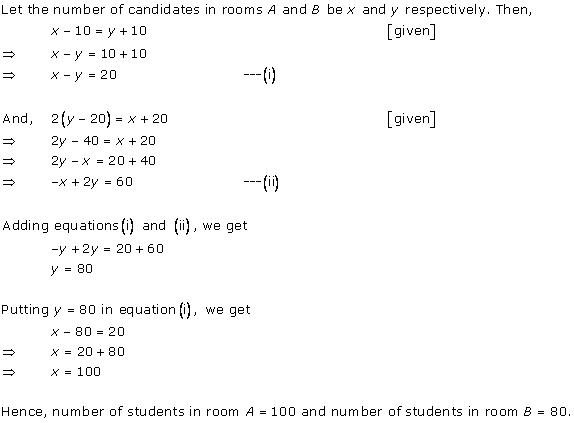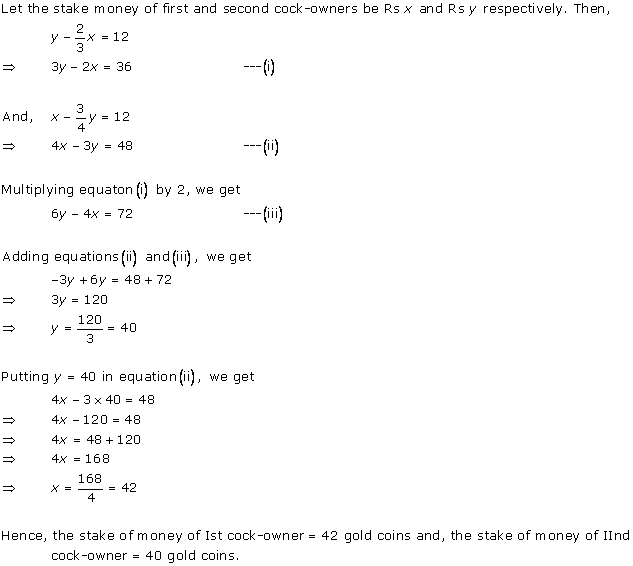Class 10 RD SHARMA Solutions Maths Chapter 3 - Pairs of Linear Equations in Two Variables
Pairs of Linear Equations in Two Variables Exercise Ex. 3.1
Solution 1
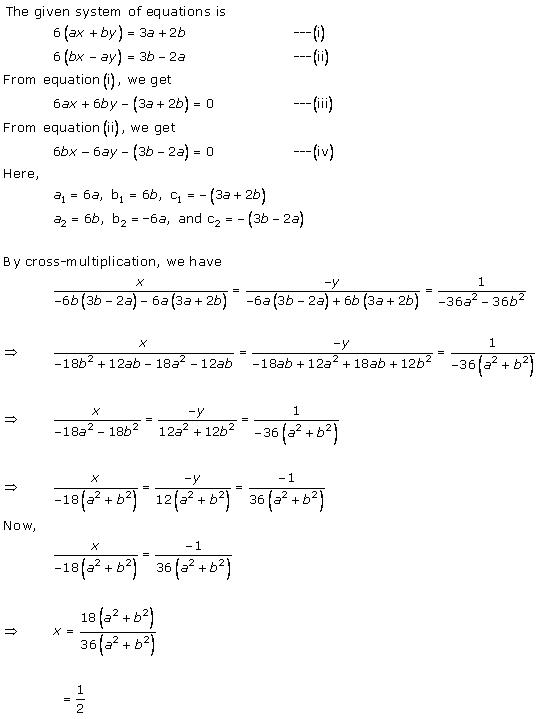
Solution 2
Seven years ago,
Age of Aftab = x - 7
Age of his daughter = y - 7
According to the given condition,
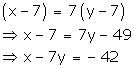
Three years hence,
Age of Aftab = x + 3
Age of his daughter = y + 3
According to the given condition,
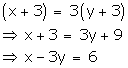
Thus, the given conditions can be algebraically represented as:
x - 7y = -42
x - 3y = 6
Three solutions of this equation can be written in a table as follows:
| x | -7 | 0 | 7 |
| y | 5 | 6 | 7 |
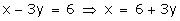
Three solutions of this equation can be written in a table as follows:
| x | 6 | 3 | 0 |
| y | 0 | -1 | -2 |
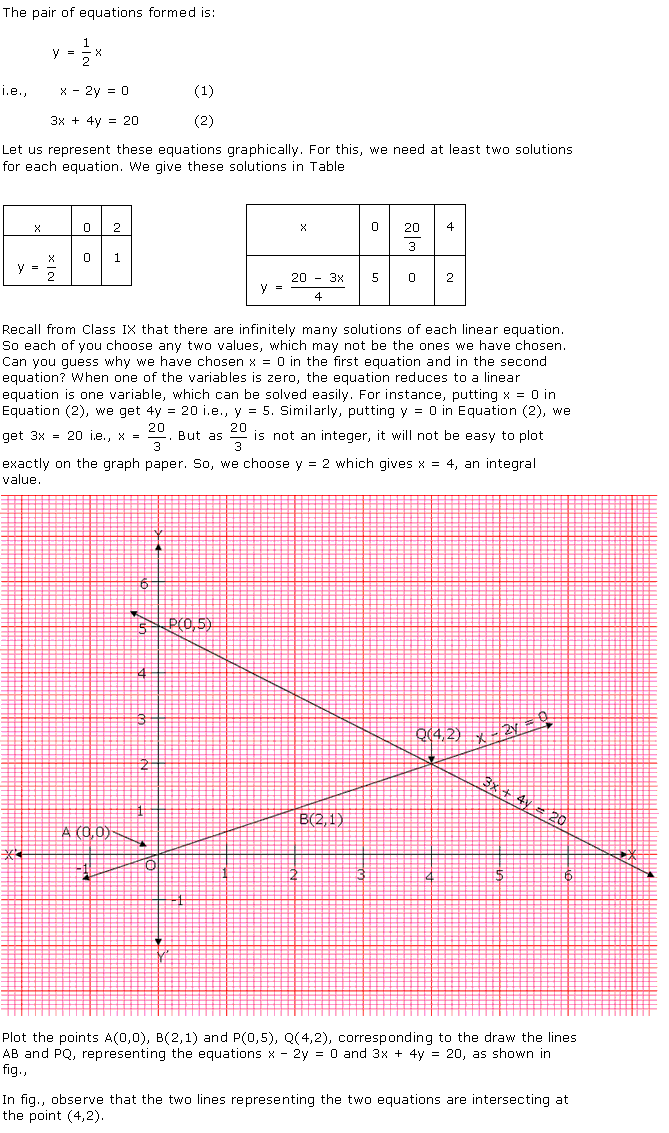
The graphical representation is as follows:
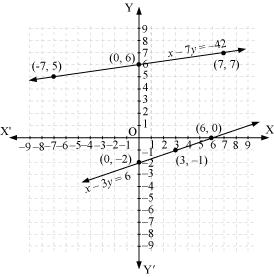
Concept insight: In order to represent a given situation mathematically, first see what we need to find out in the problem. Here, Aftab and his daughter's present age needs to be found so, so the ages will be represented by variables x and y. The problem talks about their ages seven years ago and three years from now. Here, the words 'seven years ago' means we have to subtract 7 from their present ages, and 'three years from now' or 'three years hence' means we have to add 3 to their present ages. Remember in order to represent the algebraic equations graphically the solution set of equations must be taken as whole numbers only for the accuracy. Graph of the two linear equations will be represented by a straight line.
Solution 3
Solution 4
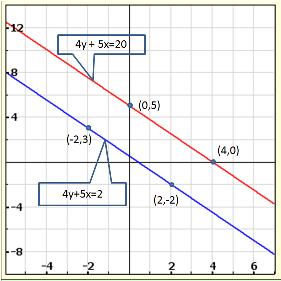
Solution 5(i)
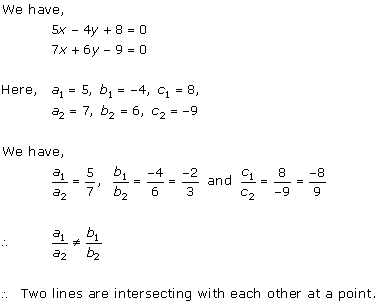
Solution 5(ii)
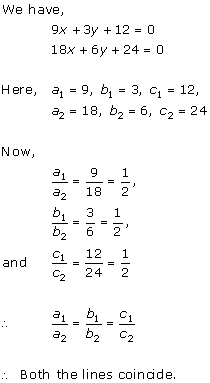
Solution 5(iii)
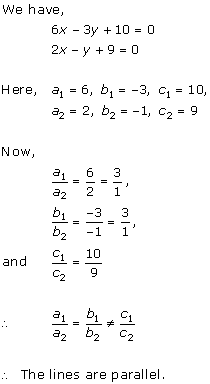
Solution 6(i)
Solution 6(ii)
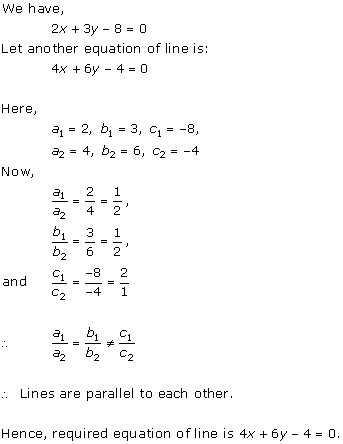
Solution 6(iii)
Solution 7
The given conditions can be algebraically represented as:
Three solutions of this equation can be written in a table as follows:
x |
50 | 60 | 70 |
| y | 60 | 40 | 20 |
Three solutions of this equation can be written in a table as follows:
x |
70 | 80 | 75 |
| y | 10 |
-10 |
0 |
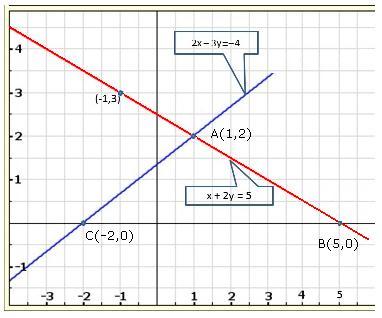
The graphical representation is as follows:
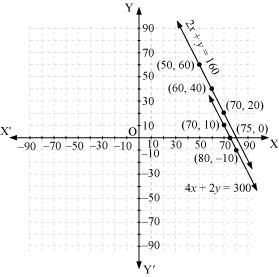
Concept insight: cost of apples and grapes needs to be found so the cost of 1 kg apples and 1 kg grapes will be taken as the variables. From the given conditions of collective cost of apples and grapes, a pair of linear equations in two variables will be obtained. Then, in order to represent the obtained equations graphically, take the values of variables as whole numbers only. Since these values are large so take the suitable scale.
Pairs of Linear Equations in Two Variables Exercise Ex. 3.2
Solution 1
Solution 2
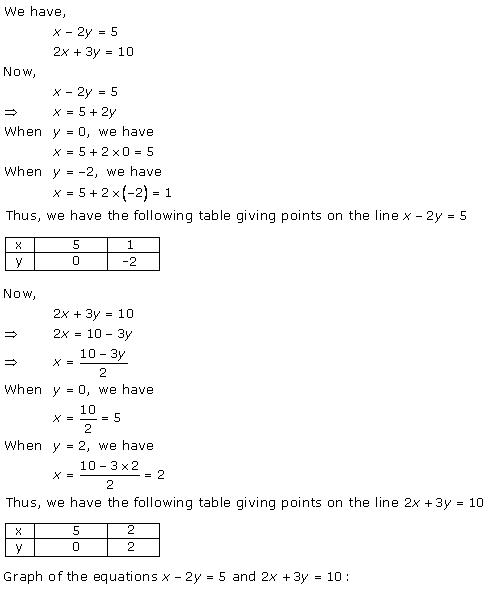
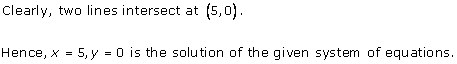
Solution 3
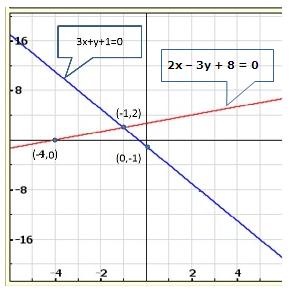
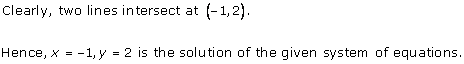
Solution 4
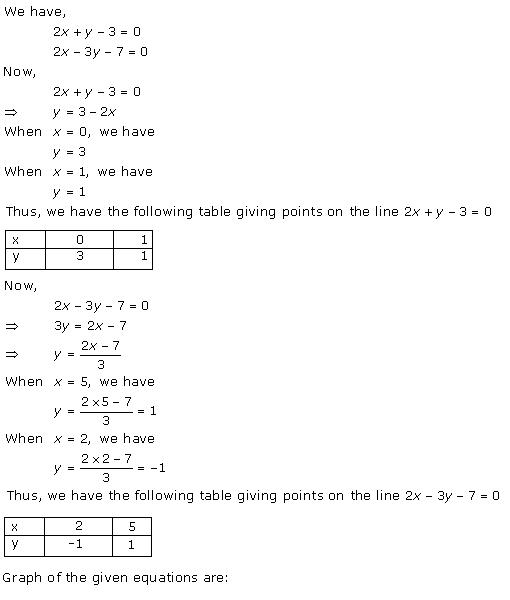
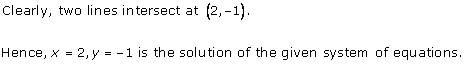
Solution 5
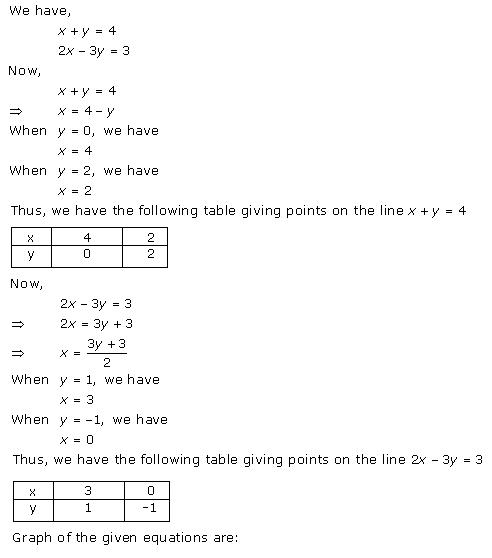
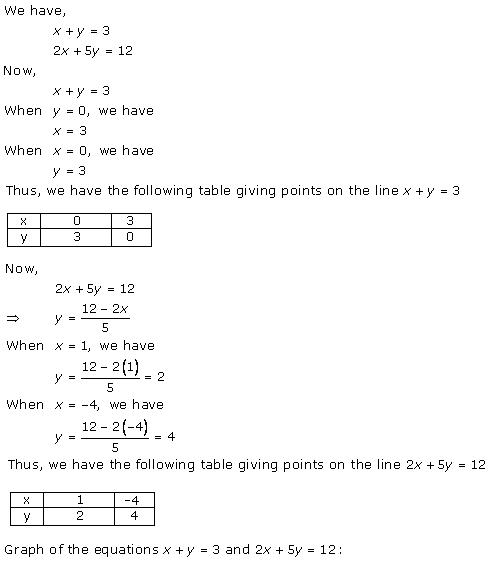
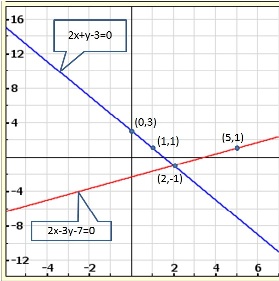
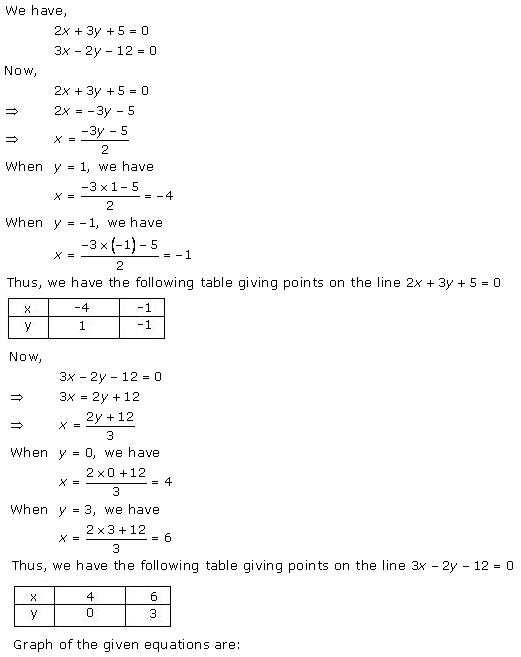
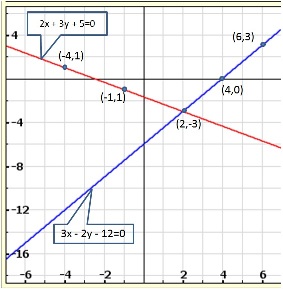
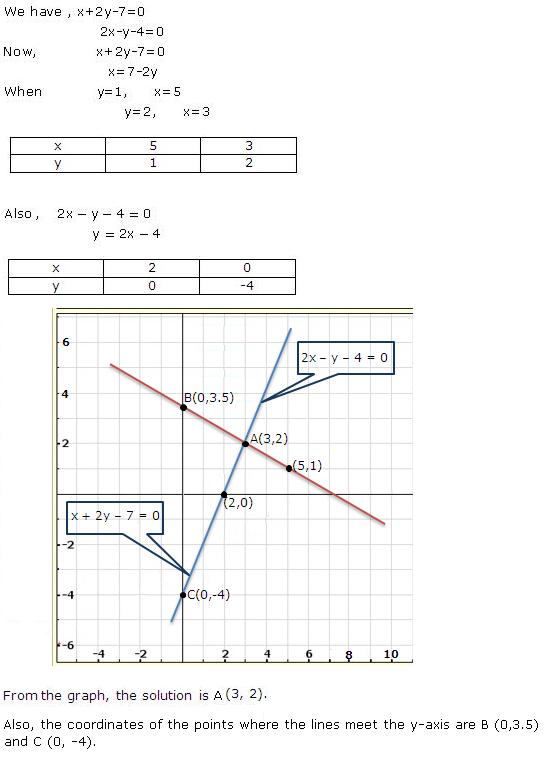
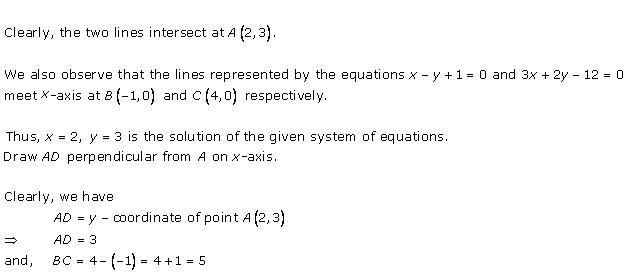
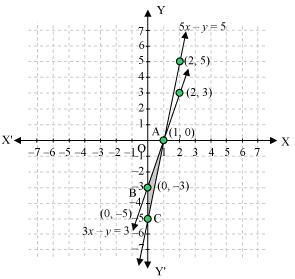
Given equations are:
x - y + 1 = 0 … (i)
3x + 2y - 12 = 0 … (ii)
From (i) we get, x = y - 1
When x = 0, y = 1
When x = -1, y = 0
When x = 1, y = 2
We have the following table:
|
x |
0 |
-1 |
1 |
|
y |
1 |
0 |
2 |
From (ii) we get,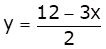 
When x = 0, y = 6
When x = 4, y = 0
When x = 2, y = 3
We have the following table:
|
x |
0 |
4 |
2 |
|
y |
6 |
0 |
3 |
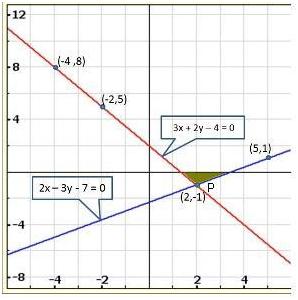
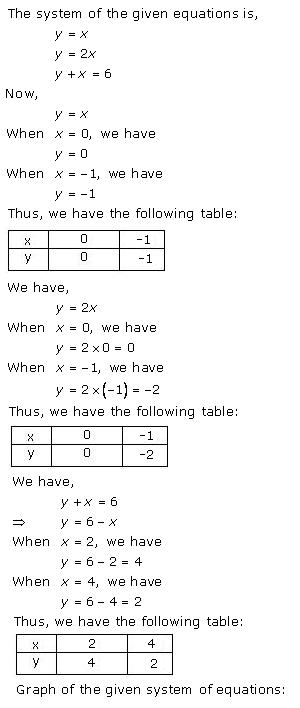
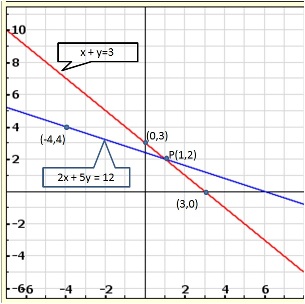
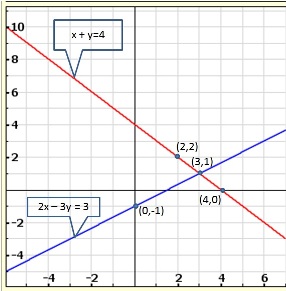
Graph of the given equations is:
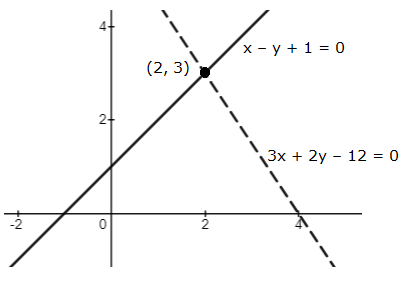
As the two lines intersect at (2, 3).
Hence, x = 2, y = 3 is the solution of the given equations.
Solution 6
Solution 7
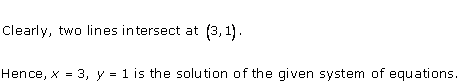
Solution 8
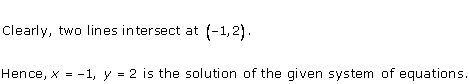
Solution 9
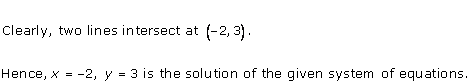
Solution 10
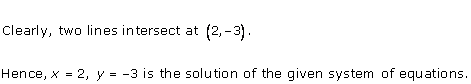
Solution 11
Solution 12
Solution 13
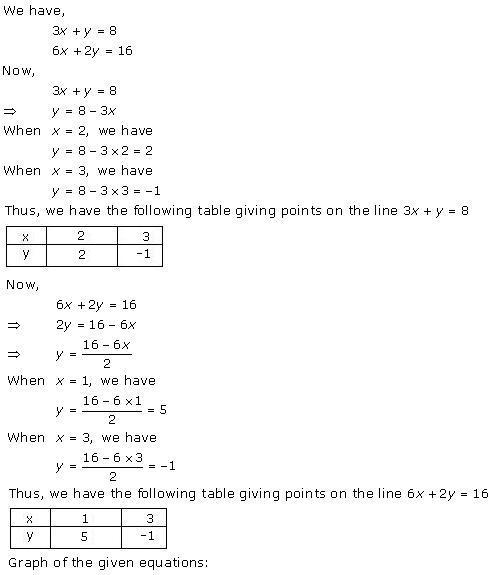
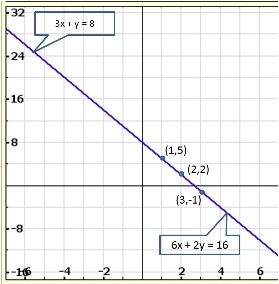
Solution 14
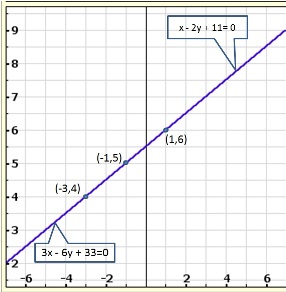
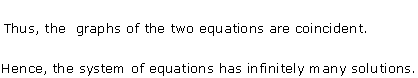
Solution 15
Solution 16
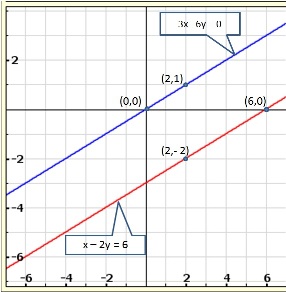
Solution 17
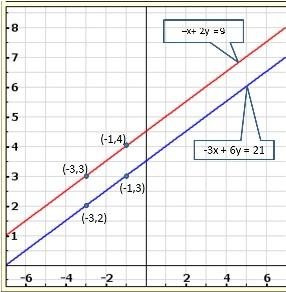
Solution 18
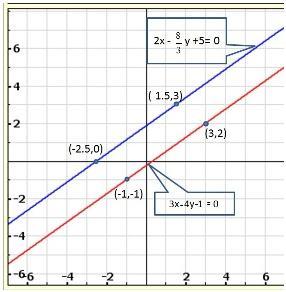
Solution 19(i)
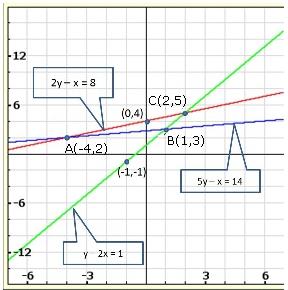
Solution 19(ii)
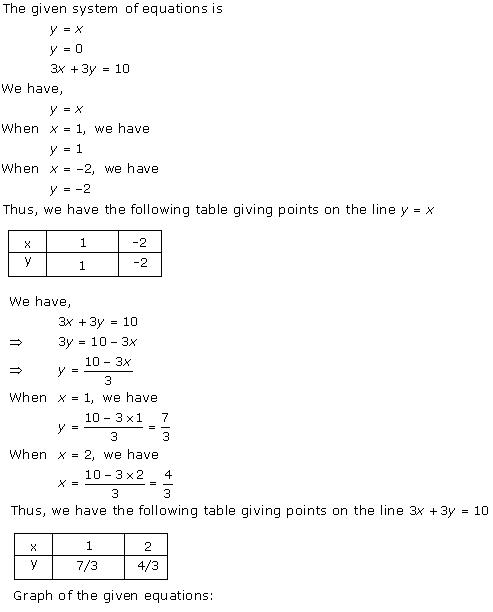
Solution 20
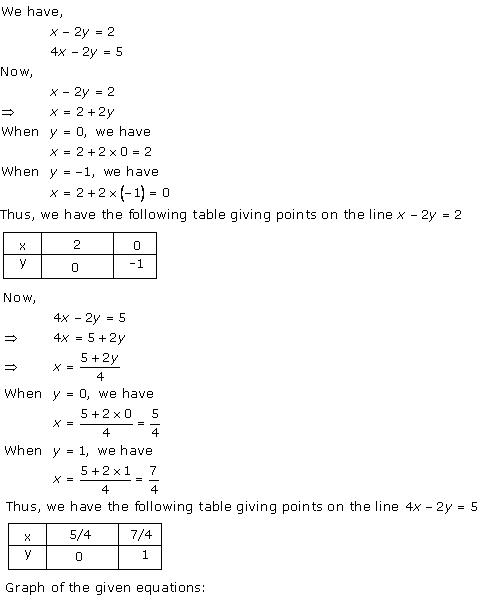
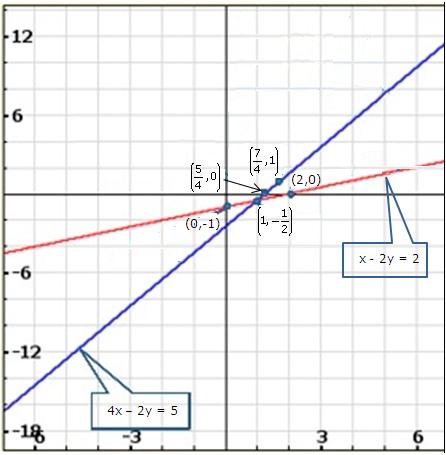
Solution 21(i)
Solution 21(ii)
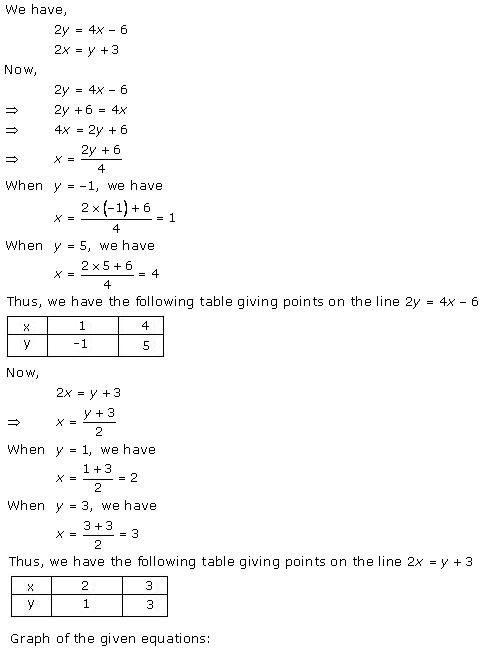
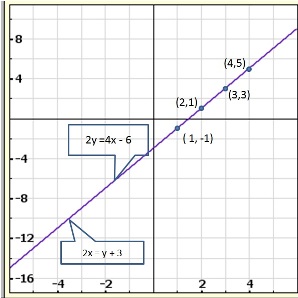
Solution 22(i)
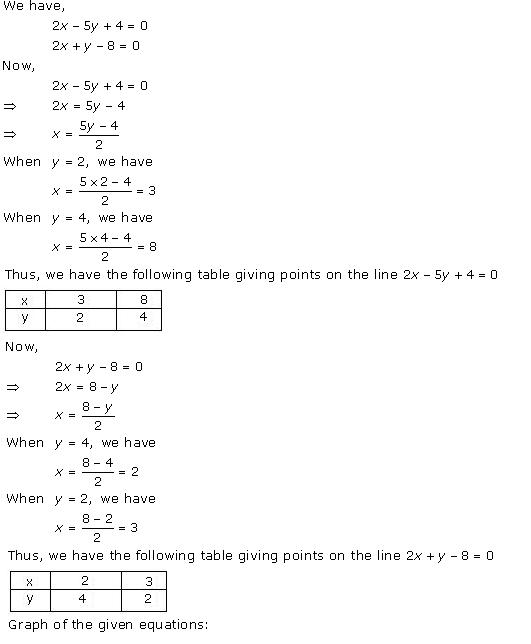
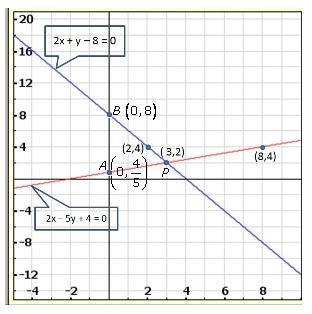
Solution 22(ii)
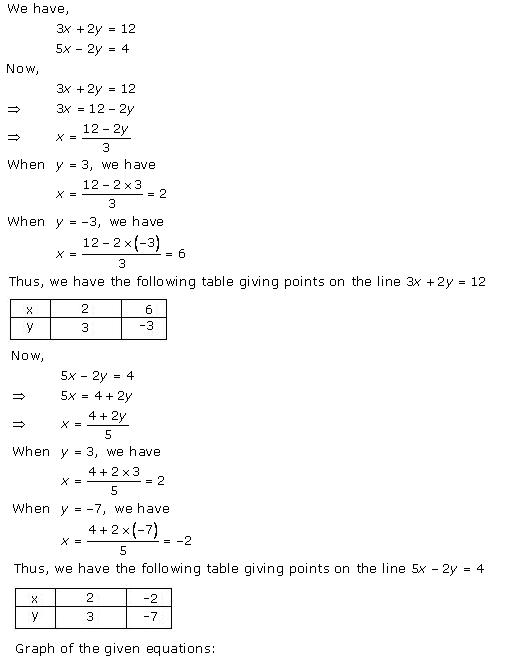
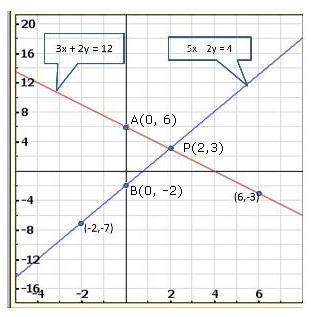
Solution 22(iii)
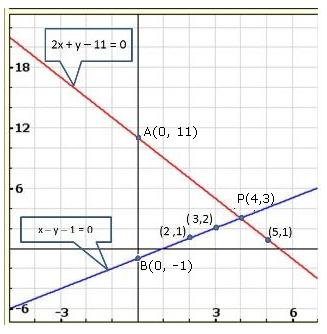
Solution 22(iv)
Solution 22(v)
Solution 22(vi)
Solution 23(i)
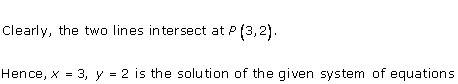
Solution 23(ii)
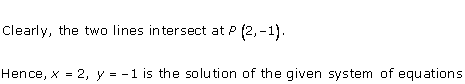
Solution 23(iii)
Solution 24
Solution 25
Solution 26
Solution 27
Solution 28(i)
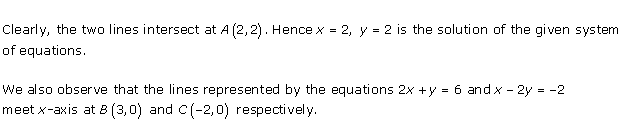
Solution 28(ii)
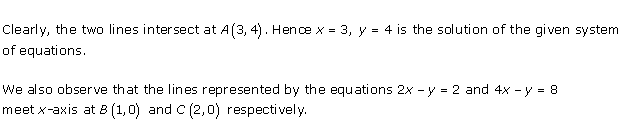
Solution 28(iii)
Solution 28(iv)
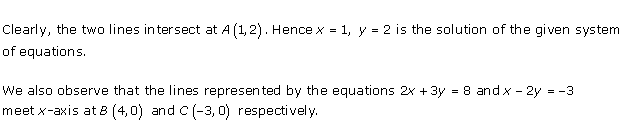
Solution 29
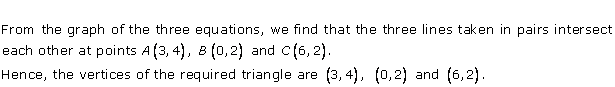
Solution 30
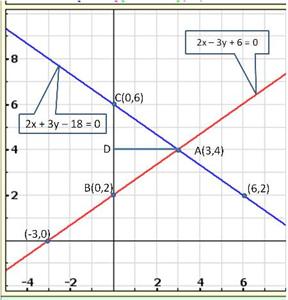
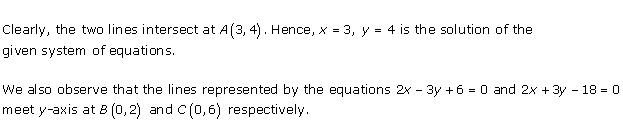
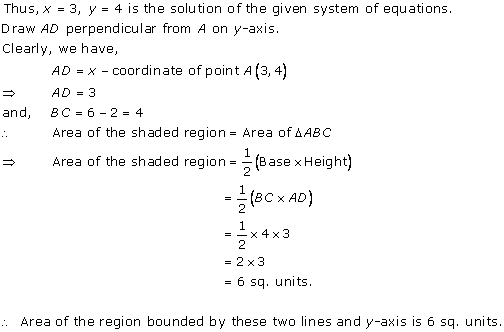
Solution 31
Solution 32
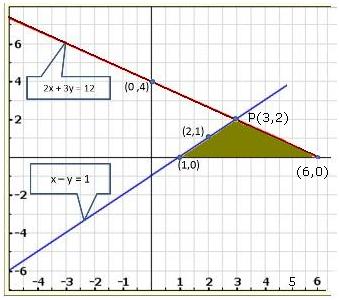
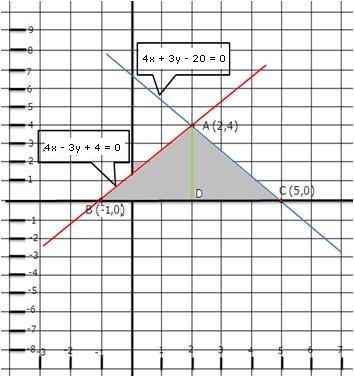
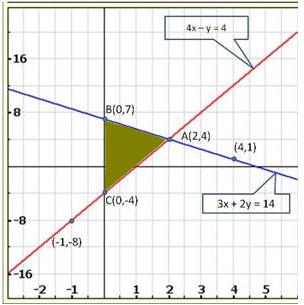
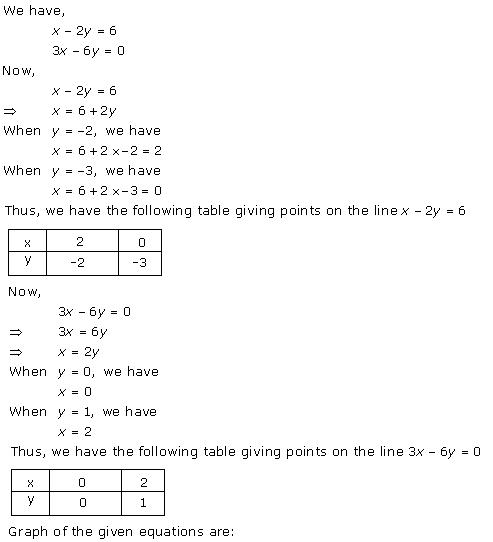
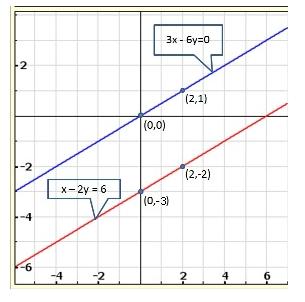
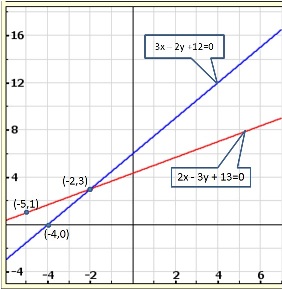
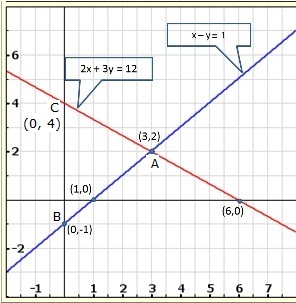
Three solutions of this equation can be written in a table as follows:
| x | 0 | 1 | 2 |
| y | -5 | 0 | 5 |
| x | 0 | 1 | 2 |
| y | -3 | 0 | 3 |
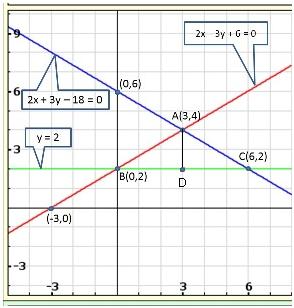
The graphical representation of the two lines will be as follows:
It can be observed that the required triangle is 
ABC.
The coordinates of its vertices are A (1, 0), B (0, -3), C (0, -5).
Concept insight: In order to find the coordinates of the vertices of the triangle so formed, find the points where the two lines intersects the y-axis and also where the two lines intersect each other. Here, note that the coordinates of the intersection of lines with y-axis is taken and not with x-axis, this is because the question says to find the triangle formed by the two lines and the y-axis.
Solution 33
(i) Let the number of girls and boys in the class be x and y respectively.
According to the given conditions, we have:
x + y = 10
x - y = 4
x + y = 10 
x = 10 - y
Three solutions of this equation can be written in a table as follows:
| x | 4 | 5 | 6 |
| y | 6 | 5 | 4 |
x - y = 4 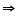x = 4 + y
Three solutions of this equation can be written in a table as follows:
| x | 5 | 4 | 3 |
| y | 1 | 0 | -1 |
The graphical representation is as follows: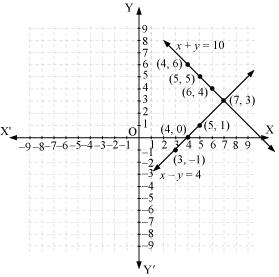 
From the graph, it can be observed that the two lines intersect each other at the point (7, 3).
So, x = 7 and y = 3.
Thus, the number of girls and boys in the class are 7 and 3 respectively.
(ii) Let the cost of one pencil and one pen be Rs x and Rs y respectively.
According to the given conditions, we have:
5x + 7y = 50
7x + 5y = 46
Three solutions of this equation can be written in a table as follows:
| x | 3 | 10 | -4 |
| y | 5 | 0 | 10 |
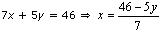
Three solutions of this equation can be written in a table as follows:
| x | 8 | 3 | -2 |
| y | -2 | 5 | 12 |
The graphical representation is as follows: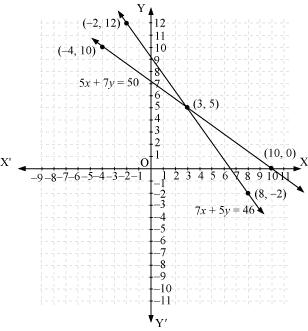 
From the graph, it can be observed that the two lines intersect each other at the point (3, 5).
So, x = 3 and y = 5.
Therefore, the cost of one pencil and one pen are Rs 3 and Rs 5 respectively.
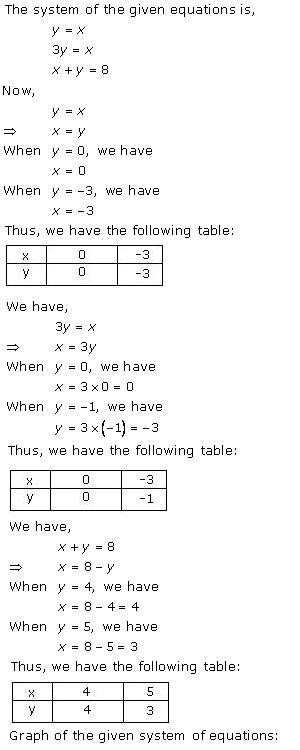
(iii)
Let us denote the number of pants by x and the number of skirts by y. Then the equations formed are:
y = 2x - 2 ...(1)
and y = 4x - 4 ...(2)
Let us draw the graphs of Equations (1) and (2) by finding two solutions for each of the equations.
They are given in Table
| x | 2 | 0 |
| y = 2x - 2 | 2 | -2 |
| x | 0 | 1 |
| y = 4x - 4 | -4 | 0 |
Plot the points and draw the lines passing through them to represent the equations, as shown in fig.,
The two lines intersect at the point (1,0). So, x = 1, y = 0 is the required solution of the pair of linear equations, i.e., the number of pants she purchased is 1 and she did not buy any skirt.
Concept insight: Read the question carefully and examine what are the unknowns. Represent the given conditions with the help of equations by taking the unknowns quantities as variables. Also carefully state the variables as whole solution is based on it. On the graph paper, mark the points accurately and neatly using a sharp pencil. Also, take at least three points satisfying the two equations in order to obtain the correct straight line of the equation. Since joining any two points gives a straight line and if one of the points is computed incorrect will give a wrong line and taking third point will give a correct line. The point where the two straight lines will intersect will give the values of the two variables, i.e., the solution of the two linear equations. State the solution point.
Solution 34(i)
Solution 34(ii)
Solution 35
Solution 36
(i) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0, to be intersecting, we must have 
So, the other linear equation can be 5x + 6y - 16 = 0 
(ii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0, to be parallel, we must have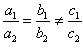 
So, the other linear equation can be 6x + 9y + 24 = 0,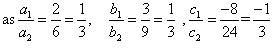 
(iii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0 to be coincident, we must have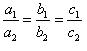 
So, the other linear equation can be 8x + 12y - 32 = 0, 
Concept insight: In order to answer such type of problems, just remember the conditions for two lines to be intersecting, parallel, and coincident. This problem will have multiple answers as their can be many equations satisfying the required conditions.
Solution 37(i)
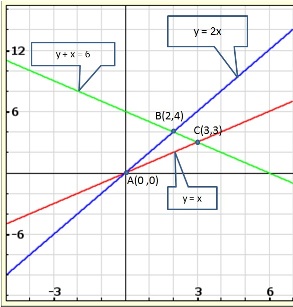
Solution 37(ii)
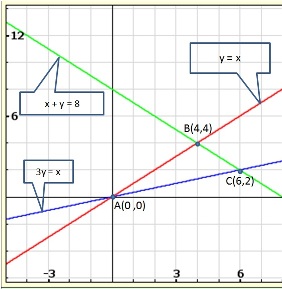
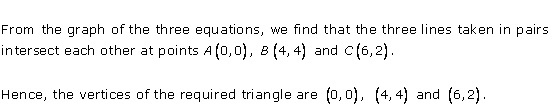
Solution 38
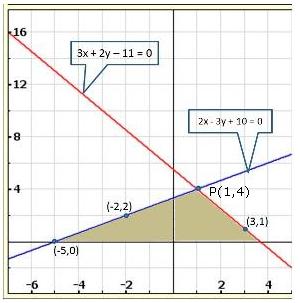
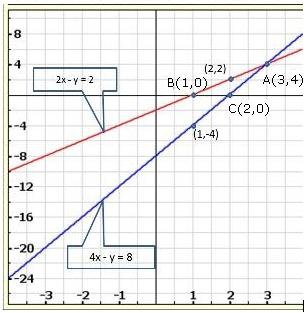
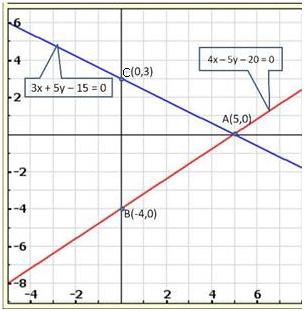
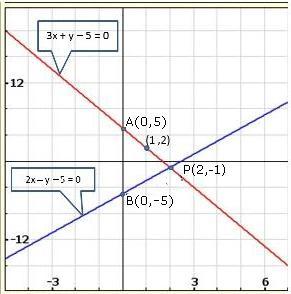
The lines AB and CD intersect at point R(1, 4). Hence, the solution of the given pair of linear equations is x = 1, y = 4.
From R, draw RM ⊥ X-axis and RN ⊥ Y-axis.
Then, from graph, we have
RM = 4 units, RN = 1 unit, AP = 4 units, BQ = 4 units
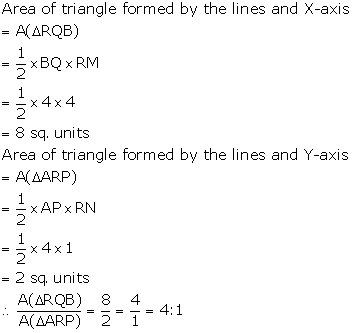
Solution 39
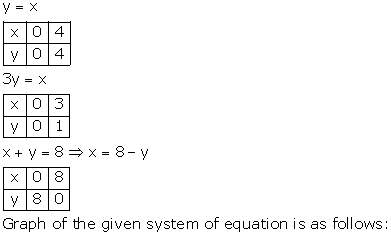
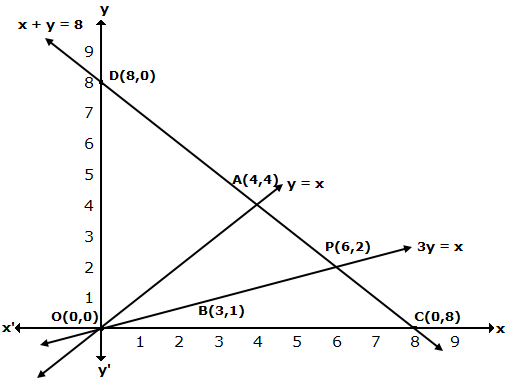
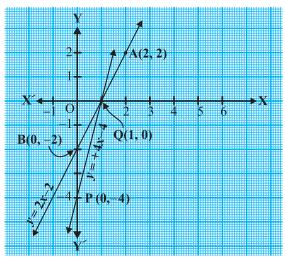
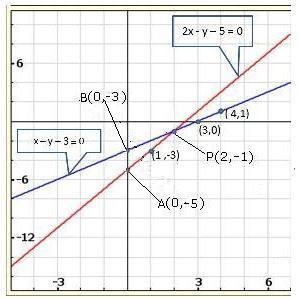
From the graph, the vertices of the triangle AOP formed by the given lines are A(4, 4), O(0, 0) and P(6, 2).
Solution 40
The graph of x = 3 is a straight line parallel to Y-axis at a distance of 3 units to the right of Y-axis.
The graph of x = 5 is a straight line parallel to Y-axis at a distance of 5 units to the right of Y-axis.
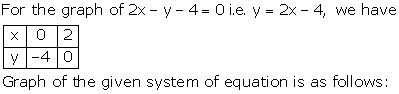
Solution 41
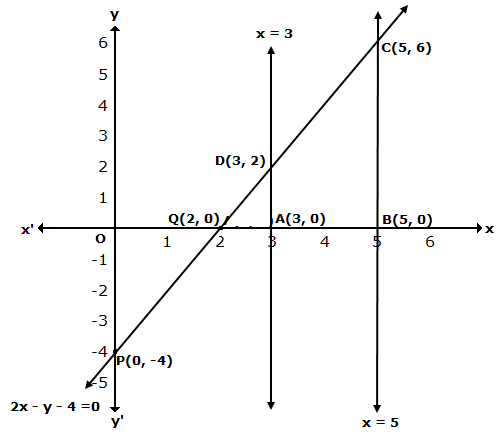
The graph of x = -2 is a straight line parallel to Y-axis at a distance of 2 units to the left of Y-axis.
The graph of y = 3 is a straight line parallel to X-axis at a distance of 3 units above X-axis.
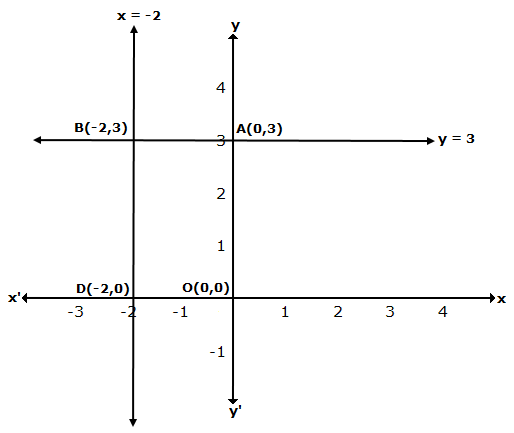
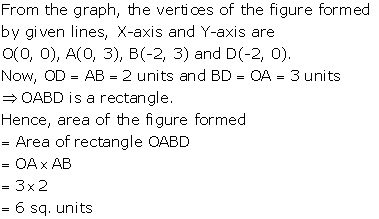
Solution 42
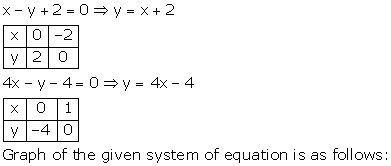
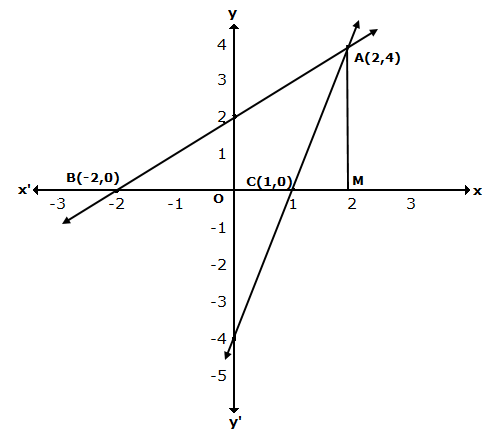
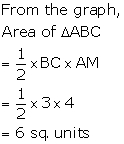
Pairs of Linear Equations in Two Variables Exercise Ex. 3.3
Solution 1
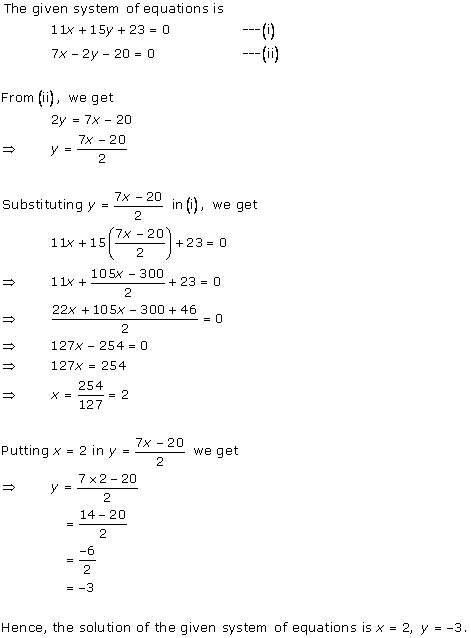
Solution 2
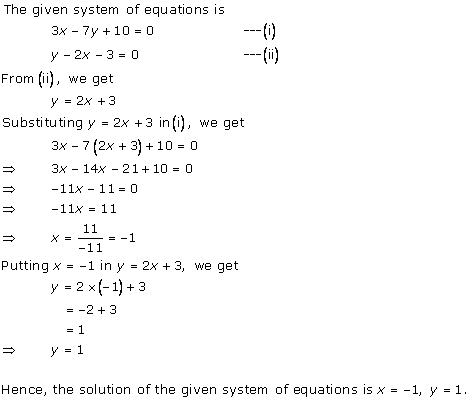
Solution 3
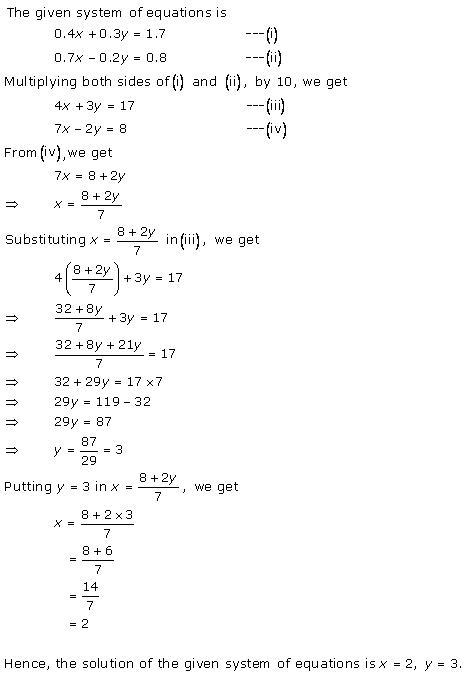
Solution 4
Solution 5
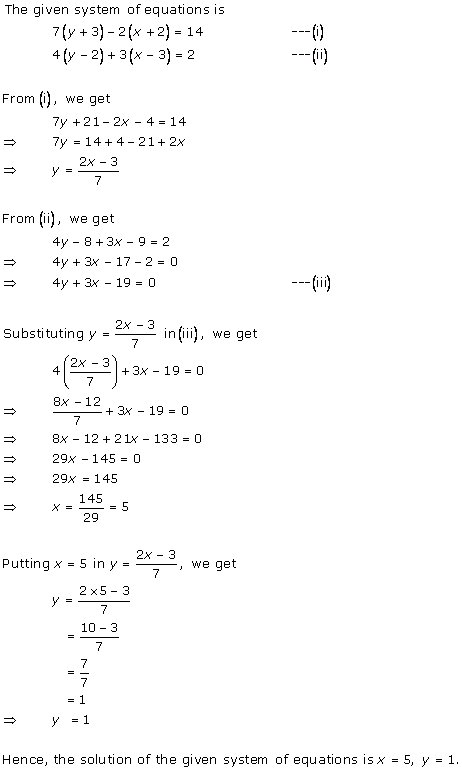
Solution 6
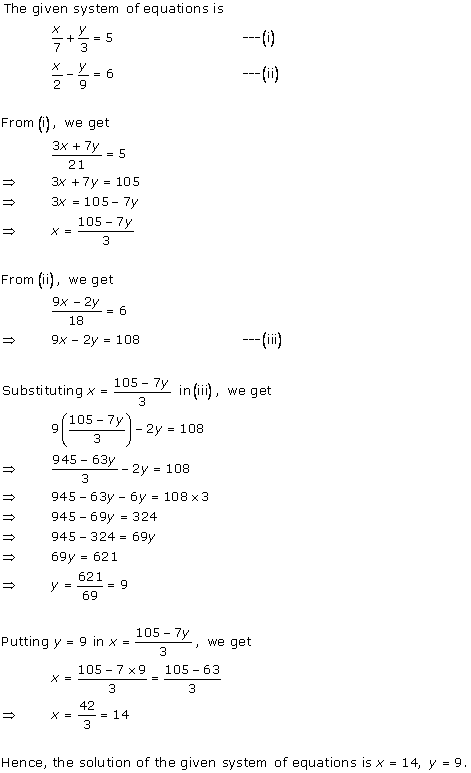
Solution 7
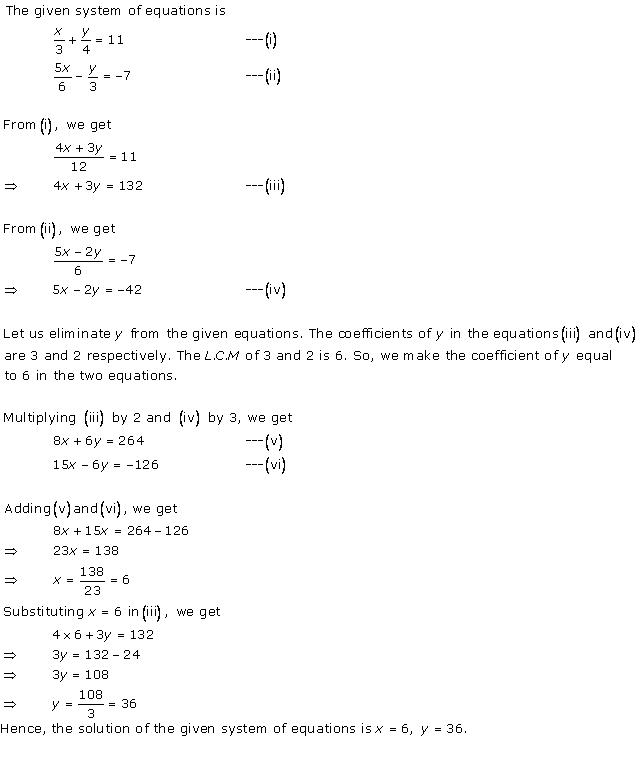
Solution 8
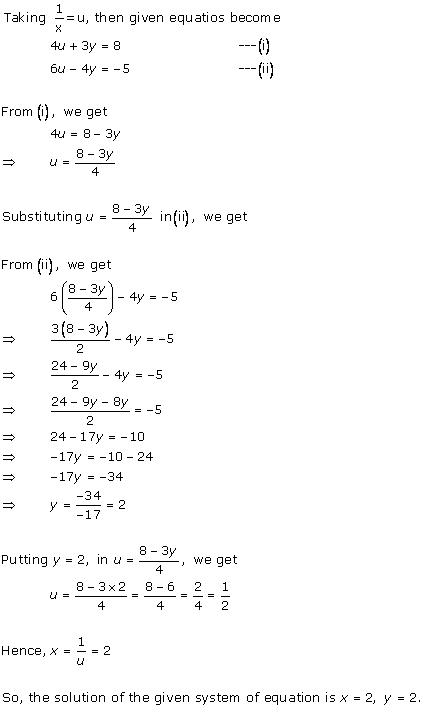
Solution 9
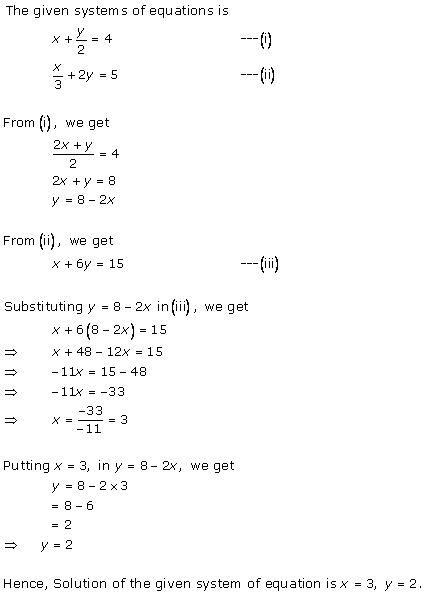
Solution 10
Solution 11
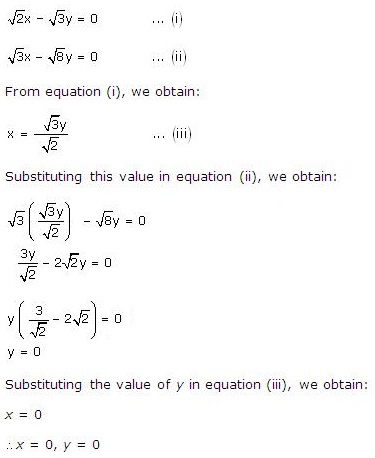
Solution 12
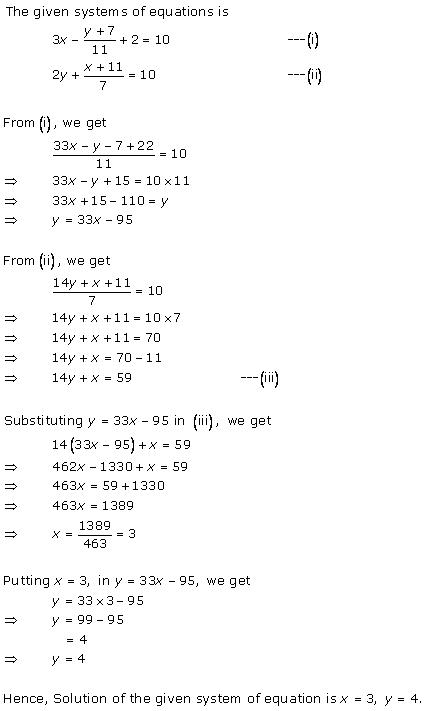
Solution 13
Solution 14
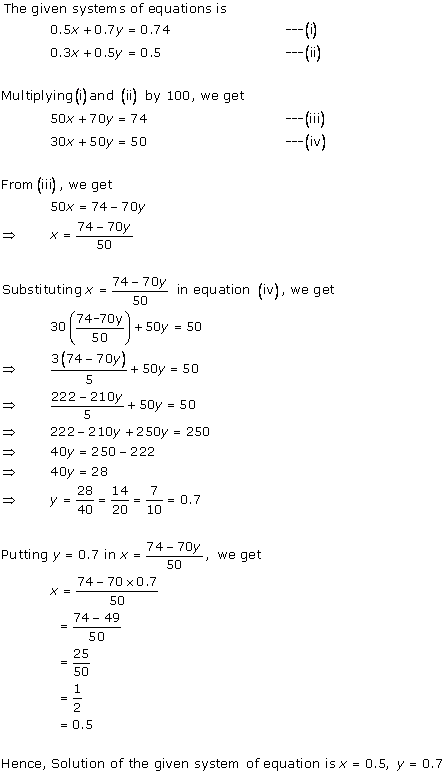
Solution 15
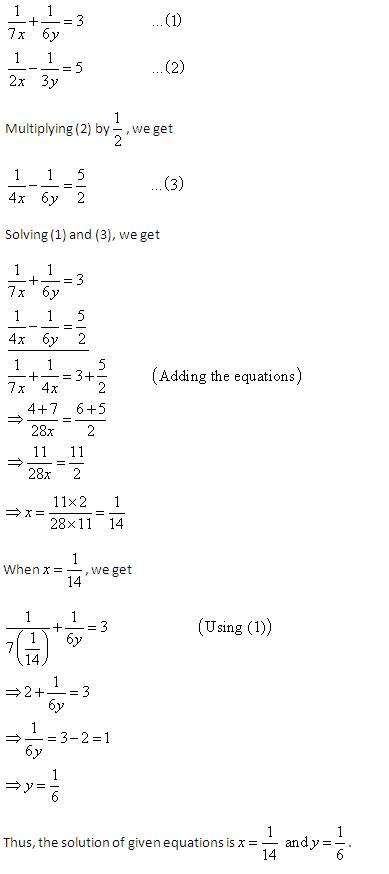
Solution 16
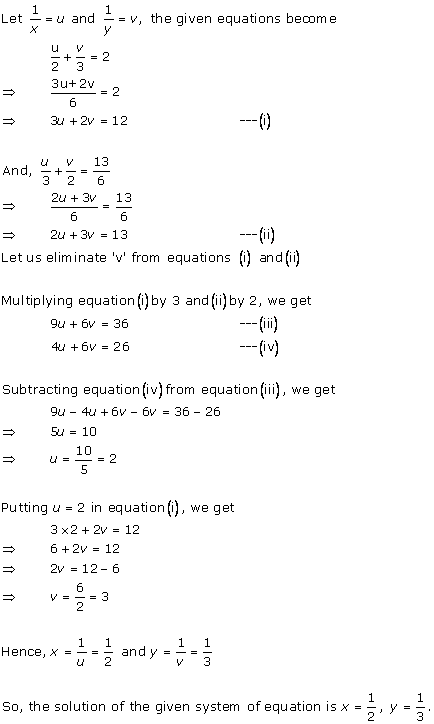
Solution 17
Solution 18
Solution 19
Solution 20
Solution 21
Solution 22
Solution 23
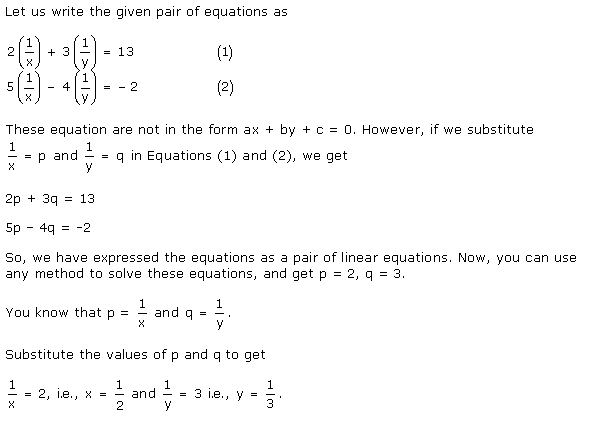
Solution 24
Solution 25
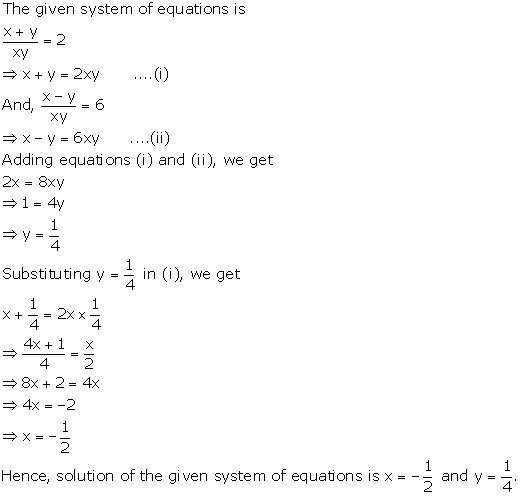
Solution 26
Solution 27
Solution 28
Solution 29
Solution 30
Solution 31
Solution 32
Solution 33
Solution 34
Solution 35
Solution 36
Solution 37
Solution 38
Solution 39
Solution 40
Solution 41
Solution 42
Solution 43
Solution 44
Solution 45
Solution 46
Solution 47
Solution 48
Solution 49
Solution 50
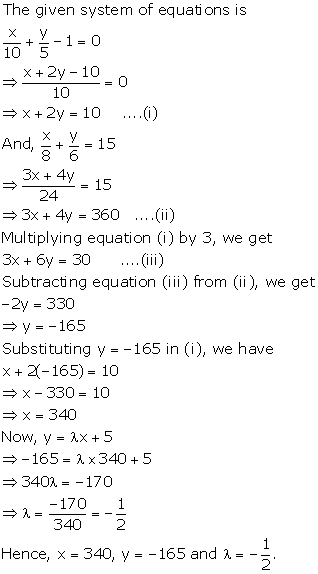
Solution 51
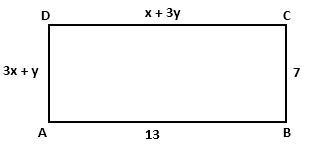
Solution 52
Solution 53
Pairs of Linear Equations in Two Variables Exercise Ex. 3.4
Solution 1
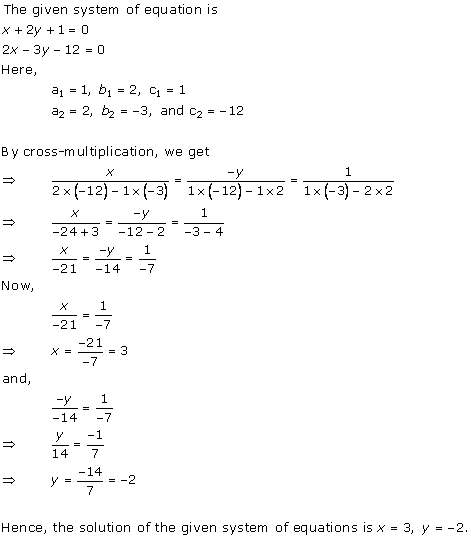
Solution 2
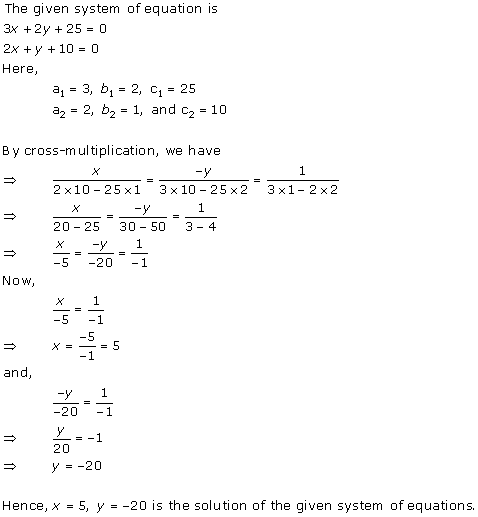
Solution 3
Solution 4
Solution 5
Solution 6
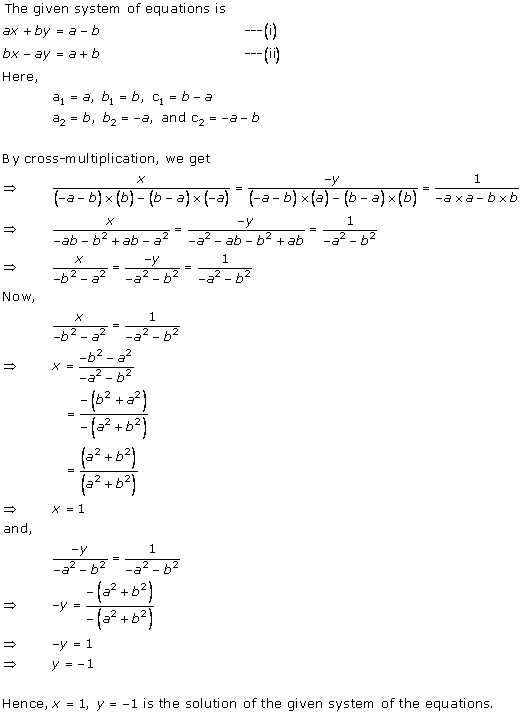
Solution 7
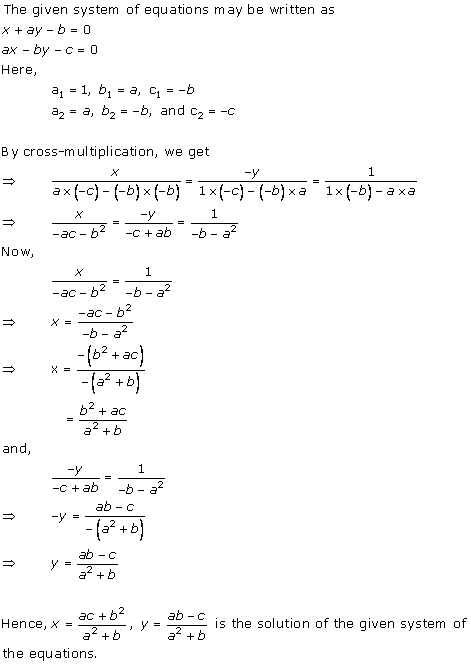
Solution 8
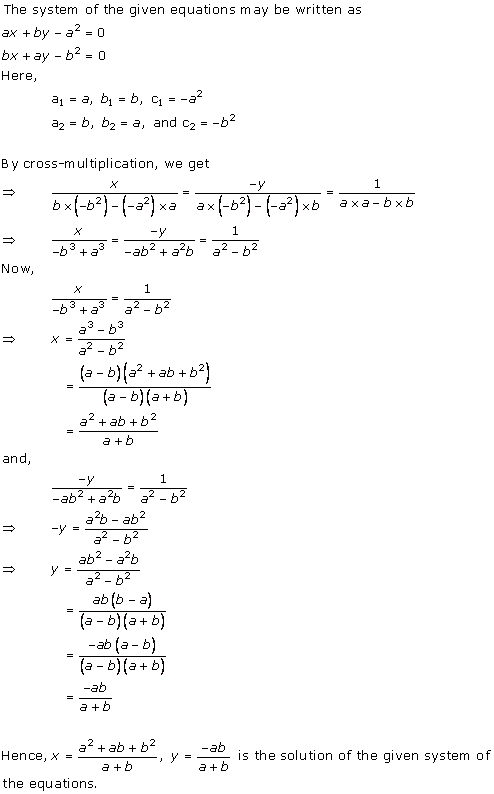
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
Solution 14
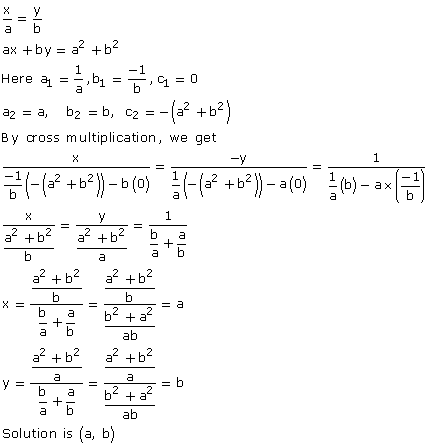
Solution 15
Solution 16
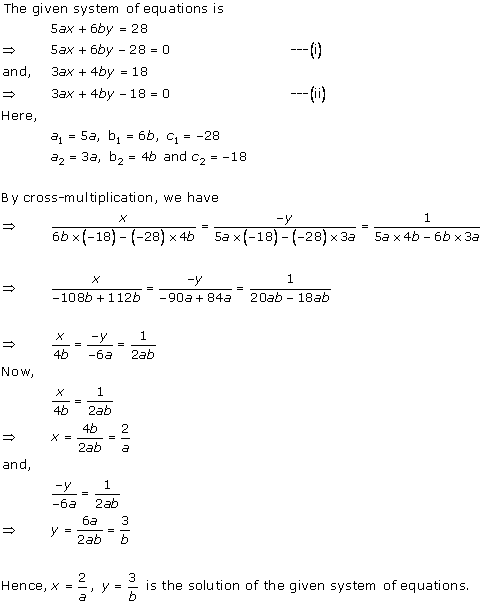
Solution 17
Solution 18
Solution 19
Solution 20
Solution 21
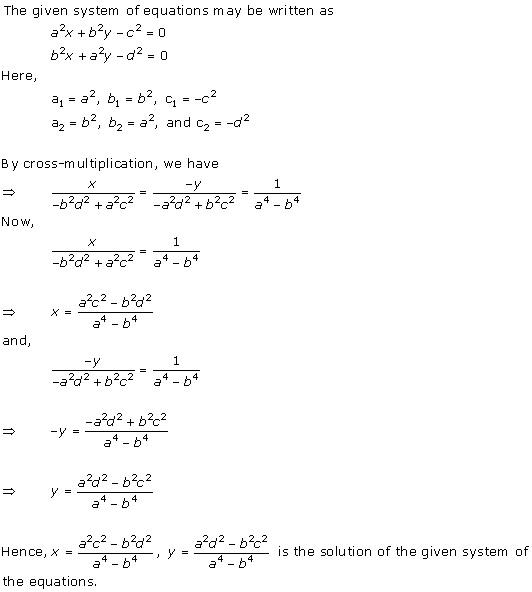
Solution 22
Solution 23
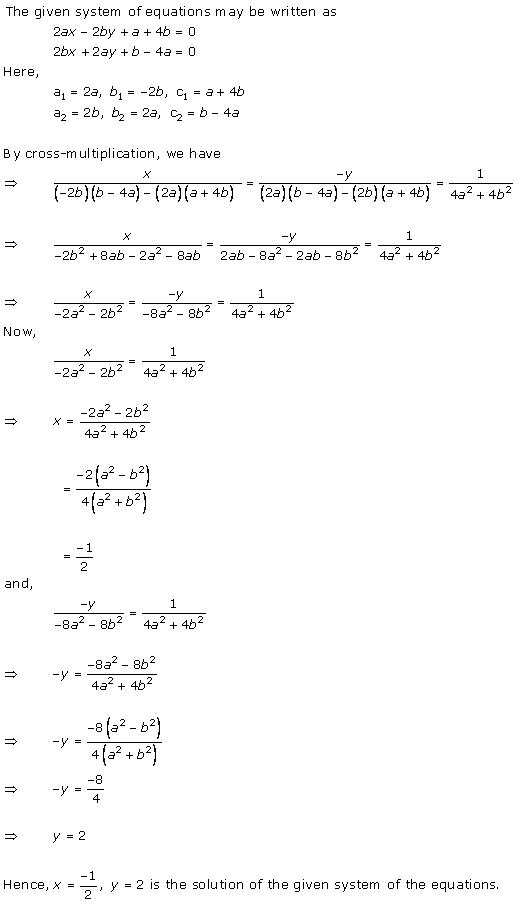
Solution 24
Solution 25
Solution 26
Solution 27
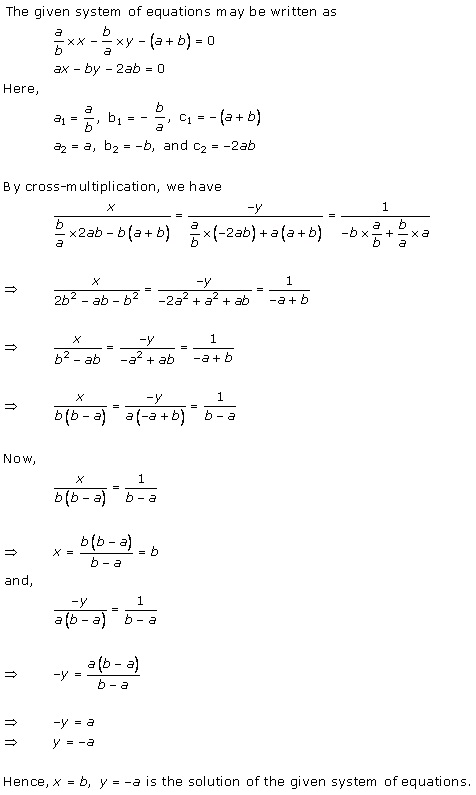
Solution 28
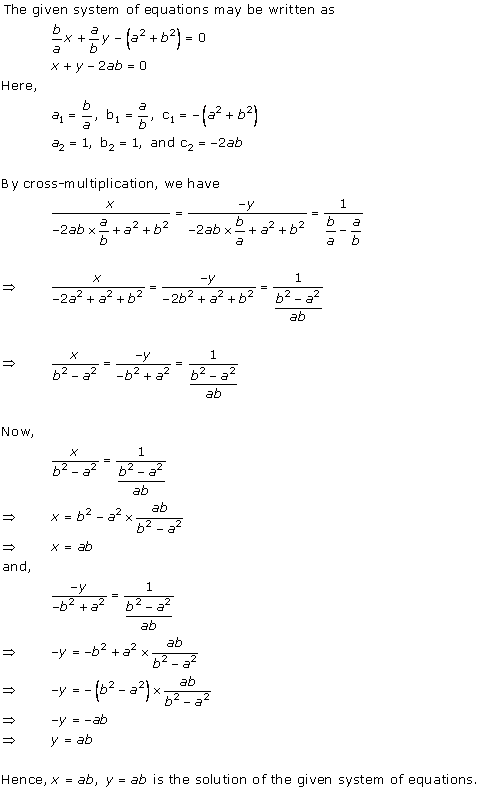
Pairs of Linear Equations in Two Variables Exercise Ex. 3.5
Solution 1
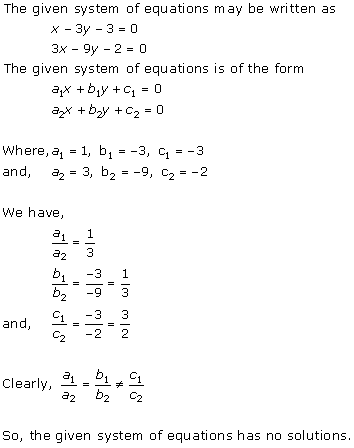
Solution 2
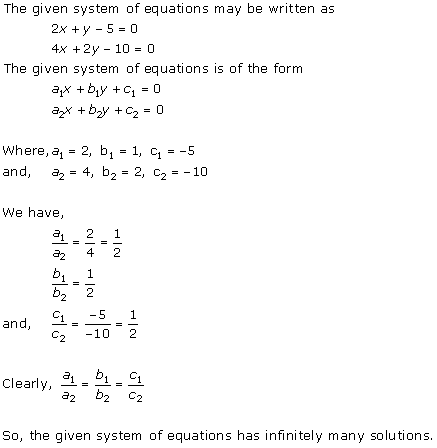
Solution 3
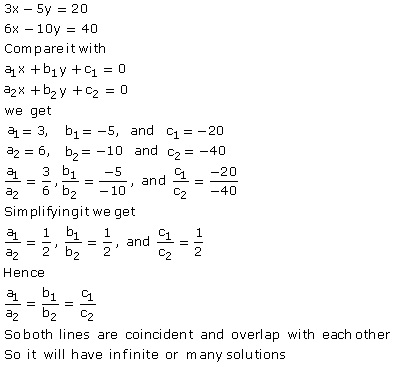
Solution 4
Solution 5
Solution 6
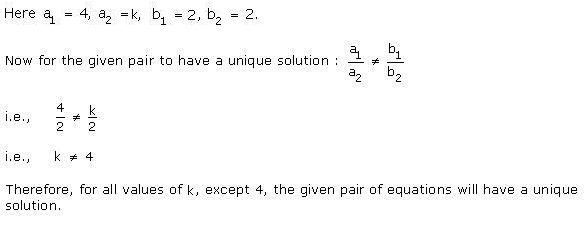
Solution 7
Solution 8
Solution 9
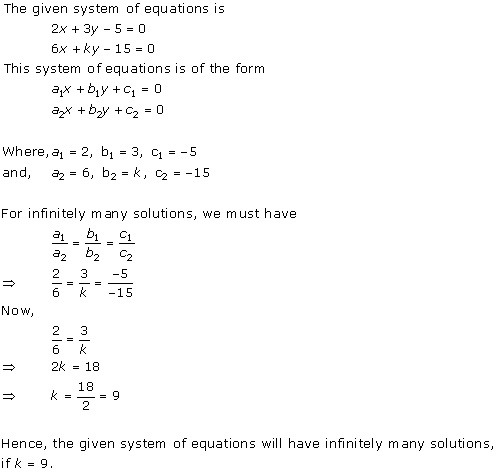
Solution 10
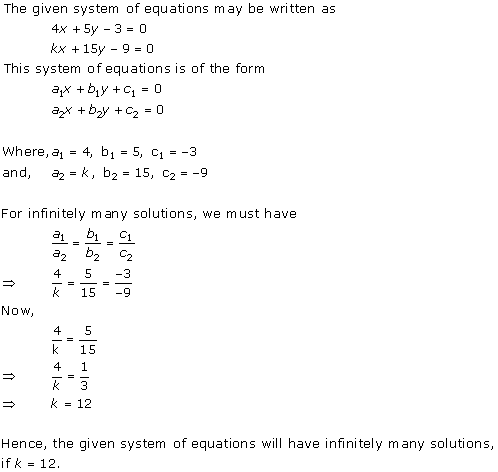
Solution 11
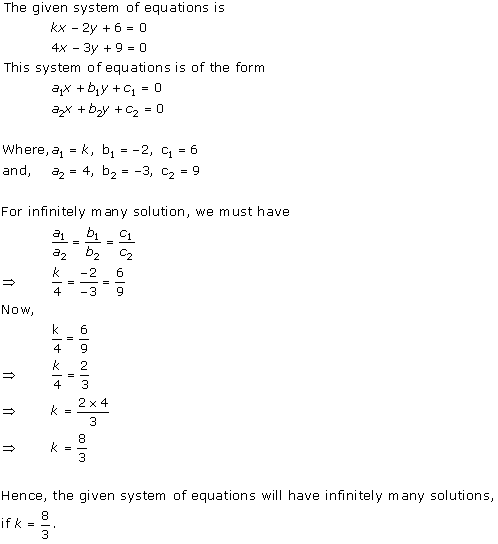
Solution 12
Solution 13
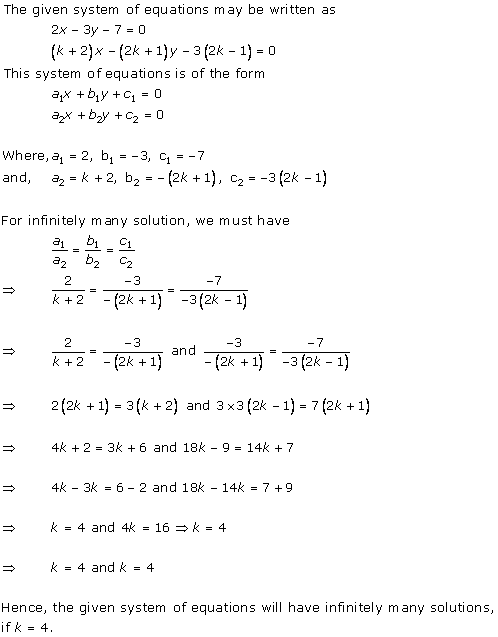
Solution 14
Solution 15
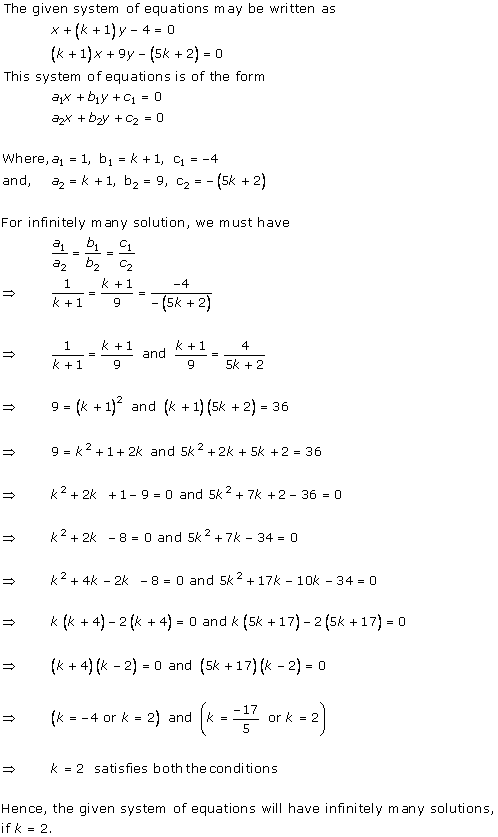
Solution 16
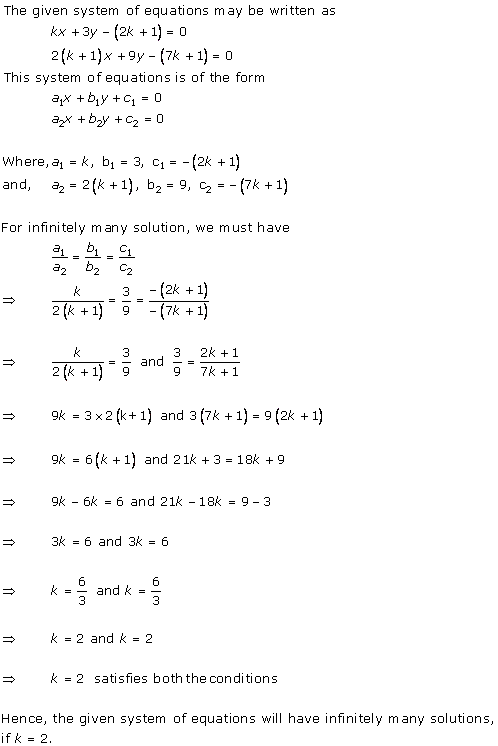
Solution 17
Solution 18
Solution 19
Solution 20
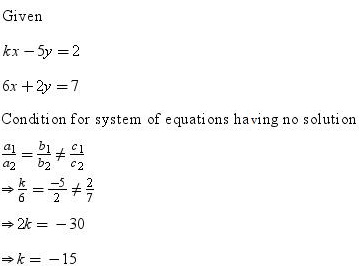
Solution 21
Solution 22
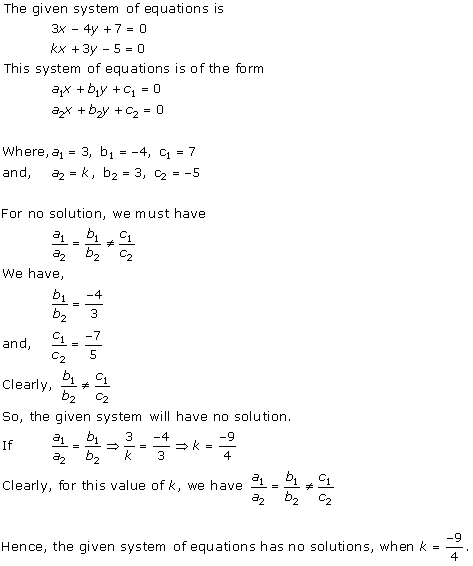
Solution 23
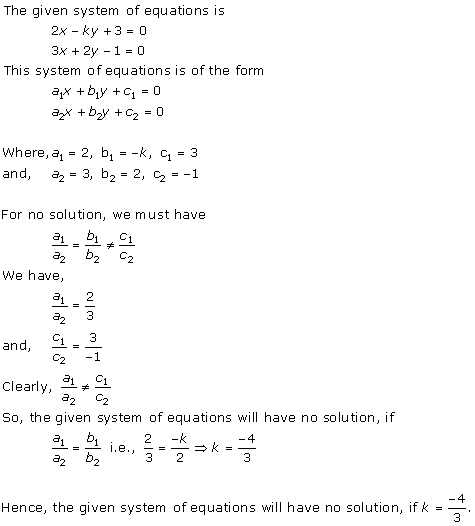
Solution 24
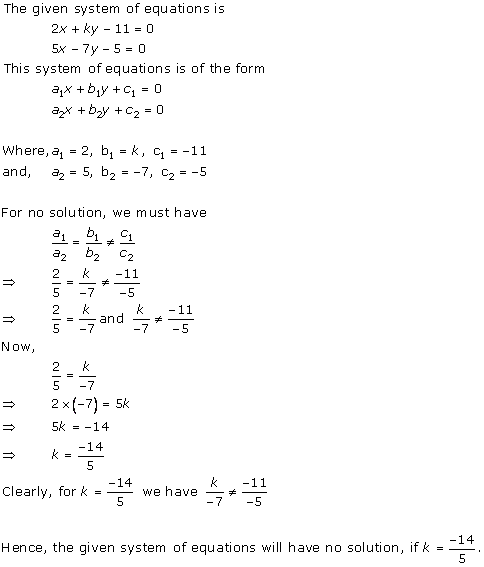
Solution 25
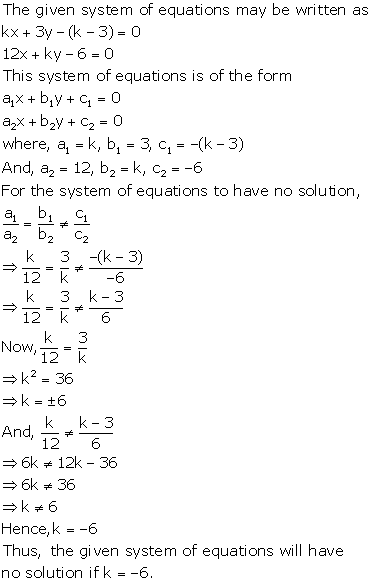
Solution 26
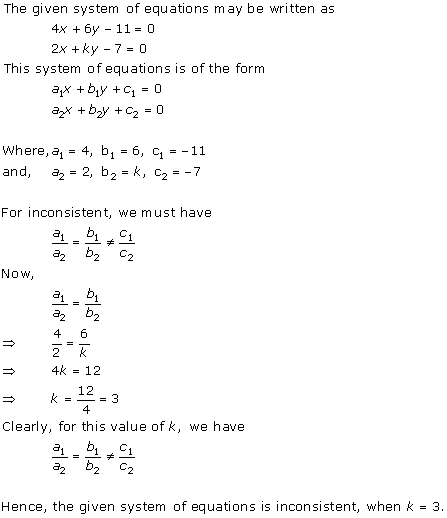
Solution 27
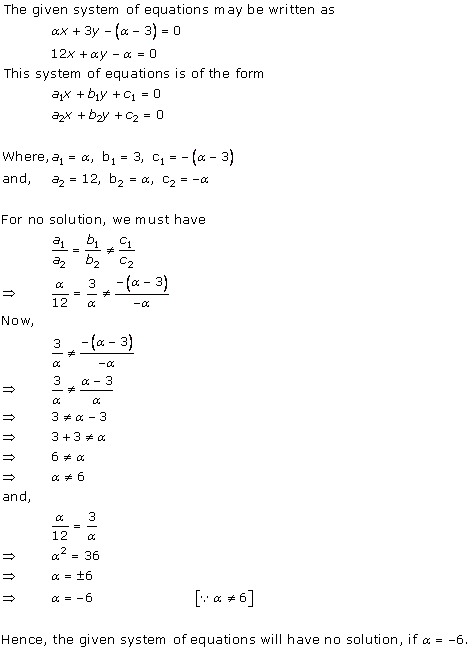
Solution 28
Solution 29
The given system of equations will have infinite number of solutions if
Solution 30
Solution 31
Solution 32
Solution 33
Solution 34
Solution 35
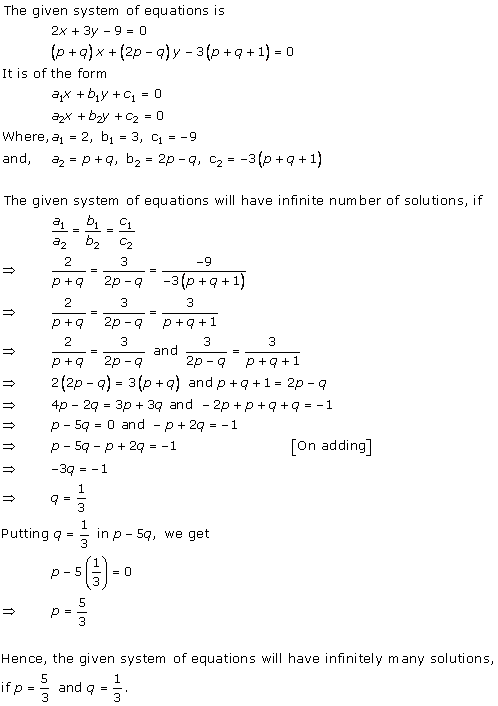
Solution 36 (i)
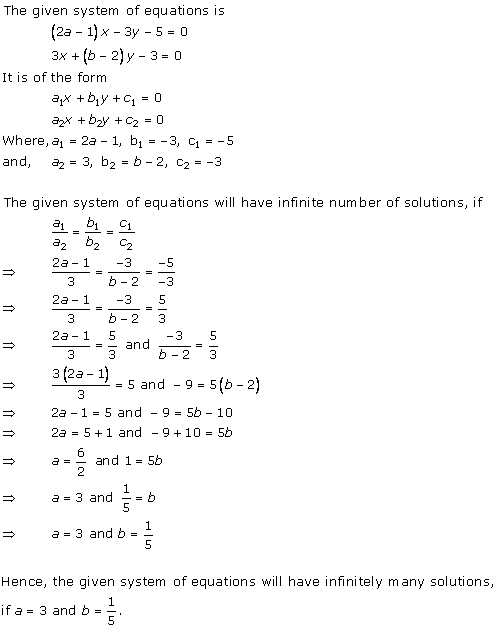
Solution 36 (ii)
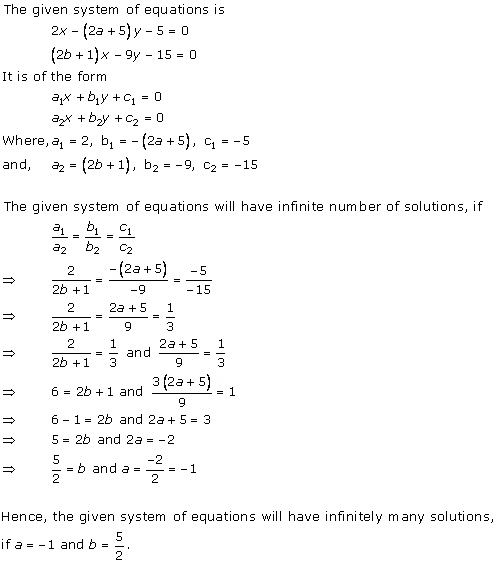
Solution 36 (iii)
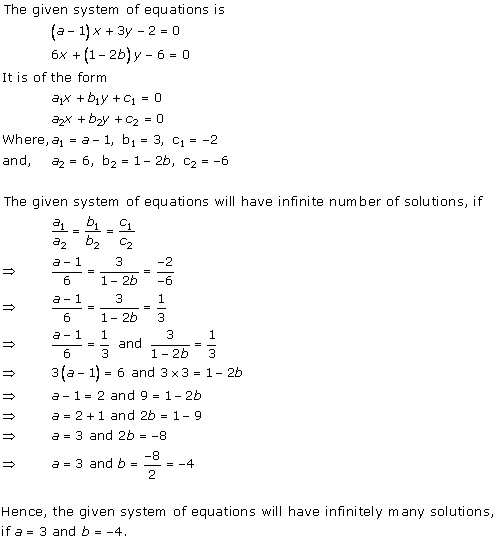
Solution 36 (iv)
Solution 36 (v)
Solution 36 (vi)
Solution 36 (vii)
Solution 36(viii)
Solution 36(ix)
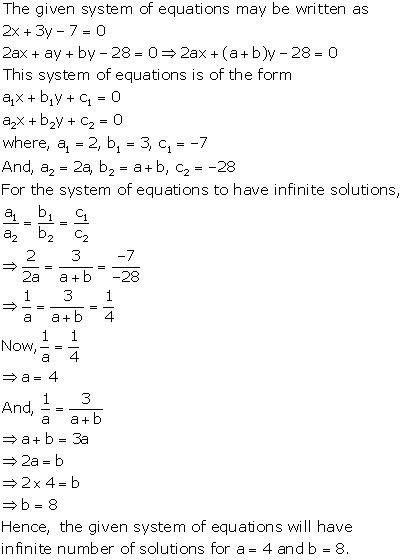
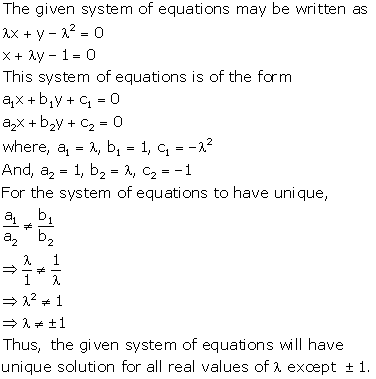
Solution 37(i)
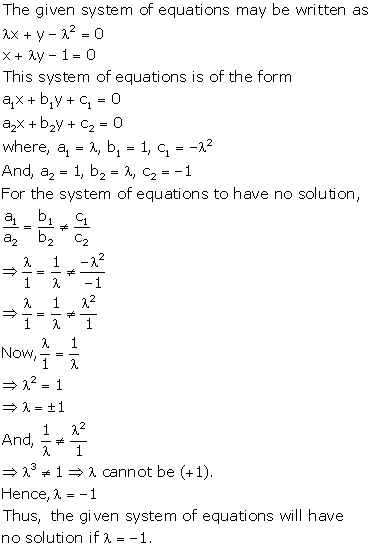
Solution 37(ii)
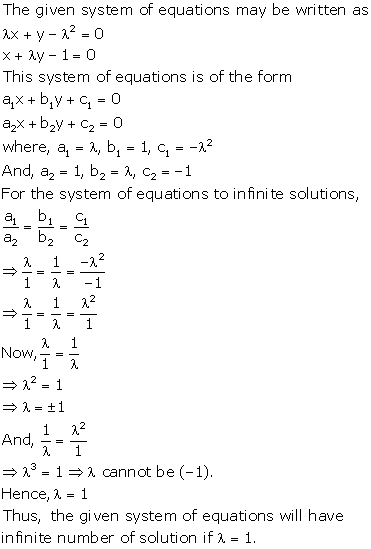
Solution 37(iii)
Pairs of Linear Equations in Two Variables Exercise Ex. 3.6
Solution 1
Solution 2
Solution 3
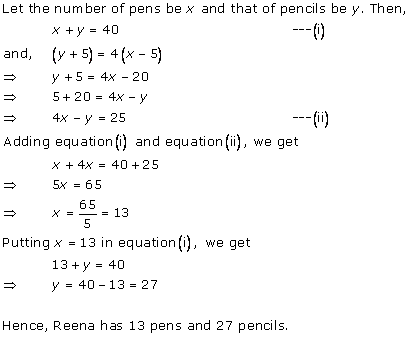
Solution 4
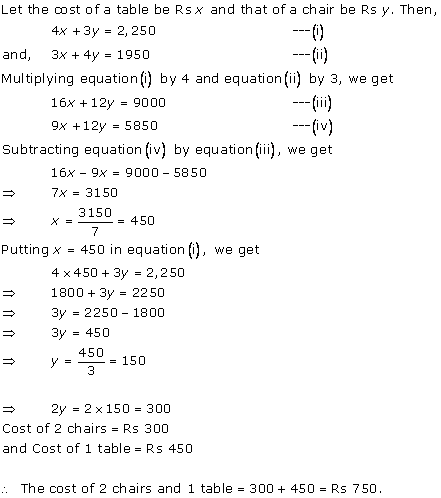
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
Solution 14
Solution 15
Pairs of Linear Equations in Two Variables Exercise Ex. 3.7
Solution 1
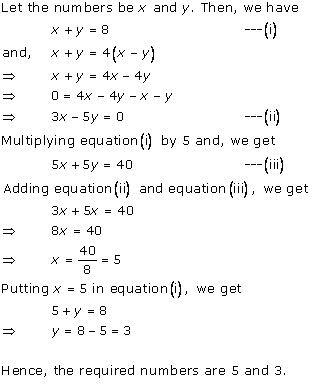
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
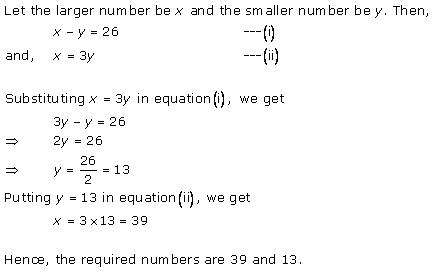
Solution 14
Solution 15
Solution 16
Solution 17
Pairs of Linear Equations in Two Variables Exercise Ex. 3.8
Solution 1
Solution 2
Solution 3
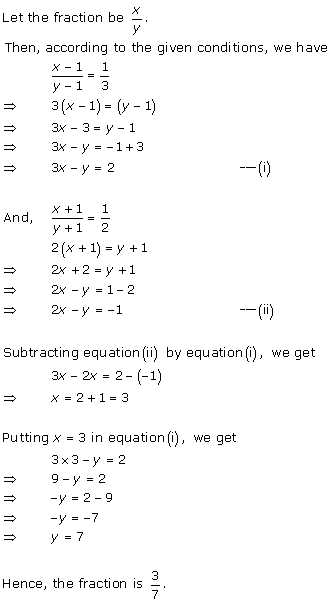
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Let
the fraction be 
According to the given conditions, we have
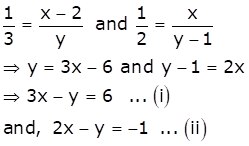
Subtracting (ii) from (i), we get x = 7
Substituting the value of x in (ii), we get
y = 15
Solution 9Old
Solution 10
Solution 11
Pairs of Linear Equations in Two Variables Exercise Ex. 3.9
Solution 1
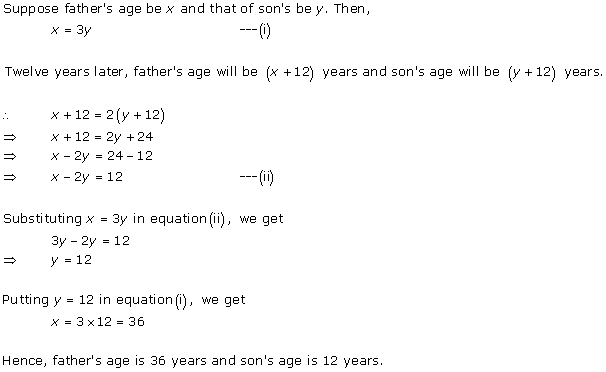
Solution 2
Solution 3
Solution 4
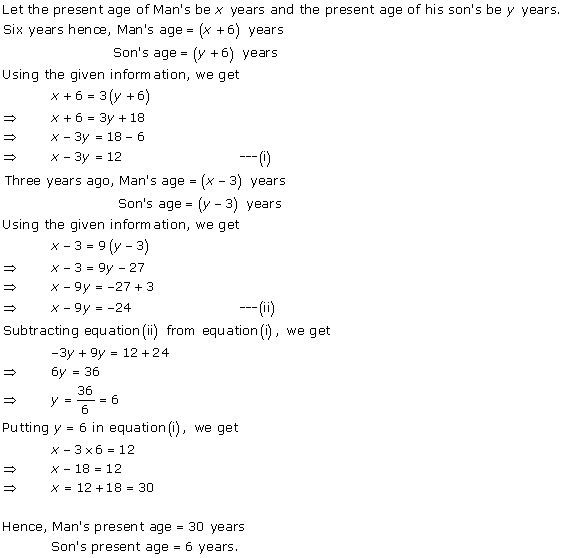
Solution 5
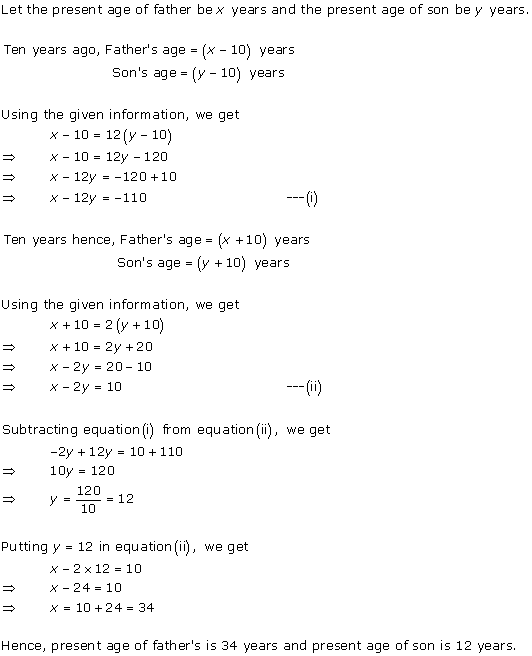
Solution 6
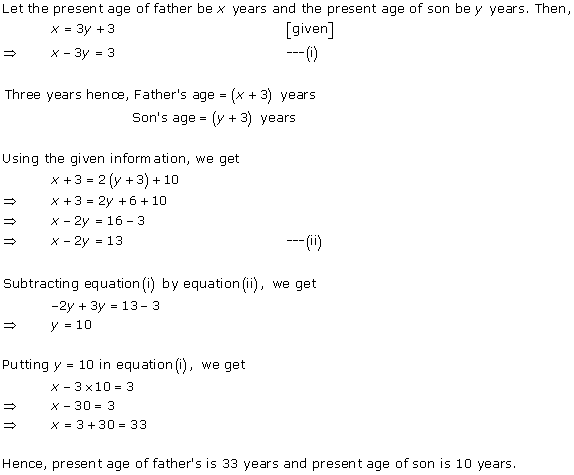
Solution 7
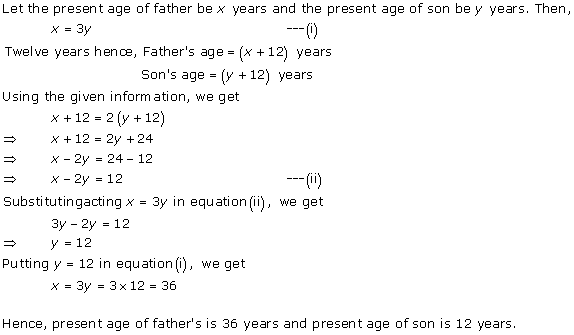
Solution 8
Solution 9
Solution 10
Solution 11
The difference between the ages of Ani and Biju is given as 3 years. So, either Biju is 3 years older than Ani or Ani is 3 years older than Biju.
Let the age of Ani and Biju be x years and y years respectively.
Age of Dharam = 2 × x = 2x years 
x - y = 3 ... (1)
Age of Biju = 19 - 3 = 16 years
Case II: Biju is older than Ani by 3 years
y - x = 3 ... (3)
4x - y = 60 ... (4)
Adding (3) and (4), we obtain:
3x = 63
x = 21
Age of Ani = 21 years
Age of Biju = 21 + 3 = 24 years
Concept Insight: In this problem, ages of Ani and Biju are the unknown quantities. So, we represent them by variables x and y. Now, note that here it is given that the ages of Ani and Biju differ by 3 years. So, it is not mentioned that which one is older. So, the most important point in this question is to consider both cases Ani is older than Biju and Biju is older than Ani. For second condition the relation on the ages of Dharam and Cathy can be implemented . Pair of linear equations can be solved using a suitable algebraic method.
Solution 12
Solution 13
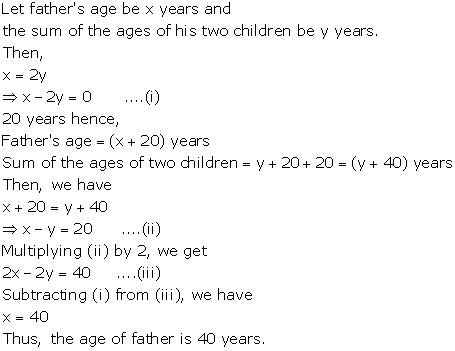
Pairs of Linear Equations in Two Variables Exercise Ex. 3.10
Solution 1
Solution 2
Solution 3
Solution 4
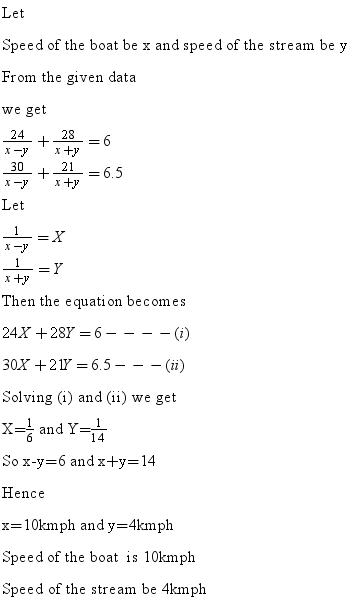
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
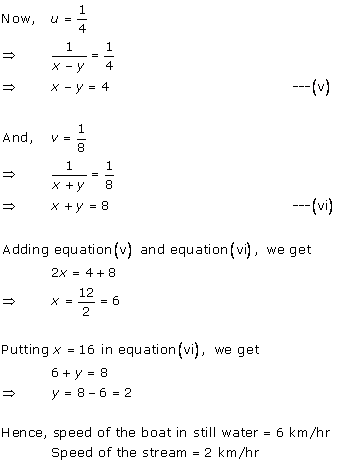
Solution 11
Solution 12
Solution 13
Solution 14
Solution 15
According to the question,
By using equation (1), we obtain:
3x - 10t = 30 ... (3)
Adding equations (2) and (3), we obtain:
x = 50
Substituting the value of x in equation (2), we obtain:
(-2) x (50) + 10t = 20
-100 + 10t = 20
10t = 120
t = 12
From equation (1), we obtain:
d = xt = 50 x 12 = 600
Thus, the distance covered by the train is 600 km.
Concept insight: To solve this problem, it is very important to remember the relation
. Now, all these three quantities are unknown. So, we will represent these by three different
variables. By using the given conditions, a pair of equations will be obtained. Mind one thing that the equations obtained will not be linear. But they can be reduced to linear form by using the fact that
. Then two linear equations can be formed which can be solved easily by elimination method.
Solution 16
Solution 17
Solution 18
Pairs of Linear Equations in Two Variables Exercise Ex. 3.11
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
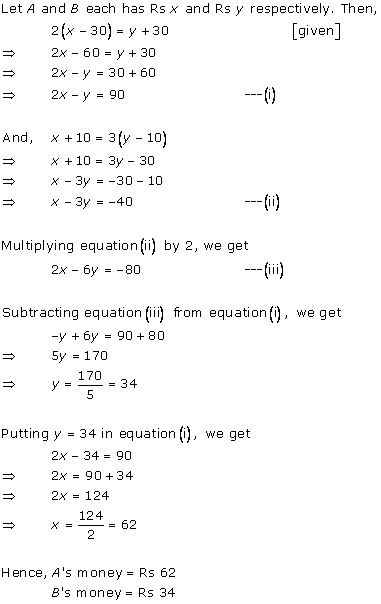
Solution 6
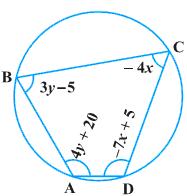
We know that the sum of the measures of opposite angles in a cyclic quadrilateral is 180°. 
A + C = 180
4y + 20 - 4x = 180
-4x + 4y = 160
x - y = -40 ... (1)
Also, B + D = 180
3y - 5 - 7x + 5 = 180
-7x + 3y = 180 ... (2)
Multiplying equation (1) by 3, we obtain:
3x - 3y = -120 ... (3)
Adding equations (2) and (3), we obtain:
-4x = 60
x = -15
Substituting the value of x in equation (1), we obtain:
-15 - y = -40
y = -15 + 40 = 25 A = 4y + 20 = 4(25) + 20 = 120oB = 3y - 5 = 3(25) - 5 = 70oC = -4x = -4(-15) = 60oD = -7x + 5 = -7(-15) + 5 = 110o
Concept insight: The most important idea to solve this problem is by using the fact that the sum of the measures of opposite angles in a cyclic quadrilateral is 180o. By using this relation, two linear equations can be obtained which can be solved easily by eliminating a suitable variable.
Solution 7
Solution 8
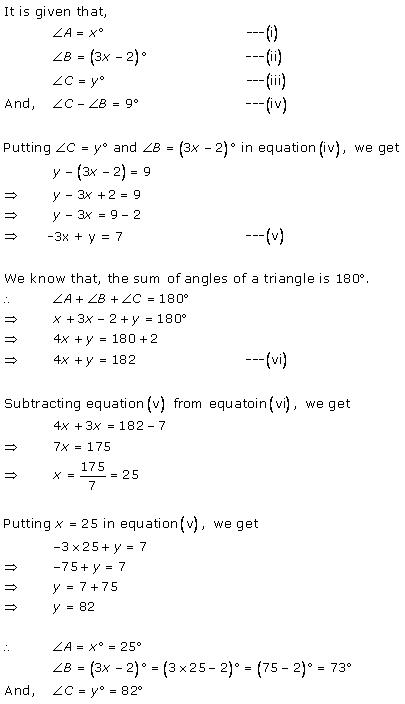
Solution 9
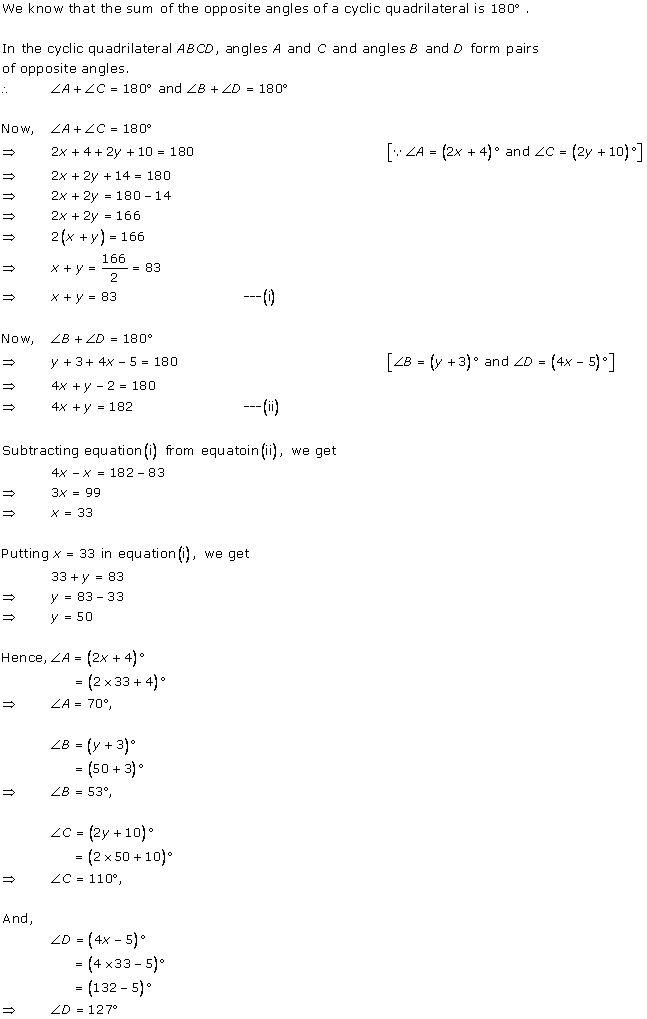
Solution 10
Solution 11
Solution 12
Solution 13
Solution 14
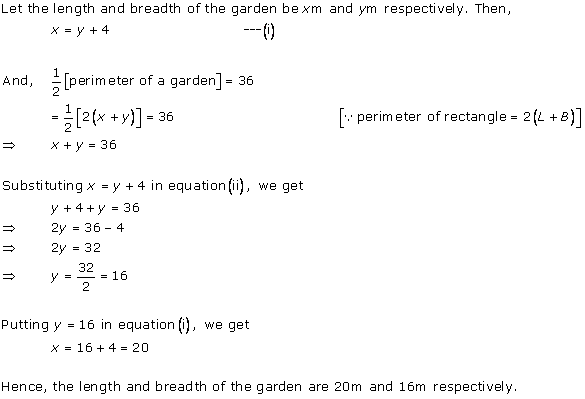
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
Solution 20
Solution 21
Solution 22
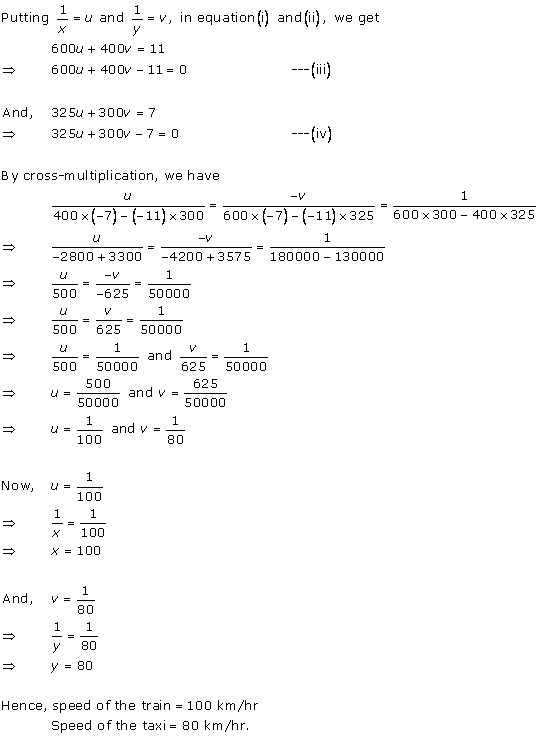
Let the money with the first person and second person be Rs x and Rs y respectively.
According to the question,
x + 100 = 2(y - 100)
x + 100 = 2y - 200
x - 2y = -300 ... (1)
6(x - 10) = (y + 10)
6x - 60 = y + 10
6x - y = 70 ... (2)
Multiplying equation (2) by 2, we obtain:
12x - 2y = 140 ... (3)
Subtracting equation (1) from equation (3), we obtain:
11x = 140 + 300
11x = 440
x = 40
Putting the value of x in equation (1), we obtain:
40 - 2y = -300
40 + 300 = 2y
2y = 340
y = 170
Thus, the two friends had Rs 40 and Rs 170 with them.
Concept insight: This problem talks about the amount of capital with two friends. So, we will represent them by variables x and y respectively. Now, using the given conditions, a pair of linear equations can be formed which can then be solved easily using elimination method.
Solution 23
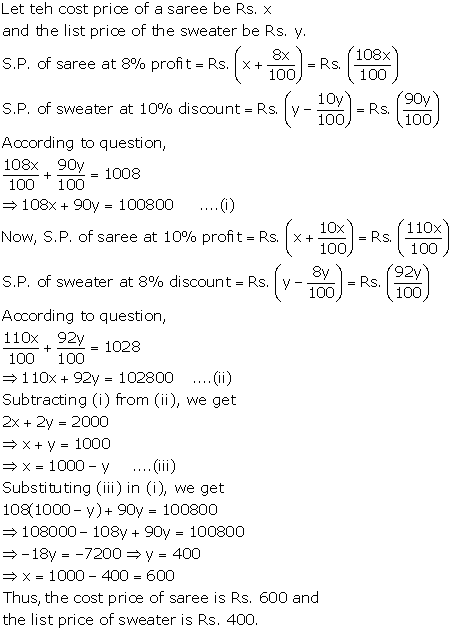
Solution 24
Solution 25
Pairs of Linear Equations in Two Variables Exercise 3.114
Solution 1
So, the correct option is (b).
Solution 2
Solution 3
Solution 4
Solution 5
Pairs of Linear Equations in Two Variables Exercise 3.115
Solution 6
Solution 7
So, the correct option is (a).
Solution 8
So, the correct option is (b).
Solution 9
So, the correct option is (a).
Solution 10
So, the correct option is (c).
Solution 11
Consistent solution means either linear equations have unique solutions or infinite solutions.
⇒ In case of unique solution; lines are intersecting
⇒ If solutions are infinite, lines are coincident.
So, lines are either intersecting or coincident
So, the correct option is (d).
Solution 12
So, the correct option is (c).
Solution 13
So, the correct option is (b).
Solution 14
So, the correct option is (d).
Solution 15
So, the correct option is (d).
Solution 16
So, the correct option is (a).
Pairs of Linear Equations in Two Variables Exercise 3.116
Solution 17
So, the correct option is (b).
Solution 18
Solution 19
Since x = a and y = b is the solution of given system of equations x - y = 2 and x + y = 4, we have
a - b = 2 ….(i)
a + b = 4 ….(ii)
Adding (i) and (ii), we have
2a = 6 ⇒ a = 3
⇒ b = 4 - 3 = 1
Hence, correct option is (a).
Solution 20
Solution 21
