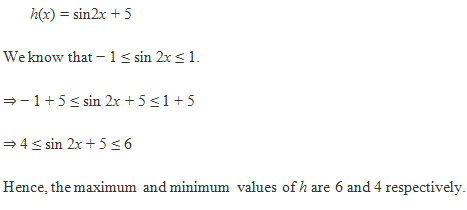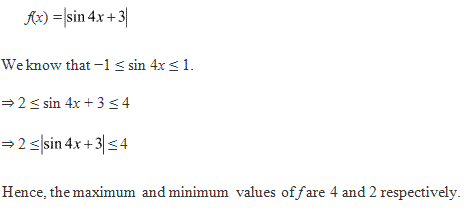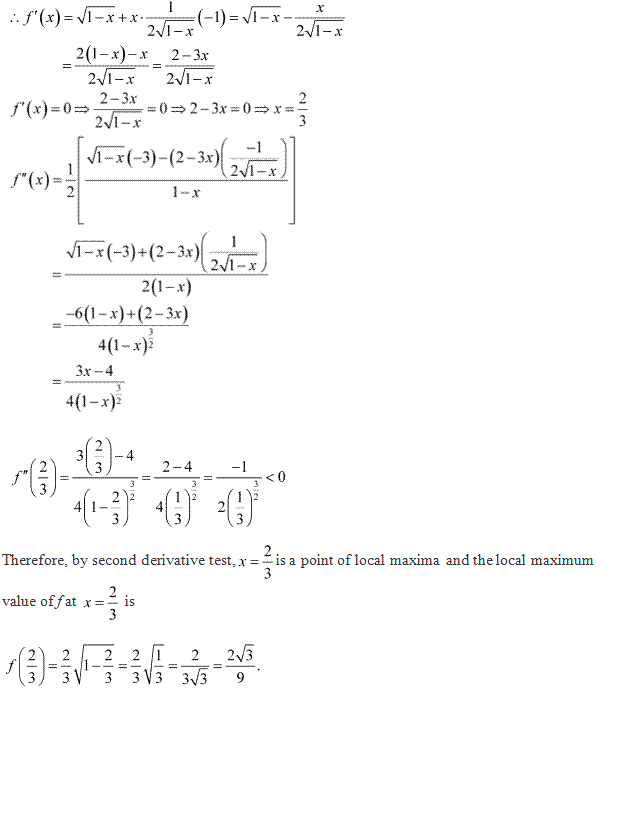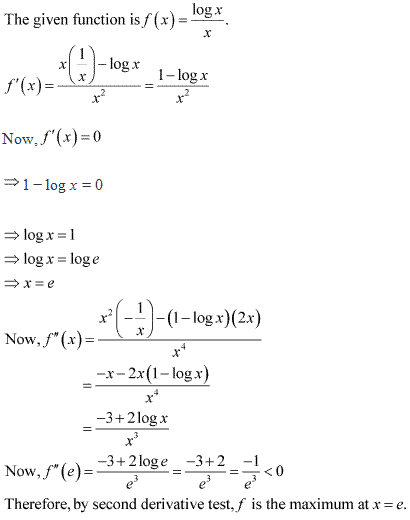Class 12-science RD SHARMA Solutions Maths Chapter 18 - Maxima and Minima
Maxima and Minima Exercise MCQ
Solution 30
Given: 
is defined as f(x) = 2x + cos
x
Differentiating w.r.t x, we get
f'(x) = 2 - sin x
As -sin x ≥ -1
Therefore, 1 - sin x > 0 for all x.
Hence, f(x) is an increasing function.
Solution 31
Let 
Differentiating w.r.t x, we get
f'(x) = 2x - 8
Take f'(x) = 0, we get
2x - 8 = 0
i.e. x = 4
Again differentiating, we get
f"(x) = 2 > 0 for all real x
Therefore, x = 4 is the point of minima.
The minimum value of f(x) is
f(4) = 16 - 32 + 17 = 1
Solution 32
Let 
Differentiating w.r.t x, we get
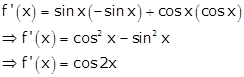
Take f'(x) = 0, we get
cos 2x = 0
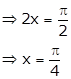
Again differentiating, we get
At 
Therefore, 
is the point of
maxima.
The maximum value of f(x) is
Solution 33
Let 
Taking log on both the sides, we get
… (i)
Differentiating w.r.t x, we get
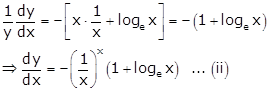
Differentiating w.r.t x, we get
… (iii)
From equation (ii), we get
Take 
we get
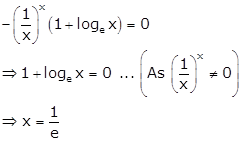
At 
we have
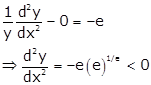
Therefore, 
is the point of
maxima.
The maximum value of f(x) is
Solution 34
Let 
Taking log on both the sides, we get
… (i)
Differentiating w.r.t x, we get
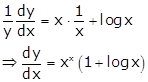
Take we get
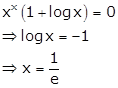
Thus, is the
stationary point.
Solution 35
Given: 
Differentiating w.r.t x, we get
which is the
slope of the curve
Differentiating 
w.r.t x, we
get
Take 
Again differentiating w.r.t x, we get
So, the slope is maximum at x = 1
Solution 36
Given: 
Differentiating w.r.t x, we get
Take f'(x) = 0, we get
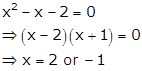
Now, let's find f(x) at x = 2 or -1
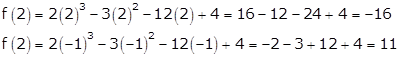
Therefore, x = -1 is the point of local maxima and the maximum value is 11.
Whereas, x = 2 is the point of local minima and the minimum value is -16.
Hence, f(x) has one maximum and one minimum.
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
Solution 20
Solution 21
Solution 22
Solution 23
Solution 24
Solution 25
Solution 26
Solution 27
Solution 28
Solution 29
Maxima and Minima Exercise Ex. 18.1
Solution 1
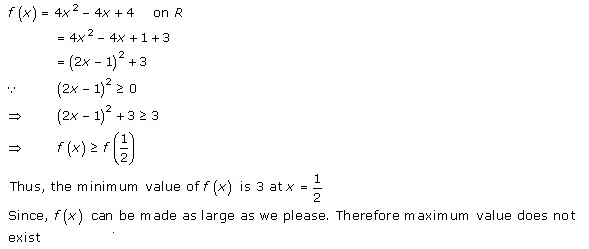
Solution 2
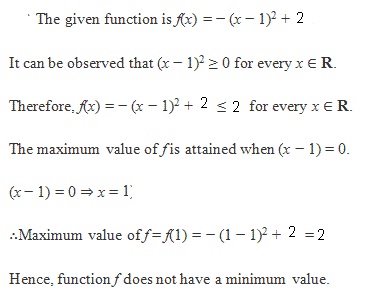
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
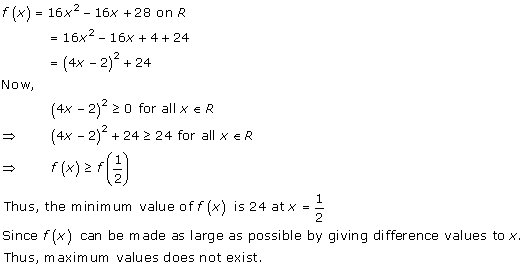
Solution 9
Maxima and Minima Exercise Ex. 18.2
Solution 1
Solution 2
Solution 3
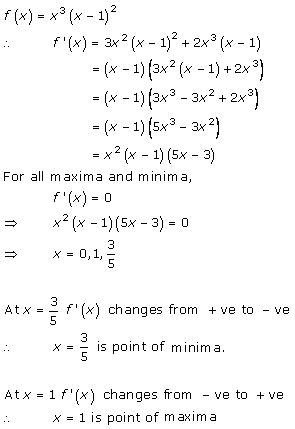
Solution 4
Solution 5
Solution 6
Solution 7
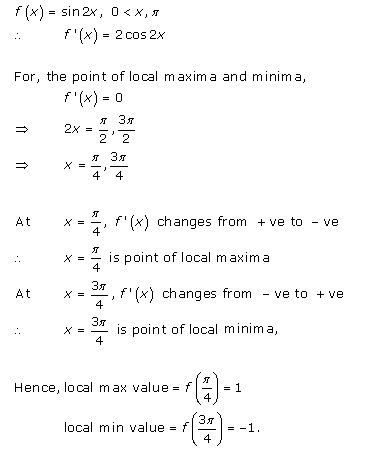
Solution 8
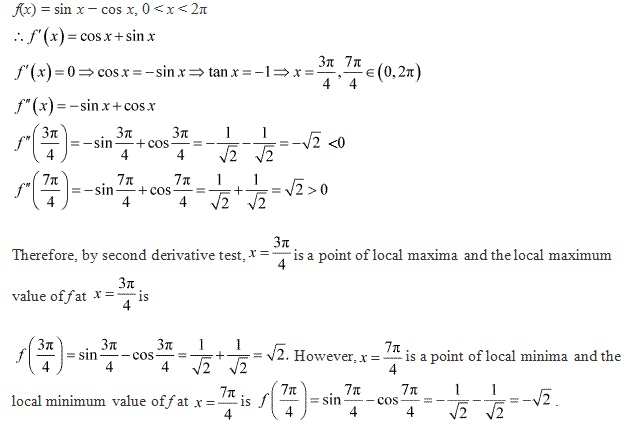
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
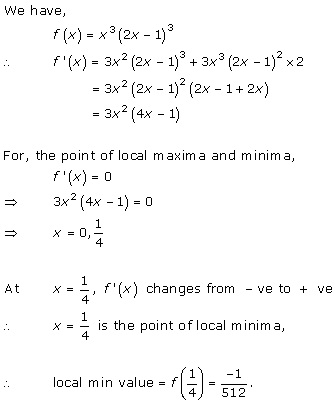
Solution 14
Maxima and Minima Exercise Ex. 18.3
Solution 1(i)
Solution 1(ii)
Solution 1(iii)
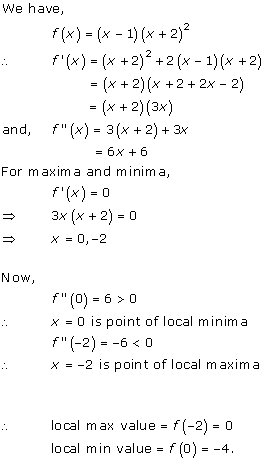
Solution 1(iv)
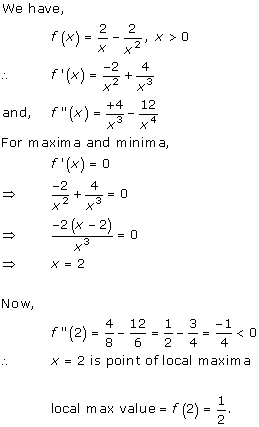
Solution 1(v)
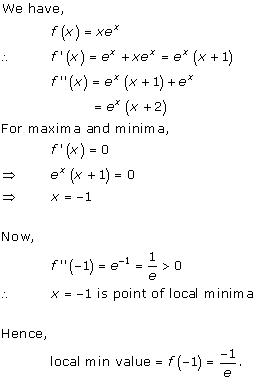
Solution 1(vi)
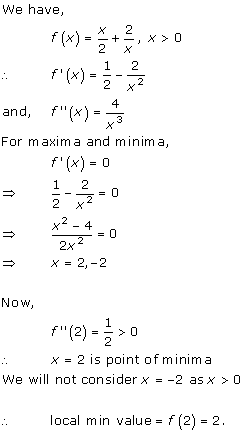
Solution 1(vii)
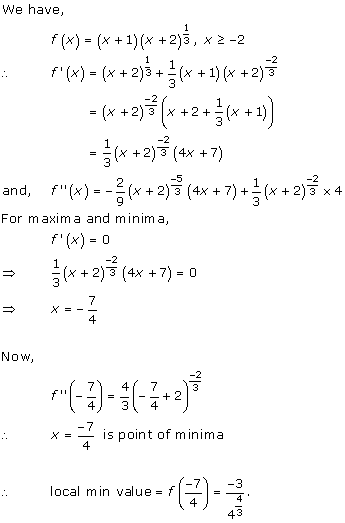
Solution 1(viii)
Solution 1(ix)
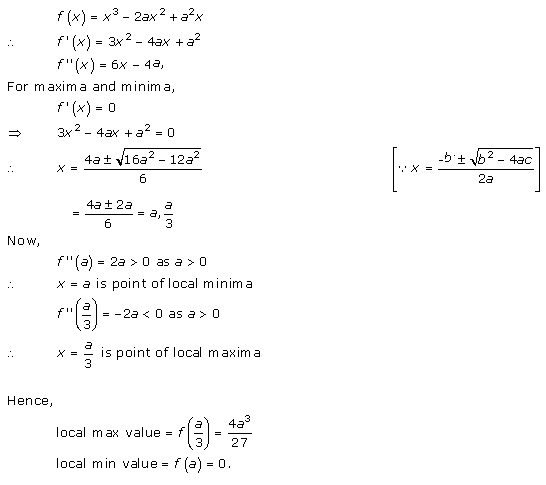
Solution 1(x)
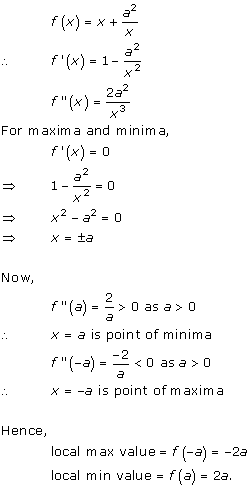
Solution 1(xi)
Solution 1(xii)
Solution 2(i)
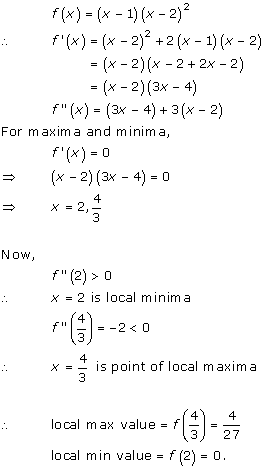
Solution 2(ii)
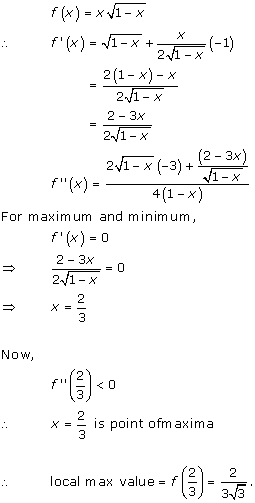
Solution 2(iii)
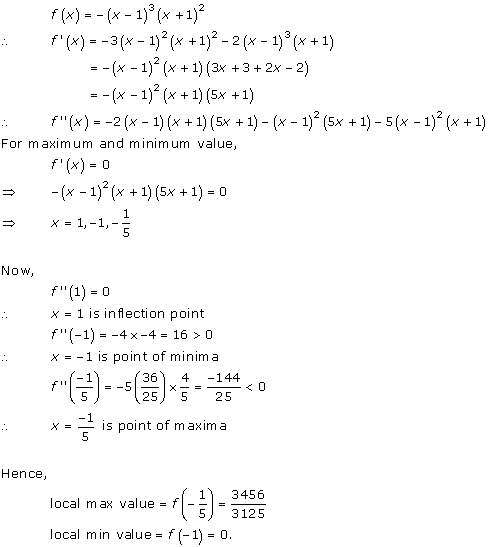
Solution 3
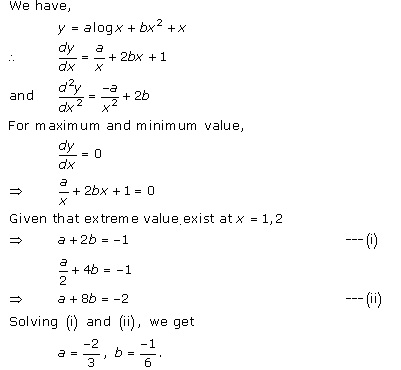
Solution 4
Solution 5
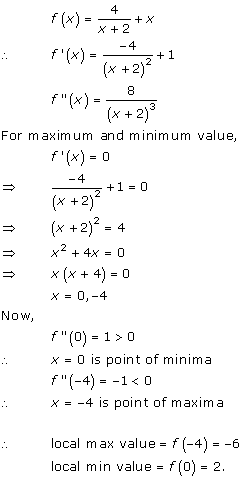
Solution 6
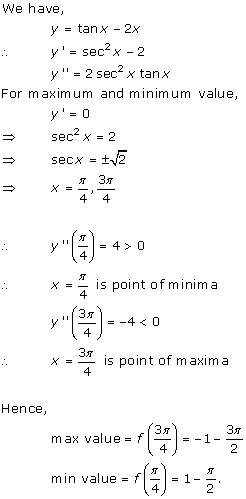
Solution 7
Solution 8
Given: 
Differentiating w.r.t x, we get
Take f'(x) = 0
Differentiating f'(x) w.r.t x, we get
At 
Clearly, f"(x)
< 0 at
Thus, is the maxima.
Hence, f(x) has
maximum value at 
.
Maxima and Minima Exercise Ex. 18.4
Solution 1(i)
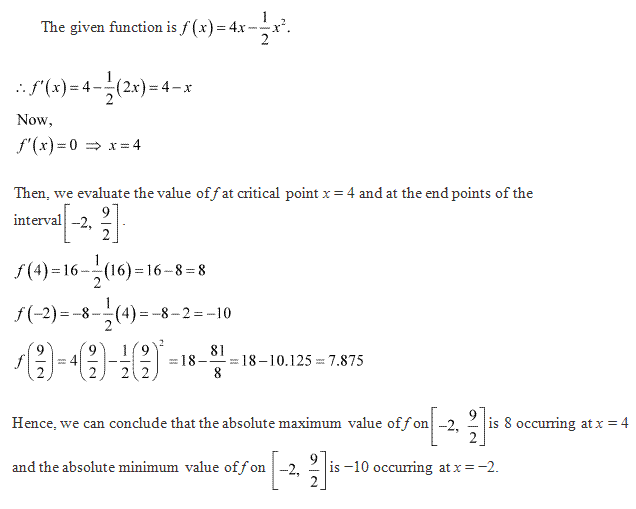
Solution 1(ii)
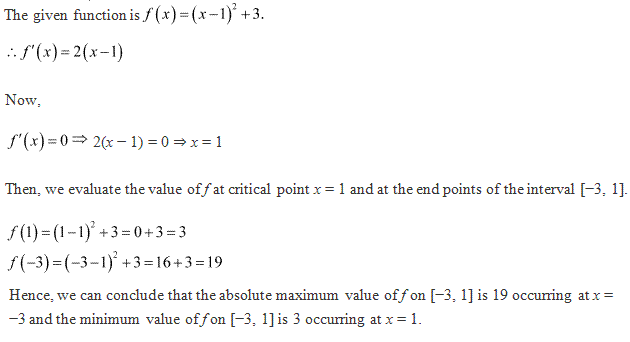
Solution 1(iii)
Solution 1(iv)
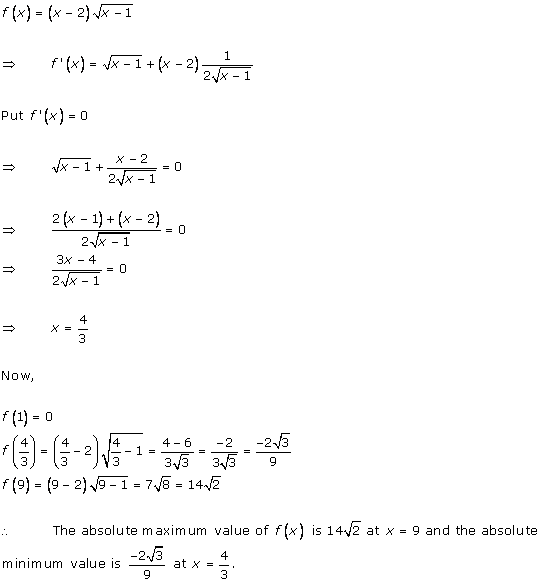
Solution 2
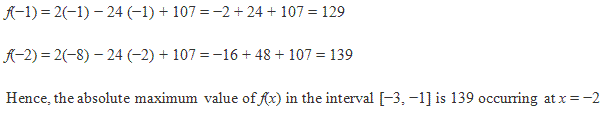
Solution 3
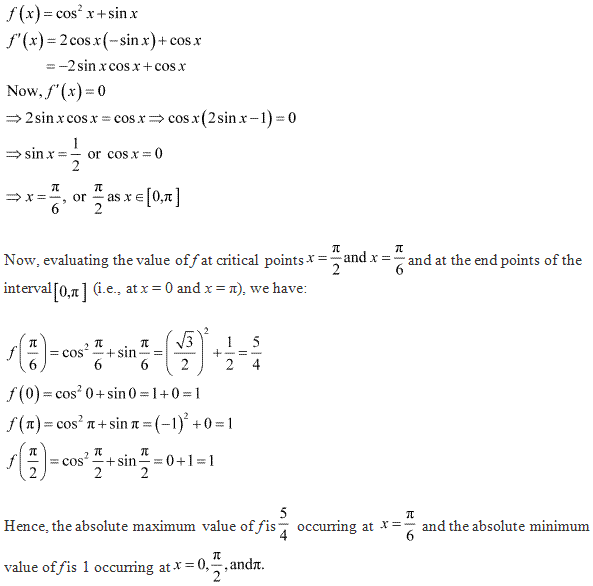
Solution 4
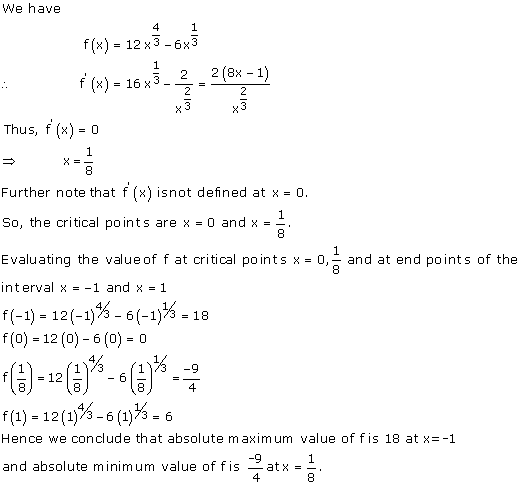
Solution 5
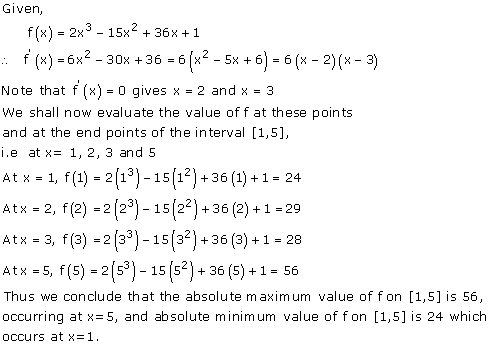
Maxima and Minima Exercise Ex. 18.5
Solution 1
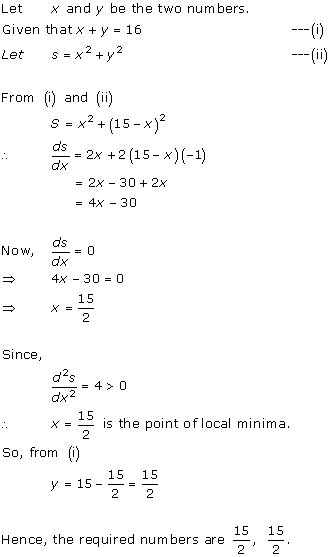
Solution 2
Solution 3
Solution 4
Solution 6
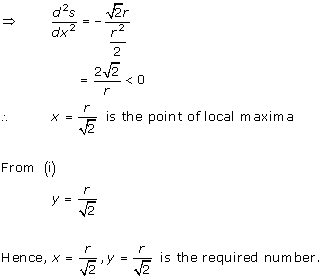
Solution 7
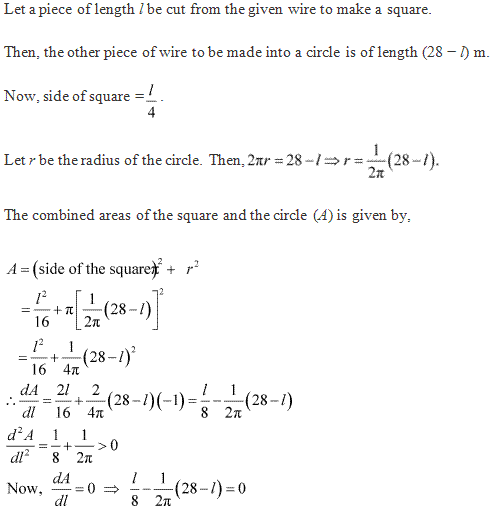
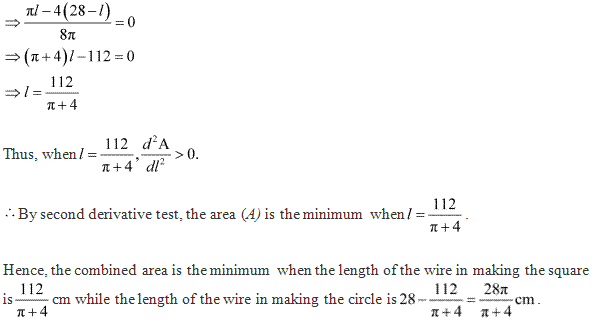
Solution 8
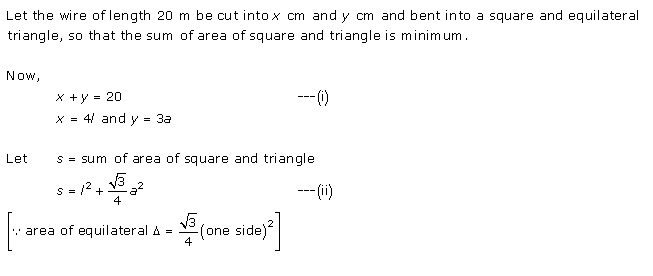
Solution 9
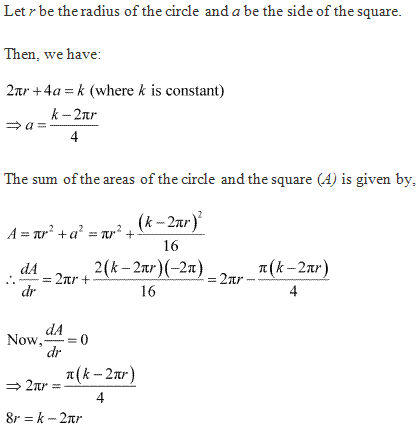
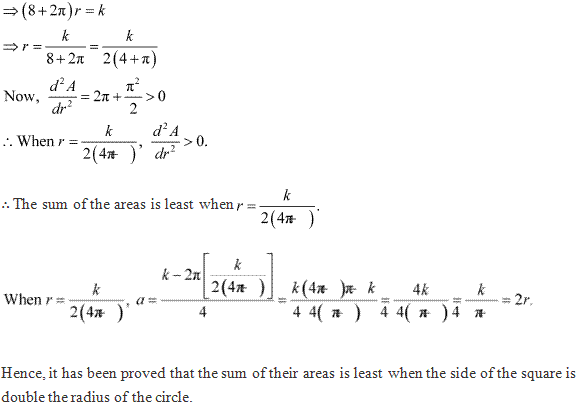
Solution 10
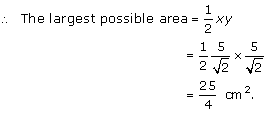
Solution 11
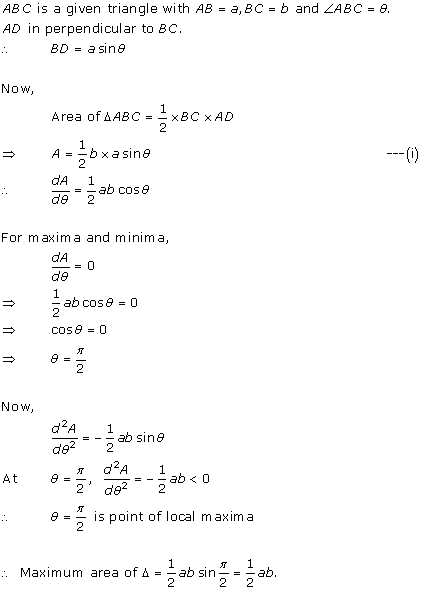
Solution 12
Solution 13
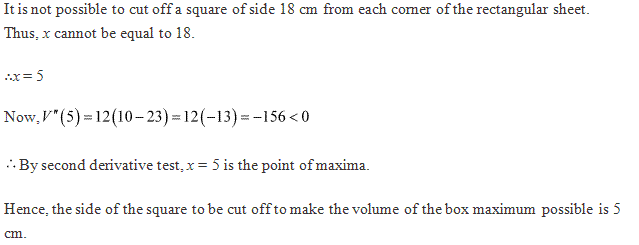
Solution 14
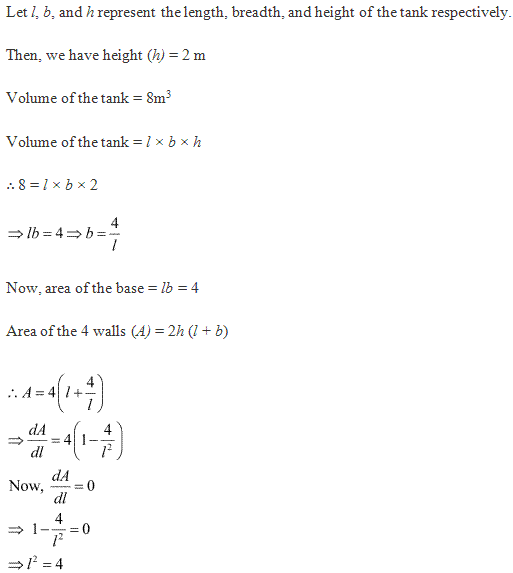
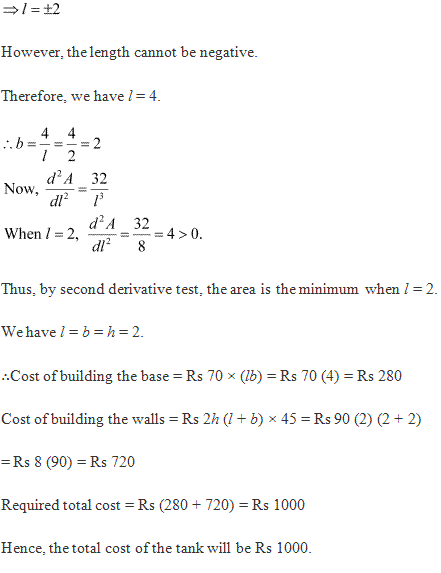
Solution 15
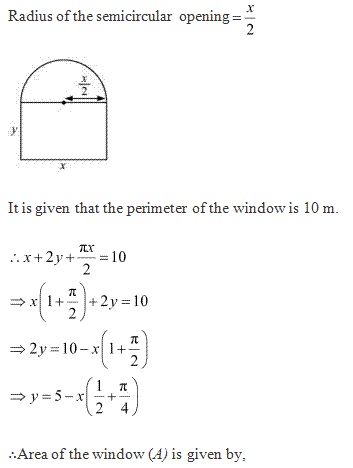
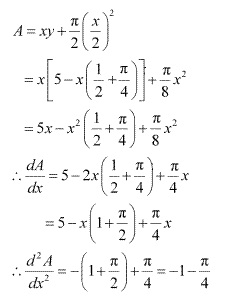
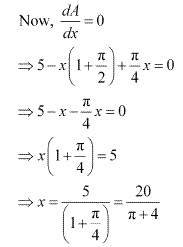
Solution 16
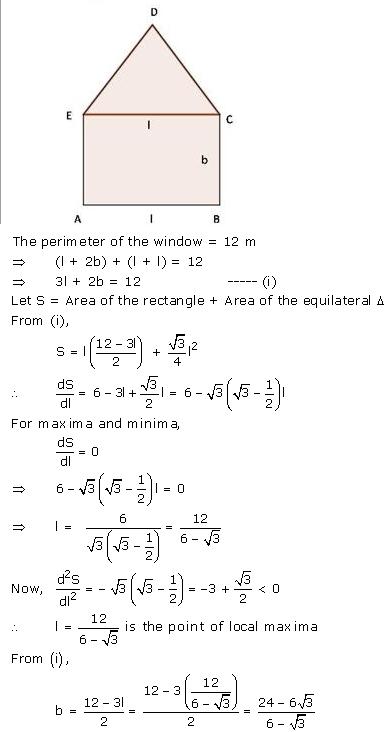
Solution 17
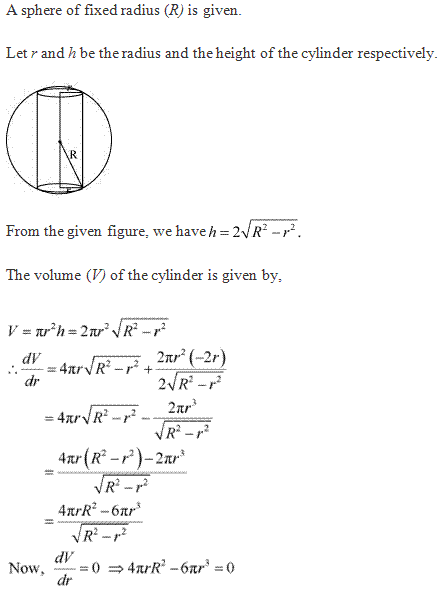
Solution 18
Solution 19
Solution 20
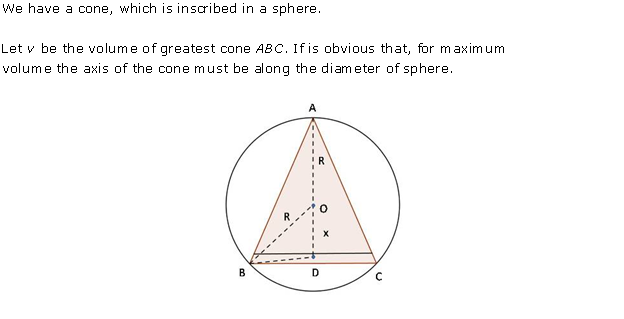
Solution 21
Solution 22
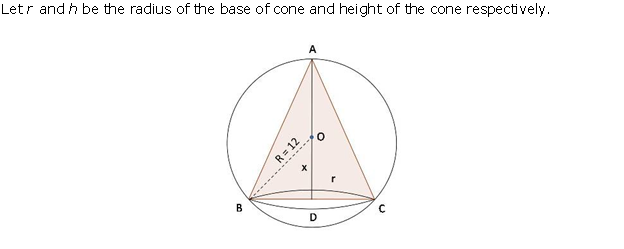
Solution 24
Solution 25
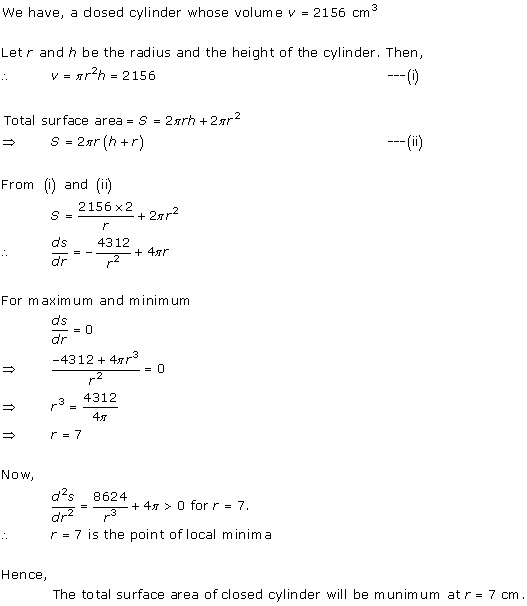
Solution 26
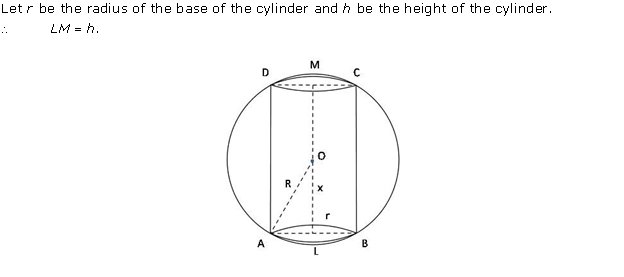
Solution 27
Solution 28
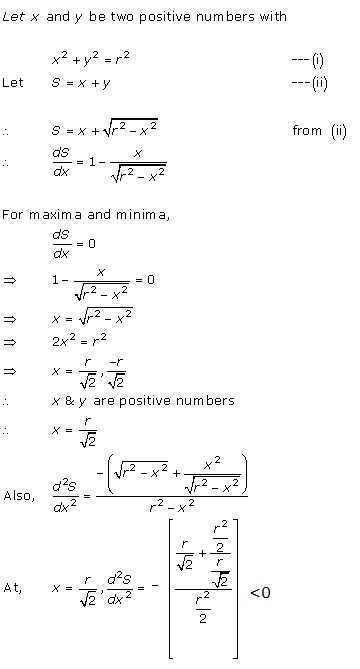
Solution 29
Solution 30
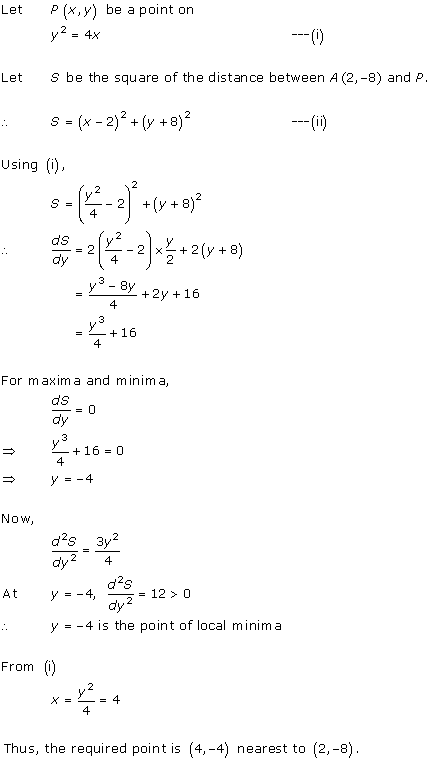
Solution 31
Solution 32
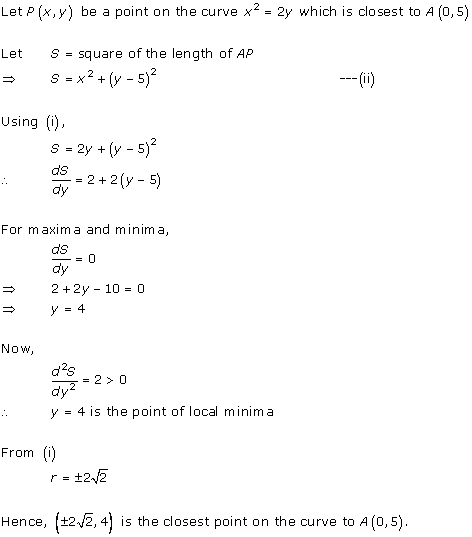
Solution 33
Solution 34
Solution 35
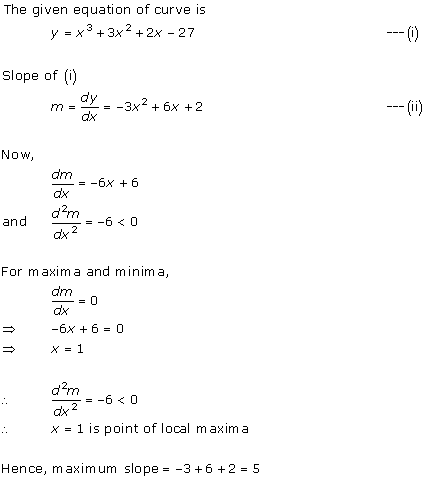
Solution 36
Solution 37
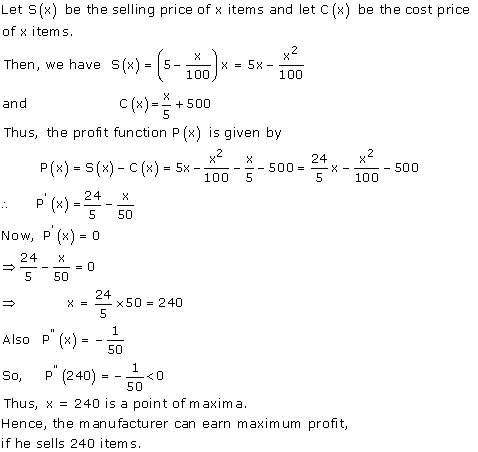
Solution 38
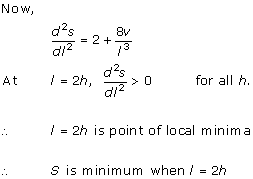
Solution 39
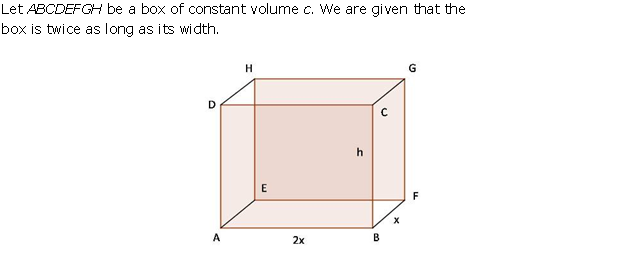
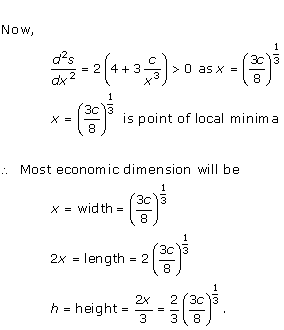
Solution 40
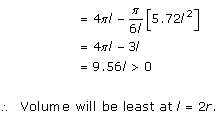
Solution 41
Solution 42
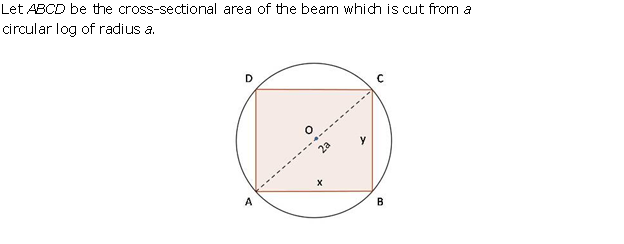
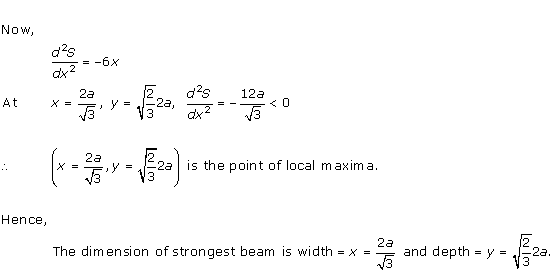
Solution 43
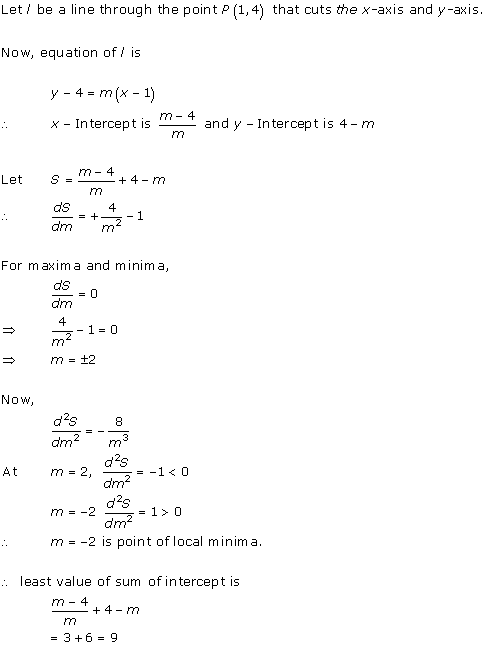
Solution 44
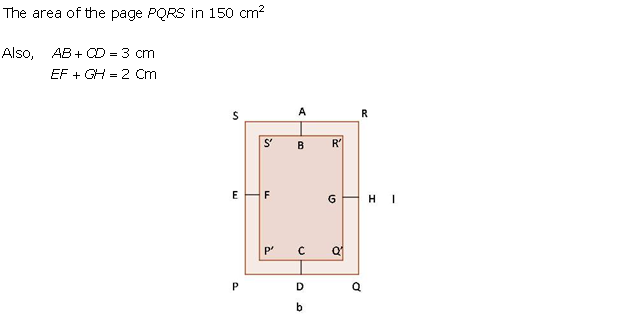
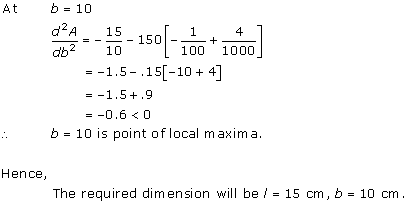
Solution 45
Solution 45
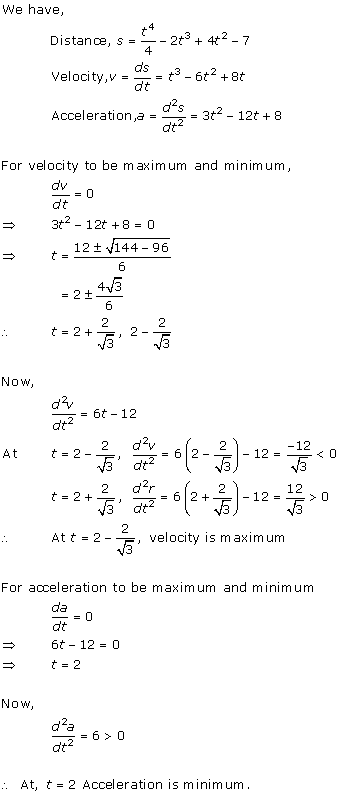
Solution 5
Let r and h be the radius and height of the cylinder.
Volume of
cylinder 
… (i)
Surface area of
cylinder 
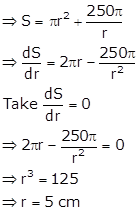
From (i), we get
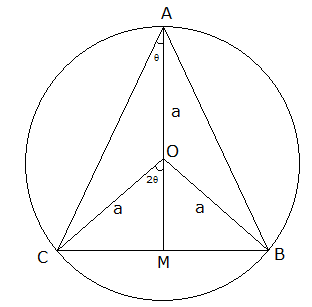
Solution 23
Let 
be an
isosceles triangle with AB = AC.
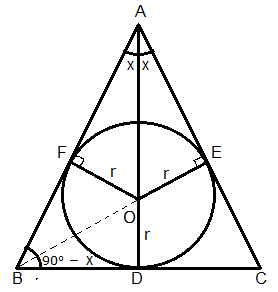
Let 
Here, AO bisects 
Taking O as the centre of the circle, join OE, OF and OD such that
OE = OF = OD = r (radius)
Now, 
In 
Similarly, AF = r cot x
In 
As OB bisect 
we have
In 
Similarly, BD =
DC = CE = 
We have,
perimeter of
P = AB + BC + CA
= AE + EC + BD + DC + AF + BF
Differentiating w.r.t x, we get
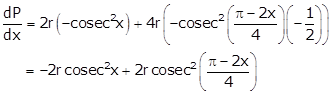
Taking 
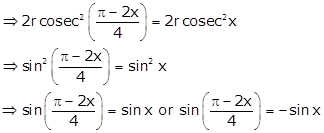
As 
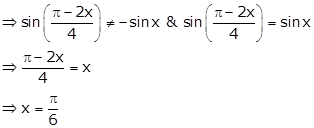
Therefore, 
is an
equilateral triangle.
Taking second derivative of P, we get
At 
Therefore, the
perimeter is minimum when 
Least value of P 
Maxima and Minima Exercise Ex. 18VSAQ
Solution 1
Solution 2
Solution 3
Solution 4
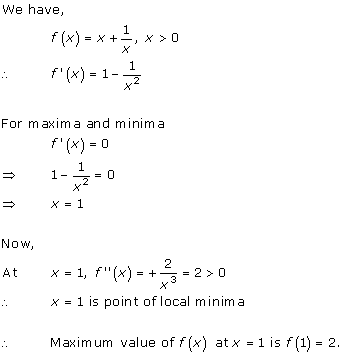
Solution 5
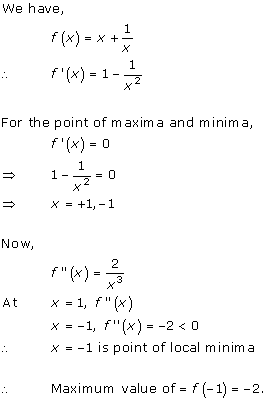
Solution 6
Solution 7
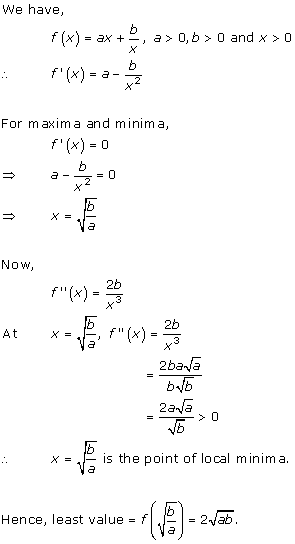
Solution 8
Solution 9
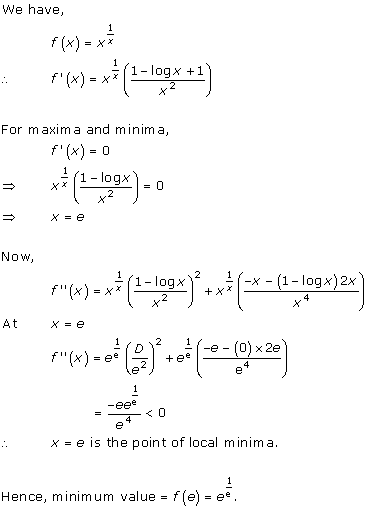
Solution 10
