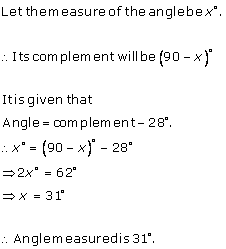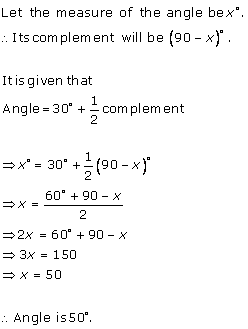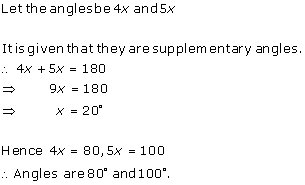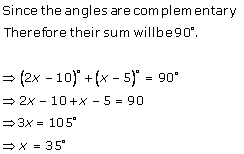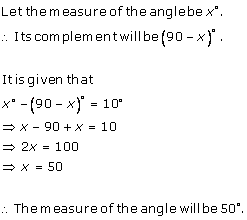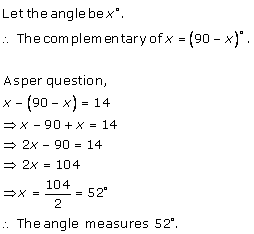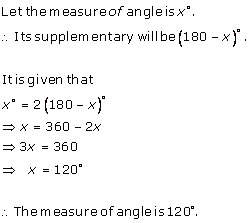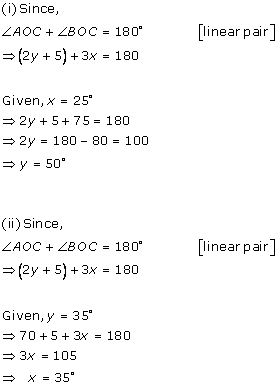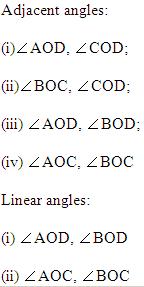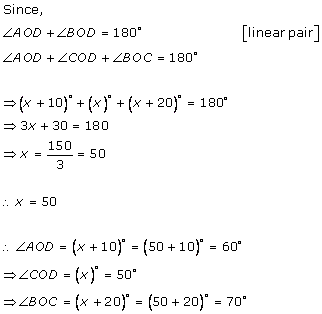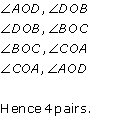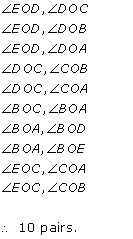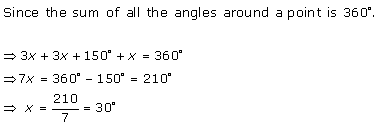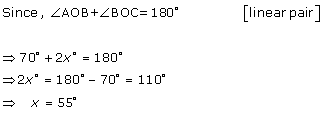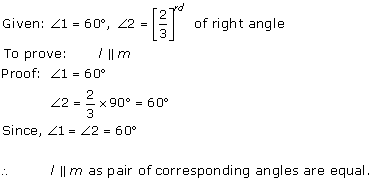Class 9 RD SHARMA Solutions Maths Chapter 10 - Lines and Angles
Lines and Angles Exercise Ex. 10.1
Solution 1
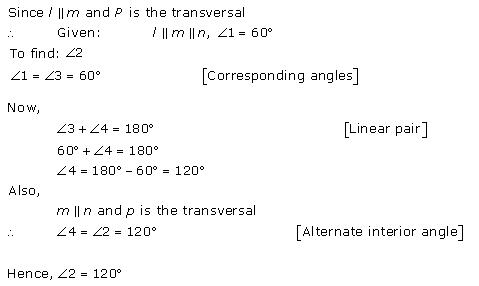
Solution 2
(i) 54°
Since, the sum of an angle and its supplement is 180°
∴Its supplement will be 180° - 54° = 126°.
(ii) 132°
Since, the sum of an angle and its supplement is 180°
∴Its supplement will be 180° - 132° = 48°.
(iii) 138°
Since, the sum of an angle and its supplement is 180°
∴Its supplement will be 180° - 138° = 42°.
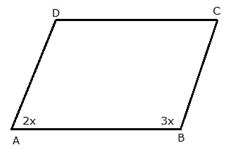
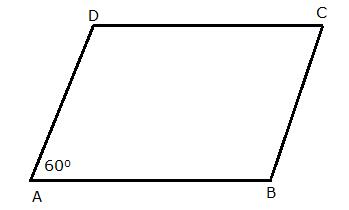
Solution 3
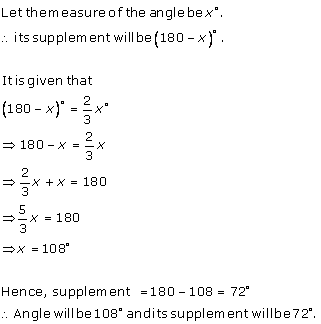
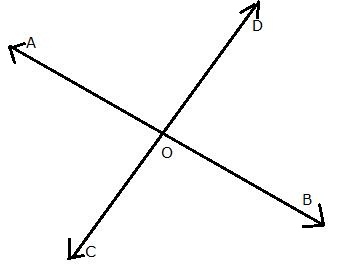
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
Let the measure of the angle be xo.
Its complement will be (90o - xo) and its supplement will be (180o - xo).
Supplement of thrice of the angle = (180o - 3xo)
According to the given information:
(90o - xo) = (180o - 3xo)
3x - x = 180 - 90
2x = 90
x = 45
Thus, the measure of the angle is 45o.
The measure of the angle is 45o
Solution 14
Lines and Angles Exercise Ex. 10.2
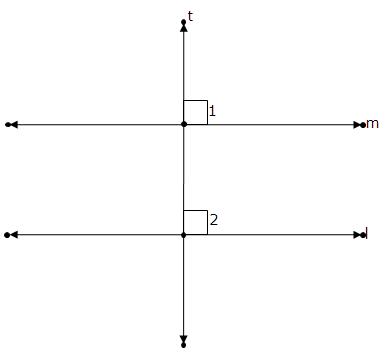
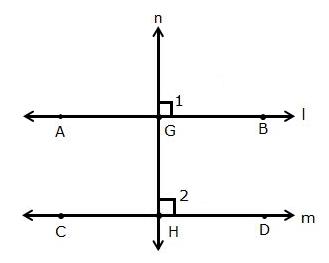
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
Lines and Angles Exercise Ex. 10.3
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
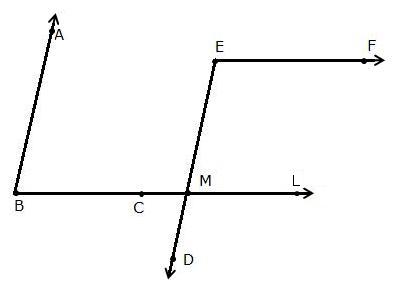
Solution 6
Solution 7

Solution 8
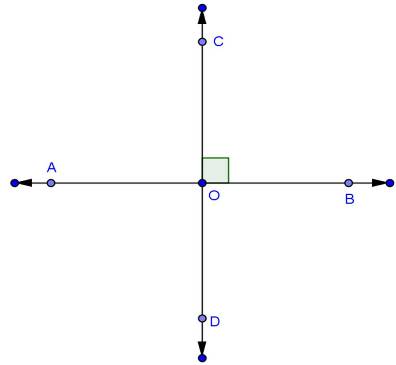
Solution 9
Solution 10
(i) True
(ii) False
(iii) False
(iv) True
Solution 11
(i) obtuse.
(ii) 180o
(iii) uncommon
Solution 12
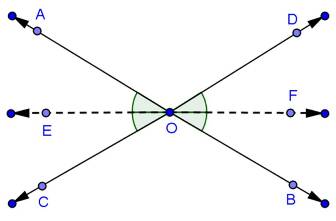
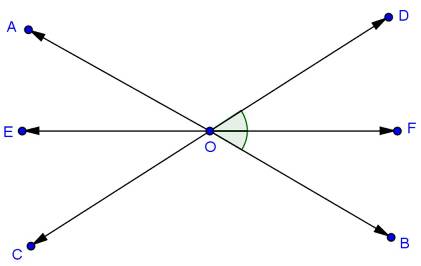
Solution 13
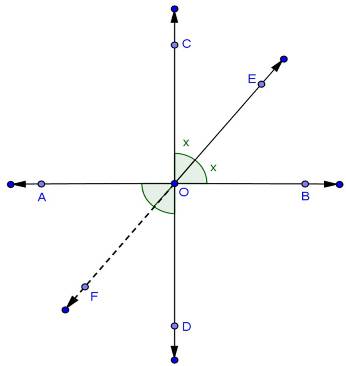
Lines and Angles Exercise Ex. 10.4
Solution 1
Solution 2
Solution 20
Solution 3
Solution 4
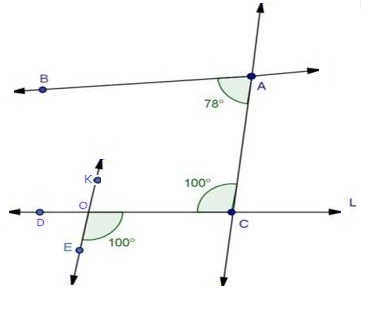
Solution 5
Solution 6
Solution 7
Solution 8

Solution 9
Solution 10
Solution 11
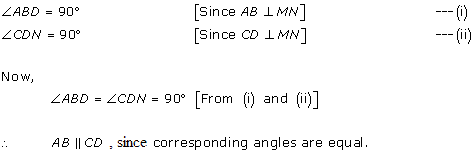
Solution 12
Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
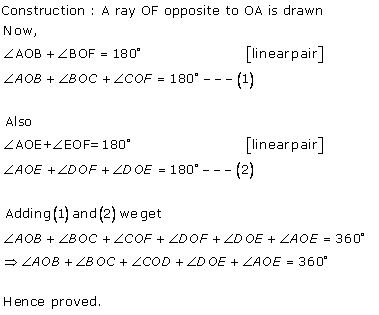
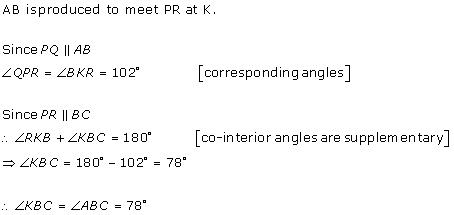
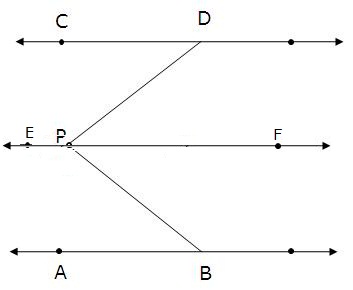
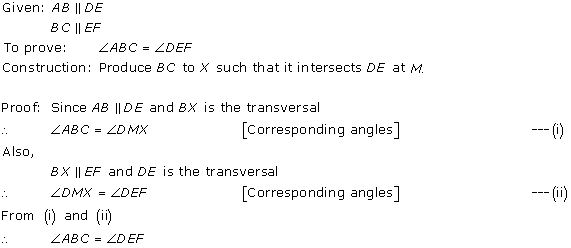
Solution 21
Solution 22
Solution 23
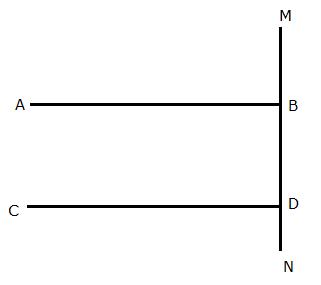
Consider the angles AOB and ACB.

Solution 24
Solution 25
Solution 26
Solution 27
Lines and Angles Exercise 10.51
Solution 1

Solution 2
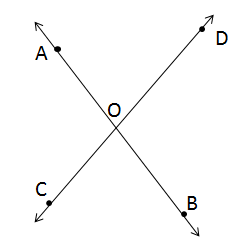

Solution 3
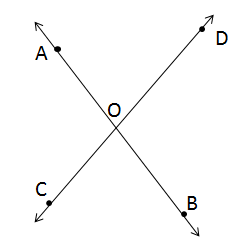

Solution 4
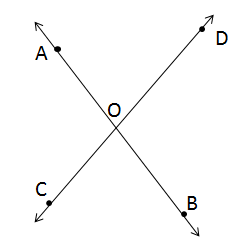

Solution 5
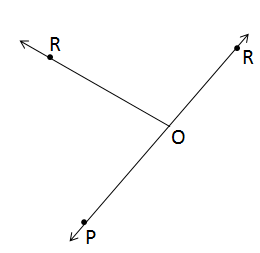

Solution 6
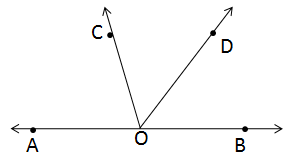

Solution 7
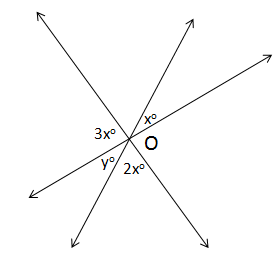

Lines and Angles Exercise 10.52
Solution 8
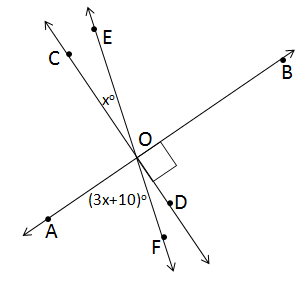

Solution 9
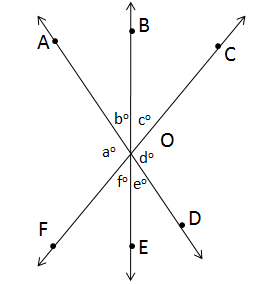

Lines and Angles Exercise 10.53
Solution 10
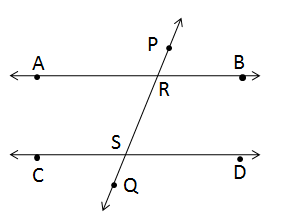

Solution 11
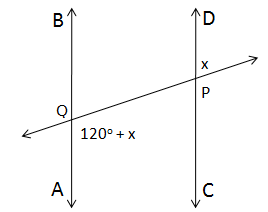

Solution 12
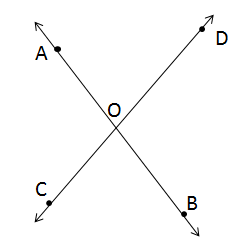

Solution 13
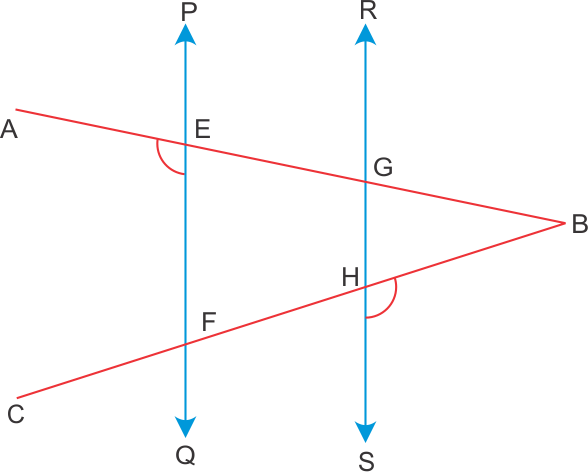

Solution 14
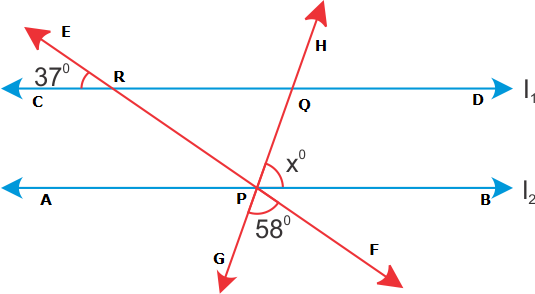

Lines and Angles Exercise 10.54
Solution 15
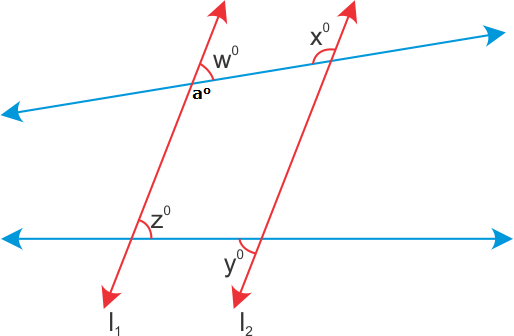

Solution 16
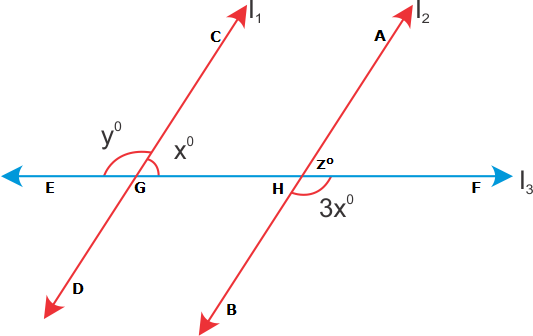

Solution 17


Lines and Angles Exercise 10.55
Solution 18
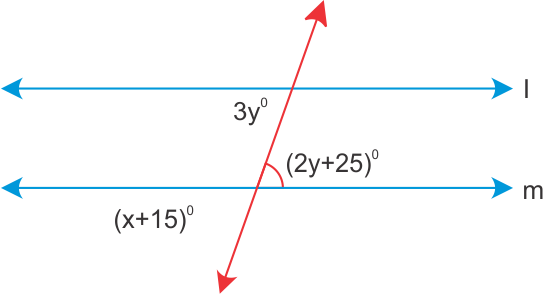

Solution 19
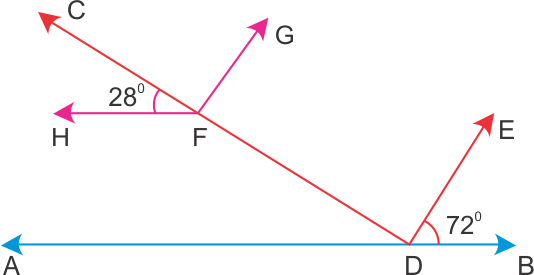

Solution 20
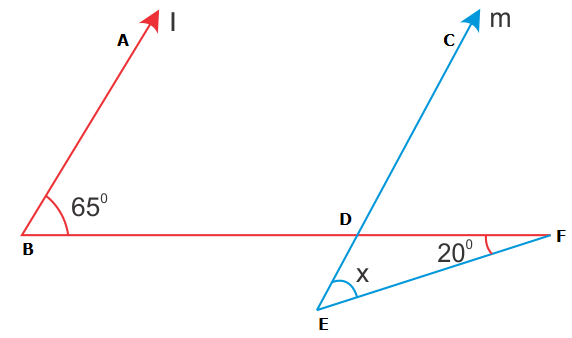

Solution 21
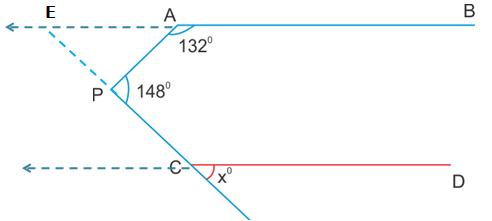

Lines and Angles Exercise 10.56
Solution 22
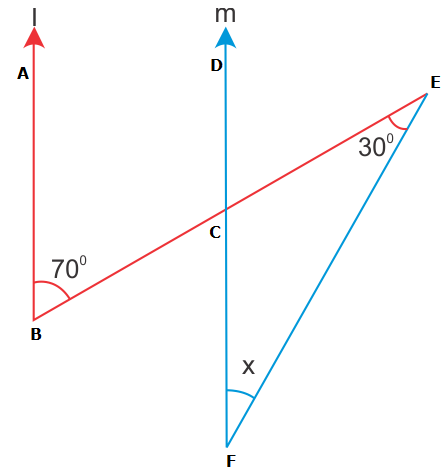

Solution 23
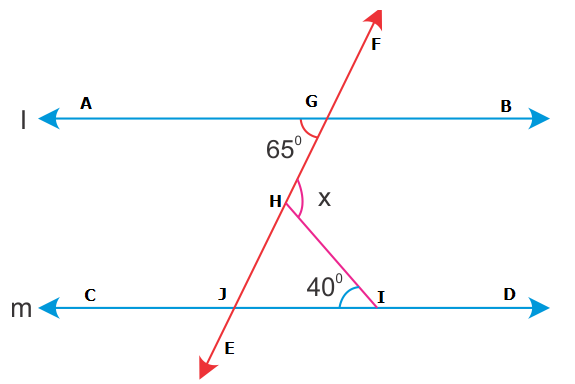

Solution 24


Solution 25
Correct option (c)
Let one angle be θ
Then, its complementary = 90 - θ
According to question,
2θ = 3(90 - θ)
5θ = 270
θ = 54°
Then, 90 - θ° = 36°
Hence, the smaller angle is 36°.
Hence, correct option is (c).
Lines and Angles Exercise 10.57
Solution 26
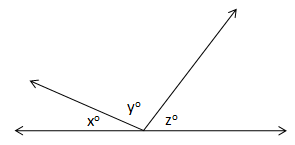

Solution 27


Solution 28
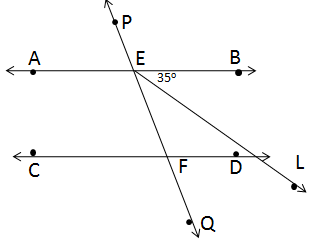

Solution 29
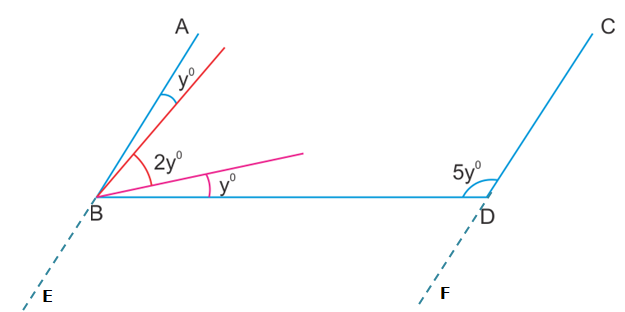

Solution 30