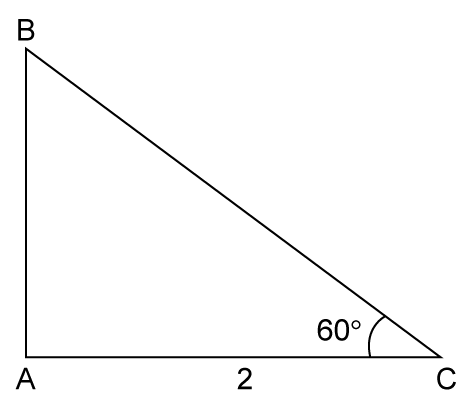
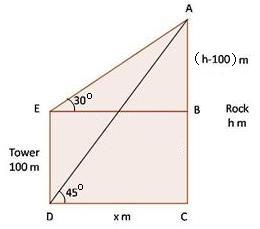
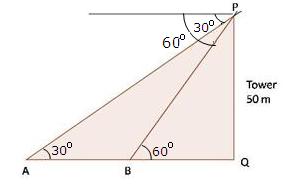
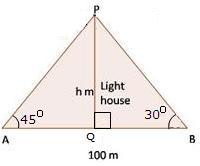
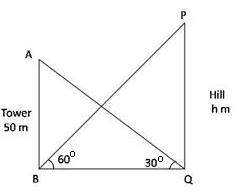
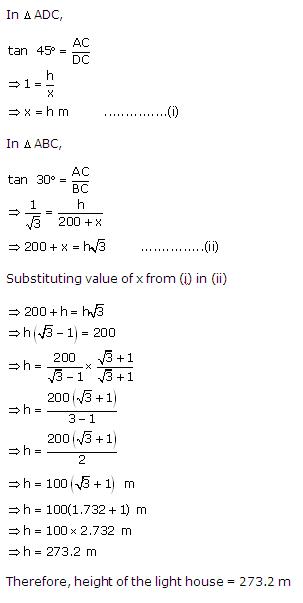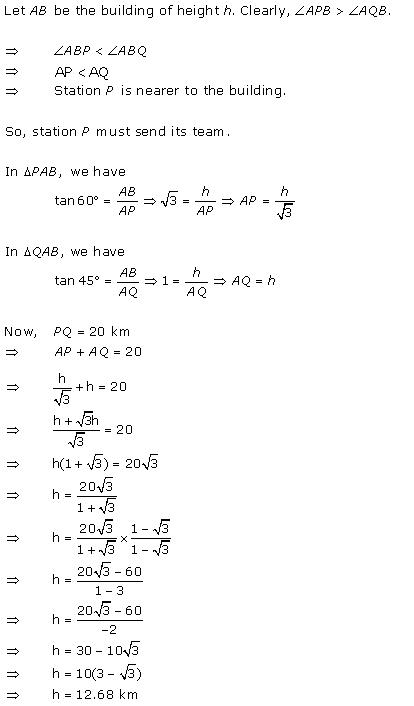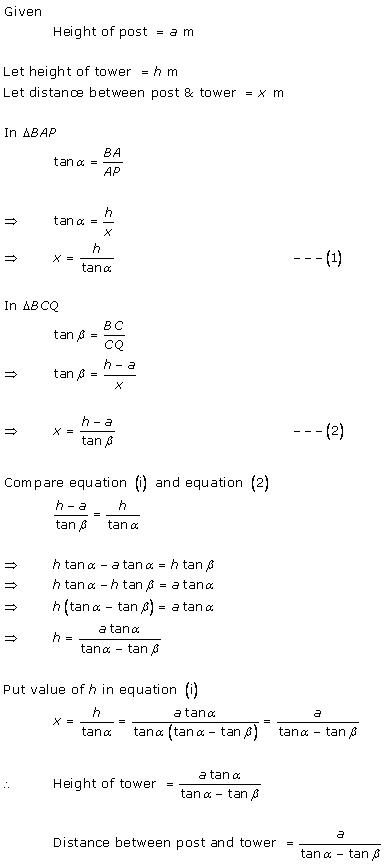Class 10 RD SHARMA Solutions Maths Chapter 12 - Heights and Distances
You can expect the right type of step-by-step explanations from TopperLearning’s class 10 R.D Sharma Solutions for Chapter 12 - Heights And Distances. The solutions come with all the concept-wise questions covering important areas from the chapter. Because everything is readily available on the website and a dedicated TopperLearning App, you can start learning and revising answers on time.
Professionally trained subject matter experts have devised the question and answers discussed within, abiding by the latest CBSE class 10 Maths guidelines. Your grades in Maths will improve with practice. Also, all the helpful resources (video lessons, ask a doubt, revision notes, sample papers and previous years' question papers) made available here stand as the ultimate help, making learning super easy. Eventually, you will score better grades, standing out from the rest of the class.
TopperLearning’s R.D. Sharma Solutions is a one-stop guide for students, offering easy access to thoroughly illustrated examples and step-by-step answers in Heights And Distances. Whether you are stuck with any particular question while measuring the heights, or it is the distance part you find hard, easy-to-understand answers will help you make it through. Further, TopperLearning makes class 10 easy with a series of subject-wise important questions, fill-in-the-blanks, multiple-choice questions, subjective questions and important revision notes.
These solutions aim to thoroughly guide you through the essential concepts in Mathematics, with steady answers to the important concepts in this chapter. Because you will appear in class 10 board exams within a few months, preparation and continuous doubt-solving become mandatory, and things get easy with the best resources. Other than R.D Sharma solutions for class 10, at TopperLearning, you can also avail of R.D Sharma solutions for class 9, R.D Sharma solutions for class 11 and R.D Sharma solutions for class 12.
Some Applications of Trigonometry Exercise Ex. 12.1
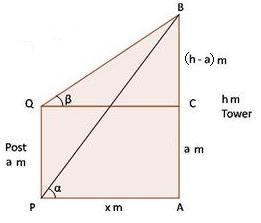
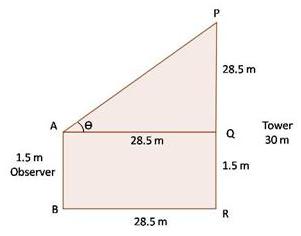
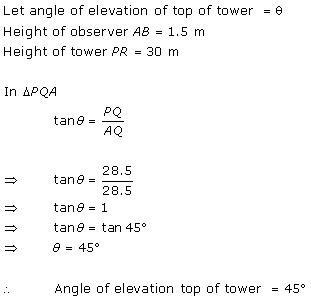
Solution 1
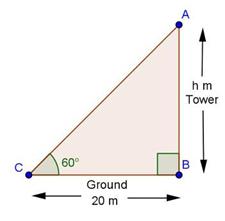

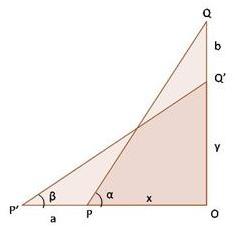
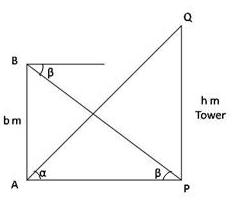
Solution 2
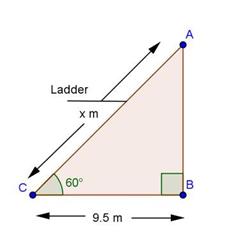

Solution 3
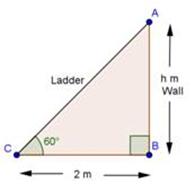
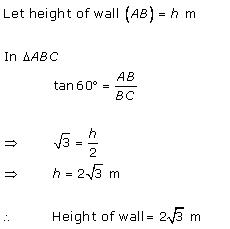
Solution 4


Solution 5
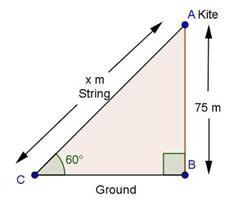
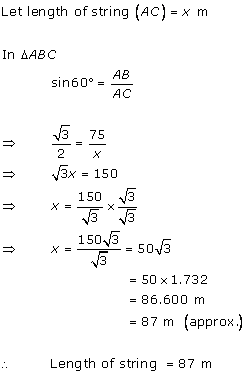
Solution 6
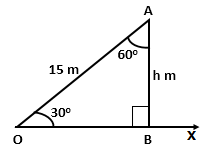

Solution 7


Solution 8
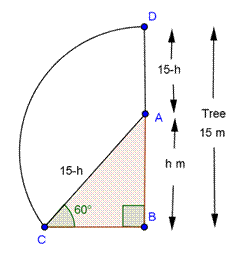

Solution 9
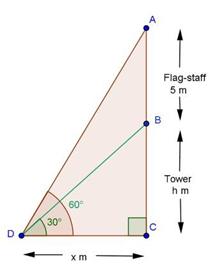
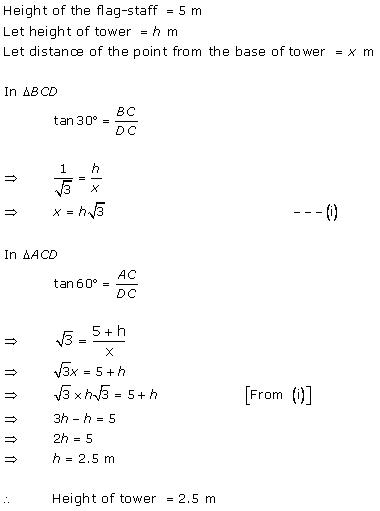
Solution 10


Solution 11

Solution 12
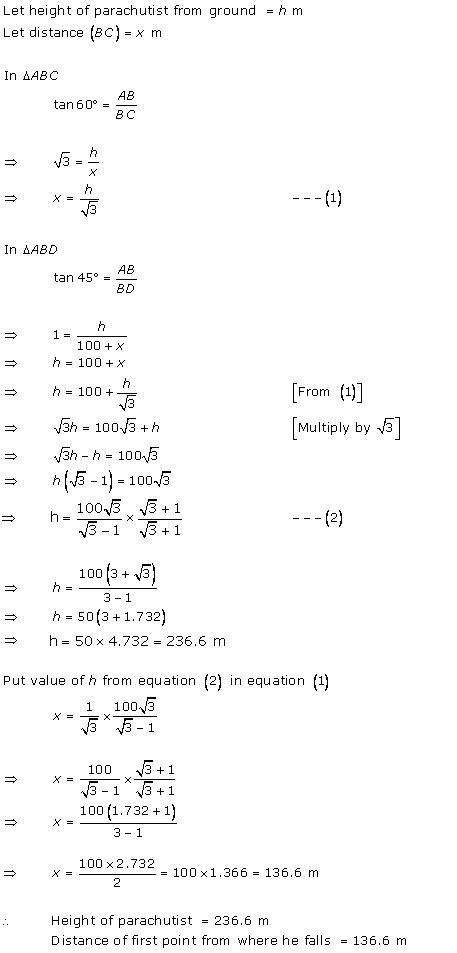
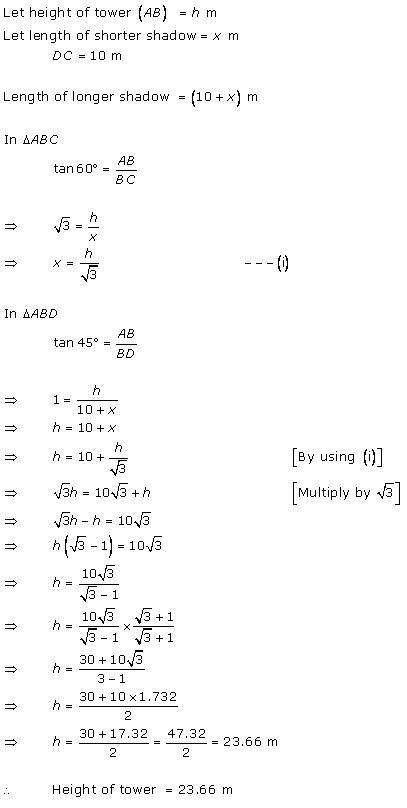
Solution 13
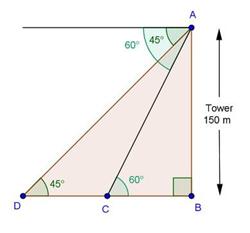

Solution 14
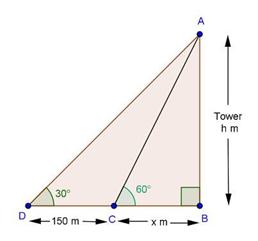
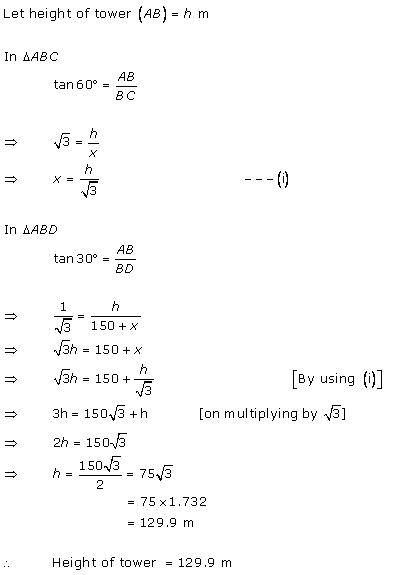
Solution 15
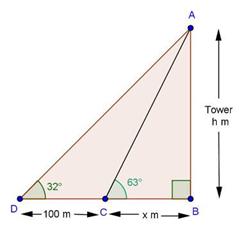

Solution 16
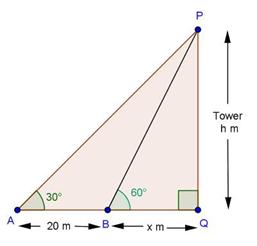
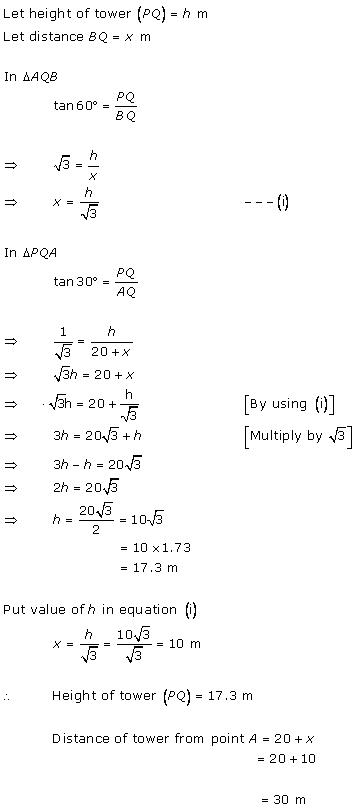
Solution 17


Solution 18
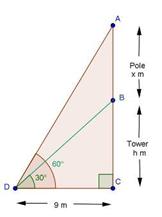
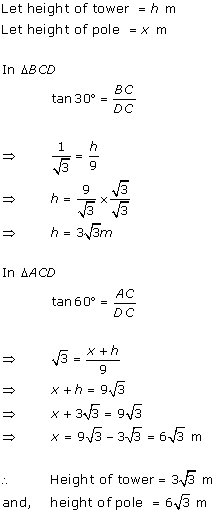
Solution 19
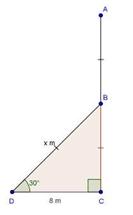
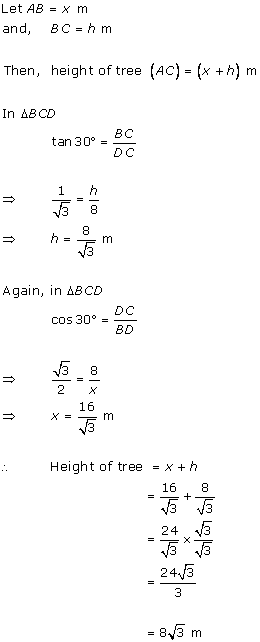
Solution 20
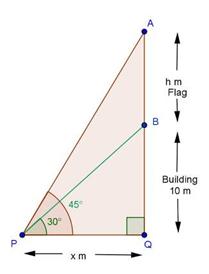

Solution 21
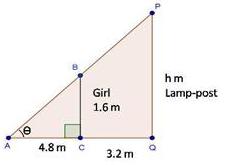

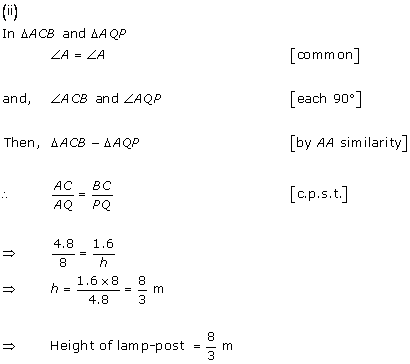
Solution 22
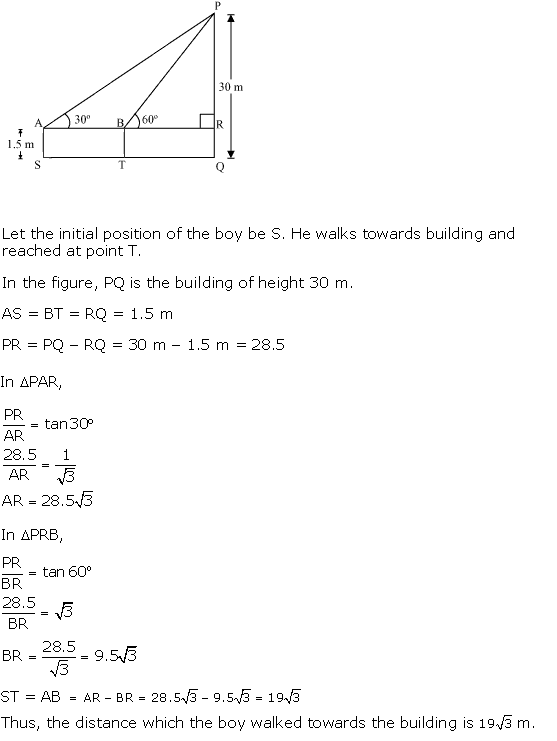
Solution 23
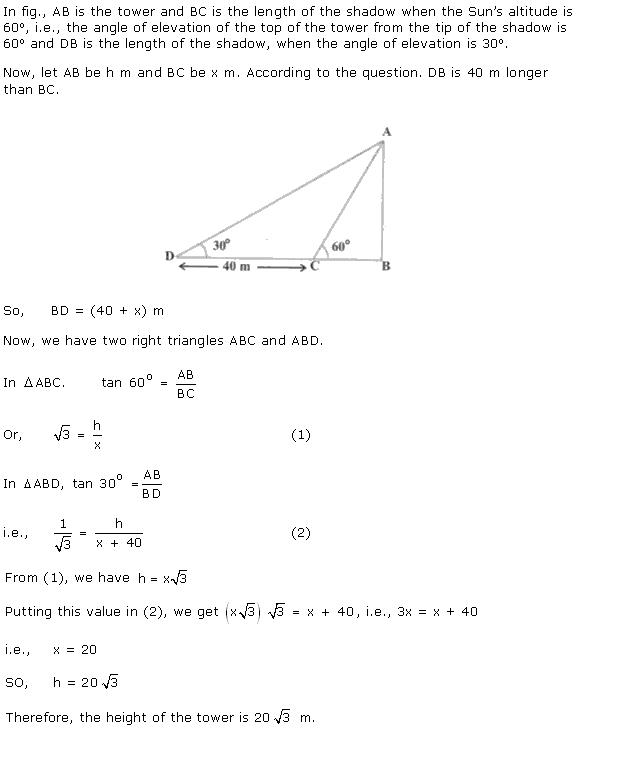
Solution 24
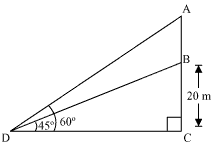
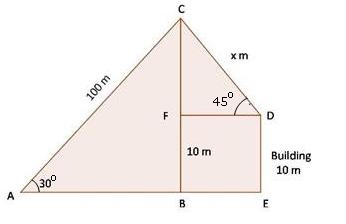
Let BC be the building, AB be the transmission tower, and D be the point on ground from where elevation angles are to be measured.

Solution 25
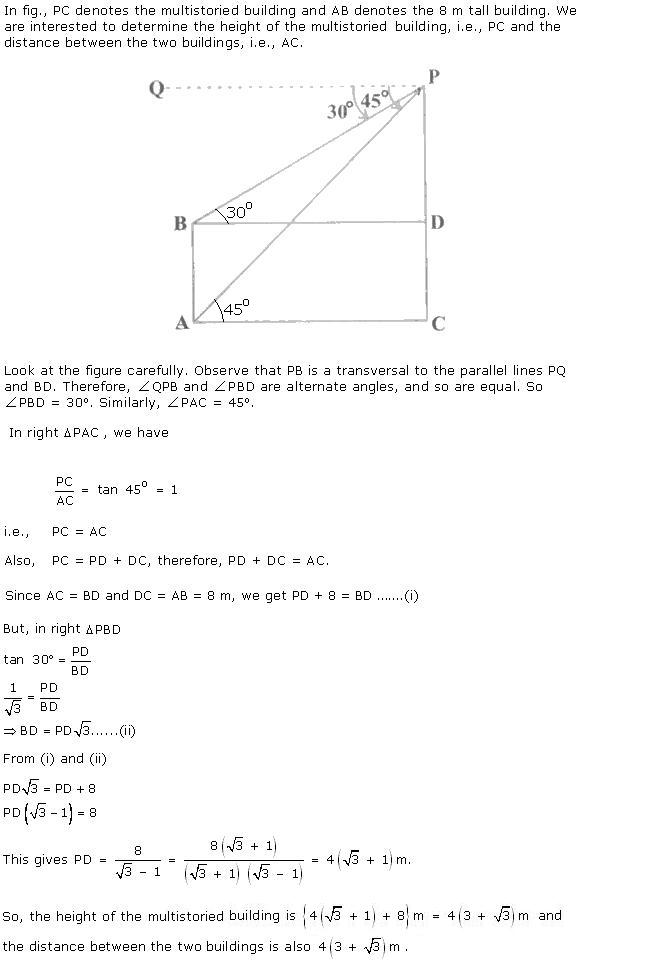
Solution 26

Let AB be the statue, BC be the pedestal and D be the point on ground from where elevation angles are to be measured.
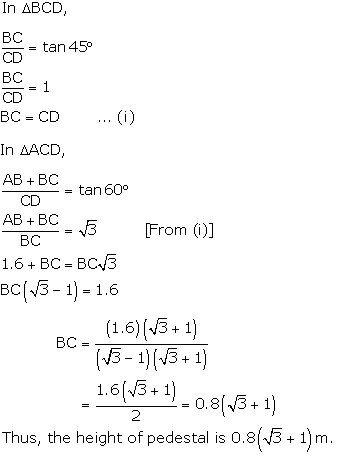
Solution 27
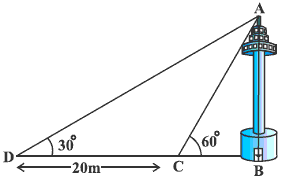
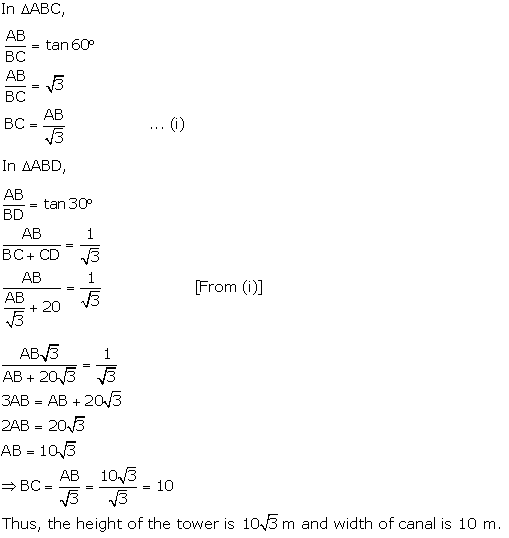
Solution 28

Solution 29
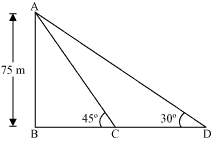
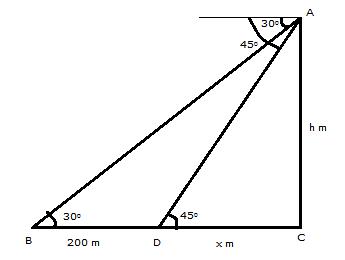
Let AB be the lighthouse and the two ships be at point C and D respectively.
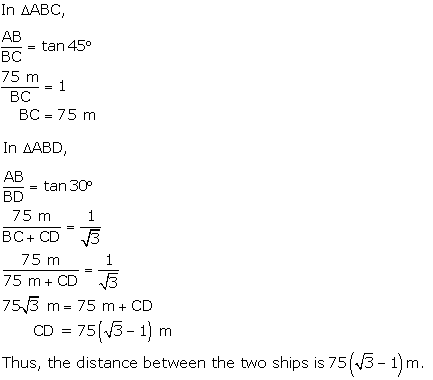
Solution 30
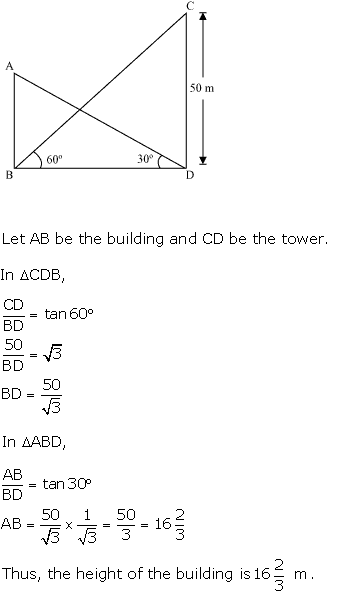
Solution 31
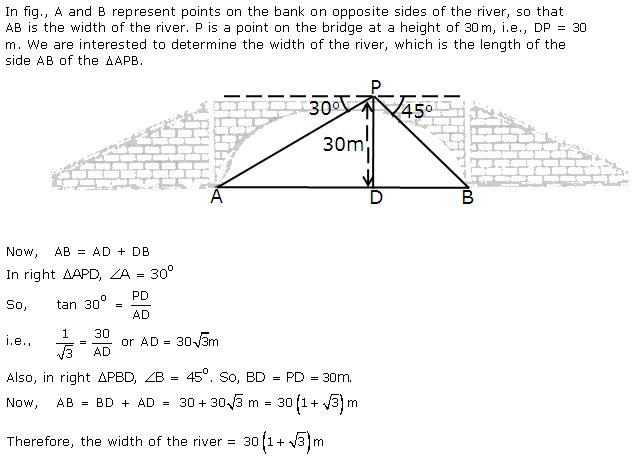
Solution 32

Solution 33
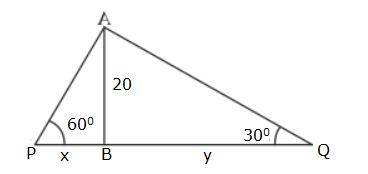
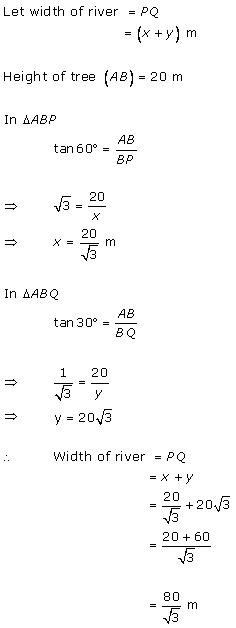
Solution 34
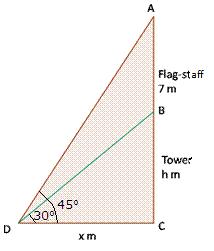
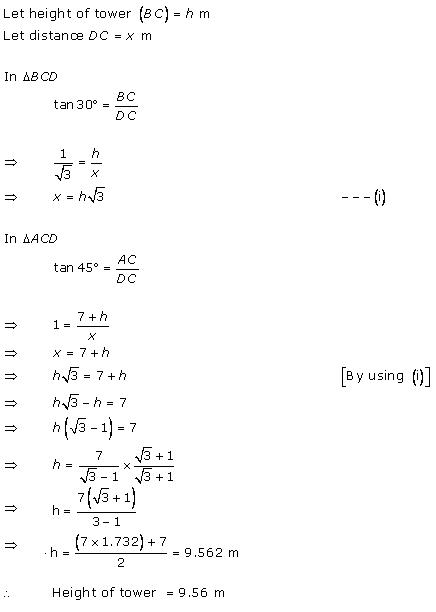
Solution 35
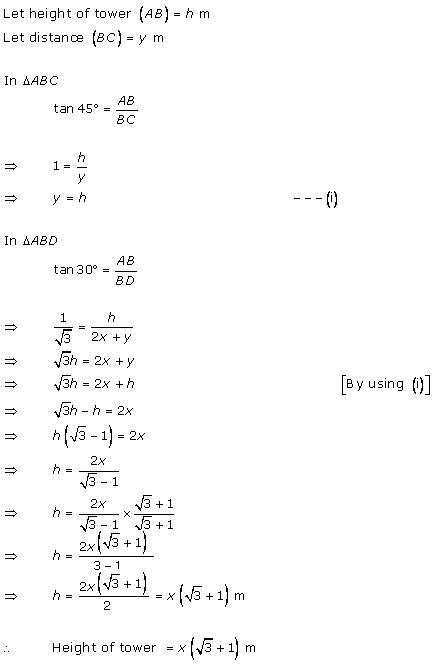
Solution 36

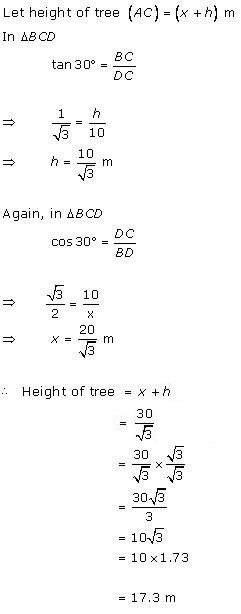
Solution 37
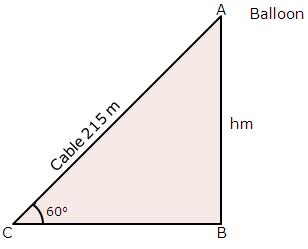
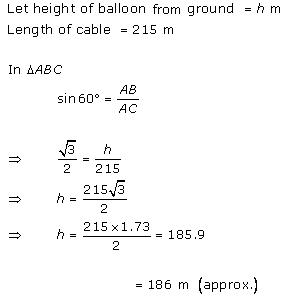
Solution 38
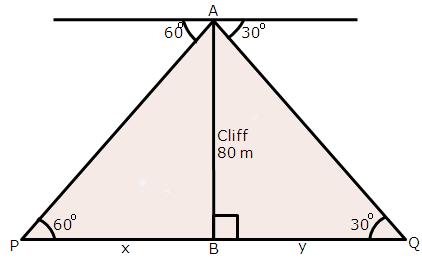

Solution 39
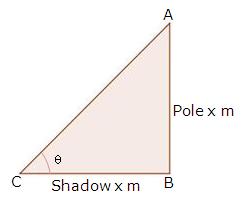

Solution 40
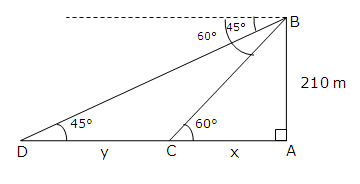

Solution 41
Let AC = h be the height of the chimney.
Height of the tower = DE = BC = 40 m
In ∆ABE,
![]()
![]()
∴AB = BE√3….(i)
In ∆CBE,
tan 30° = ![]()

Substituting BE in (i),
AB = 40√3 × √3
= 120 m
Height of the chimney = AB + BC = 120 + 40 = 160 m
Yes, the height of the chimney meets the pollution control norms.
Solution 42
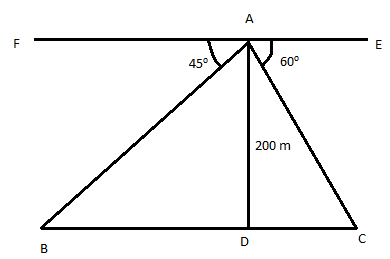
Let the ships be at B and C.
In D ABD,
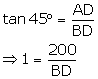
∴ BD = 200 m
In D ADC,

Distance between the two ships = BC = BD + DC
![]()
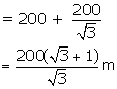
Solution 43
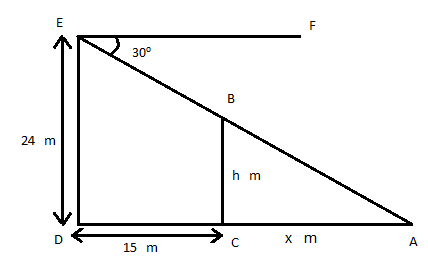
Here m∠CAB = m∠FEB = 30°.
Let BC = h m, AC = x m
In D ADE,
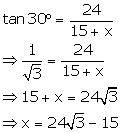
In D BAC,

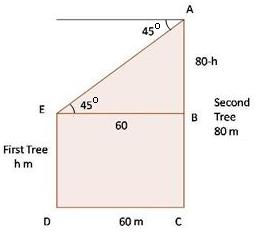
Height of the second pole is 15.34 m
Solution 44
Solution 45
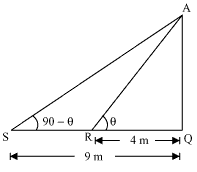
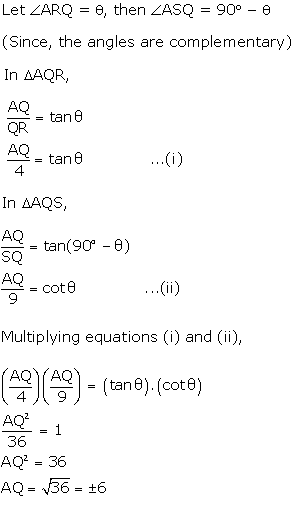
Let AQ be the tower and R, S respectively be the points which are 4m, 9m away from base of tower.
As the height can not be negative, the height of the tower is 6 m.
Solution 46
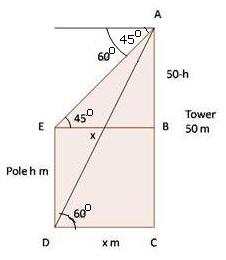

Solution 47

Solution 48
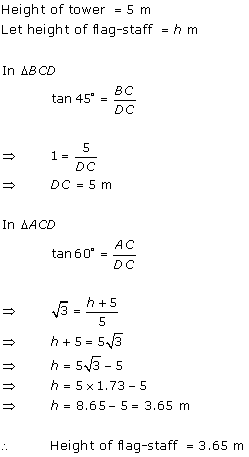
Solution 49
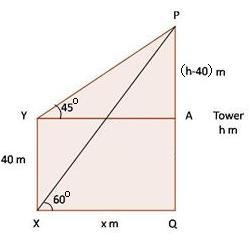

Solution 50

Solution 51

Solution 52
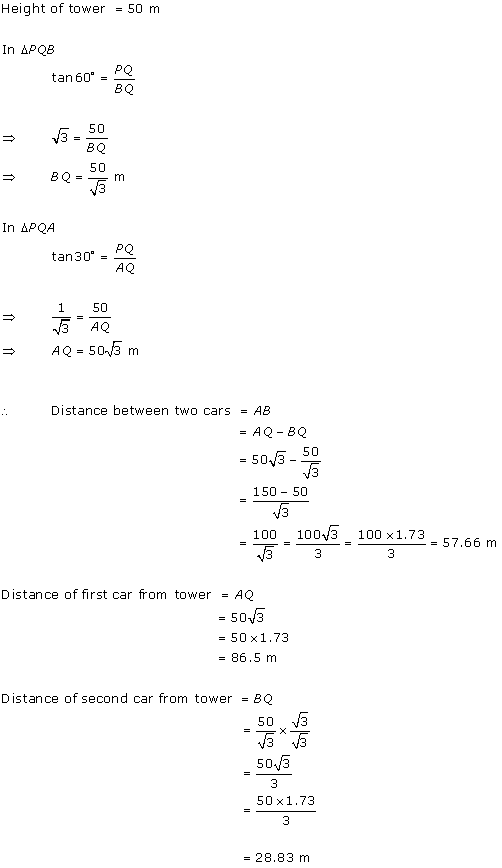
Solution 53
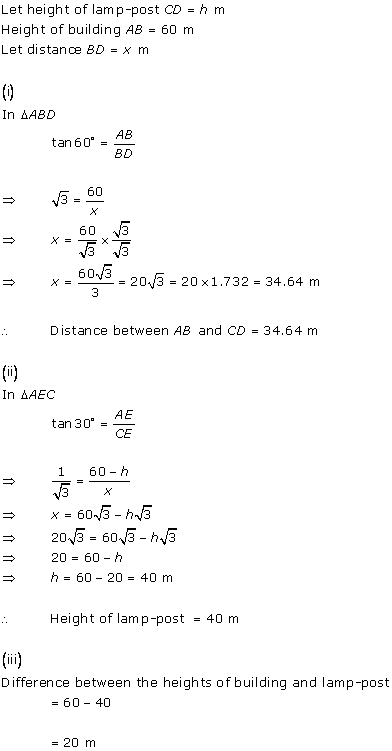
Solution 54

Solution 55
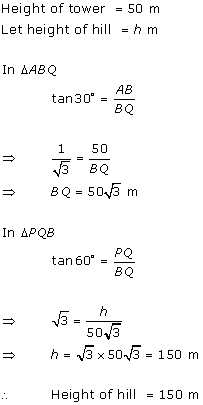
Solution 56 (i)
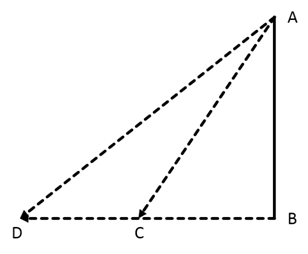
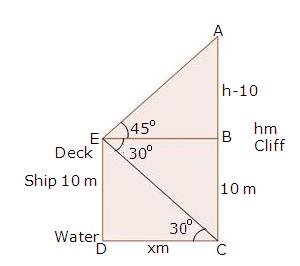
Let AB be the cliff, so AB=150m.
C and D are positions of the boat.
DC is the distance covered in 2 min.
∠ACB = 60o and ∠ADB = 45o
∠ABC = 90o
In ΔABC,
tan(∠ACB)= ![]()

In ΔABD,
tan(∠ADB)= ![]()
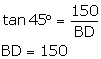
So, DC=BD - BC
=![]()
Now,

Solution 56 (ii)
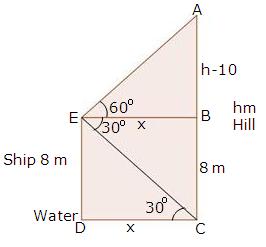
Let AB be the lighthouse and C be the position of man initially.
Suppose, a man changes his position from C to D.
As per the question, we obtain the following figure
Let speed of the boat be x metres per minute.
Therefore, CD = 2x
Using trigonometry, we have
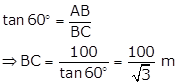
Also,
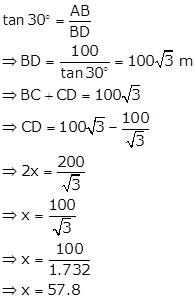
Hence, speed of the boat is 57.8 m.
Solution 57
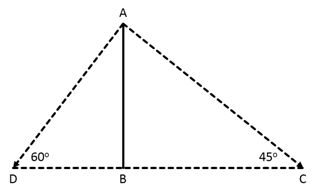
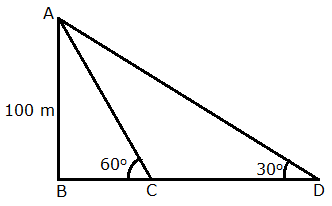
AB is the tower.
DC is the distance between cars.
AB=120m
In ΔABC,
tan(∠ACB) = ![]()
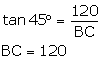
In ΔABD,
tan(∠ADB) = ![]()
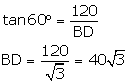
So, DC=BD+BC
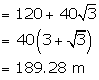
Solution 58
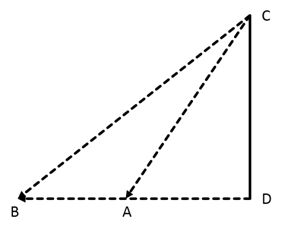
Let CD be the tower.
So CD =15m
AB is the distance between the points.
∠CAD = 60o and ∠CBD = 45o
∠ADC = 90o
In ΔADC,
tan(∠CAD)= ![]()
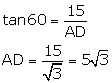
In ΔCBD,
tan(∠CBD)= ![]()
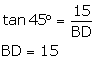
So AB=BD - AD
Solution 59
Now, in triangle APB,
sin 60o = AB/ BP
√3/2 = h/ BP
This gives
h = 14.64 km
Solution 60

Solution 61

Solution 62

Solution 63
Solution 64
Solution 65
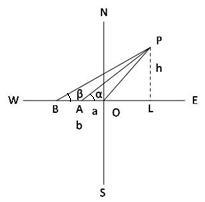

Solution 66
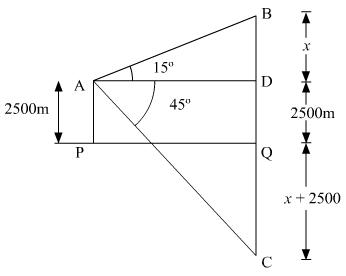

Solution 67
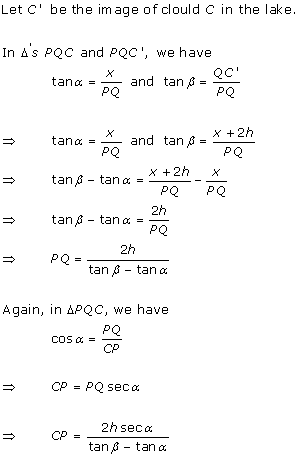
Solution 68
Solution 69
Solution 70

Solution 71

Solution 72
Solution 73

Solution 74
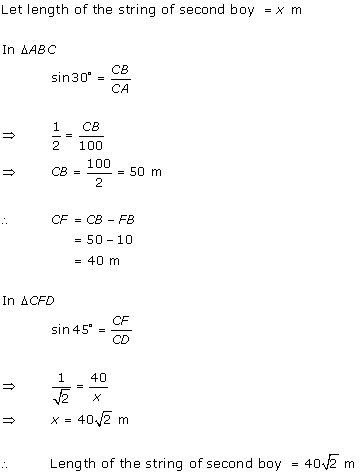
Solution 75

Solution 76


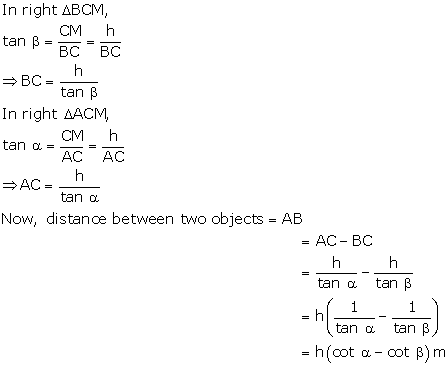
Solution 77
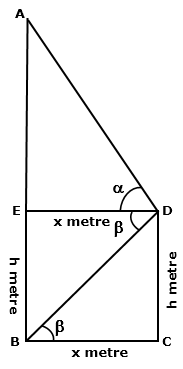

Solution 78
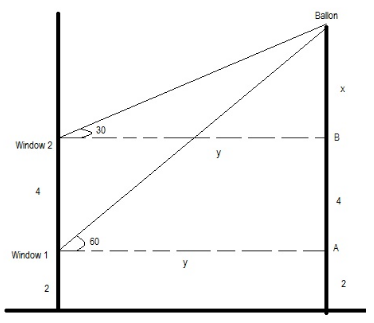
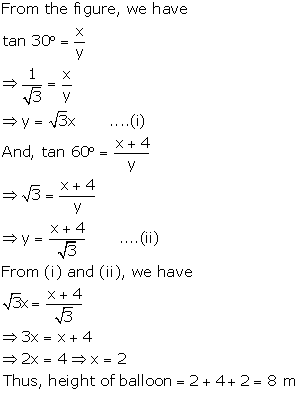
Some Applications of Trigonometry Exercise 12.41
Solution 1


Solution 2
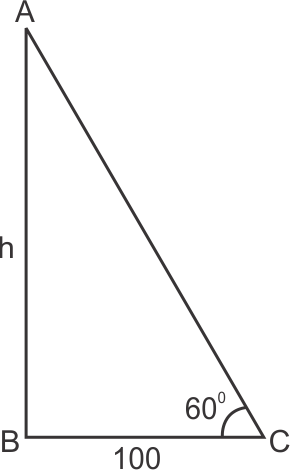

Solution 3
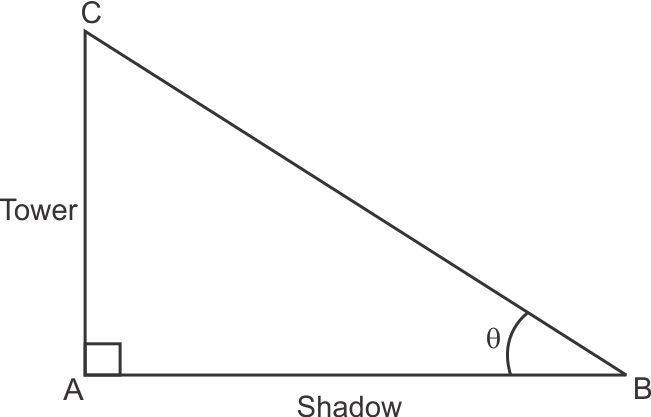

Solution 4
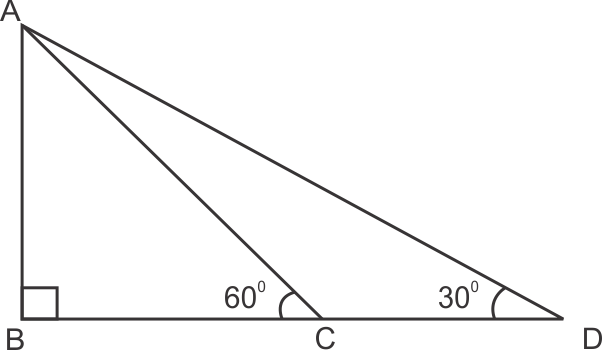

Solution 5


Solution 6
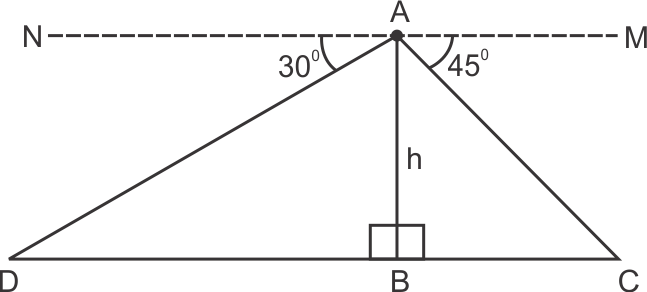

Solution 7
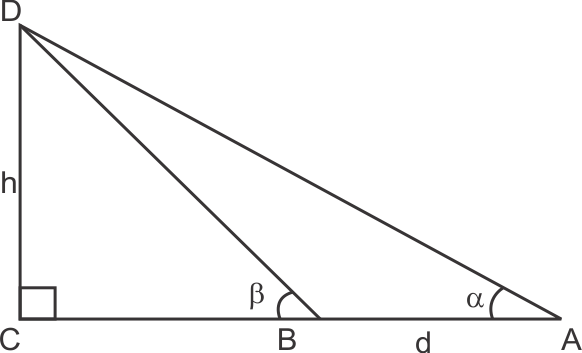

Solution 8
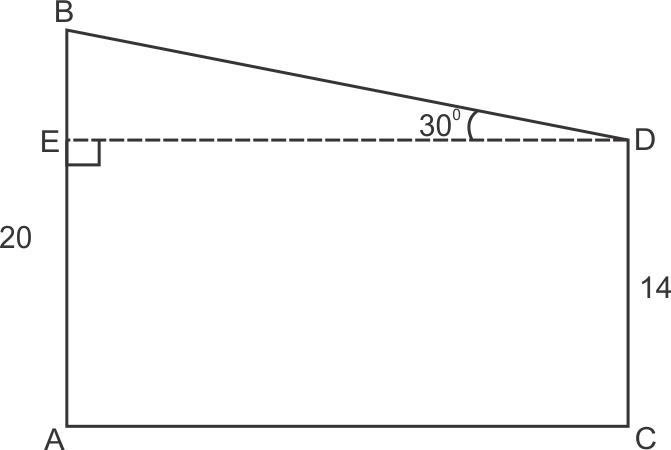
Wire BD
ED || AC
So, EA = DC and ED = AC
EA = 14
AB = EA + EB
20 = 14 + EB
EB = 6

So, the correct option is (a).
Solution 9
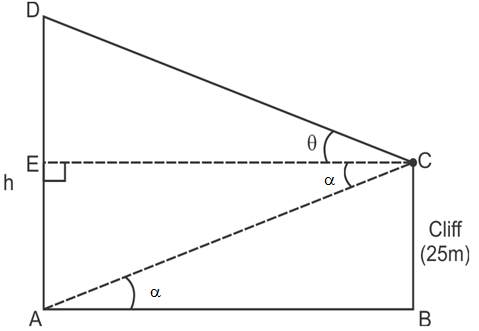

Solution 10
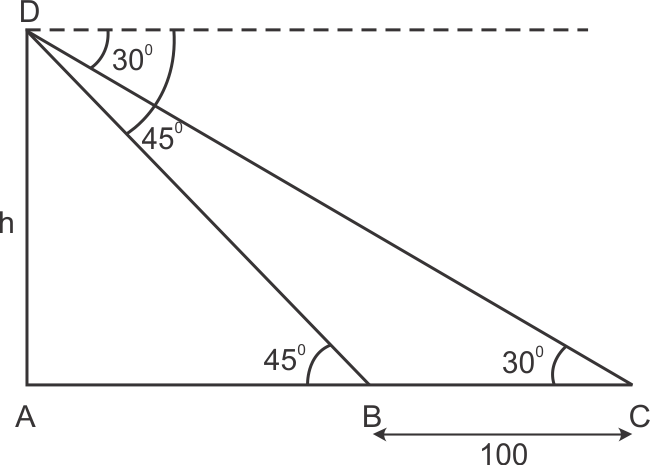

Some Applications of Trigonometry Exercise 12.42
Solution 11
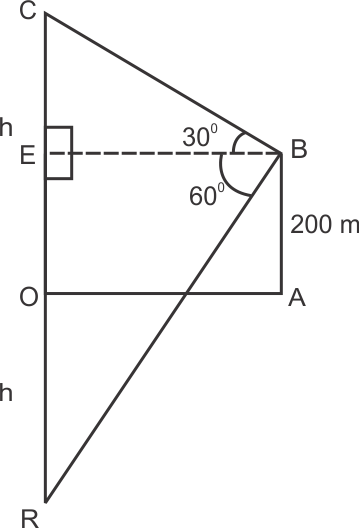

Solution 12
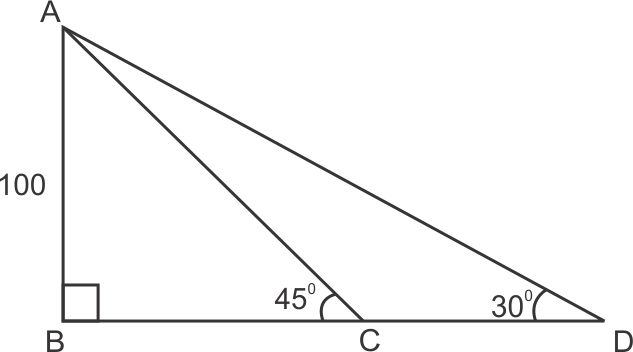

Solution 13
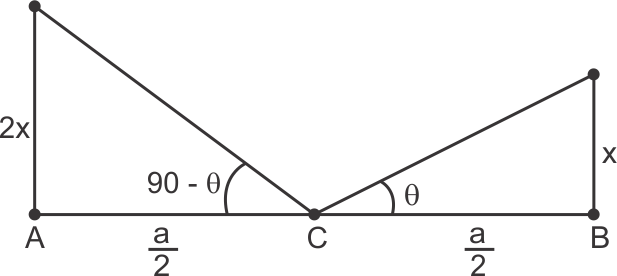
If height of one person is x then height of another one is 2x. Also If angle of elevation of one is θ then for another it is 90 - θ.
AB = a
C is mid point.

So, the correct option is (d).
Solution 14

Solution 15

Solution 16

Solution 17
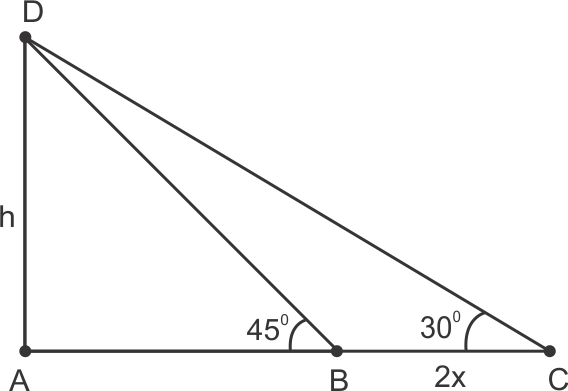

Solution 18

If height of one pole is x then height of the other one is 2x. Also If the angle of elevation of one is θ then for the other it is
90 - θ.
AB = a
C is mid point.

So, the correct option is (b).
Solution 19
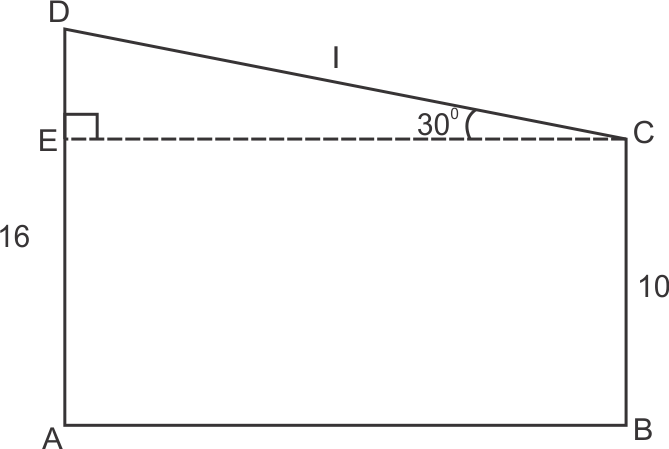
EC || AB
Hence
EA = CB = 10
AD = AE + ED
ED = AD - AE
= 16 - 10 = 6

So, the correct option is (c).
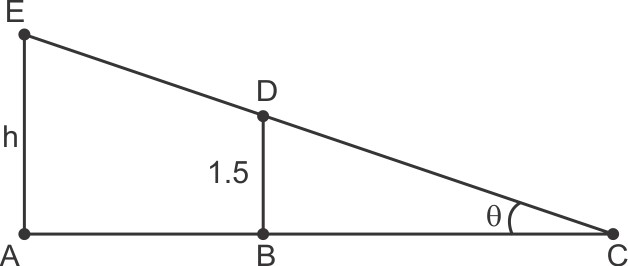
Solution 20

Some Applications of Trigonometry Exercise 12.43
Solution 21
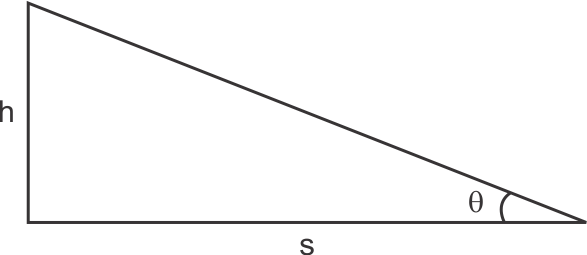

Solution 22
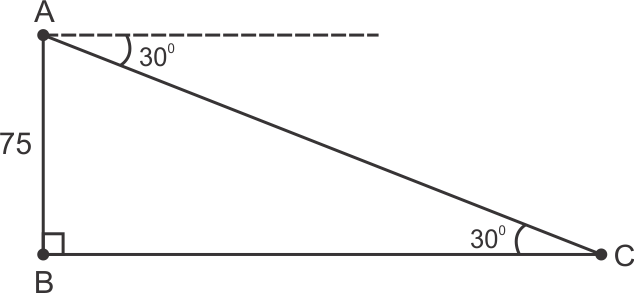
From the figure, it is cleared that we have to find the length of BC.

Solution 23
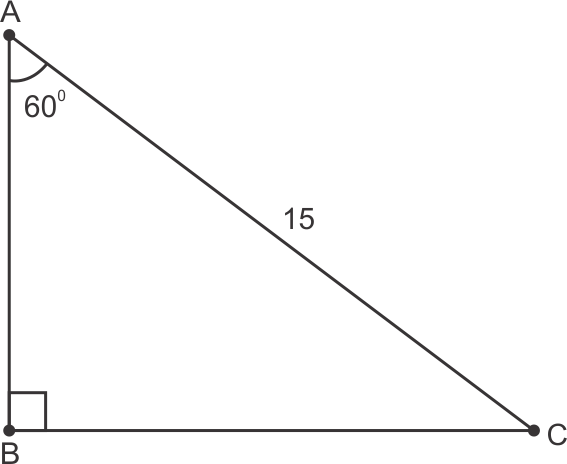

Solution 24


Solution 25


Solution 26
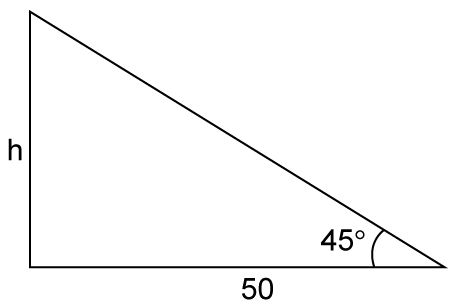

Solution 27