Class 10 SELINA Solutions Maths Chapter 23 - Graphical Representation (Histograms and Ogives)
Graphical Representation (Histograms and Ogives) Exercise Ex. 23
Solution 1
(i)
|
Class Interval |
Frequency |
|
0-10 |
12 |
|
10-20 |
20 |
|
20-30 |
26 |
|
30-40 |
18 |
|
40-50 |
10 |
|
50-60 |
06 |
Steps of construction:
(a) Taking suitable scales, mark class intervals on x-axis and frequency on y-axis.
(b)Construct rectangles with class intervals as bases and corresponding frequencies as heights.
(ii)
|
Class Interval |
Frequency |
|
10-16 |
15 |
|
16-22 |
23 |
|
22-28 |
30 |
|
28-34 |
20 |
|
34-40 |
16 |
Steps of construction:
(a) Taking suitable scales, mark class intervals on x-axis and frequency on y-axis.
(b) Construct rectangles with class intervals as bases and corresponding frequencies as heights.
(iii)
|
Class Interval (Inclusive form) |
Class Interval (Exclusive Form) |
Frequency |
|
30-39 |
29.5-39.5 |
24 |
|
40-49 |
39.5-49.5 |
16 |
|
50-59 |
49.5-59.5 |
09 |
|
60-69 |
59.5-69.5 |
15 |
|
70-79 |
69.5-79.5 |
20 |
Steps of construction:
(a) Convert the data into exclusive form.
(b) Taking suitable scales, mark class intervals on x-axis and frequency on y-axis.
(c) Construct rectangles with class intervals as bases and corresponding frequencies as heights.
(iv)
Since the difference between each class marks is 8, therefore, subtract 8/2 = 4 from each class mark to get the lower limit of the corresponding class interval and add 5 to each class mark to get the upper limit.
|
Class Marks |
Class Intervals |
Frequency |
|
16 |
12-20 |
08 |
|
24 |
20-28 |
12 |
|
32 |
28-36 |
15 |
|
40 |
36-44 |
18 |
|
48 |
44-52 |
25 |
|
56 |
52-60 |
19 |
|
64 |
60-68 |
10 |
Steps of construction:
(a) Convert the class marks into class intervals.
(b) Taking suitable scales, mark class intervals on x-axis and frequency on y-axis.
(c) Construct rectangles with class intervals as bases and corresponding frequencies as heights.
Solution 2
(i)
The cumulative frequency table for the given distribution is as follows:
|
Class Interval |
Frequency |
Cumulative frequency |
|
10-15 |
10 |
10 |
|
15-20 |
15 |
25 |
|
20-25 |
17 |
42 |
|
25-30 |
12 |
54 |
|
30-35 |
10 |
64 |
|
35-40 |
8 |
72 |
Taking upper limits along X-axis and corresponding cumulative frequencies along Y-axis, mark the points (15,10), (20,25), (25,42), (30,54), (35, 64) and (40, 72).
Join the points marked by a free hand curve.
Solution 3
(i)
|
Marks Obtained |
No. of students (c.f.) |
|
less than 10 |
8 |
|
less than 20 |
25 |
|
less than 30 |
38 |
|
less than 40 |
50 |
|
less than 50 |
67 |

Steps Of construction:
(a) Plot the points (10,8), (20, 25), (30, 38), (40, 50) and (50, 67) on the graph.
(b) Join them with free hand to obtain an ogive.
(ii)
|
Age in years (less than) |
Cumulative Frequency |
|
10 |
0 |
|
20 |
17 |
|
30 |
32 |
|
40 |
37 |
|
50 |
53 |
|
60 |
58 |
|
70 |
65 |
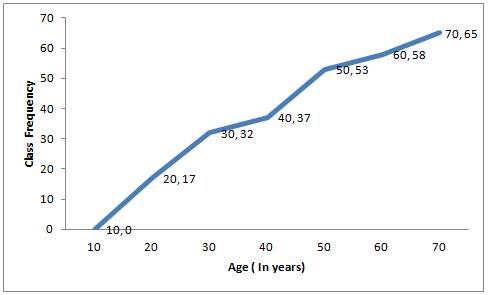
Steps Of construction:
(a) Plot the points (10, 0), (20, 17), (30, 32), (40, 37), (50, 53), (60, 58) and (70, 65) on the graph.
(b) Join them with free hand to obtain an ogive.
Solution 4
(i)
For constructing histogram, we convert the classes in continuous form.
|
Class Interval |
Frequency |
|
20.5-30.5 |
4 |
|
30.5-40.5 |
9 |
|
40.5-50.5 |
9 |
|
50.5-60.5 |
7 |
|
60.5-70.5 |
8 |
|
70.5-80.5 |
3 |
(ii)
For constructing an ogive, the table is as follows:
|
Class Interval |
Frequency |
Cumulative frequency |
|
20.5-30.5 |
4 |
4 |
|
30.5-40.5 |
9 |
13 |
|
40.5-50.5 |
9 |
22 |
|
50.5-60.5 |
7 |
29 |
|
60.5-70.5 |
8 |
37 |
|
70.5-80.5 |
3 |
40 |
Solution 5
(a)
|
Class Interval |
Frequency |
c.f. |
|
8-12 |
9 |
9 |
|
12-16 |
16 |
25 |
|
16-20 |
22 |
47 |
|
20-24 |
18 |
65 |
|
24-28 |
12 |
77 |
|
28-32 |
4 |
81 |
(b) Now plot the points (12, 9), (16, 25), (20, 47), (24, 65), (28, 77), (32, 81) and join them to obtain an ogive.
Solution 6
(a)
Difference in consecutive class marks = 17.5 - 12.5 = 5
![]() first class interval will be 10-15 and so on.
first class interval will be 10-15 and so on.
|
Class Mark |
Class Interval |
Frequency |
c.f. |
|
12.5 |
10-15 |
12 |
12 |
|
17.5 |
15-20 |
17 |
29 |
|
22.5 |
20-25 |
22 |
51 |
|
27.5 |
25-30 |
27 |
78 |
|
32.5 |
30-35 |
30 |
108 |
|
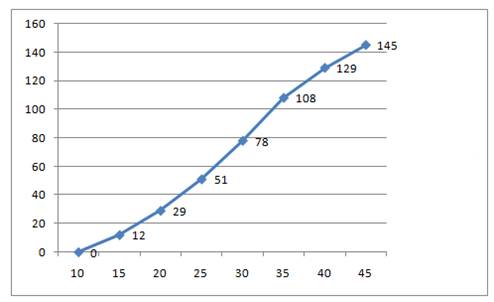
37.5 |
35-40 |
21 |
129 |
|
42.5 |
40-45 |
16 |
145 |
(b)
Now plot the points (15,12), (20,29), (25,51), (30,78), (35,108), (40,129), (45,145) and join them to obtain an ogive.
Solution 7
(i)
|
Wages |
No. Of workers |
c.f. |
|
6500-7000 |
10 |
10 |
|
7000-7500 |
18 |
28 |
|
7500-8000 |
22 |
50 |
|
8000-8500 |
25 |
75 |
|
8500-9000 |
17 |
92 |
|
9000-9500 |
10 |
102 |
|
9500-10000 |
8 |
110 |
Total = 110
(ii)
Now plot the points (7000,10), (7500,28), (8000,50), (8500,75), (9000,92), (9500,102) and (10000,110) and join them to obtain an ogive.
Solution 8
(i)
|
Height (in cm) |
No. Of workers |
c.f. |
|
150-155 |
6 |
6 |
|
155-160 |
12 |
18 |
|
160-165 |
18 |
36 |
|
165-170 |
20 |
56 |
|
170-175 |
13 |
69 |
|
175-180 |
8 |
77 |
|
180-185 |
6 |
83 |
(ii)
We plot the points (155, 6), (160, 18), (165, 36), (170, 56), (175, 69), (180, 77) and (185, 83) on the graph and join them in free hand to obtain an ogive.
Solution 9
(i)
|
Marks (less than) |
Cumulative frequency |
Frequency |
|
0-10 |
7 |
7 |
|
10-20 |
28 |
28-7=21 |
|
20-30 |
54 |
54-28=26 |
|
30-40 |
71 |
71-54=17 |
|
40-50 |
84 |
84-71=13 |
|
50-60 |
105 |
105-84=21 |
|
60-70 |
147 |
147-105=42 |
|
70-80 |
180 |
180-147=33 |
|
80-90 |
196 |
196-180=16 |
|
90-100 |
200 |
200-196=4 |
|
Total |
|
200 |
(ii)
|
Marks (more than) |
Cumulative frequency |
Frequency |
|
0-10 |
100 |
13 |
|
10-20 |
87 |
22 |
|
20-30 |
65 |
10 |
|
30-40 |
55 |
13 |
|
40-50 |
42 |
6 |
|
50-60 |
36 |
5 |
|
60-70 |
31 |
10 |
|
70-80 |
21 |
3 |
|
80-90 |
18 |
11 |
|
90-100 |
7 |
7 |
|
Total |
|
100 |

