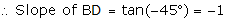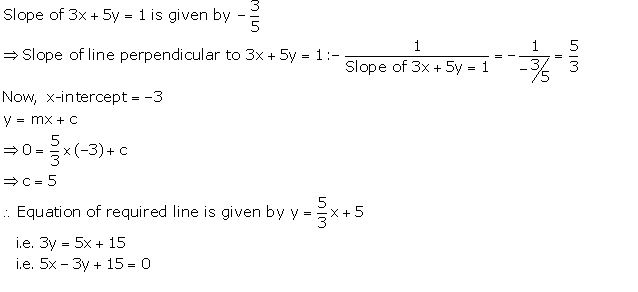Class 10 SELINA Solutions Maths Chapter 14 - Equation of a Line
The ICSE curriculum for Mathematics helps students develop their mathematical aptitude, logical reasoning, critical thinking, and analytical and problem-solving skills. Thus, it aims at building a strong foundation in core mathematical concepts.
The ICSE Class 10 Math syllabus covers a wide range of topics such as Algebra, Geometry, Trigonometry, Linear Equations, and Statistics. One of the important chapters is Chapter 14- Equation of a Line. It focuses on understanding the relationship between lines and their equations. Students have to learn various forms, including slope-intercept form, point-slope form, general form, etc., and express the Equation of a line in different ways. The knowledge they gain through this chapter applies to various fields such as physics, engineering, and geometry.
Selina Solutions offers comprehensive and detailed solutions for the problems presented in the Equation of a Line chapter. It provides clear and step-by-step explanations by breaking down each problem in an easily understandable form. The solutions provide answers for all the textbook questions, which allow students to comprehend, reinforce their understanding and retain the knowledge. Every answer thoroughly aligns with the ICSE curriculum and exam pattern, which helps in effective preparation and better exam performance.
Selina Solutions also provide ample graphical representations to enhance understanding of linear equations. It illustrates how to plot lines and interpret them on a coordinate plane which enables students to visualise the relationship between equations and their representations.
You can find Selina Solutions for Physics, Chemistry, Maths, and Biology for class 9 and class 10 at TopperLearning. As an online education platform, we provide comprehensive learning resources prepared by expert educators. With a wide range of study materials such as video lessons, practice tests, revision notes, textbook solutions, sample papers, etc, we offer a personalised learning approach. Take a free demo to understand our study session, or join to become a topper!
Equation of a Line Exercise Ex. 14(A)
Solution 1
The given line is x - 2y + 5 = 0.
(i) Substituting x = 1 and y = 3 in the given equation, we have:
L.H.S. = 1 - 2 
3 + 5 = 1 - 6 + 5 = 6 - 6 = 0 = R.H.S.
Thus, the point (1, 3) lies on the given line.
(ii) Substituting x = 0 and y = 5 in the given equation, we have:
L.H.S. = 0 - 2 5 + 5 = -10 + 5 = -5 
R.H.S.
Thus, the point (0, 5) does not lie on the given line.
(iii) Substituting x = -5 and y = 0 in the given equation, we have:
L.H.S. = -5 - 2 0 + 5 = -5 - 0 + 5 = 5 - 5 = 0 = R.H.S.
Thus, the point (-5, 0) lies on the given line.
(iv) Substituting x = 5 and y = 5 in the given equation, we have:
L.H.S. = 5 - 2 5 + 5 = 5 - 10 + 5 = 10 - 10 = 0 = R.H.S.
Thus, the point (5, 5) lies on the given line.
(v) Substituting x = 2 and y = -1.5 in the given equation, we have:
L.H.S. = 2 - 2 (-1.5) + 5 = 2 + 3 + 5 = 10 R.H.S.
Thus, the point (2, -1.5) does not lie on the given line.
(vi) Substituting x = -2 and y = -1.5 in the given equation, we have:
L.H.S. = -2 - 2 (-1.5) + 5 = -2 + 3 + 5 = 6 R.H.S.
Thus, the point (-2, -1.5) does not lie on the given line.
Solution 2
(i)
The given line is 
Substituting x = 2 and y = 3 in the given equation,
Thus, the given statement is false.
(ii)
The given line is
Substituting x = 4 and y = -6 in the given equation,
Thus, the given statement is true.
(iii) L.H.S = y - 7 = 7 - 7 = 0 = R.H.S.
Thus, the point (8, 7) lies on the line y - 7 = 0.
The given statement is true.
(iv) L.H.S. = x + 3 = -3 + 3 = 0 = R.H.S
Thus, the point (-3, 0) lies on the line x + 3 = 0.
The given statement is true.
(v) The point (2, a) lies on the line 2x - y = 3.
2(2) - a = 3
4 - a = 3
a = 4 - 3 = 1
Thus, the given statement is false.
Solution 3
Given, the line
given by the equation 
passes through the
point (k, 6).
Substituting x = k and y = 6 in the given equation, we have:
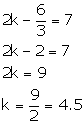
Solution 4
The given equation of the line is 9x + 4y = 3.
Put x = 3 and y = -k, we have:
9(3) + 4(-k) = 3
27 - 4k = 3
4k = 27 - 3 = 24
k = 6
Solution 5
The equation of the given line is 
Putting x = m, y = 2m - 1, we have:
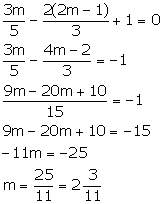
Solution 6
The given line will bisect the join of A (5, -2) and B (-1, 2), if the co-ordinates of the mid-point of AB satisfy the equation of the line.
The co-ordinates of the mid-point of AB are
Substituting x = 2 and y = 0 in the given equation, we have:
L.H.S. = 3x - 5y = 3(2) - 5(0) = 6 - 0 = 6 = R.H.S.
Hence, the line 3x - 5y = 6 bisects the join of (5, -2) and (-1, 2).
Solution 7
(i) The given line bisects the join of A (a, 3) and B (2, -5), so the co-ordinates of the mid-point of AB will satisfy the equation of the line.
The co-ordinates of the mid-point of AB are
Substituting x = 
and y = -1 in the
given equation, we have:
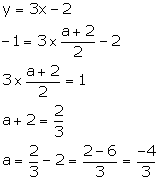
(ii) The given line bisects the join of A (8, -1) and B (0, k), so the co-ordinates of the mid-point of AB will satisfy the equation of the line.
The co-ordinates of the mid-point of AB are
Substituting x = 4
and y = 
in the given
equation, we have:
Solution 8
(i) Given, the point (-3, 2) lies on the line ax + 3y + 6 = 0.
Substituting x = -3 and y = 2 in the given equation, we have:
a(-3) + 3(2) + 6 = 0
-3a + 12 = 0
3a = 12
a = 4
(ii) Given, the line y = mx + 8 contains the point (-4, 4).
Substituting x = -4 and y = 4 in the given equation, we have:
4 = -4m + 8
4m = 4
m = 1
Solution 9
Given, the point P divides the join of (2, 1) and (-3, 6) in the ratio 2: 3.
Co-ordinates of the point P are
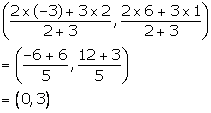
Substituting x = 0 and y = 3 in the given equation, we have:
L.H.S. = 0 - 5(3) + 15 = -15 + 15 = 0 = R.H.S.
Hence, the point P lies on the line x - 5y + 15 = 0.
Solution 10
Given, the line segment joining the points (5, -4) and (2, 2) is divided by the point Q in the ratio 1: 2.
Co-ordinates of the point Q are
Substituting x = 4 and y = -2 in the given equation, we have:
L.H.S. = x - 2y = 4
- 2(-2) = 4 + 4 = 8 
R.H.S.
Hence, the given line does not contain point Q.
Solution 11
Consider the given equations:
4x + 3y = 1 ....(1)
3x - y + 9 = 0 ....(2)
Multiplying (2) with 3, we have:
9x - 3y = -27 ....(3)
Adding (1) and (3), we get,
13x = -26
x = -2
From (2), y = 3x + 9 = -6 + 9 = 3
Thus, the point of intersection of the given lines (1) and (2) is (-2, 3).
The point (-2, 3) lies on the line (2k - 1)x - 2y = 4.
(2k - 1)(-2) - 2(3) = 4
-4k + 2 - 6 = 4
-4k = 8
k = -2
Solution 12
We know that two or more lines are said to be concurrent if they intersect at a single point.
We first find the point of intersection of the first two lines.
2x + 5y = 1 ....(1)
x - 3y = 6 ....(2)
Multiplying (2) by 2, we get
2x - 6y = 12 ....(3)
Subtracting (3) from (1), we get
11y = -11
y = -1
From (2), x = 6 + 3y = 6 - 3 = 3
So, the point of intersection of the first two lines is (3, -1).
If this point lie on the third line, i.e., x + 5y + 2 = 0, then the given lines will be concurrent.
Substituting x = 3 and y = -1 in x + 5y + 2 = 0, we have
L.H.S. = x + 5y + 2 = 3 + 5(-1) + 2 = 5 - 5 = 0 = R.H.S.
Thus, (3, -1) lies on the third line also.
Hence, the given lines are concurrent.
Equation of a Line Exercise Ex. 14(B)
Solution 1
(i) Slope = tan 0o = 0
(ii) Slope = tan 30o = 
(iii) Slope = tan 72o 30' = 3.1716 [From the trigonometrical table]
(iv) Slope = tan 46o = 1.0355 [From the trigonometrical table]
Solution 2
(i) Slope = tan 
= 0
= 0o
(ii) Slope = tan = 
= 60o
(iii) Slope = tan = 0.7646
= 37o
24'
(iv)
Slope = tan = 1.0875
= 47o
24'
Solution 3
We know:
Slope = 
(i) Slope = 
(ii) Slope = 
(iii) Slope = 
Solution 4
(i) Slope of AB = 
Slope of the line parallel to AB = Slope of AB = 1
(ii) Slope of AB = 
Slope of the line parallel to AB = Slope of AB = -4
Solution 5
(i) Slope of AB = 
Slope of the line
perpendicular to AB = 
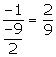
(ii) Slope of AB = 
Slope of the line
perpendicular to AB = 1
Solution 6
Slope of the line
passing through (0, 2) and (-3, -1) = 
Slope of the line
passing through (-1, 5) and (4, a) = 
Since, the lines are parallel.
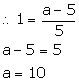
Solution 7
Slope of the line
passing through (-4, -2) and (2, -3) = 
Slope of the line
passing through (a, 5) and (2, -1) = 
Since, the lines are perpendicular.
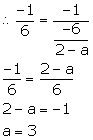
Solution 8
The given points are A (4, -2), B (-4, 4) and C (10, 6).
It can be seen that:
Hence, AB 
AC.
Thus, the given points are the vertices of a right-angled triangle.
Solution 9
The given points are A (4, 5), B (1, 2), C (4, 3) and D (7, 6).
Since, slope of AB = slope of CD
Therefore AB || CD
Since, slope of BC = slope of DA
Therefore, BC || DA
Hence, ABCD is a parallelogram
Solution 10
Let the given points be A (-2, 4), B (4, 8), C (10, 7) and D (11, -5).
Let P, Q, R and S be the mid-points of AB, BC, CD and DA respectively.
Co-ordinates of P are
Co-ordinates of Q are
Co-ordinates of R are
Co-ordinates of S are
Since, slope of PQ = Slope of RS, PQ || RS.
Since, slope of QR = Slope of SP, QR || SP.
Hence, PQRS is a parallelogram.
Solution 11
The points P, Q, R will be collinear if slope of PQ and QR is the same.
Since slope of PQ = slope of QR, the points P, Q, and R are collinear.
Solution 12
Let A = (x, 2) and B = (8, -11)
Slope of AB = 
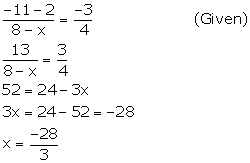
Solution 13
We know that the slope of any line parallel to x-axis is 0.
Therefore, slope of AB = 0
Since, ABC is an equilateral triangle, 
Slope of AC = tan 60o =
Slope of BC = −tan 60o = −
Solution 14
We know that the slope of any line parallel to x-axis is 0.
Therefore, slope of AB = 0
As CD || AB, slope of CD = Slope of AB = 0
As BC 
AB, slope of BC = 
As AD AB, slope of AD =
(i) The diagonal AC makes an angle of 45o with the positive direction of x axis.
(ii) The diagonal BD makes an angle of -45o with the positive direction of x axis.
Solution 15
Given, A (5, 4), B (-3, -2) and C (1, -8) are the vertices of a triangle ABC.
(i) Slope of AB = 
Slope of the altitude of AB =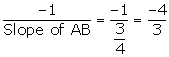 
(ii) Since, D is the mid-point of BC.
Co-ordinates of point D are
Slope of AD = 
(iii) Slope of AC = 
Slope of line parallel to AC = Slope of AC = 3
Solution 16
(i) Since, BC is perpendicular to AB,
Slope of AB =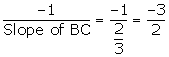 
(ii) Since, AD is parallel to BC,
Slope of AD = Slope of BC = 
Solution 17
(i) A = (-3, -2) and B = (1, 2)
Slope of AB = 
Inclination of line AB = 
= 45o
(ii) A = (0, 
) and B = (3, 0)
Slope of AB = 
Inclination of line AB = = 30o
(iii) A = (-1, 2
) and B = (-2, )
Slope of AB = 
Inclination of line AB = = 60o
Solution 18
Given, points A (-3, 2), B (2, -1) and C (a, 4) are collinear.
Slope of AB = Slope of BC
Solution 19
Given, points A (K, 3), B (2, -4) and C (-K + 1, -2) are collinear.
Slope of AB = Slope of BC
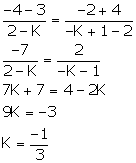
Solution 20
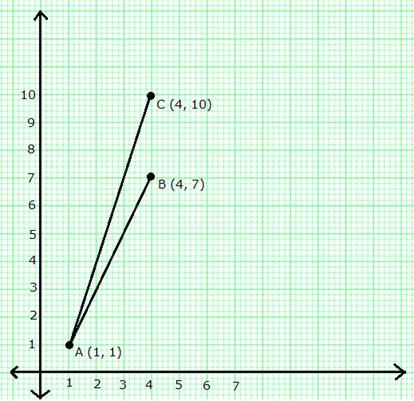
From the graph, clearly, AC has steeper slope.
Slope of AB = 
Slope of AC = 
The line with greater slope is steeper. Hence, AC has steeper slope.
Solution 21
Since, PQ || RS,
Slope of PQ = Slope of RS
(i) Slope of PQ = 
Slope of RS = 
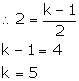
(ii) Slope of PQ = 
Slope of RS = 
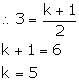
(iii) Slope of PQ = 
Slope of RS = 
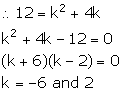
Equation of a Line Exercise Ex. 14(C)
Solution 1
Given, y-intercept = c = 2 and slope = m = 3.
Substituting the values of c and m in the equation y = mx + c, we get,
y = 3x + 2, which is the required equation.
Solution 2
Given, y-intercept = c = -1 and inclination = 45o.
Slope = m = tan 45o = 1
Substituting the values of c and m in the equation y = mx + c, we get,
y = x - 1, which is the required equation.
Solution 3
Given, slope = 
The line passes through (-3, 4) = (x1, y1)
Substituting the values in y - y1 = m(x - x1), we get,
y - 4 = (x + 3)
3y - 12 = -4x - 12
4x + 3y = 0, which is the required equation.
Solution 4
Slope of the line =
tan 60o = 
The line passes through the point (5, 4) = (x1, y1)
Substituting the values in y - y1 = m(x - x1), we get,
y - 4 = (x - 5)
y - 4 = x - 5
y =x + 4 - 5, which is the
required equation.
Solution 5
(i) Let (0, 1) = (x1, y1) and (1, 2) = (x2, y2)
The required equation of the line is given by:
y - y1 = m(x - x1)
y - 1 = 1(x - 0)
y - 1 = x
y = x + 1
(ii) Let (-1, -4) = (x1, y1) and (3, 0) = (x2, y2)
The required equation of the line is given by:
y - y1 = m(x - x1)
y + 4 = 1(x + 1)
y + 4 = x + 1
y = x - 3
Solution 6
Given, co-ordinates of two points P and Q are (2, 6) and (-3, 5) respectively.
(i) Gradient of PQ
= 
(ii) The equation of the line PQ is given by:
y - y1 = m(x - x1)
y - 6 = 
(x - 2)
5y - 30 = x - 2
5y = x + 28
(iii) Let the line PQ intersects the x-axis at point A (x, 0).
Putting y = 0 in the equation of the line PQ, we get,
0 = x + 28
x = -28
Thus, the co-ordinates of the point where PQ intersects the x-axis are A (-28, 0).
Solution 7
(i) Given, co-ordinates of two points A and B are (−3, 4) and (2, −1).
Slope =
The equation of the line AB is given by:
y − y1 = m(x − x1)
y − 4 = −1(x + 3)
y − 4 = −x − 3
x + y = 1
(ii) Let the line AB intersects the y-axis at point (0, y).
Putting x = 0 in the equation of the line, we get,
0 + y = 1
y = 1
Thus, the co-ordinates of the point where the line AB intersects the y-axis are (0, 1).
Solution 8
Slope of line AB = tan 45o = 1
The line AB passes through P (3, 4). So, the equation of the line AB is given by:
y - y1 = m(x - x1)
y - 4 = 1(x - 3)
y - 4 = x - 3
y = x + 1
Slope of line CD =
tan 60o = 
The line CD passes through P (3, 4). So, the equation of the line CD is given by:
y - y1 = m(x - x1)
y - 4 = (x - 3)
y - 4 = x - 3
y = x + 4 - 3
Solution 9
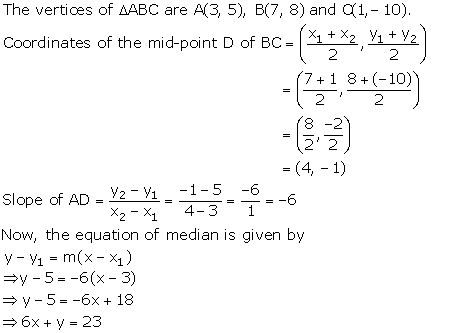
Solution 10
Since, ABCD is a parallelogram,
B = 180o
- 60o = 120o
Slope
of BC = tan 120o = tan (90o + 30o) = cot30o
= 
Equation of the line BC is given by:
y - y1 = m(x - x1)
y - 5
= (x - 7)
y - 5
= x - 7
y = x + 5 - 7
Since, CD || AB and AB || x-axis, slope of CD = Slope of AB = 0
Equation of the line CD is given by:
y - y1 = m(x - x1)
y - 5 = 0(x - 7)
y = 5
Solution 11
The given equations are:
x + 2y = 7 ....(1)
x - y = 4 ....(2)
Subtracting (2) from (1), we get,
3y = 3
y = 1
From (2), x = 4 + y = 4 + 1 = 5
The required line passes through (0, 0) and (5, 1).
Required equation of the line is given by:
Solution 12
Given, the co-ordinates of vertices A, B and C of a triangle ABC are (4, 7), (-2, 3) and (0, 1) respectively.
Let AD be the median through vertex A.
Co-ordinates of the point D are
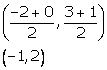
Slope of AD = 
The equation of the median AD is given by:
y - y1 = m(x - x1)
y - 7 = 1(x - 4)
y - 7 = x - 4
x - y + 3 = 0
The slope of the line which is parallel to line AC will be equal to the slope of AC.
Slope of AC = 
The equation of the line which is parallel to AC and passes through B is given by:
y - 3 = 
(x + 2)
2y - 6 = 3x + 6
2y = 3x + 12
3x - 2y + 12 = 0
Solution 13
Slope of BC = 
Slope of line
perpendicular to BC = 
The equation of the line through A and perpendicular to BC is given by:
y - y1 = m(x - x1)
y - 3 = 1(x - 0)
y - 3 = x
y = x + 3
Solution 14
Let A = (1, 4), B = (2, 3), and C = (-1, 2).
Slope of AB = 
Slope of line perpendicular to AB = 
The equation of the perpendicular drawn through C onto AB is given by:
y - y1 = m(x - x1)
y - 2 = 1(x + 1)
y - 2 = x + 1
y = x + 3
Solution 15
(i) When x-intercept = 5, corresponding point on x-axis is (5, 0)
When y-intercept = 3, corresponding point on y-axis is (0, 3).
Let (x1, y1) = (5, 0) and (x2, y2) = (0, 3)
Slope = 
The required equation is:
y - y1 = m(x - x1)
y - 0 = 
(x - 5)
5y = -3x + 15
3x + 5y = 15
(ii) When x-intercept = -4, corresponding point on x-axis is (-4, 0)
When y-intercept = 6, corresponding point on y-axis is (0, 6).
Let (x1, y1) = (-4, 0) and (x2, y2) = (0, 6)
Slope = 
The required equation is:
y - y1 = m(x - x1)
y - 0 = 
(x + 4)
2y = 3x + 12
(iii) When x-intercept = -8, corresponding point on x-axis is (-8, 0)
When y-intercept = -4, corresponding point on y-axis is (0, -4).
Let (x1, y1) = (-8, 0) and (x2, y2) = (0, -4)
Slope = 
The required equation is:
y - y1 = m(x - x1)
y - 0 = 
(x + 8)
2y = -x - 8
x + 2y + 8 = 0
Solution 16
Since, x-intercept is 6, so the corresponding point on x-axis is (6, 0).
Slope = m = 
Required equation of the line is given by:
y - y1 = m(x - x1)
y - 0 = (x - 6)
6y = -5x + 30
5x + 6y = 30
Solution 17
Since, x-intercept is 5, so the corresponding point on x-axis is (5, 0).
The line also passes through (-3, 2).
Slope of the line =
Required equation of the line is given by:
y - y1 = m(x - x1)
y - 0 = 
(x - 5)
4y = -x + 5
x + 4y = 5
Solution 18
Since, y-intercept = 5, so the corresponding point on y-axis is (0, 5).
The line passes through (1, 3).
Slope of the line =
Required equation of the line is given by:
y - y1 = m(x - x1)
y - 5 = -2(x - 0)
y - 5 = -2x
2x + y = 5
Solution 19
Let AB and CD be two equally inclined lines as shown in the figure.
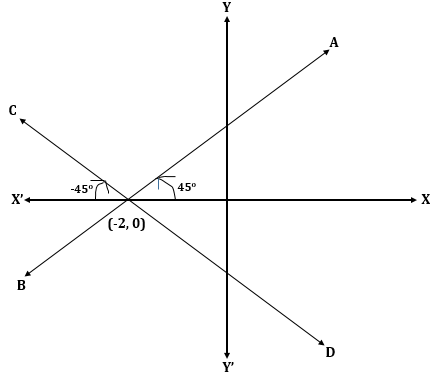
For line AB:
Slope = m = tan 45o = 1
(x1, y1) = (-2, 0)
Equation of the line AB is:
y - y1 = m(x - x1)
y - 0 = 1(x + 2)
y = x + 2
For line CD:
Slope = m = tan (-45o) = -1
(x1, y1) = (-2, 0)
Equation of the line CD is:
y - y1 = m(x - x1)
y - 0 = -1(x + 2)
y = -x - 2
x + y + 2 = 0
Solution 20
(i)
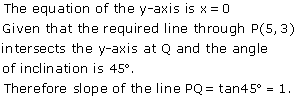
(ii)
(iii)
Solution 21
Given, P divides the line segment joining A (4, -8) and B (12, 0) in the ratio 3: 1.
Co-ordinates of point P are
Slope = m =
(Given)
Thus, the required equation of the line is
y - y1 = m(x - x1)
y + 2 = (x - 10)
5y + 10 = -2x + 20
2x + 5y = 10
Solution 22
(i) Co-ordinates of the centroid of triangle ABC are
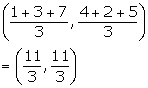
(ii) Slope of AB = 
Slope of the line parallel to AB = Slope of AB = -1
Thus, the required equation of the line is
y - y1 = m(x - x1)
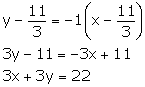
Solution 23
Given, AP: CP = 2: 3
Co-ordinates of P
are
Slope of BP = 
Required equation of the line passing through points B and P is
y - y1 = m(x - x1)
y - 1 = 0(x - 3)
y = 1
Equation of a Line Exercise Ex. 14(D)
Solution 1
(i) y = 4
Comparing this equation with y = mx + c, we have:
Slope = m = 0
y-intercept = c = 4
(ii) ax - by = 0 
by = ax y = 
Comparing this equation with y = mx + c, we have:
Slope = m = 
y-intercept = c = 0
(iii) 3x - 4y = 5 
Comparing this equation with y = mx + c, we have:
Slope = m = 
y-intercept = c = 
Solution 2
Given equation of a line is x - y = 4.
y = x - 4
Comparing this equation with y = mx + c, we have
Slope = m = 1
y-intercept = c = -4
Let the inclination be 
.
Slope = 1 = tan = tan 45o
Solution 3
(i) 3x + 4y + 7 = 0
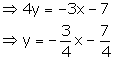
Slope of this line =
28x - 21y + 50 = 0
Slope of this line = 
Since, product of slopes of the two lines = -1, the lines are perpendicular to each other.
(ii) x - 3y = 4
3y = x - 4
y =
Slope of this line =
3x - y = 7
y = 3x - 7
Slope of this line = 3
Product of slopes of the two lines = 1 
-1
So, the lines are not perpendicular to each other.
(iii) 3x + 2y = 5
2y = -3x + 5
y = 
Slope of this line = 
x + 2y = 1
2y = -x + 1
y = 
Slope of this line = 
Since the slopes of two lines are not equal, the lines are not parallel to each other.
(iv) Given, the slope of the line through (1, 4) and (x, 2) is 2.
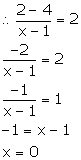
Solution 4
(i) x + 2y + 3 = 0
2y = -x - 3
y = 
Slope of this line
= 
Slope of the line
which is parallel to the given line = Slope of the given line =
(ii) 
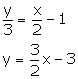
Slope of this line =
Slope of the line
which is parallel to the given line = Slope of the given line =
Solution 5
(i) 
Slope of this line = 2
Slope of the line
which is perpendicular to the given line = 
(ii) 
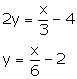
Slope of this line
= 
Slope of the line
which is perpendicular to the given line =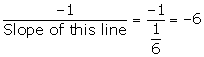 
Solution 6
(i) 2x - by + 5 = 0
by = 2x + 5
Slope of this line =
ax + 3y = 2
3y = -ax + 2
y = 
Slope of this line =
Since, the lines are parallel, the slopes of the two lines must be equal.
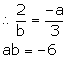
(ii) mx + 3y + 7 = 0
3y = -mx - 7
y = 
Slope of this line = 
5x - ny - 3 = 0
ny = 5x - 3
y = 
Slope of this line = 
Since, the lines are perpendicular; the product of their slopes is -1.
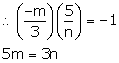
Solution 7
2x - y + 5 = 0
y = 2x + 5
Slope of this line = 2
px + 3y = 4
3y = -px + 4
y = 
Slope of this line = 
Since, the lines are perpendicular to each other, the product of their slopes is -1.
Solution 8
(i) 2x - 2y + 3 = 0
2y = 2x + 3
y = x + 
Slope of the line AB = 1
(ii) Required angle
= 
Slope = tan = 1 = tan 45o
= 45o
Solution 9
4x + 3y = 9
3y = -4x + 9
y = 
+ 3
Slope of this line
= 
px - 6y + 3 = 0
6y = px + 3
y = 
Slope of this line
= 
Since, the lines are parallel, their slopes will be equal.
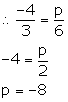
Solution 10
y = 3x + 7
Slope of this line = 3
2y + px = 3
2y = -px + 3
y = 
Slope of this line
= 
Since, the lines are perpendicular to each other, the product of their slopes is -1.
Solution 11
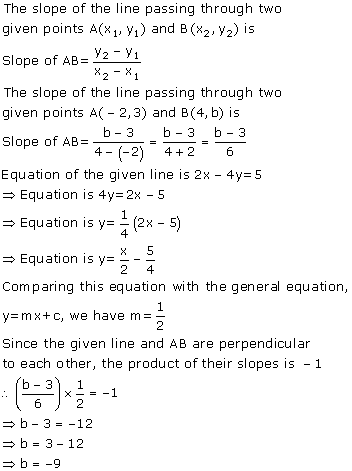
Solution 12
(i) The slope of the line parallel to x-axis is 0.
(x1, y1) = (-5, 7)
Required equation of the line is
y - y1 = m(x - x1)
y - 7 = 0(x + 5)
y = 7
(ii) The equation of a line parallel to y-axis is x = a where 'a' is the x-intercept.
Since the line passes through (-5, 7), the equation of a line parallel to given line x = -5.
That is, x + 5 = 0
Solution 13
(i) x - 3y = 4
3y = x - 4
Slope of this line = 
Slope of a line parallel to this line =
Required equation of the line passing through (5, -3) is
y - y1 = m(x - x1)
y + 3 = (x - 5)
3y + 9 = x - 5
x - 3y - 14 = 0
(ii) 3x + 2y = 8
2y = -3x + 8
y = 
Slope of given line = 
Since the required line is parallel to given line, their slopes are equal.
Slope of required line (m) =
Now, the equation of the line passing through (0, 1) is given by:
y - y1 = m(x - x1)
y - 1 = 
2y - 2 = -3x
3x + 2y = 2
Solution 14
4x + 5y = 6
5y = -4x + 6
y = 
Slope of this line
= 
The required line is perpendicular to the line 4x + 5y = 6.
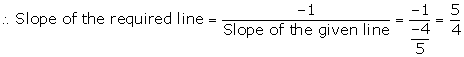
The required equation of the line is given by
y - y1 = m(x - x1)
y - 1 = 
(x + 2)
4y - 4 = 5x + 10
5x - 4y + 14 = 0
Solution 15
Let A = (6, -3) and B = (0, 3).
We know the perpendicular bisector of a line is perpendicular to the line and it bisects the line.
That is, it passes through the mid-point of the line.
Co-ordinates of the mid-point of AB are
Thus, the required line passes through (3, 0).
Slope of AB = 
Slope of the required line = 
Thus, the equation of the required line is given by:
y - y1 = m(x - x1)
y - 0 = 1(x - 3)
y = x - 3
Solution 16
(i) The co-ordinates of points A, B and C are (2, 3), (-1, 2) and (3, 0) respectively.
(ii) Slope of BC = 
Slope of a line parallel to BC = Slope of BC = 
Required equation of a line passing through A and parallel to BC is given by
y - y1 = m(x - x1)
y - 3 = (x - 2)
2y - 6 = -x + 2
x + 2y = 8
Solution 17
We know that in a rhombus, diagonals bisect each other at right angle.
Let O be the point of intersection of the diagonals AC and BD.
Co-ordinates of O are
Slope of BD = 
For line BD:
Slope = m = 
, (x1, y1)
= (-5, 6)
Equation of the line BD is
y - y1 = m(x - x1)
y - 6 = (x + 5)
3y - 18 = -x - 5
x + 3y = 13
For line AC:
Slope = m = 
, (x1, y1)
= (-2, 5)
Equation of the line AC is
y - y1 = m(x - x1)
y - 5 = 3(x + 2)
y - 5 = 3x + 6
y = 3x + 11
Solution 18
We know that in a square, diagonals bisect each other at right angle.
Let O be the point of intersection of the diagonals AC and BD.
Co-ordinates of O are
Slope of AC = 
For line AC:
Slope = m = 
, (x1, y1)
= (7, -2)
Equation of the line AC is
y - y1 = m(x - x1)
y + 2 = (x - 7)
2y + 4 = x - 7
2y = x - 11
For line BD:
Slope = m =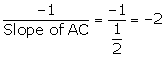 
, (x1, y1)
= (3, -4)
Equation of the line BD is
y - y1 = m(x - x1)
y + 4 = -2(x - 3)
y + 4 = -2x + 6
2x + y = 2
Solution 19
(i) We know the median through A will pass through the mid-point of BC. Let AD be the median through A.
Co-ordinates of the mid-point of BC, i.e., D are
Slope of AD = 
Equation of the median AD is
y - 3 = -8(x - 0)
8x + y = 3
(ii) Let BE be the altitude of the triangle through B.
Slope of AC = 
Slope of BE = 
Equation of altitude BE is
y - 2 = 
(x - 2)
3y - 6 = x - 2
3y = x + 4
(iii) Slope of AB =
Slope of the line parallel to AB = Slope of AB = 7
So, the equation of the line passing through C and parallel to AB is
y - 4 = 7(x + 2)
y - 4 = 7x + 14
y = 7x + 18
Solution 20
(i) 2y = 3x + 5
Slope of this line = 
Slope of the line AB =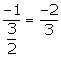 
(x1, y1) = (3, 2)
The required equation of the line AB is
y - y1 = m(x - x1)
y - 2 = 
(x - 3)
3y - 6 = -2x + 6
2x + 3y = 12
(ii) For the point A (the point on x-axis), the value of y = 0.
2x + 3y = 12 
2x = 12 x = 6
Co-ordinates of point A are (6, 0).
For the point B (the point on y-axis), the value of x = 0.
2x + 3y = 12 3y = 12 y = 4
Co-ordinates of point B are (0, 4).
Area of 
OAB = 
OA OB = 6 4 = 12 square units
Solution 21
For the point A (the point on x-axis), the value of y = 0.
4x - 3y + 12 = 0 
4x = -12 x = -3
Co-ordinates of point A are (-3, 0).
Here, (x1, y1) = (-3, 0)
The given line is 4x - 3y + 12 = 0
3y = 4x + 12
y =
+ 4
Slope of this line
= 
Slope of a line
perpendicular to the given line =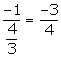 
Required equation of the line passing through A is
y - y1 = m(x - x1)
y - 0 = 
(x + 3)
4y = -3x - 9
3x + 4y + 9 = 0
Solution 22
(i) The given equation is
2x - 3y + 18 = 0
3y = 2x + 18
y = 
x + 6
Slope of this line
=
Slope of a line
perpendicular to this line =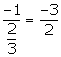 
(x1, y1) = (-5, 7)
The required equation of the line AP is given by
y - y1 = m(x - x1)
y - 7 = 
(x + 5)
2y - 14 = -3x - 15
3x + 2y + 1 = 0
(ii) P is the foot of perpendicular from point A.
So P is the point of intersection of the lines 2x - 3y + 18 = 0 and 3x + 2y + 1 = 0.
2x - 3y + 18 = 0 
4x - 6y + 36 = 0
3x + 2y + 1 = 0 9x + 6y + 3 = 0
Adding the two equations, we get,
13x + 39 = 0
x = -3
3y = 2x + 18 = -6 +
18 = 12
y = 4
Thus, the co-ordinates of the point P are (-3, 4).
Solution 23
For the line AB:
(x1, y1) = (4, 0)
Equation of the line AB is
y - y1 = m(x - x1)
y - 0 = -1(x - 4)
y = -x + 4
x + y = 4 ....(1)
For the line BC:
(x1, y1) = (2, 2)
Equation of the line BC is
y - y1 = m(x - x1)
y - 2 = -2(x - 2)
y - 2 = -2x + 4
2x + y = 6 ....(2)
Given that AB cuts the y-axis at P. So, the abscissa of point P is 0.
Putting x = 0 in (1), we get,
y = 4
Thus, the co-ordinates of point P are (0, 4).
Given that BC cuts the x-axis at Q. So, the ordinate of point Q is 0.
Putting y = 0 in (2), we get,
2x = 6 
x = 3
Thus, the co-ordinates of point Q are (3, 0).
Solution 24
Consider 
Putting x = 0 and y = 0 in the equation y = 2x, we have
LHS = 0 and RHS = 0
Thus, the line y = 2x passes through the origin.
Hence, A
L3
Consider 
Putting x = 0 in y - 2x + 2 = 0, we get, y = -2
Putting y = 0 in y - 2x + 2 = 0, we get, x = 1
So, x-intercept = 1 and y-intercept = -2
So, x-intercept is positive and y-intercept is negative.
Hence, BL4
Consider 
Putting x = 0 in 3x + 2y = 6, we get, y = 3
Putting y = 0 in 3x + 2y = 6, we get, x = 2
So, both x-intercept and y-intercept are positive.
Hence, CL2
Consider 
The slope of the line y = 2 is 0.
So, the line y = 2 is parallel to x-axis.
Hence, DL1
Solution 25
Equation of a Line Exercise Ex. 14(E)
Solution 1
Using section formula, the co-ordinates of the point P are
3x + 5y = 7
Slope of this line = 
As the required line is parallel to the line 3x + 5y = 7,
Slope of the required line = Slope of the given line =
Thus, the equation of the required line is
y - y1 = m(x - x1)
y + 3 = (x - 11)
5y + 15 = -3x + 33
3x + 5y = 18
Solution 2
Using section formula, the co-ordinates of the point P are
The equation of the given line is
5x - 3y = 4
Slope of this line = 
Since, the required line is perpendicular to the given line,
Slope of the required line =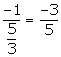 
Thus, the equation of the required line is
y - y1 = m(x - x1)
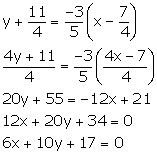
Solution 3
Point P lies on y-axis, so putting x = 0 in the equation 5x + 3y + 15 = 0, we get, y = -5
Thus, the co-ordinates of the point P are (0, -5).
x - 3y + 4 = 0 
Slope of this line =
The required equation is perpendicular to given equation x - 3y + 4 = 0.
Slope of the required line =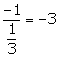 
(x1, y1) = (0, -5)
Thus, the required equation of the line is
y - y1 = m(x - x1)
y + 5 = -3(x - 0)
3x + y + 5 = 0
Solution 4
kx - 5y + 4 = 0
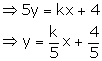
Slope of this line = m1 =
5x - 2y + 5 = 0
Slope of this line = m2 =
Since, the lines are perpendicular, m1 × m2 = -1
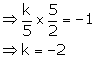
Solution 5
(i) Slope of PQ = 
Equation of the line PQ is given by
y - y1 = m(x - x1)
y - 4 = -1(x + 1)
y - 4 = -x - 1
x + y = 3
(ii) For point A (on x-axis), y = 0.
Putting y = 0 in the equation of PQ, we get,
x = 3
Thus, the co-ordinates of point A are (3, 0).
For point B (on y-axis), x = 0.
Putting x = 0 in the equation of PQ, we get,
y = 3
Thus, the co-ordinates of point B are (0, 3).
(iii) M is the mid-point of AB.
So, the co-ordinates of point M are
Solution 6
A = (1, 5) and C = (-3, -1)
Slope of AC = 
For line AC:
Slope = m = 
, (x1, y1) = (1, 5)
Equation of the line AC is
y - y1 = m(x - x1)
y - 5 = (x - 1)
2y - 10 = 3x - 3
3x - 2y + 7 = 0
For line BD:
We know that in a rhombus, diagonals bisect each other at right angle.
Let O be the point of intersection of the diagonals AC and BD.
Co-ordinates of O are
Slope od line BD = m = 
, (x1, y1) = (-1, 2)
Equation of the line BD is
y - y1 = m(x - x1)
y - 2 = 
(x + 1)
3y - 6 = -2x - 2
2x + 3y = 4
Solution 7
Solution 8
The given line is
x = 3y + 2 ...(1)
3y = x - 2
Slope of this line is 
.
The required line intersects the given line at right angle.
Slope of the required line =
The required line passes through (0, 0) = (x1, y1)
The equation of the required line is
y - y1 = m(x - x1)
y - 0 = -3(x - 0)
3x + y = 0 ...(2)
Point X is the intersection of the lines (1) and (2).
Using (1) in (2), we get,
9y + 6 + y = 0
Thus, the co-ordinates of the point X are 
.
Solution 9
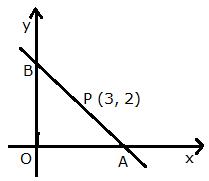
Let the line intersect the x-axis at point A (x, 0) and y-axis at point B (0, y).
Since, P is the mid-point of AB, we have:
Thus, A = (6, 0) and B = (0, 4)
Slope of line AB = 
Let (x1, y1) = (6, 0)
The required equation of the line AB is given by
y - y1 = m(x - x1)
y - 0 = 
(x - 6)
3y = -2x + 12
2x + 3y = 12
Solution 10
7x + 6y = 71 
28x + 24y = 284 ...(1)
5x - 8y = -23 15x - 24y = -69 ...(2)
Adding (1) and (2), we get,
43x = 215
x = 5
From equation 5x - 8y = -23,
8y = 5x + 23
8y = 25 + 23
8y = 48
y = 6
Thus, the required line passes through the point (5, 6).
4x - 2y = 1
2y = 4x - 1
y = 2x -
Slope of this line = 2
Slope of the required line = 
The required equation of the line is
y - y1 = m(x - x1)
y - 6 = (x - 5)
2y - 12 = -x + 5
x + 2y = 17
Solution 11
The given line is
Slope of this line =
Slope of the required line =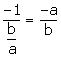 
Let the required line passes through the point P (0, y).
Putting x = 0 in the equation 
, we get,
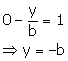
Thus, P = (0, -b) = (x1, y1)
The equation of the required line is
y - y1 = m(x - x1)
y + b = 
(x - 0)
by + b2 = -ax
ax + by + b2 = 0
Solution 12
(i) Let the median through O meets AB at D. So, D is the mid-point of AB.
Co-ordinates of point D are
Slope of OD = 
(x1, y1) = (0, 0)
The equation of the median OD is
y - y1 = m(x - x1)
y - 0 = -1(x - 0)
x + y = 0
(ii) The altitude through vertex B is perpendicular to OA.
Slope of OA = 
Slope of the required altitude =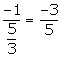 
The equation of the required altitude through B is
y - y1 = m(x - x1)
y + 3 = 
(x + 5)
5y + 15 = -3x - 15
3x + 5y + 30 = 0
Solution 13
Let A = (-2, 3) and B = (4, 1)
Slope of AB = m1 = 
Slope of the given line 3x = y + 1 is 3 = m2.
Hence, the line through points A and B is perpendicular to the given line.
Equation of line AB is
y - y1 = m1(x - x1)
y - 3 = 
(x + 2)
3y - 9 = -x - 2
x + 3y = 7 ...(1)
Given line is 3x = y +1 ...(2)
Solving (1) and (2), we get,
x = 1 and y = 2
So, the two lines intersect at point P = (1, 2).
The co-ordinates of the mid-point of AB are
Hence, the line 3x = y + 1 bisects the line segment joining the points A and B.
Solution 14
x cos 
+ y sin = 2
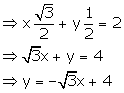
Slope of this line = 
Slope of a line which is parallel to this given line =
Let (4, 3) = (x1, y1)
Thus, the equation of the required line is given by:
y - y1 = m(x - x1)
y - 3 = (x - 4)
Solution 15
(k - 2)x + (k + 3)y - 5 = 0 ....(1)
(k + 3)y = -(k - 2)x + 5
y =
Slope of this line = m1 = 
(i) 2x - y + 7 = 0
y = 2x + 7
Slope of this line = m2 = 2
Line (1) is perpendicular to 2x - y + 7 = 0.
(ii) Line (1) is parallel to 2x - y + 7 = 0.
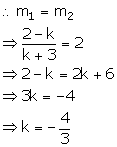
Solution 16
Slope of BC = 
Equation of the line BC is given by
y - y1 = m(x - x1)
y + 2 = 
(x + 1)
4y + 8 = 3x + 3
3x - 4y = 5....(1)
(i) Slope of line perpendicular to BC =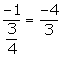 
Required equation of the line through A (0, 5) and perpendicular to BC is
y - y1 = m(x - x1)
y - 5 = 
(x - 0)
3y - 15 = -4x
4x + 3y = 15 ....(2)
(ii) The required point will be the point of intersection of lines (1) and (2).
(1) 
9x - 12y = 15
(2) 16x + 12y = 60
Adding the above two equations, we get,
25x = 75
x = 3
So, 4y = 3x - 5 = 9 - 5 = 4
y = 1
Thus, the co-ordinates of the required point is (3, 1).
Solution 17
(i) A = (2, 3), B = (-1, 2), C = (3, 0)
(ii) Slope of BC = 
Slope of required line which is parallel to BC = Slope of BC =
(x1, y1) = (2, 3)
The required equation of the line through A and parallel to BC is given by:
y - y1 = m(x - x1)
y - 3 = (x - 2)
2y - 6 = -x + 2
x + 2y = 8
Solution 18
The median (say RX) through R will bisect the line PQ.
The co-ordinates of point X are
Slope of RX =
(x1, y1) = (-2, -1)
The required equation of the median RX is given by:
y - y1 = m1(x - x1)
y + 1 = 
(x + 2)
7y + 7 = 2x + 4
7y = 2x - 3
Solution 19
P is the mid-point of AB. So, the co-ordinate of point P are
Q is the mid-point of AC. So, the co-ordinate of point Q are
Slope of PQ = 
Slope of BC = 
Since, slope of PQ = Slope of BC,
PQ || BC
Also, we have:
Slope of PB = 
Slope of QC =
Thus, PB is not parallel to QC.
Hence, PBCQ is a trapezium.
Solution 20
(i) Let the co-ordinates of point A (lying on x-axis) be (x, 0) and the co-ordinates of point B (lying y-axis) be (0, y).
Given, P = (-4, -2) and AP: PB = 1:2
Using section formula, we have:
Thus, the co-ordinates of A and B are (-6, 0) and (0, -6).
(ii) Slope of AB = 
Slope of the required line perpendicular to AB = 
(x1, y1) = (-4, -2)
Required equation of the line passing through P and perpendicular to AB is given by
y - y1 = m(x - x1)
y + 2 = 1(x + 4)
y + 2 = x + 4
y = x + 2
Solution 21
The required line intersects x-axis at point A (-2, 0).
Also, y-intercept = 3
So, the line also passes through B (0, 3).
Slope of line AB = 
= m
(x1, y1) = (-2, 0)
Required equation of the line AB is given by
y - y1 = m(x - x1)
y - 0 = 
(x + 2)
2y = 3x + 6
Solution 22
The required line passes through A (2, 3).
Also, x-intercept = 4
So, the required line passes through B (4, 0).
Slope of AB = 
(x1, y1) = (4, 0)
Required equation of the line AB is given by
y - y1 = m(x - x1)
y - 0 = 
(x - 4)
2y = -3x + 12
3x + 2y = 12
Solution 23
Equation of the line AB is y = x + 1
Slope of AB = 1
Inclination of line AB = 
(Since, tan 45o = 1)
Equation of line CD is y = 
x - 1
Slope of CD =
Inclination of line CD = 60o (Since, tan 60o =)
Using angle sum property in 
PQR,
Solution 24
Given, P divides the line segment joining A (-2, 6) and B (3, -4) in the ratio 2: 3.
Co-ordinates of point P are
Slope of the required line = m =
The required equation of the line is given by
y - y1 = m(x - x1)
y - 2 = (x - 0)
2y - 4 = 3x
2y = 3x + 4
Solution 25
Let A = (6, 4) and B = (7, -5)
Slope of the line AB = 
(x1, y1) = (6, 4)
The equation of the line AB is given by
y - y1 = m(x - x1)
y - 4 = -9(x - 6)
y - 4 = -9x + 54
9x + y = 58 ...(1)
Now, given that the ordinate of the required point is -23.
Putting y = -23 in (1), we get,
9x - 23 = 58
9x = 81
x = 9
Thus, the co-ordinates of the required point is (9, -23).
Solution 26
Given points are A(7, -3) and B(1, 9).
(i) Slope of AB = 
(ii) Slope of perpendicular bisector of AB 
Mid-point of AB = 
=(4, 3)
Equation of perpendicular bisector is:
y - 3 = 
(x - 4)
2y - 6 = x - 4
x - 2y + 2 = 0
(iii) Point (-2, p) lies on x - 2y + 2 = 0.
-2 - 2p + 2 = 0
2p = 0
p = 0
Solution 27
(i) Let the co-ordinates be A(x, 0) and B(0, y).
Mid-point of A and B
is given by 
(ii) Slope of line
AB, m = 
(iii) Equation of line AB, using A(4, 0)
2y = 3x - 12
Solution 28
3x + 4y - 7 = 0 ...(1)
4y = -3x + 7
y = 
(i) Slope of the line = m = 
(ii) Slope of the line perpendicular to the given line =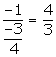 
Solving the equations x - y + 2 = 0 and 3x + y - 10 = 0, we get x = 2 and y = 4.
So, the point of intersection of the two given lines is (2, 4).
Given that a line with slope 
passes through point (2, 4).
Thus, the required equation of the line is
y - 4 = (x - 2)
3y - 12 = 4x - 8
4x - 3y + 4 = 0
Solution 29
In parallelogram ABCD, A(x, y), B(5, 8), C(4, 7) and D(2, -4).
The diagonals of the parallelogram bisect each other.
O is the point of intersection of AC and BD.
Since O is the midpoint of BD, its coordinates will be
(i)
Since O is the midpoint of AC also,
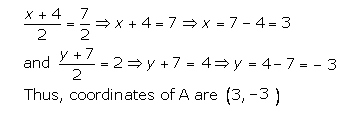
(ii)
Slope of BD
Therefore, the equation of BD is given by
Solution 30
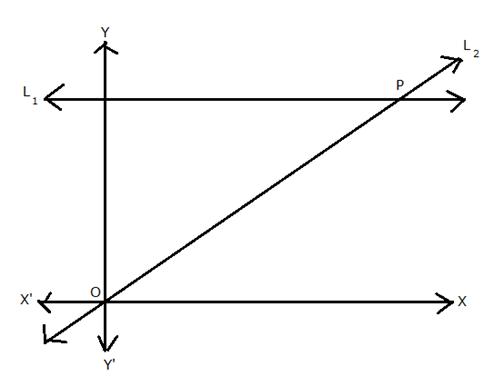
(i) 
Since L2 is the bisector of angle O,
(ii) Let the co-ordinates of P be (x, y).
Since P lies on L1, its y-coordinate is 4.
Also, L2 passes through orgin,
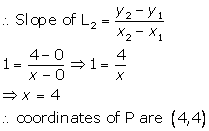
(iii)
Solution 31
Solution 32
Solution 33
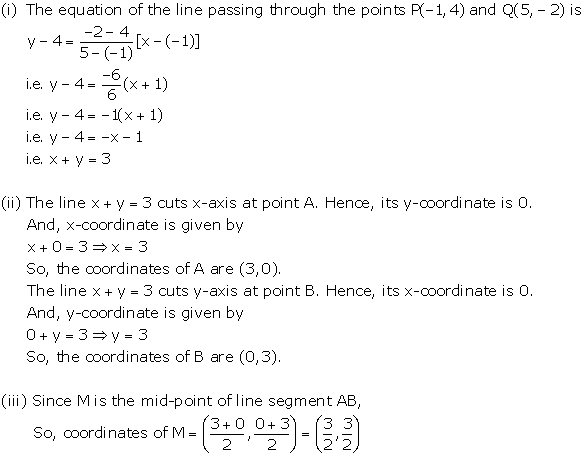
Solution 34
Solution 35
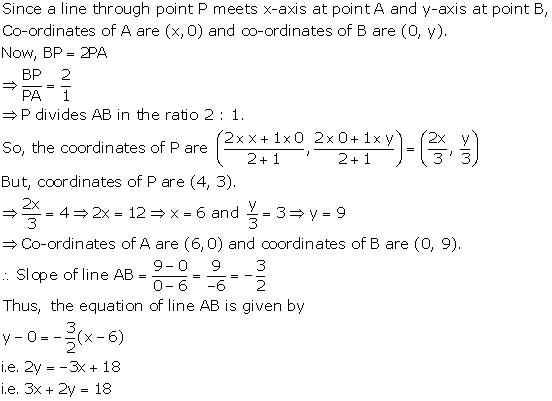
Solution 36
Solution 37
Solution 38
Solution 39
Solution 40
Solution 41
i. Since A lies on the X-axis, let the co-ordinates of A be (x, 0).
Since B lies on the Y-axis, let the co-ordinates of B be (0, y).
Let m = 1 and n = 2
Using Section formula,
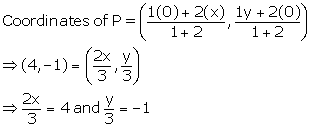
⇒ x = 6 and y = -3
So, the co-ordinates of A are (6, 0) and that of B are (0, -3).
⇒ Slope of line perpendicular to AB = m = -2
P = (4, -1)
Thus, the required equation is
y - y1 = m(x - x1)
⇒ y - (-1) = -2(x - 4)
⇒ y + 1 = -2x + 8
⇒ 2x + y = 7
