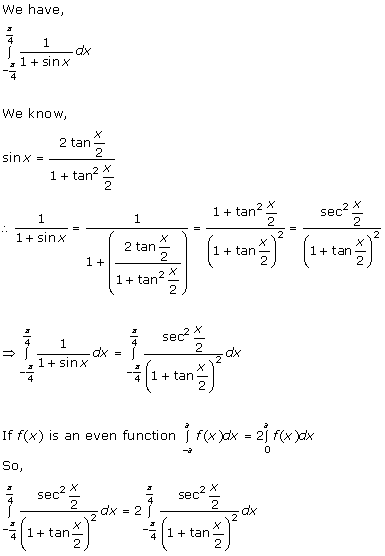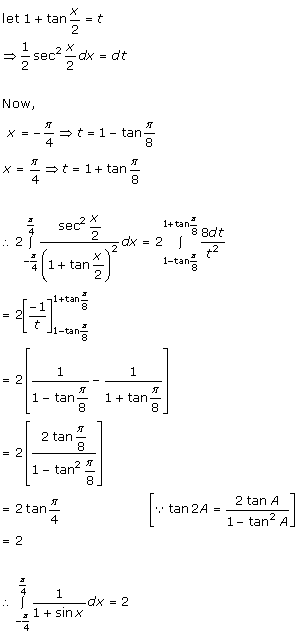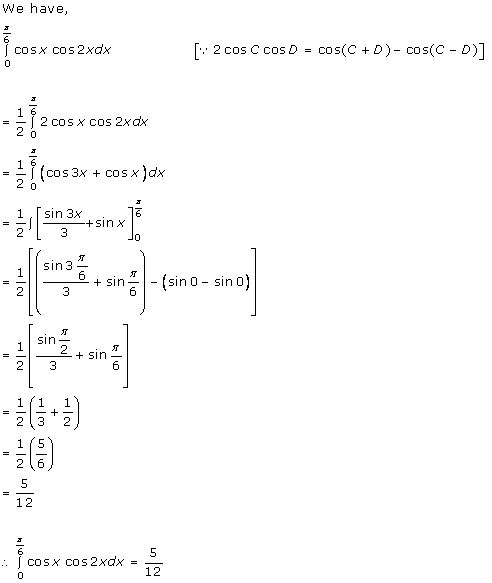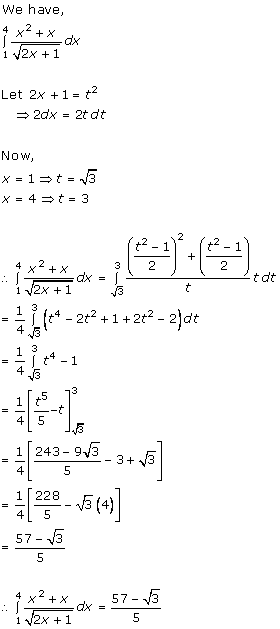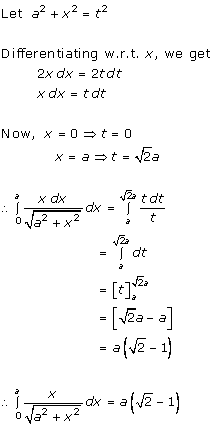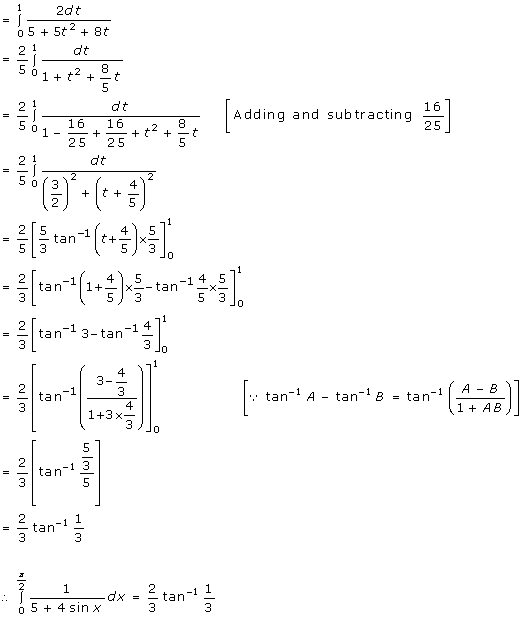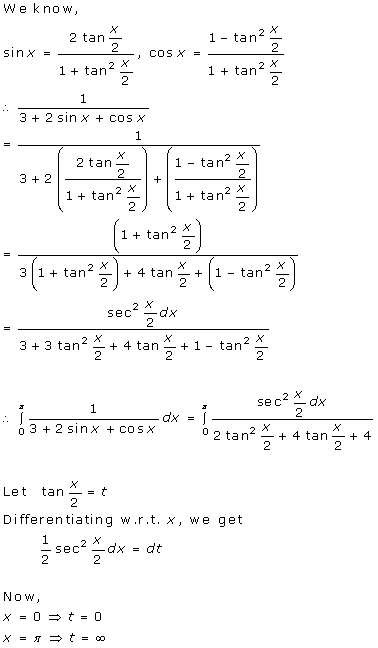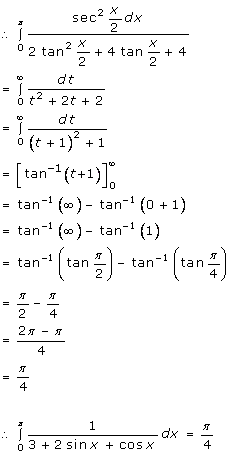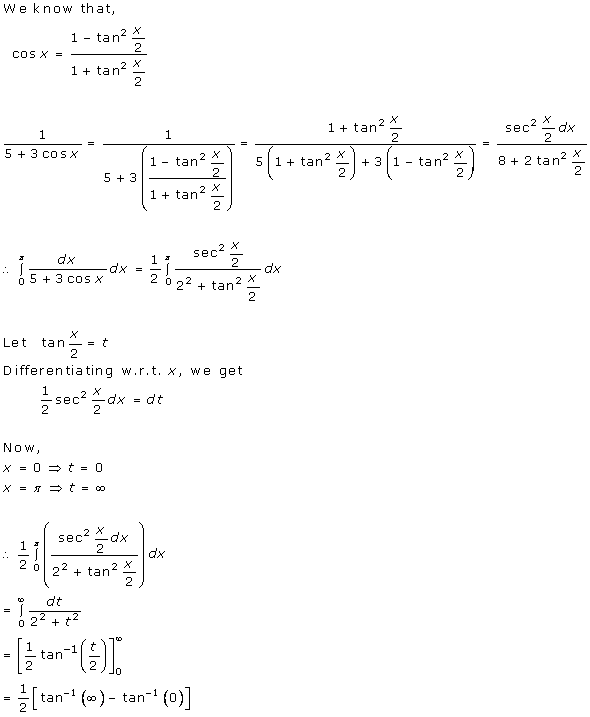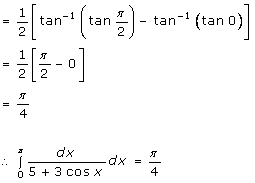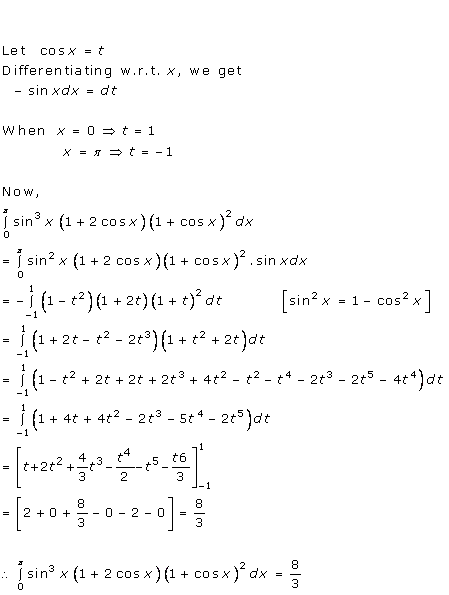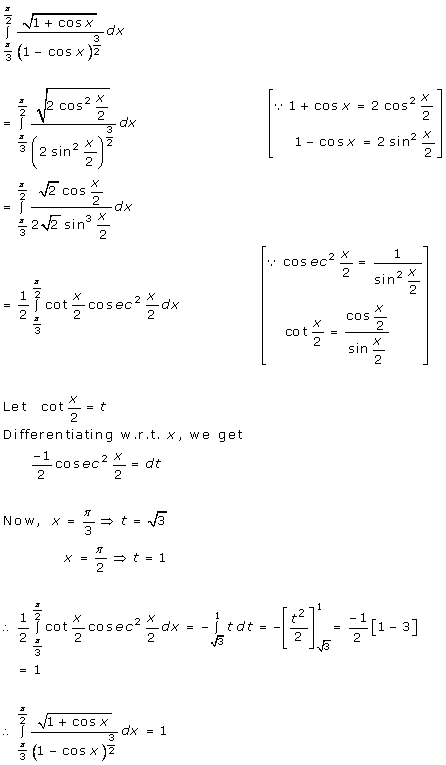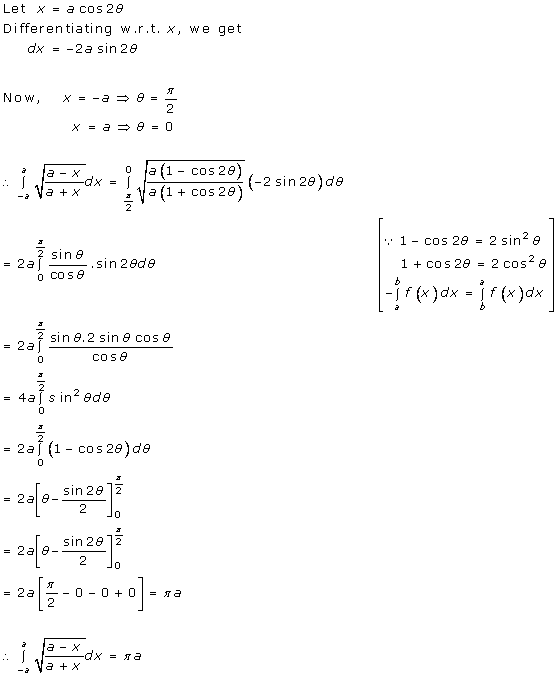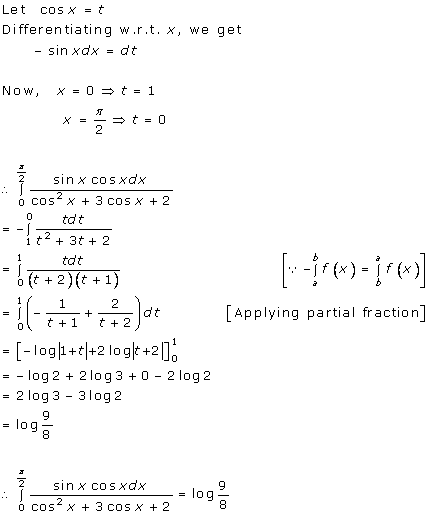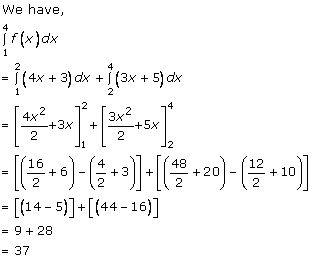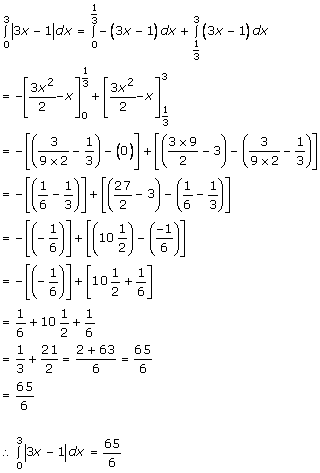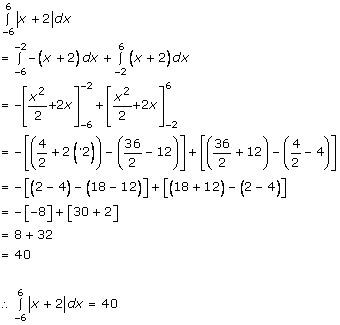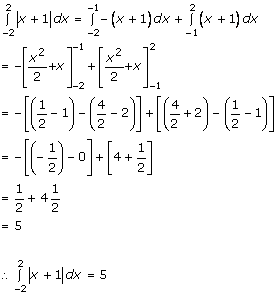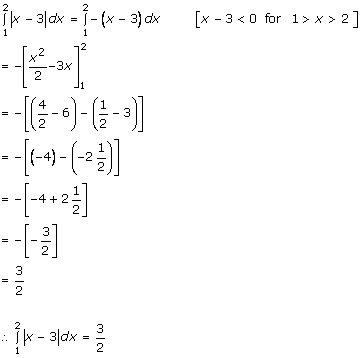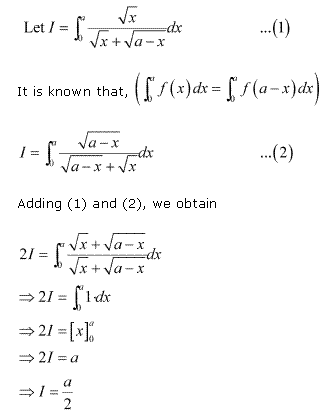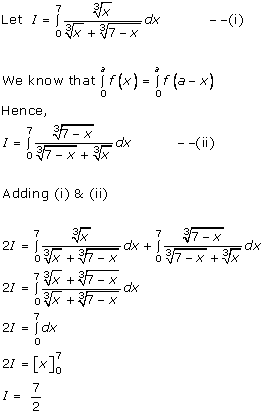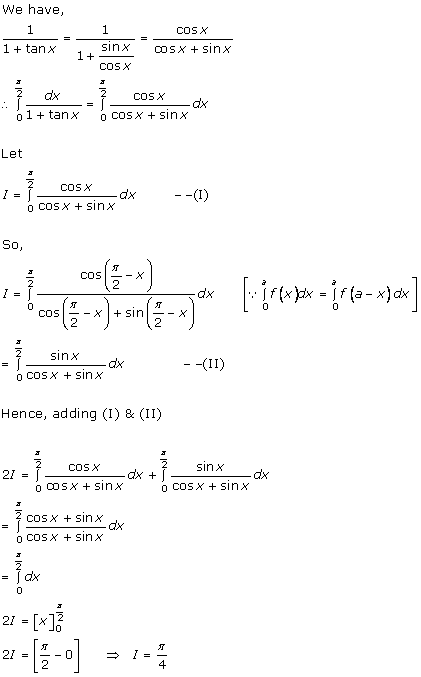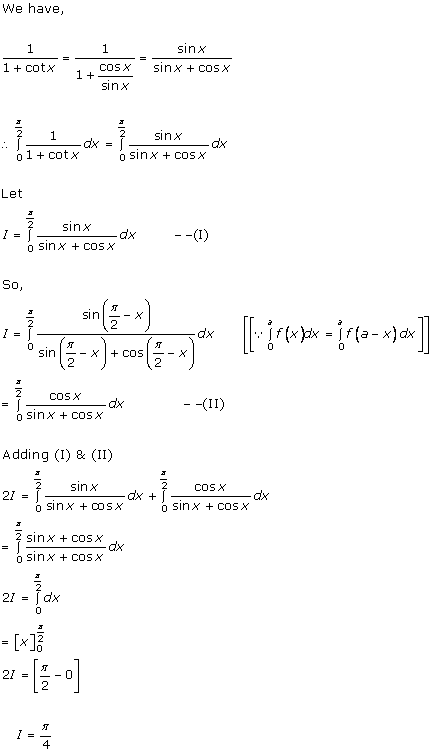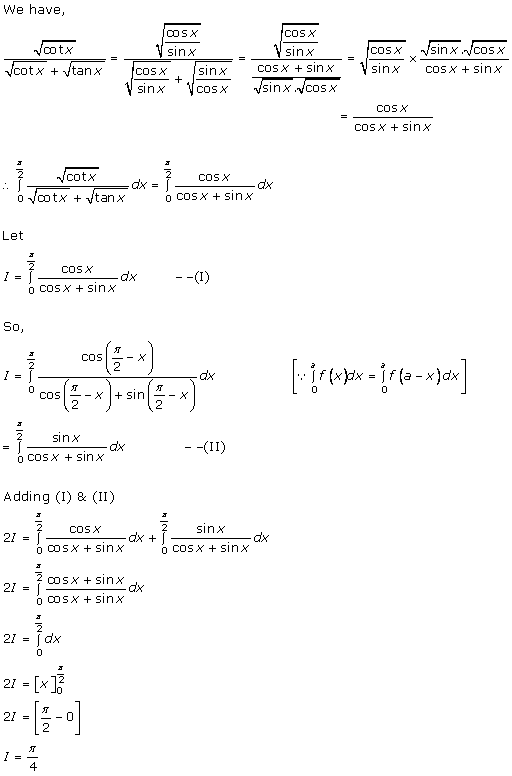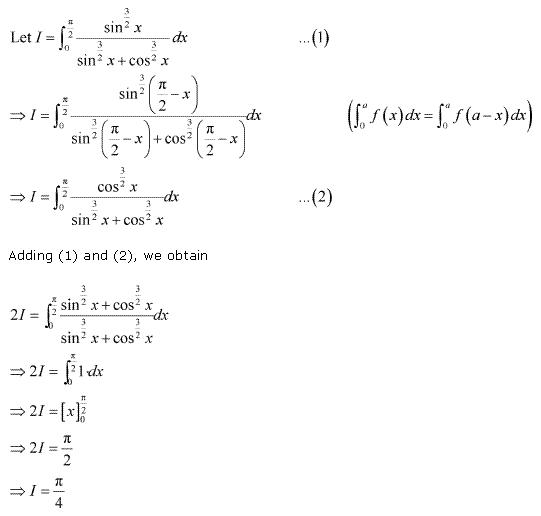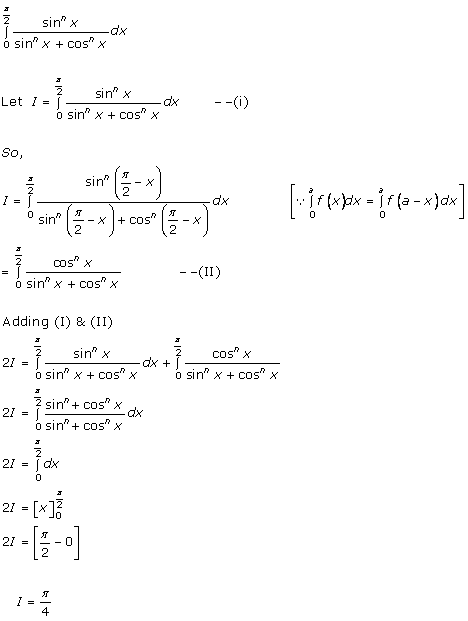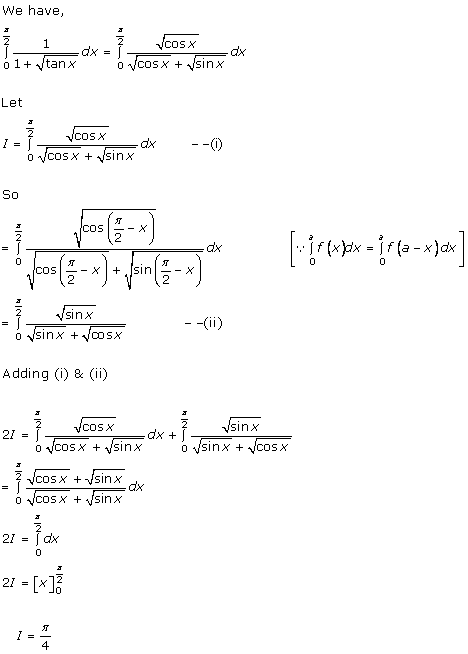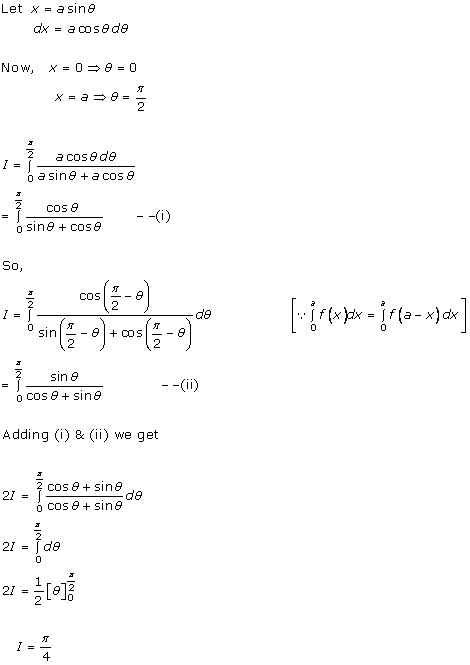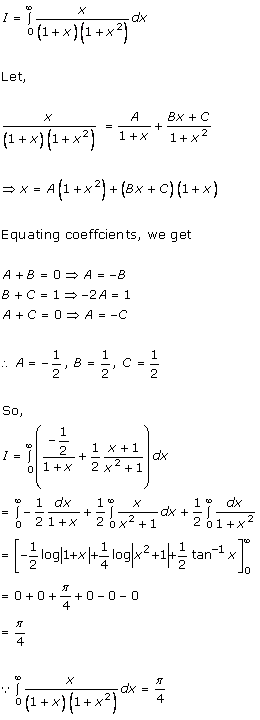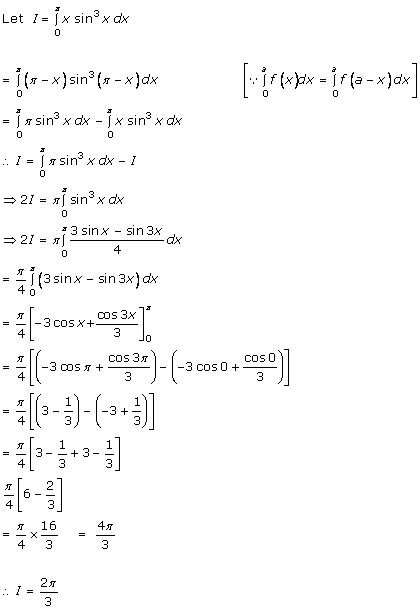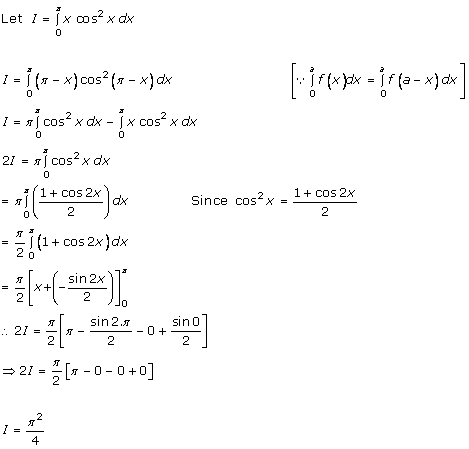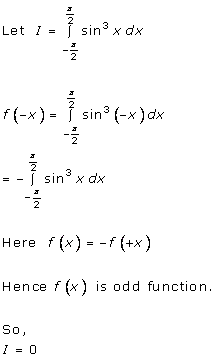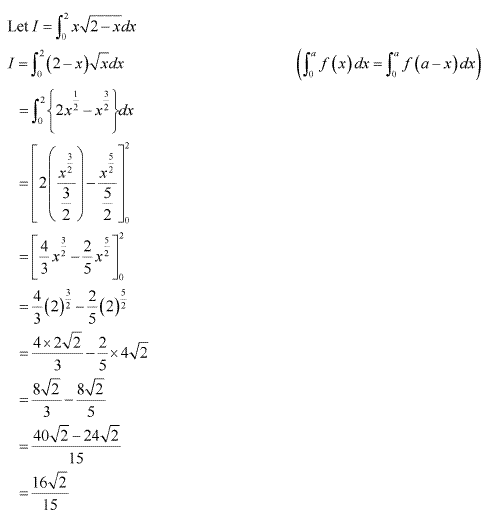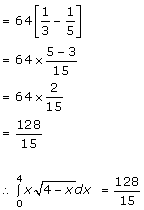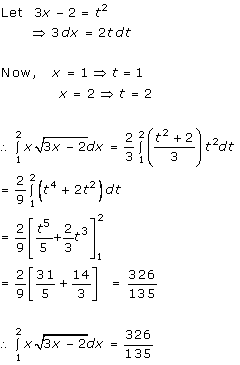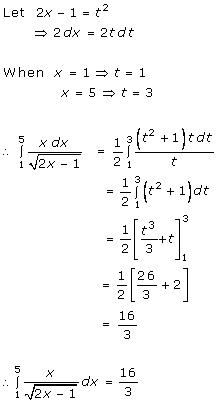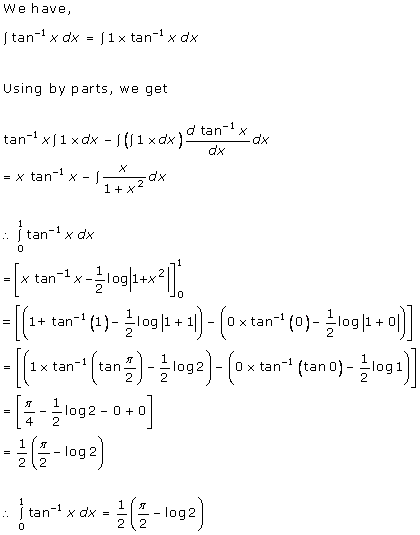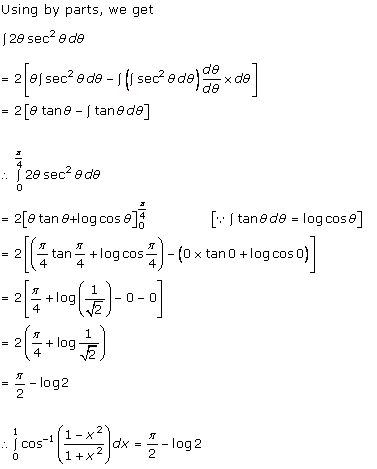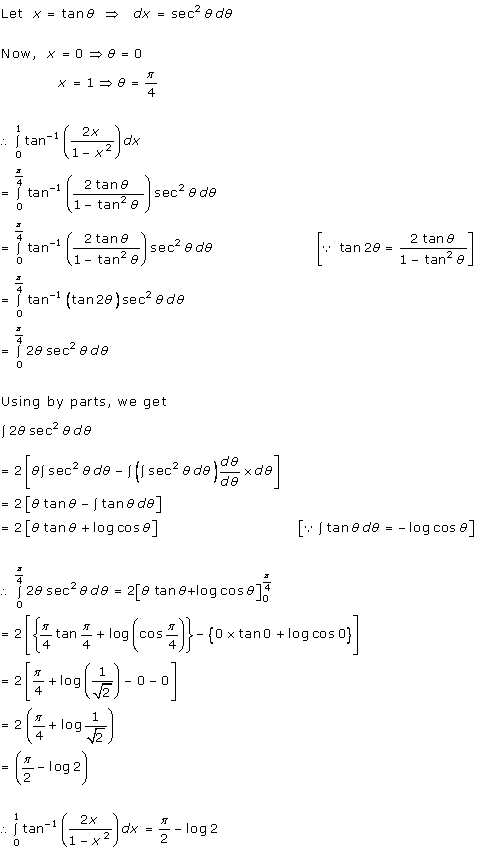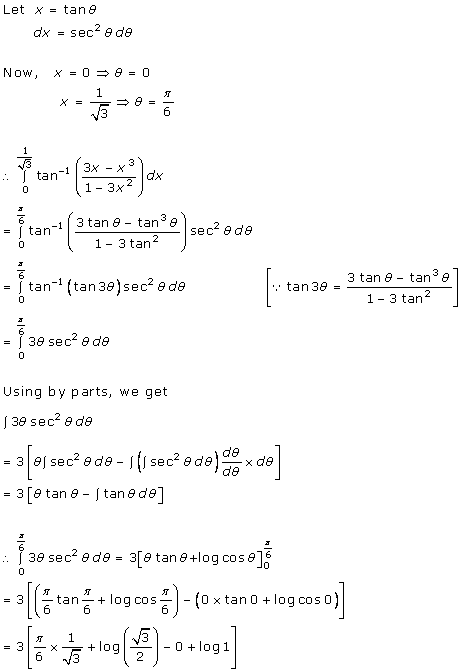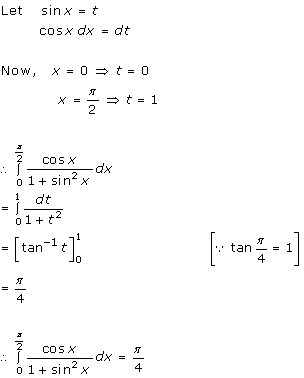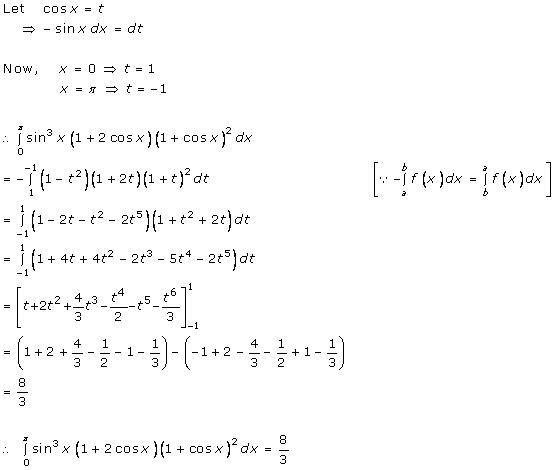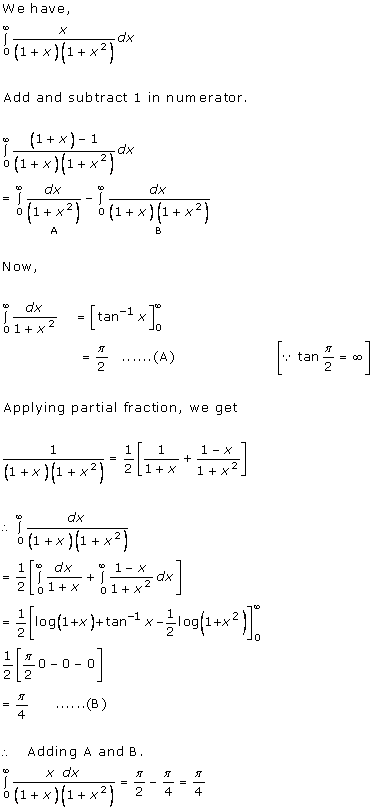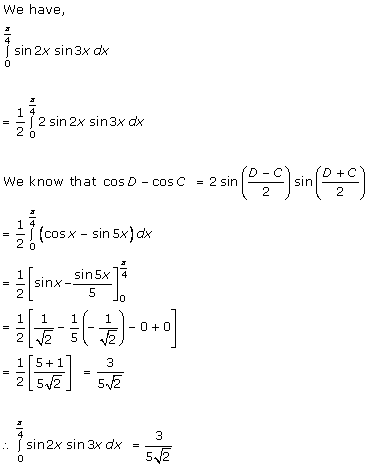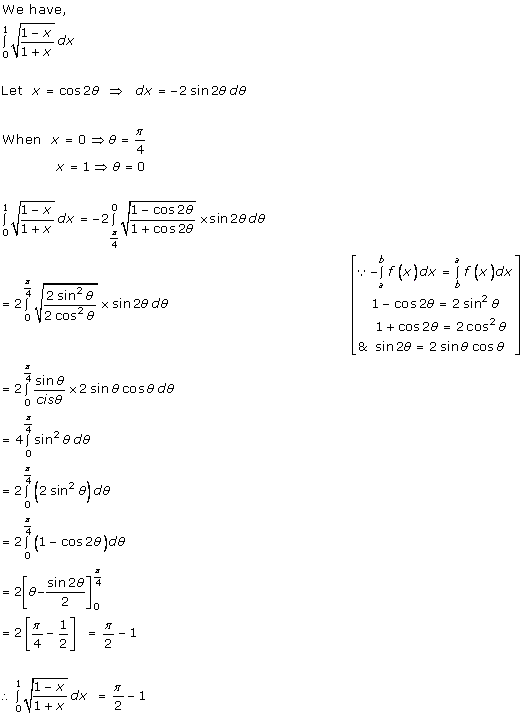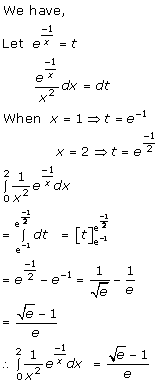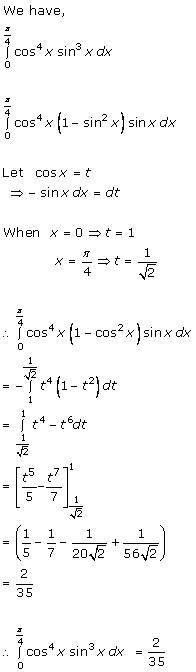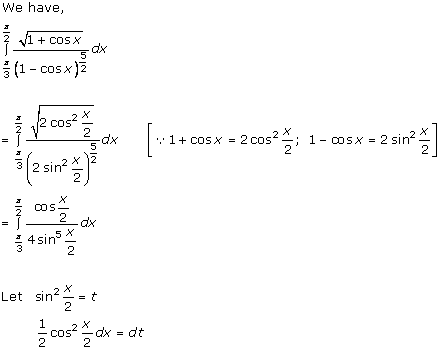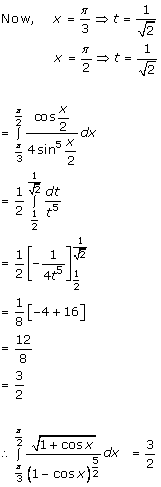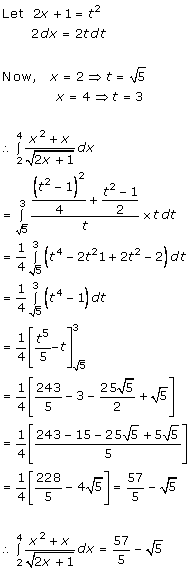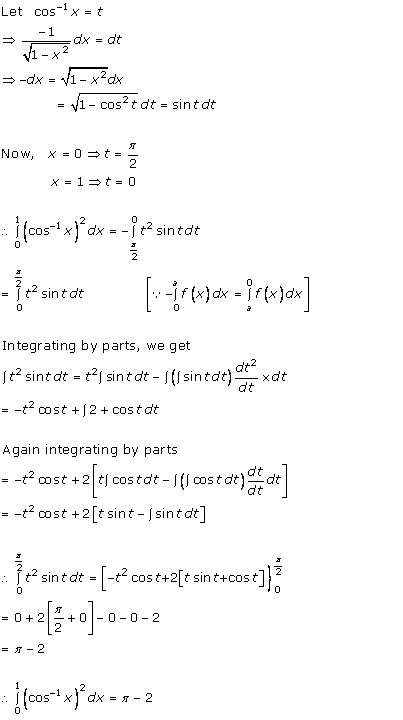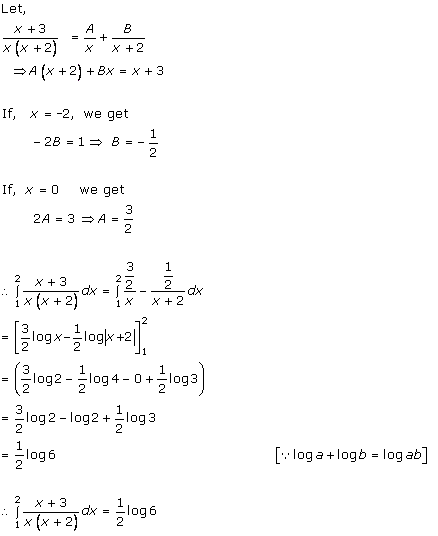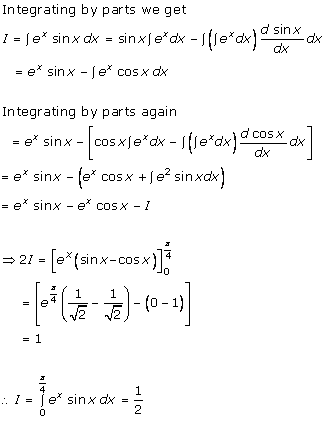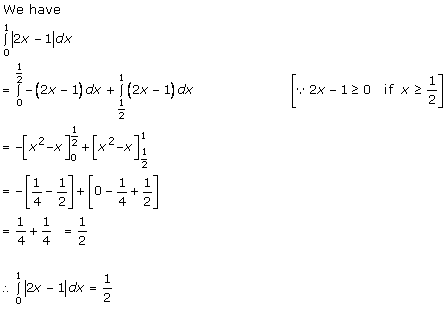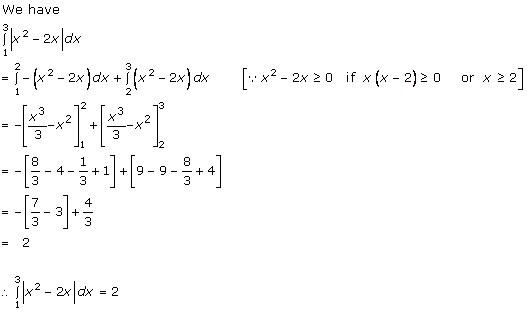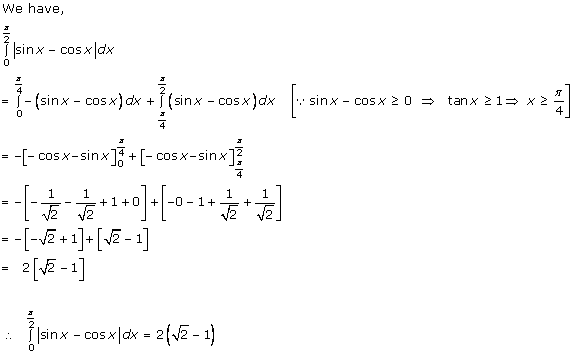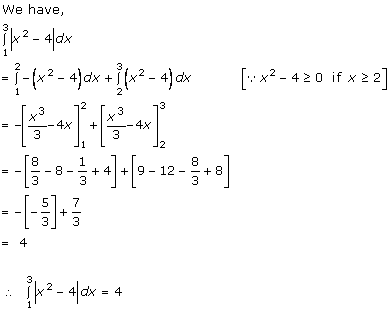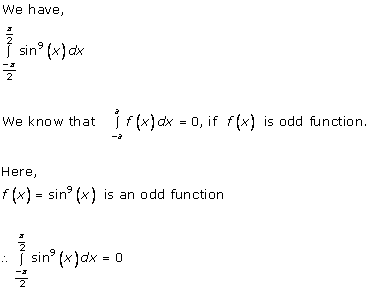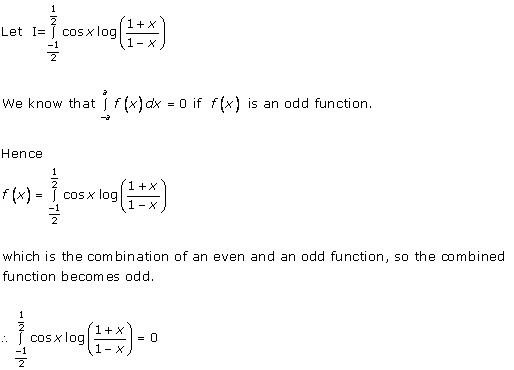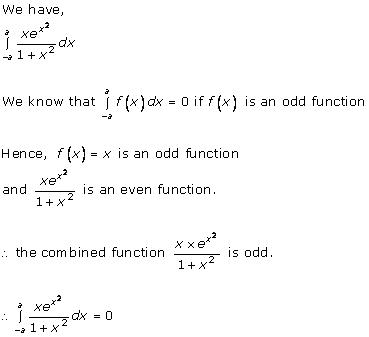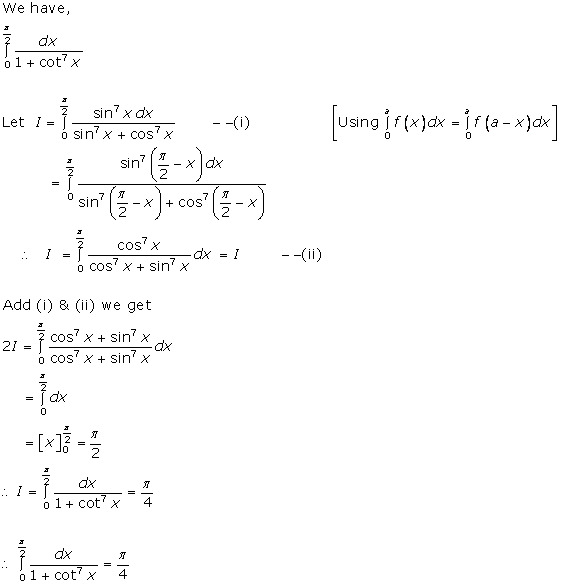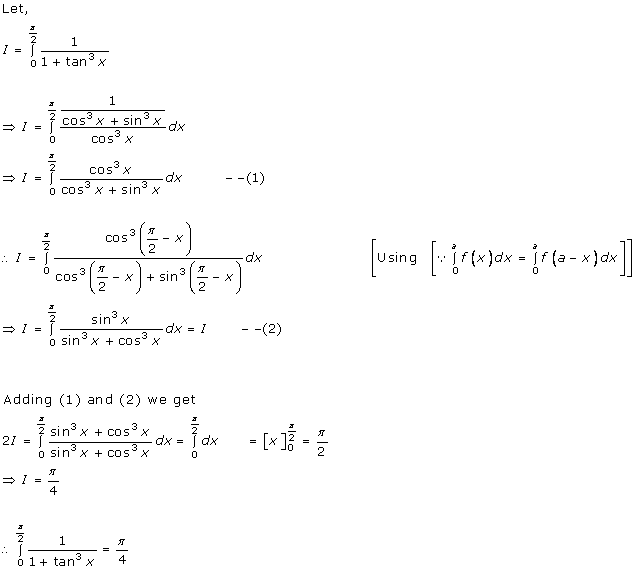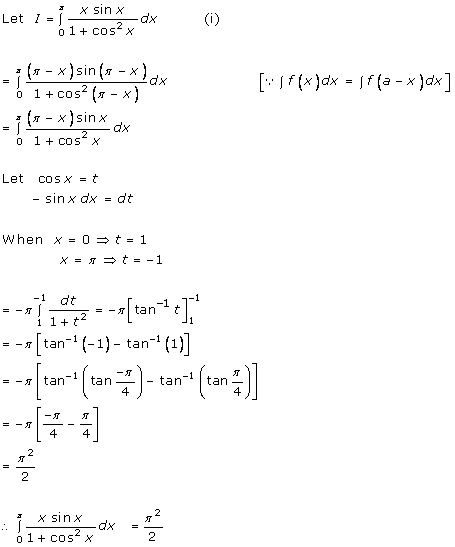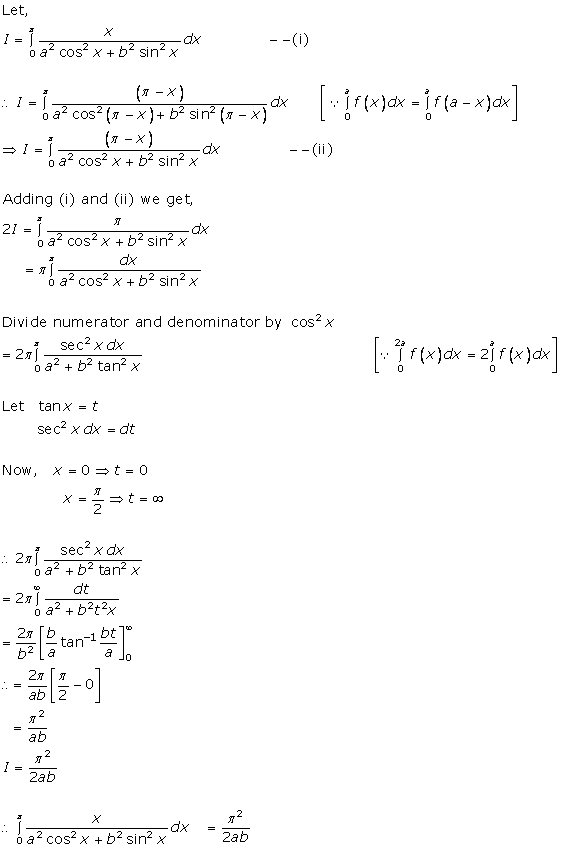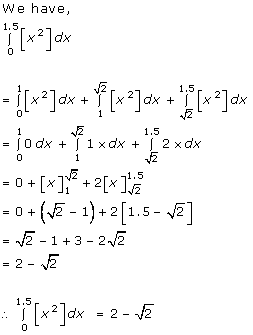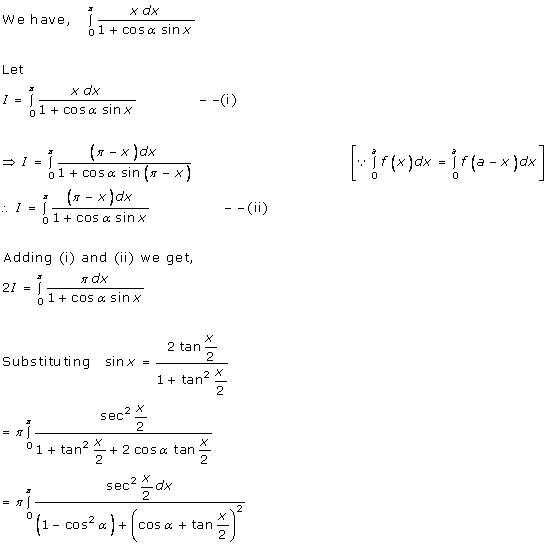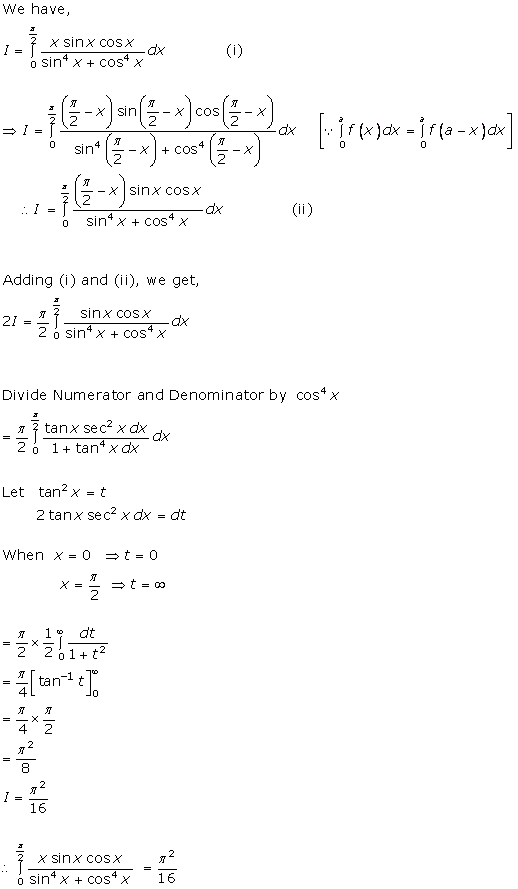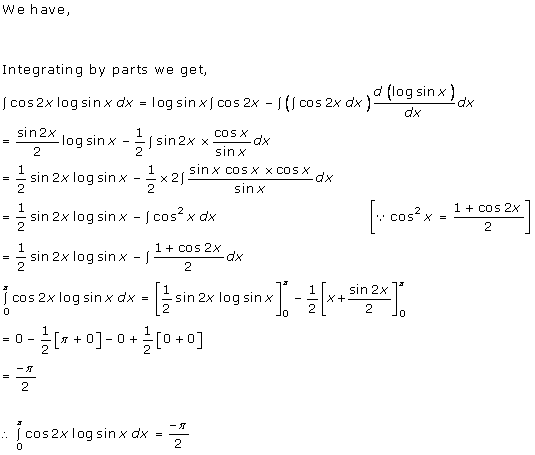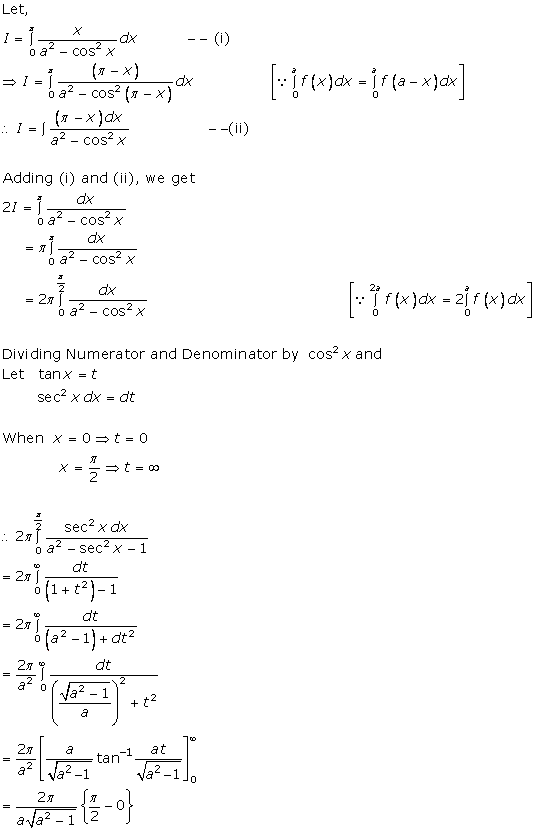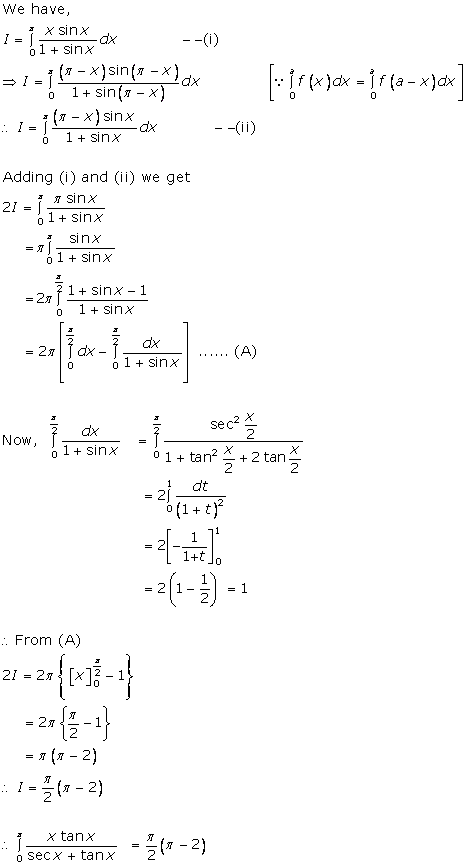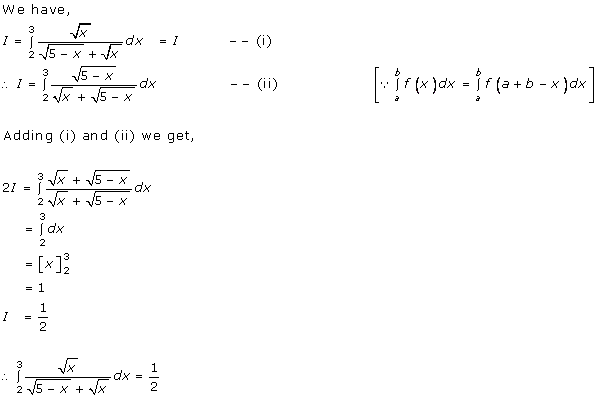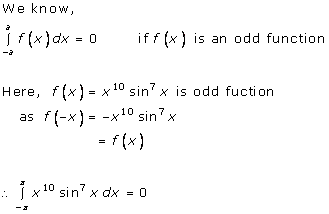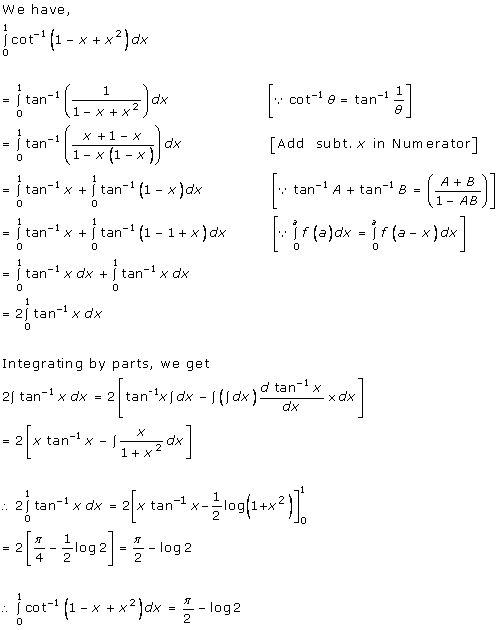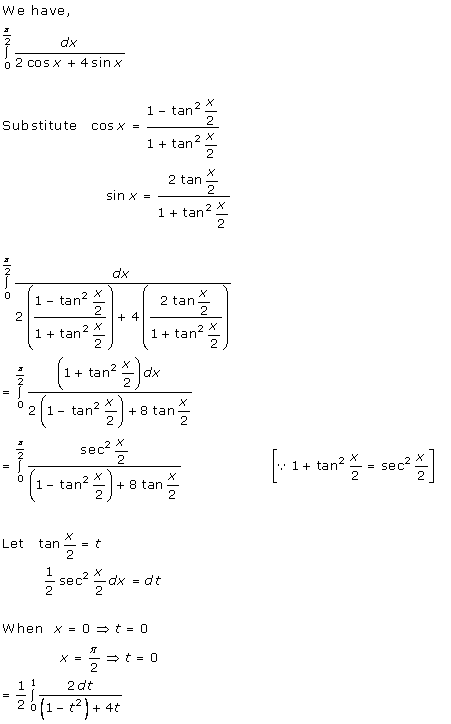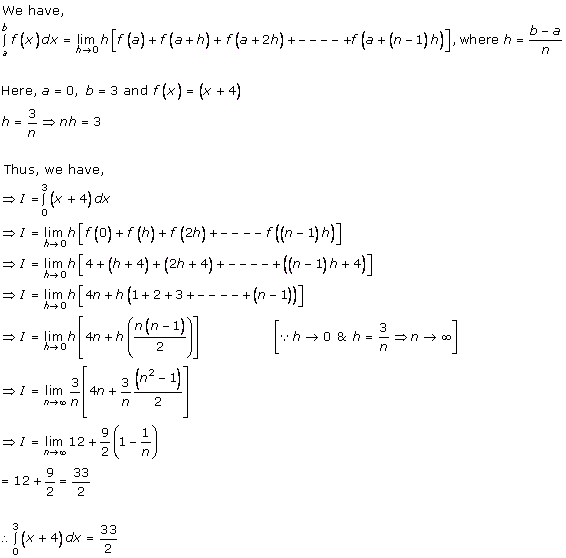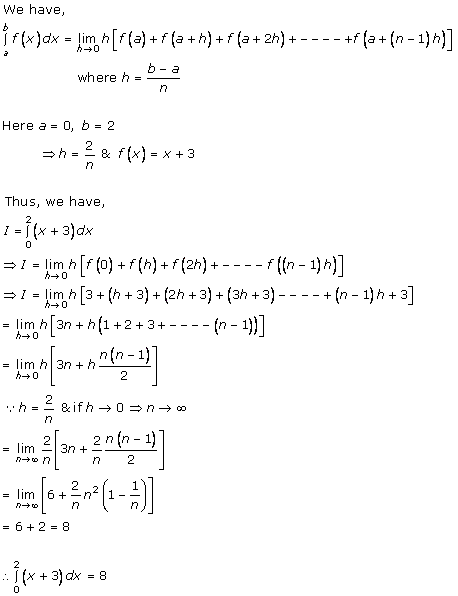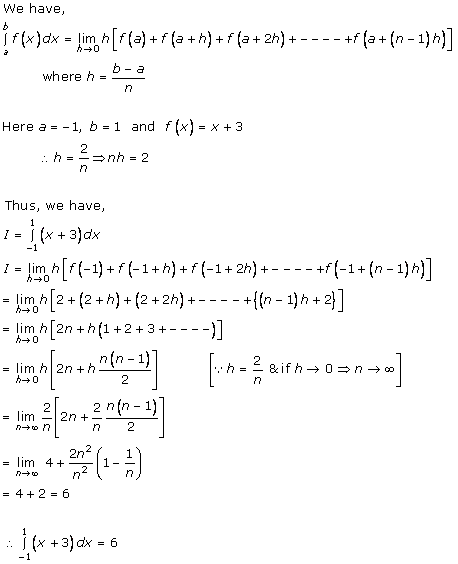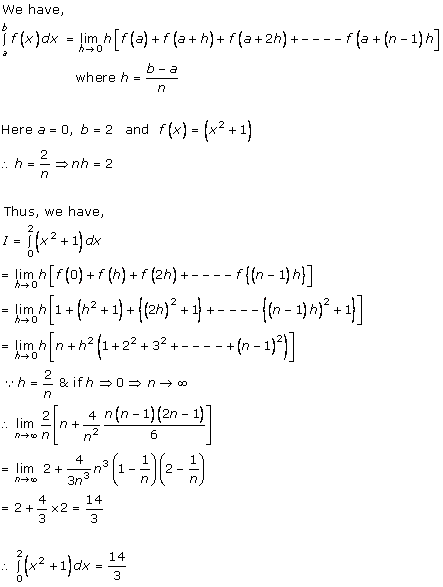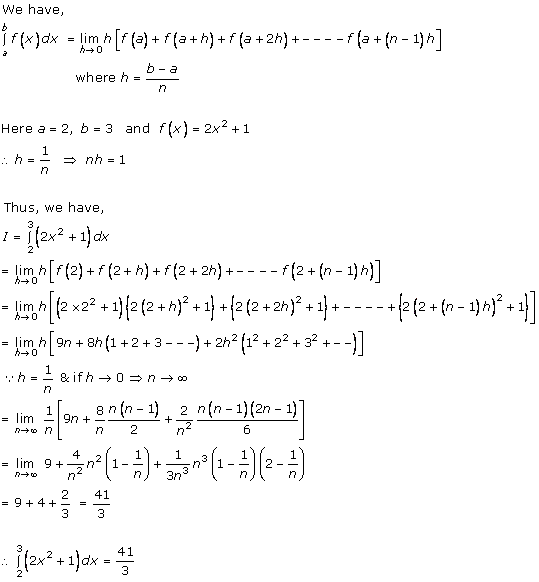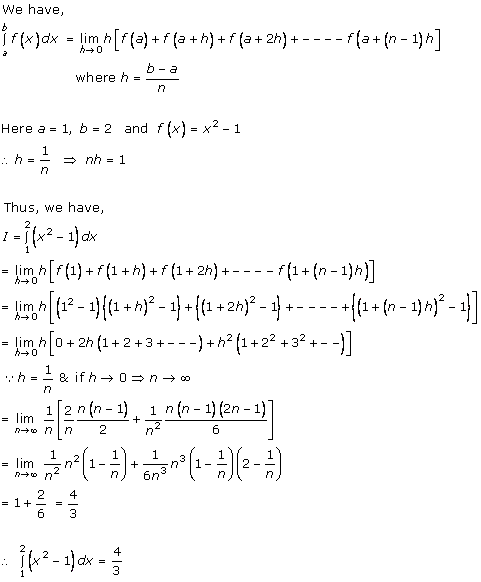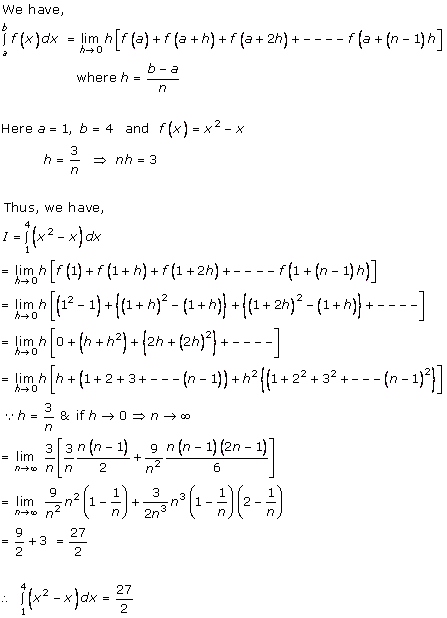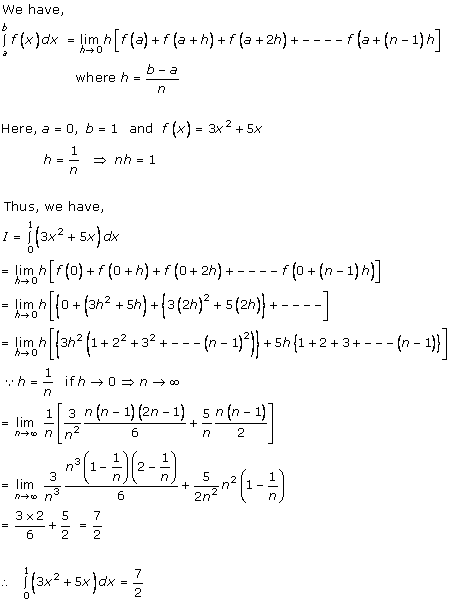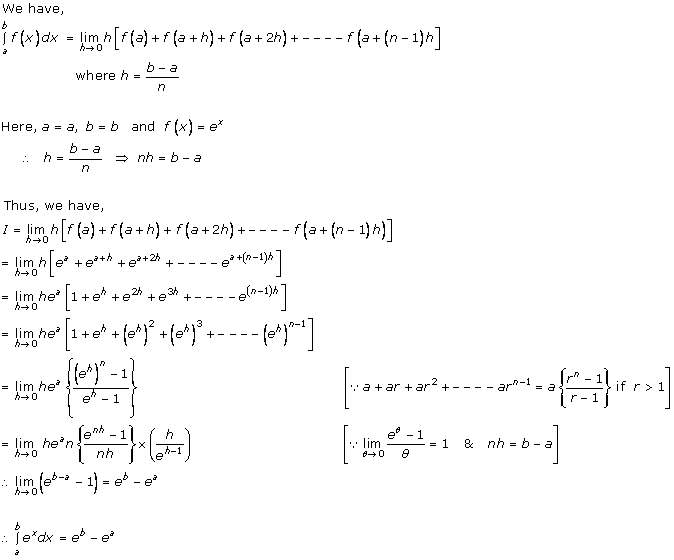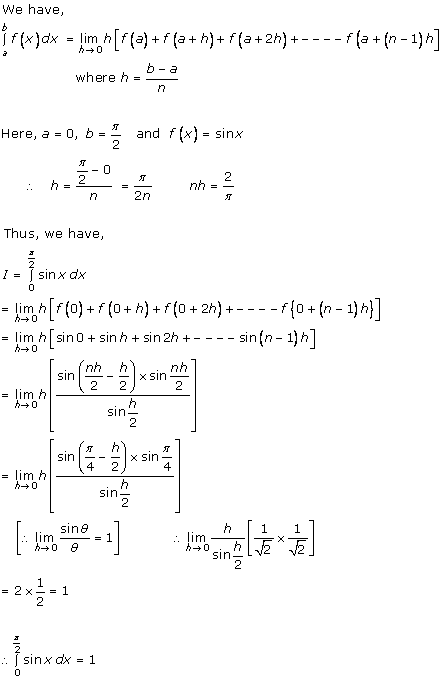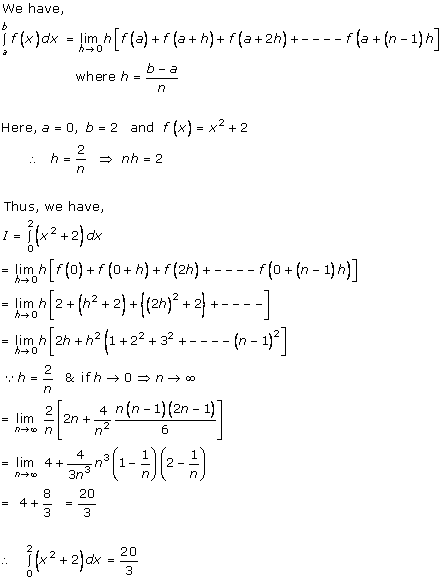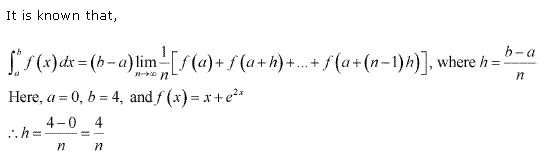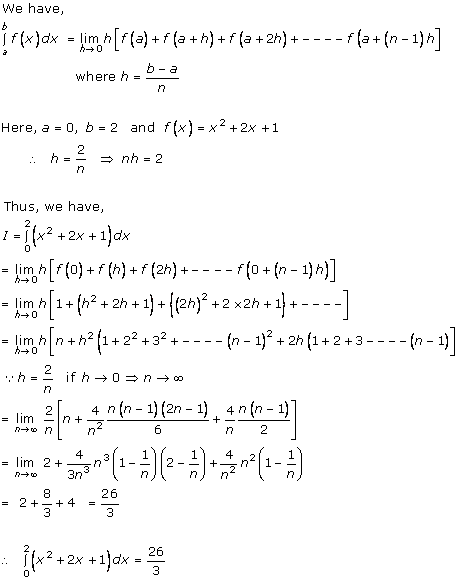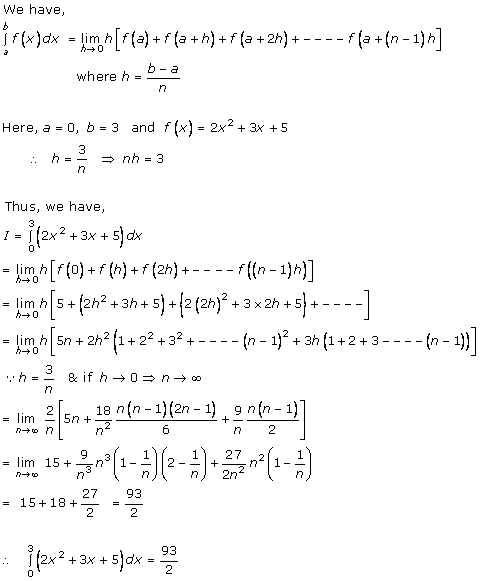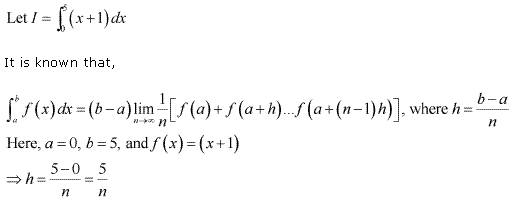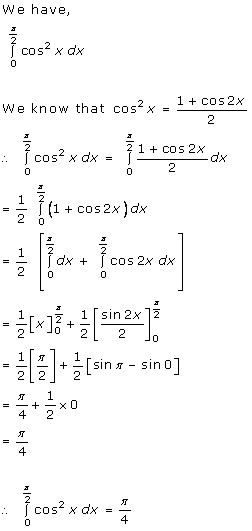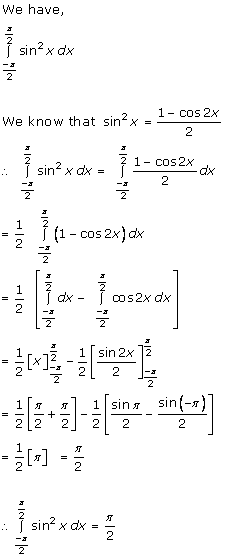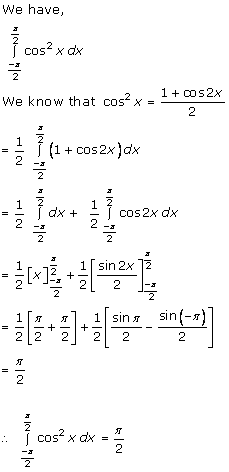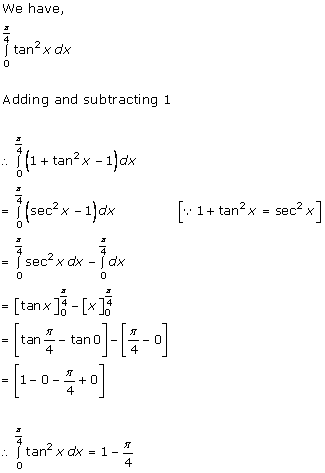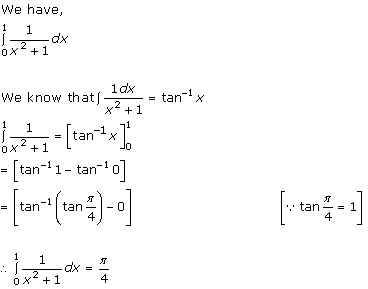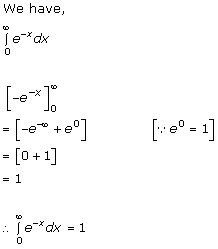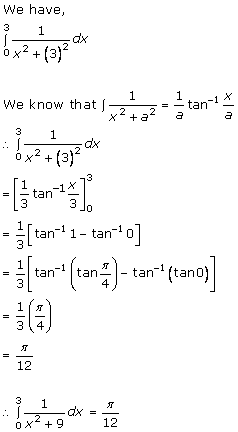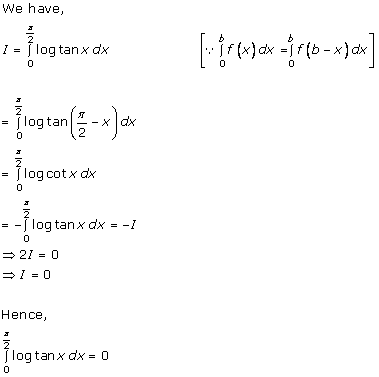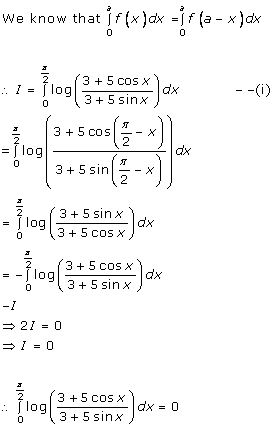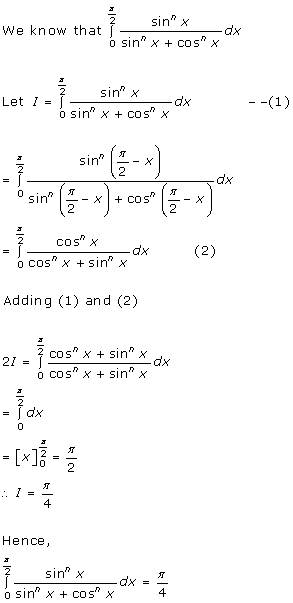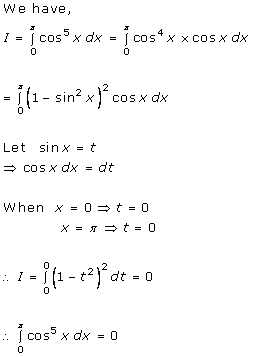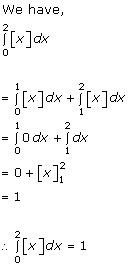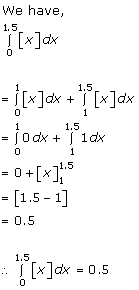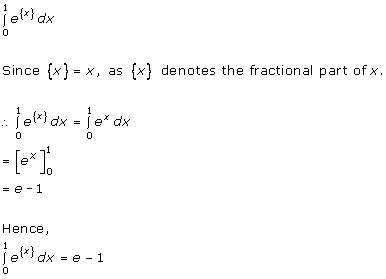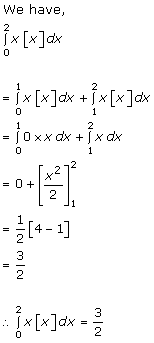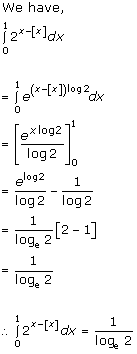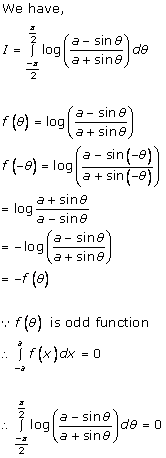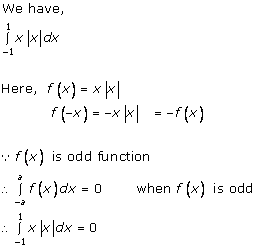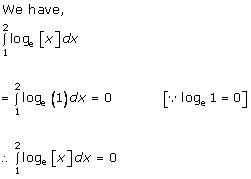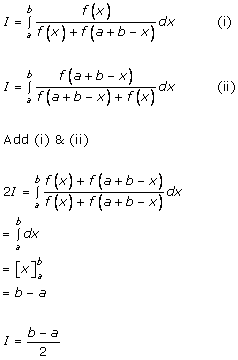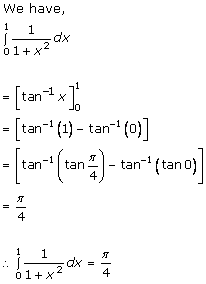Class 12-science RD SHARMA Solutions Maths Chapter 20 - Definite Integrals
Definite Integrals Exercise Ex. 20.1
Solution 1
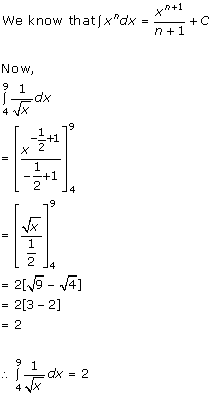
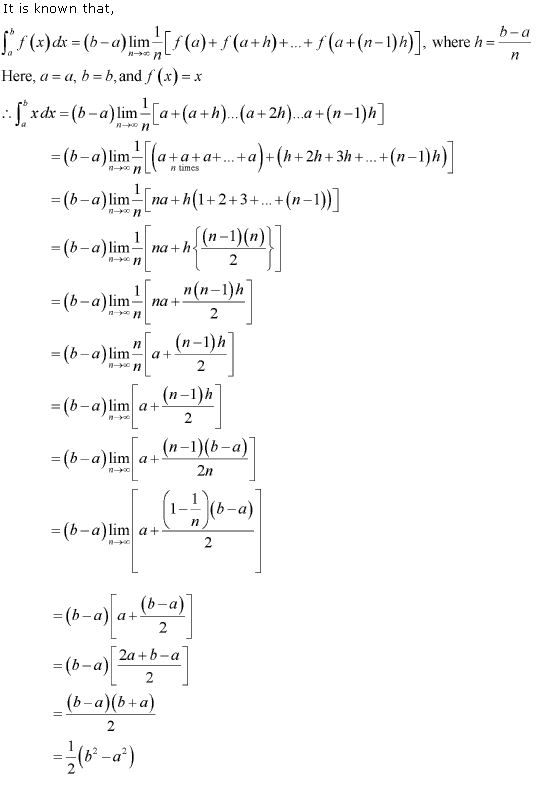
Solution 2
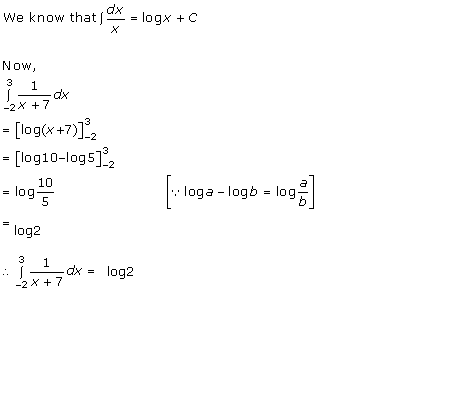
Solution 3
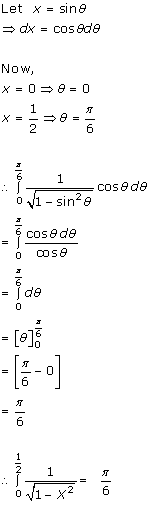
Solution 4
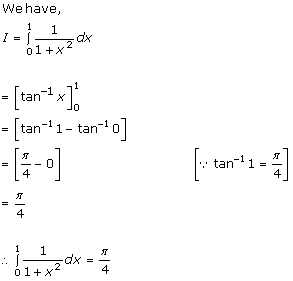
Solution 5

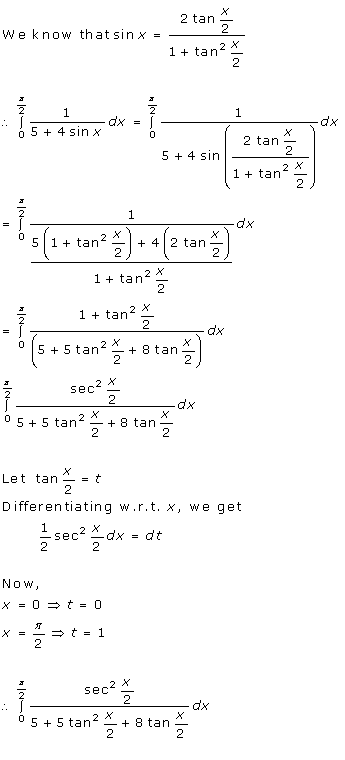
Solution 6
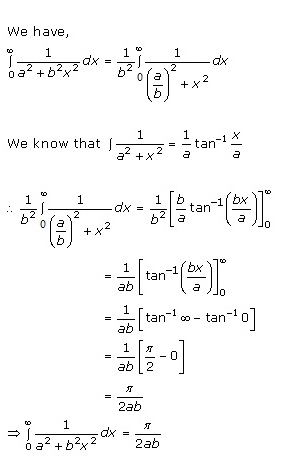
Solution 7
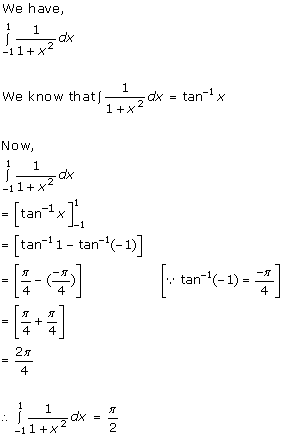
Solution 8

Solution 9
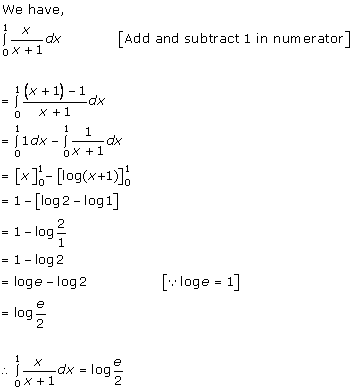
Solution 10
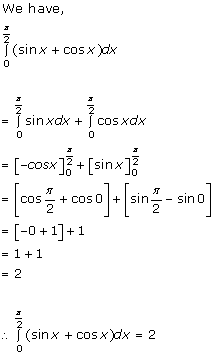
Solution 11

Solution 12

Solution 13
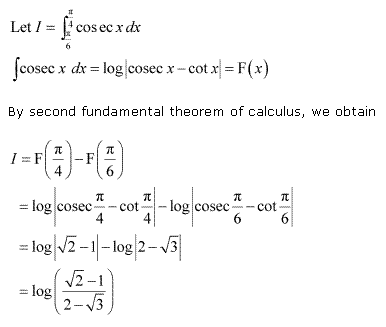
Solution 14

Solution 15

Solution 16
Solution 17
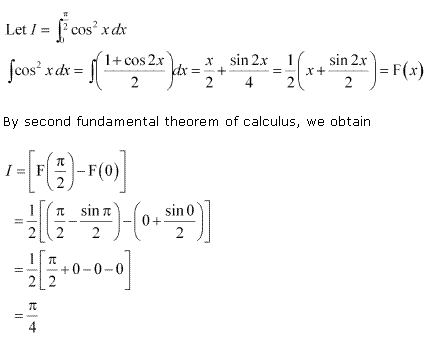
Solution 18
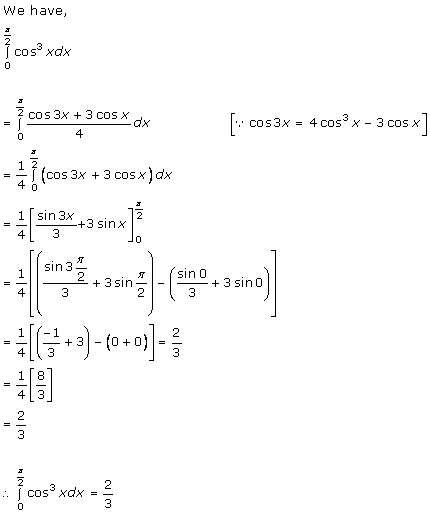
Solution 19
Solution 20
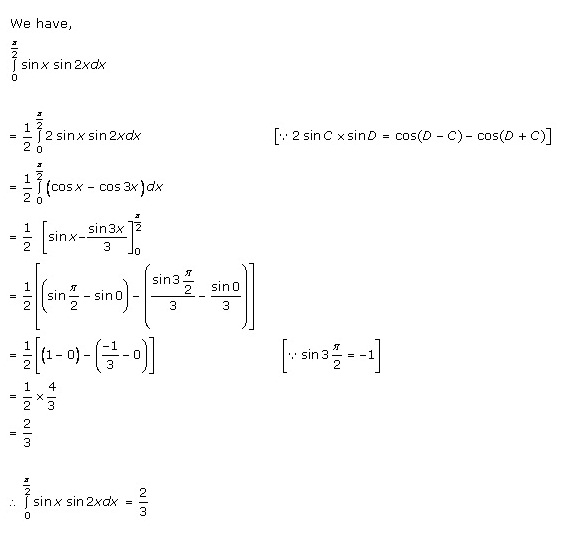
Solution 21
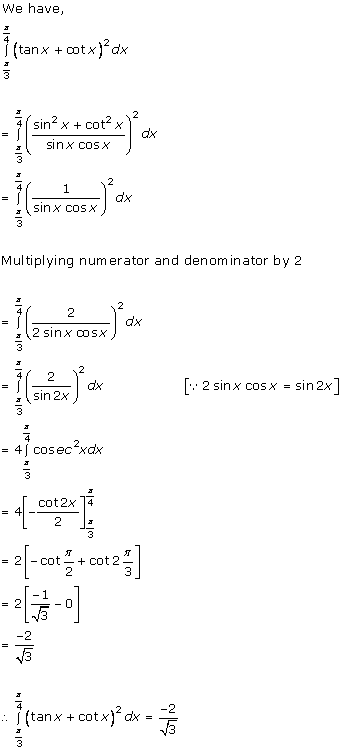
Solution 22
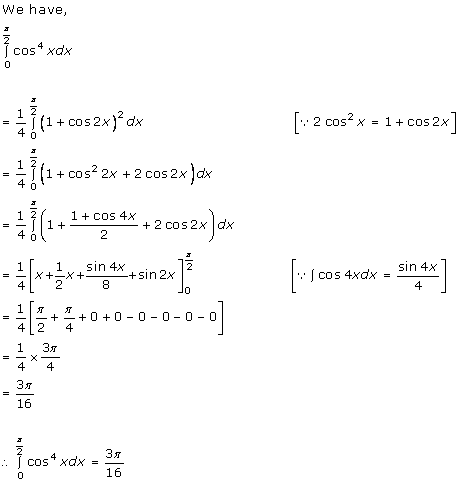
Solution 23
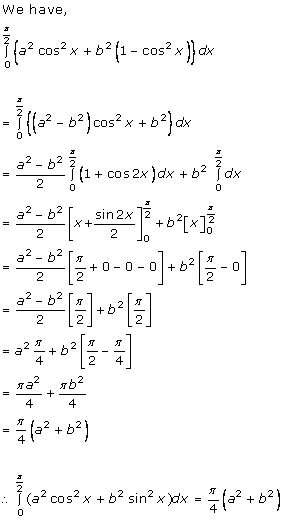
Solution 24
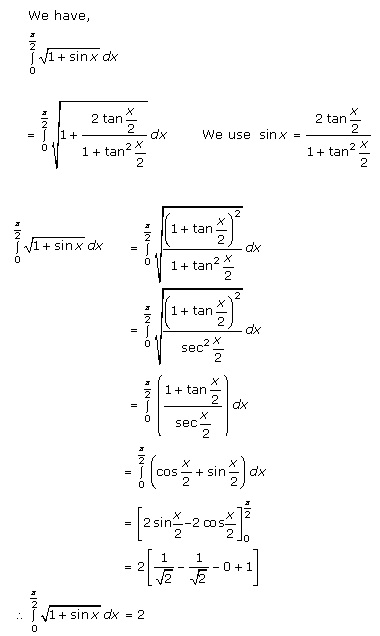
Solution 25
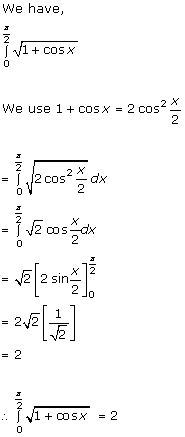
Solution 26

Solution 27

Solution 28

Solution 29
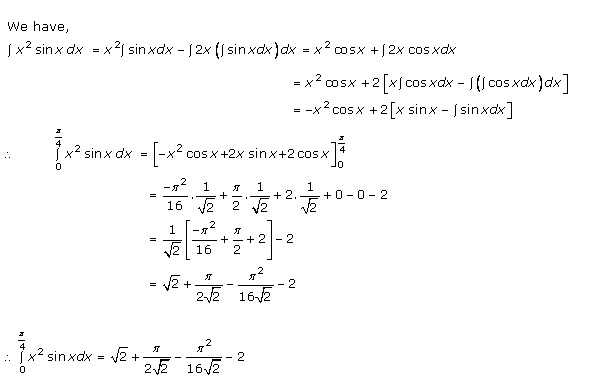
Solution 30

Solution 31

Solution 32

Solution 33
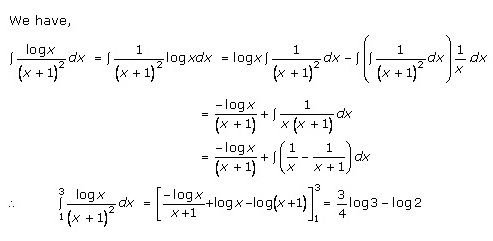
Solution 34

Solution 35
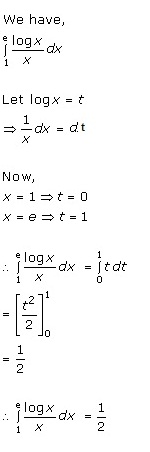
Solution 36

Solution 37
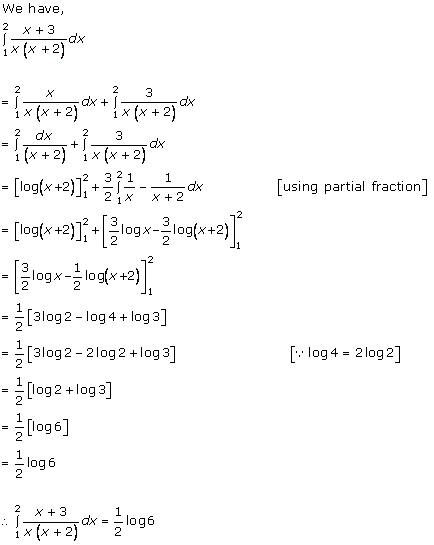
Solution 38
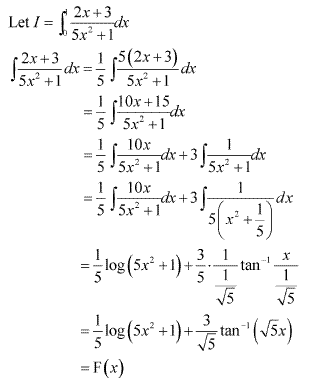
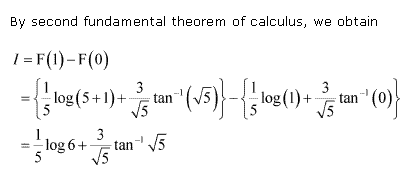
Solution 39
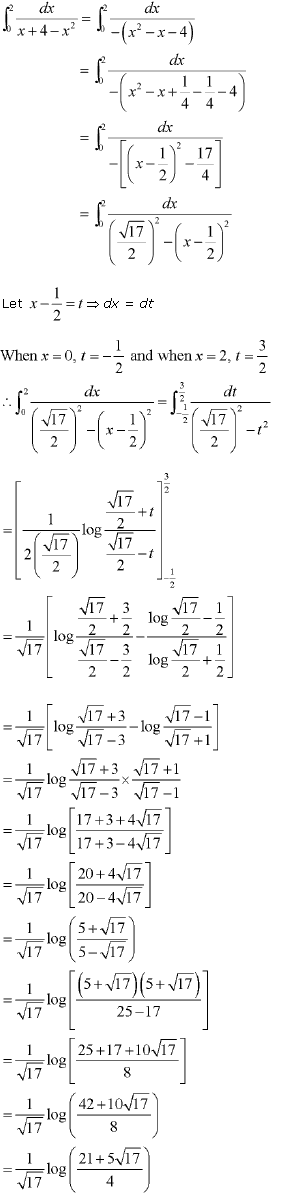
Solution 40
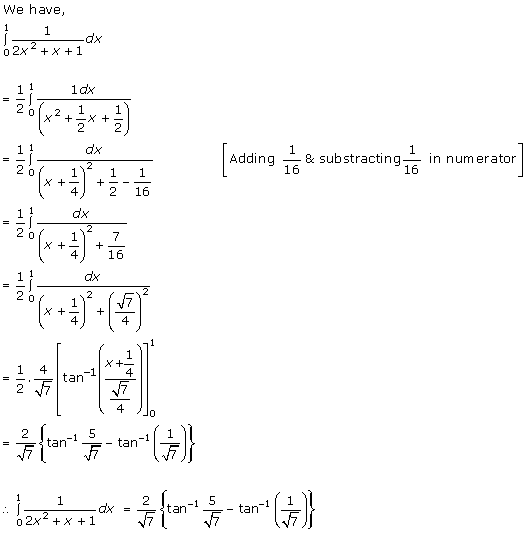
Solution 41
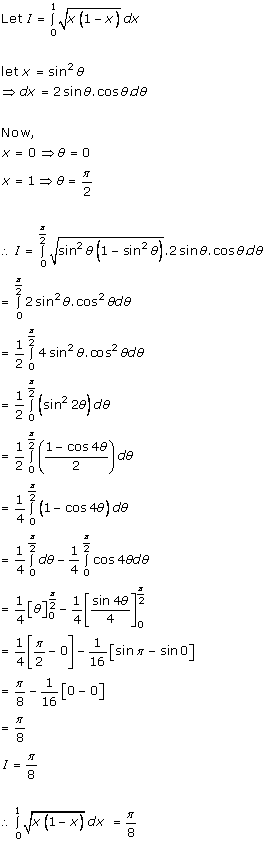
Solution 42
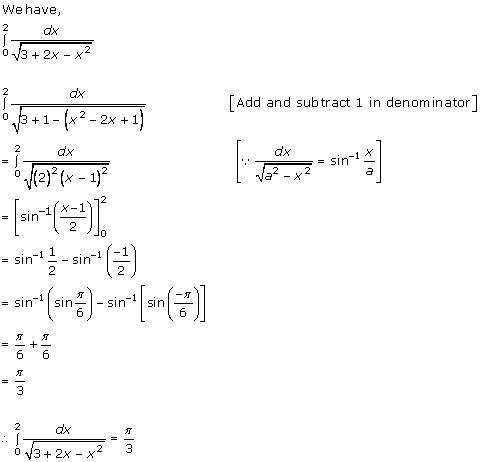
Solution 43

Solution 44

Solution 45
Solution 46

Solution 47

Solution 48

Solution 49

Solution 50

Solution 51
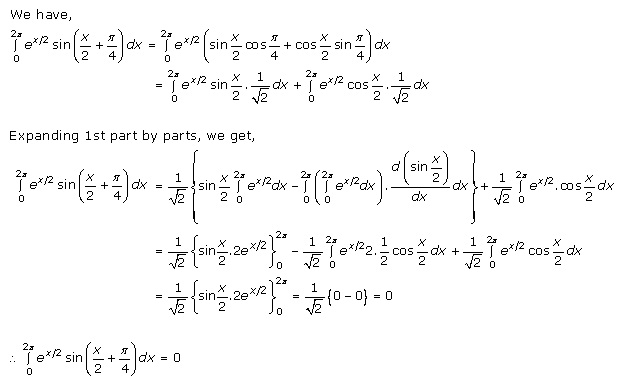
Solution 52

Solution 54
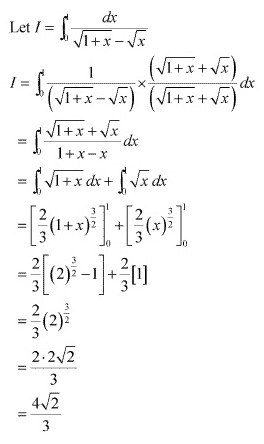

Solution 55

Solution 56
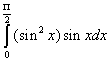
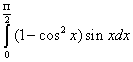
Let cosx =u , Then
![]()
![]()
Hence
![]()
![]()
![]()
![]()
Solution 57
Solution 58
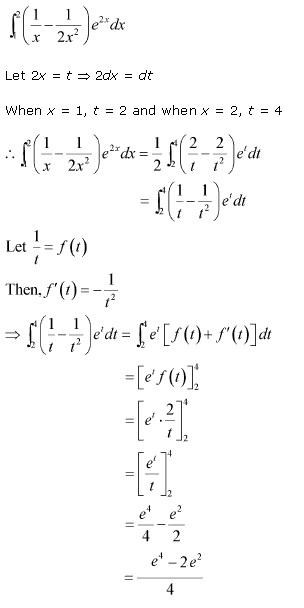
Solution 59

Solution 60
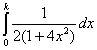

![]()
![]()
![]()
Given :
![]()
![]()
![]()
![]()
![]()
Solution 61
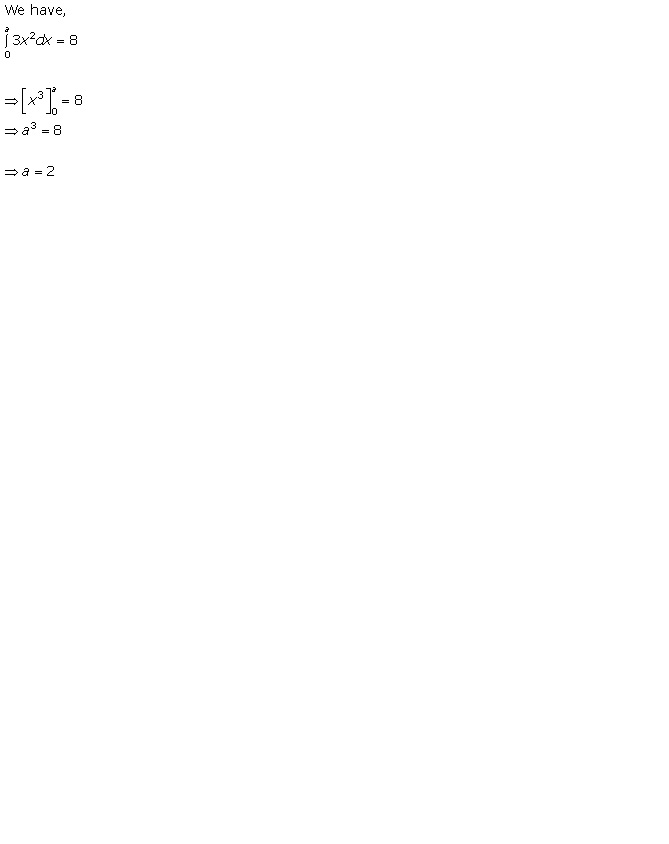
Solution 62
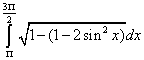
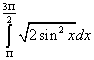
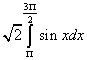
![]()
![]()
Solution 63
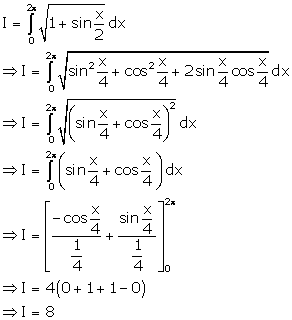
Solution 64
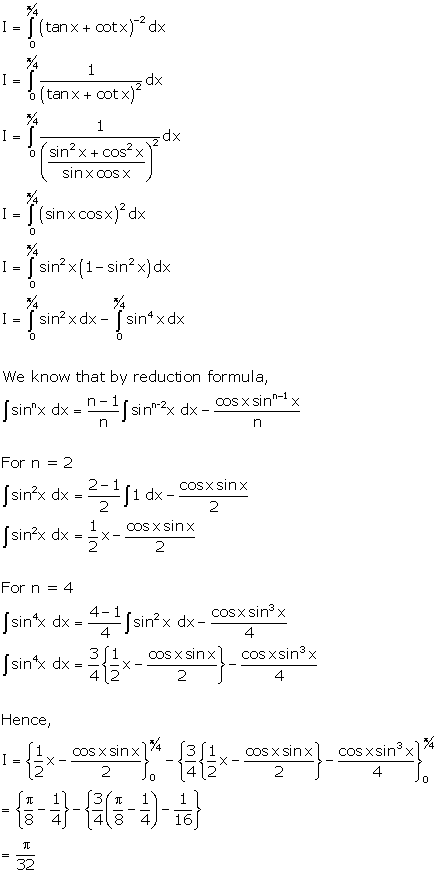

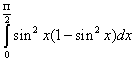
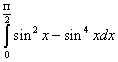
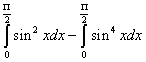
We know , By reduction formula
![]()
For n=2
![]()
![]()
For n=4
![]()
![]()
Hence

![]()
![]()
Note: Answer given at back is incorrect.
Solution 65
Using Integration By parts
![]()
![]()
![]()

Solution 66
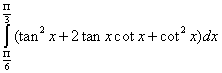
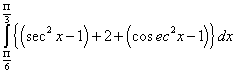
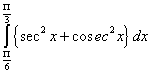
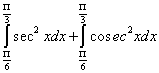
![]()
![]()
![]()
![]()
Solution 67
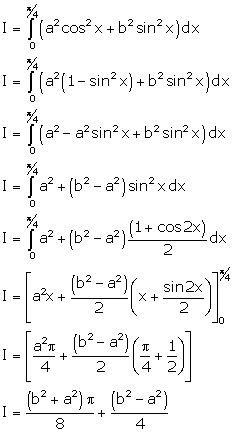
Note: Answer given in the book is incorrect.
Solution 68
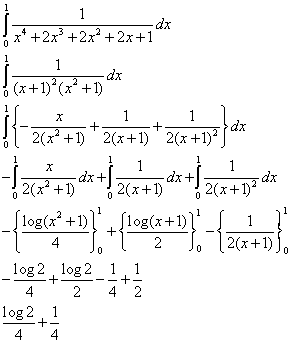
=(1/4)log(2e)
Definite Integrals Exercise Ex. 20.2
Solution 1
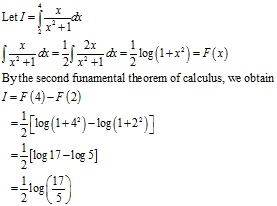
Solution 2
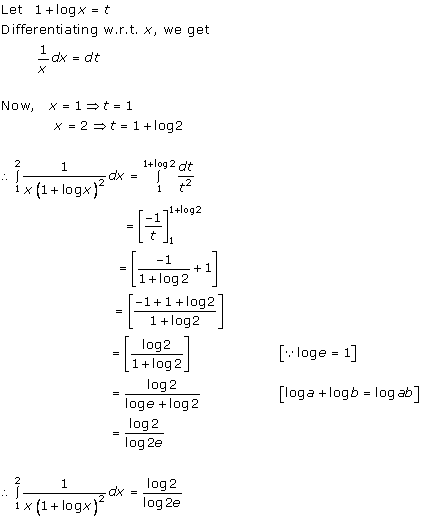
Solution 3
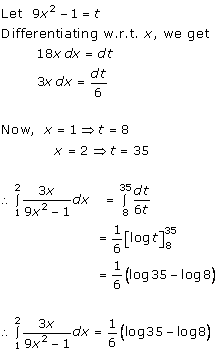
Solution 4

Solution 5
Solution 6
Solution 7
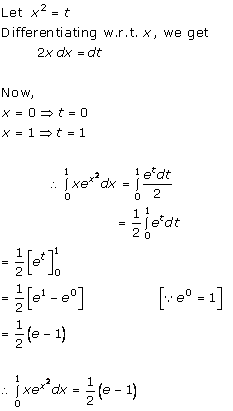
Solution 8
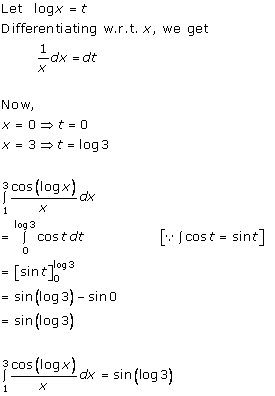
Solution 9
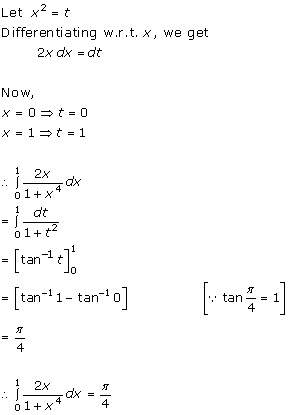
Solution 10
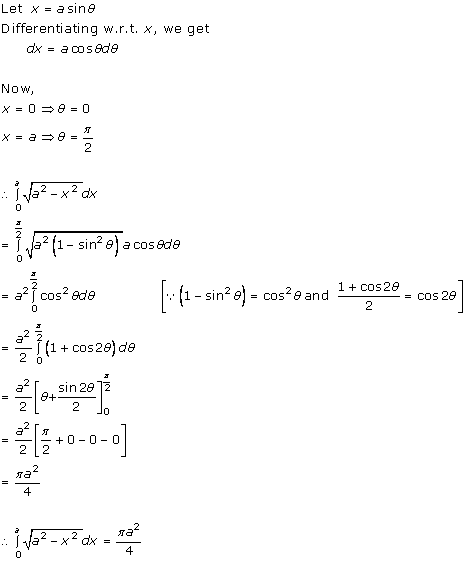
Solution 11
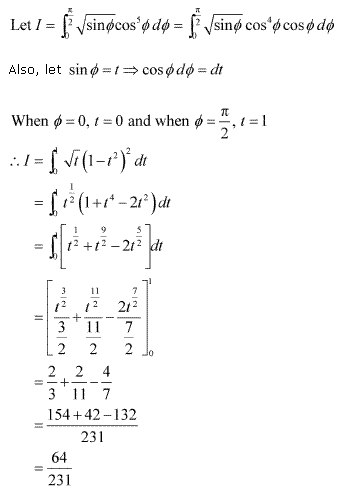
Solution 12
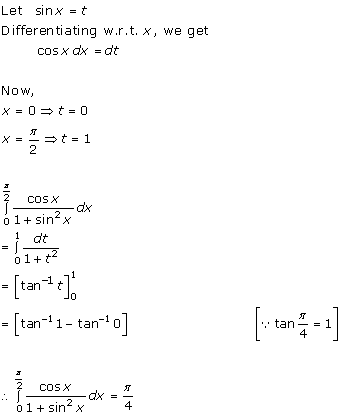
Solution 13
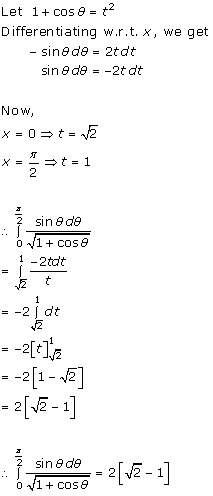
Solution 14
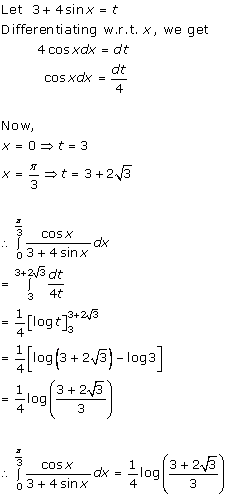
Solution 15

Solution 16
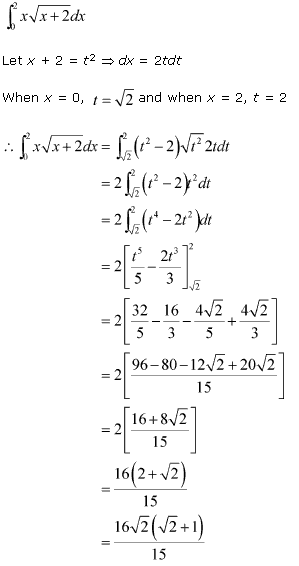
Solution 17
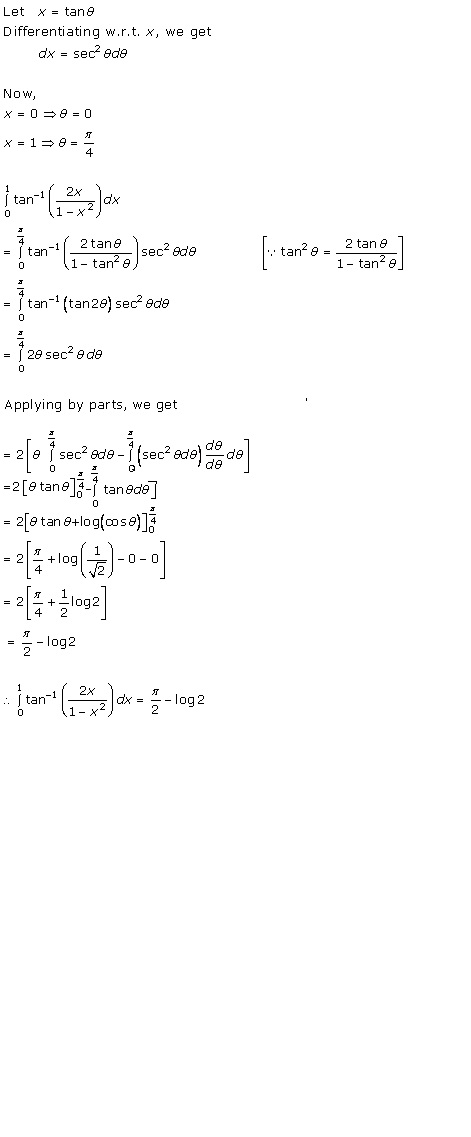
Solution 18
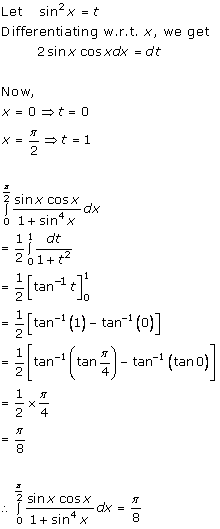
Solution 19

Solution 20
Solution 21
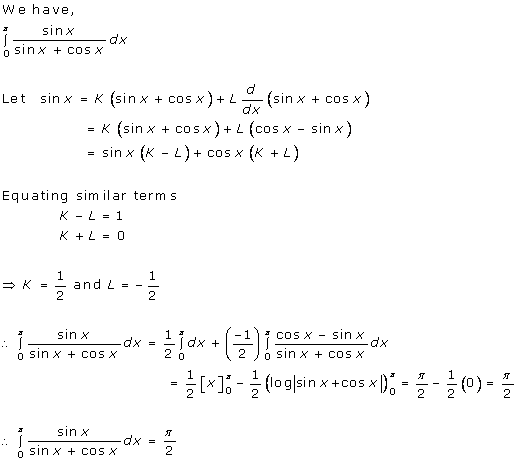
Solution 22
Solution 23

Solution 24
Using Integration By parts
![]()
![]()
![]()
![]()
![]()
Hence
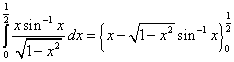
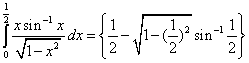
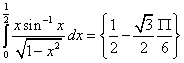
Solution 25
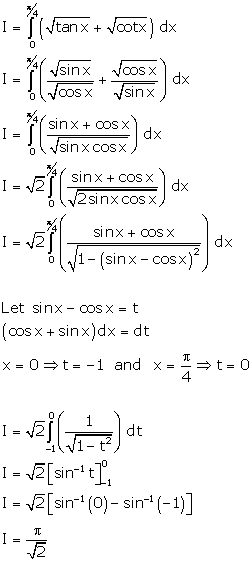
Solution 26
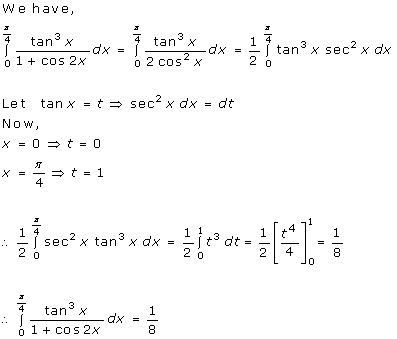
Solution 27
Solution 28
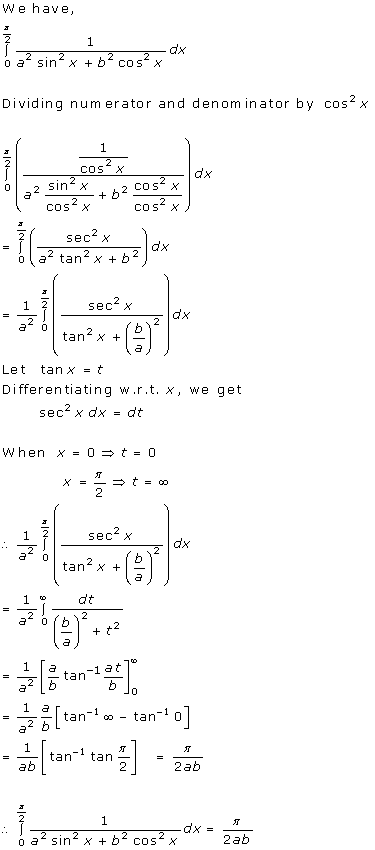
Solution 29
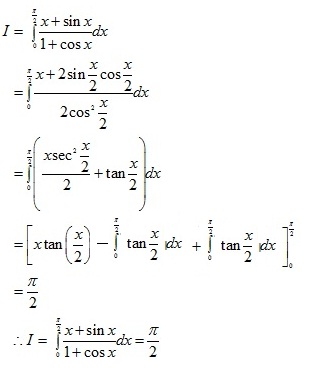
Solution 30

Solution 31
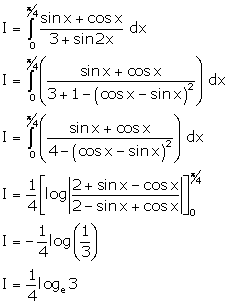
Solution 32

Solution 33
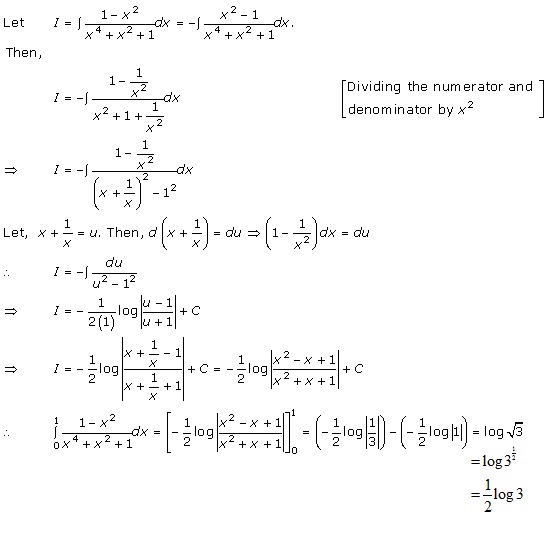
Solution 34
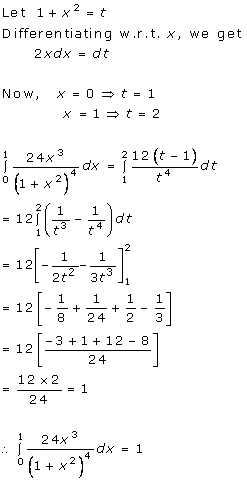
Solution 35
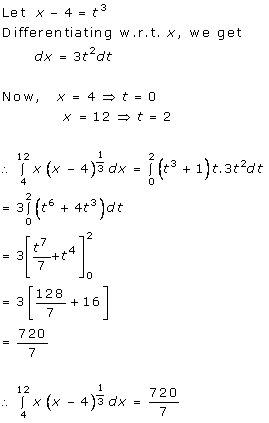
Solution 36
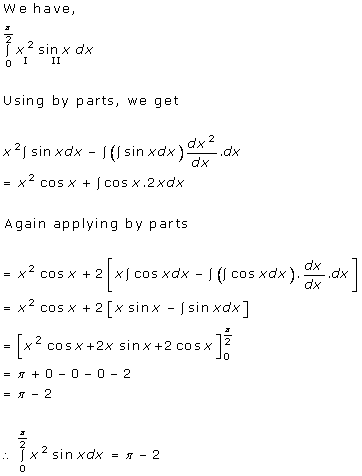
Solution 37
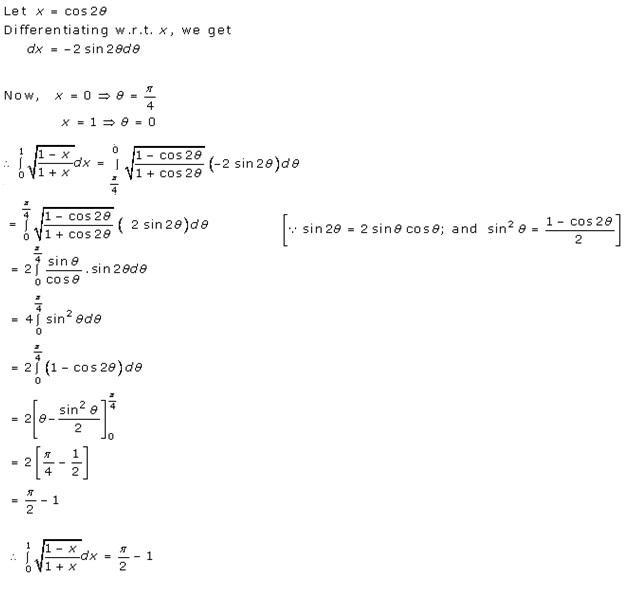
Solution 38
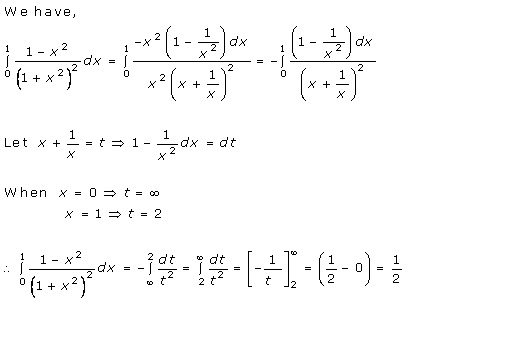
Solution 39
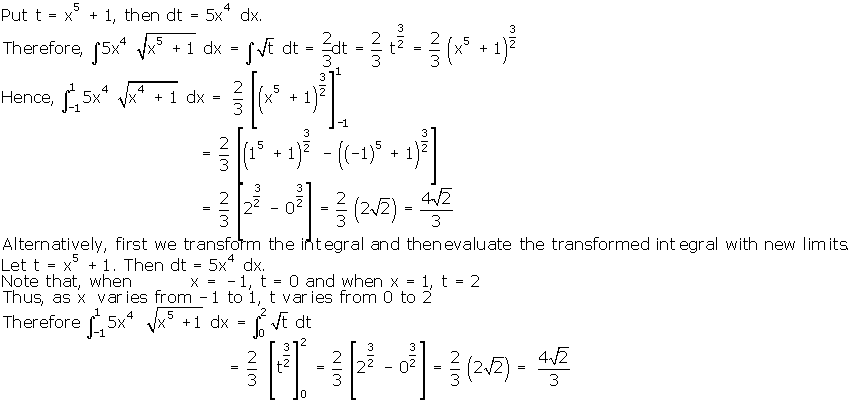
Solution 40

Solution 41

Solution 42
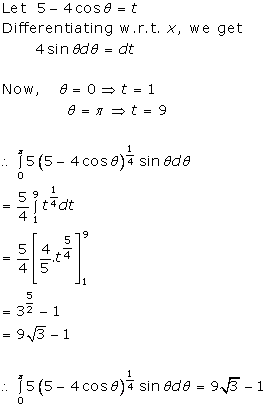
Solution 43
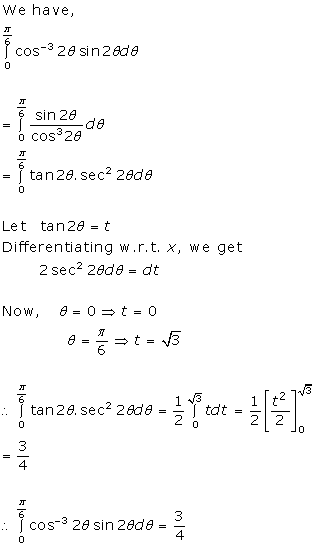
Solution 44
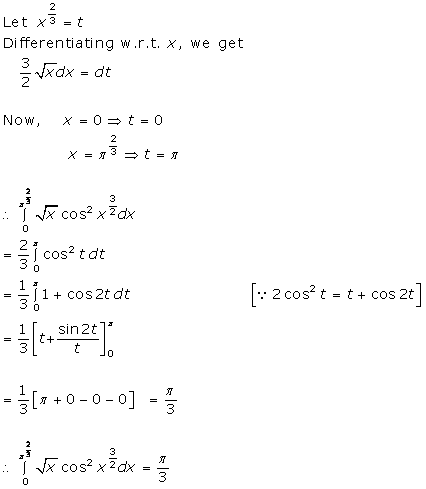
Solution 45

Solution 46
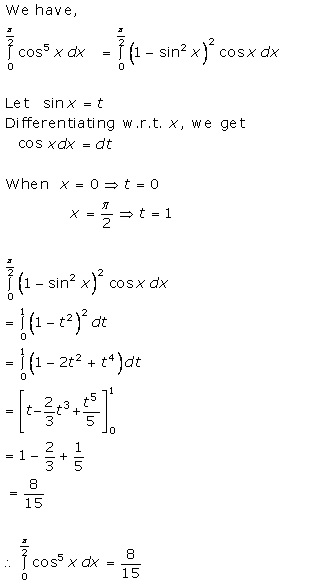
Solution 47

Solution 48
Solution 49
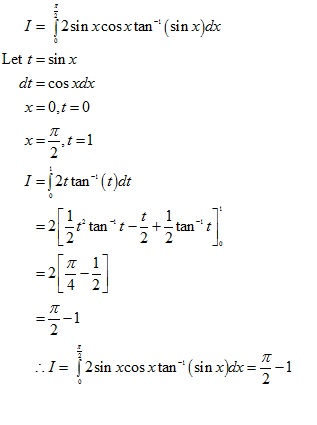
Solution 50
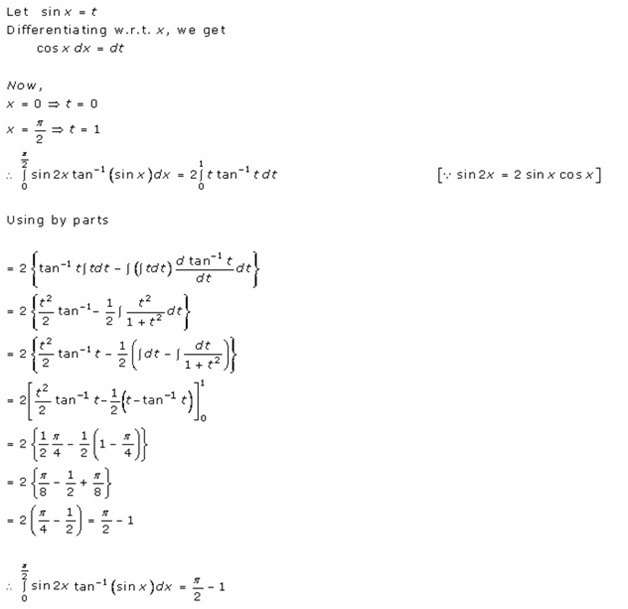
Solution 51

Solution 52

Solution 53
Solution 54

Solution 55
Solution 56
Solution 57

Solution 58

Solution 59
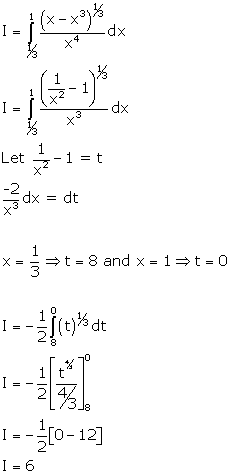
Solution 60
![]()
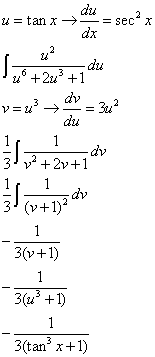

Solution 61
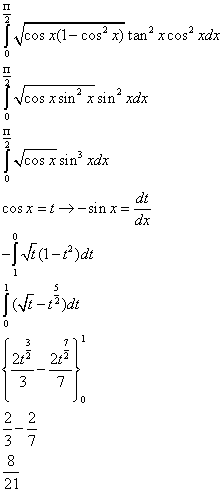
Solution 62
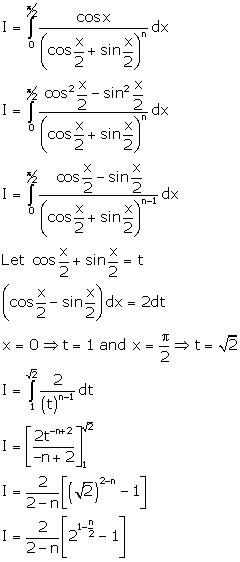
Definite Integrals Exercise Ex. 20.3
Solution 1(i)
Solution 1(ii)
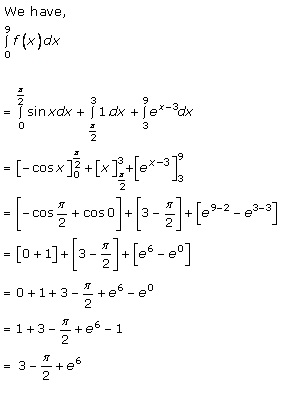
Solution 1(iii)
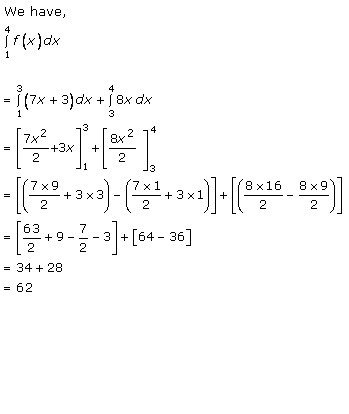
Solution 2
Solution 3

Solution 4
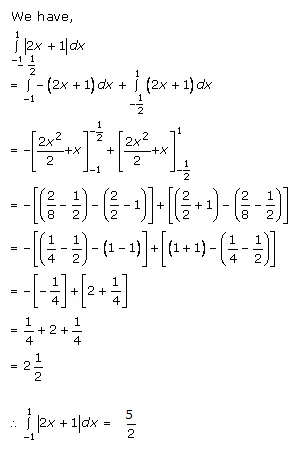
Solution 5
2x+3 is positive for x>-1.5 . Hence
![]()
![]()
![]()
![]()
![]()
Solution 6
![]()
![]()
![]()
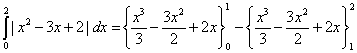
![]()
![]()
![]()
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
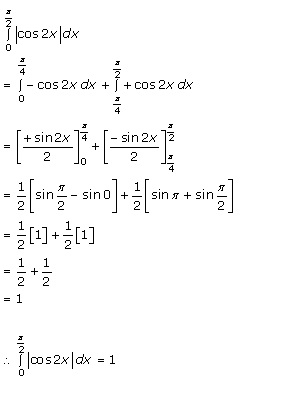
Solution 12
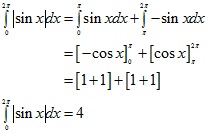
Solution 13
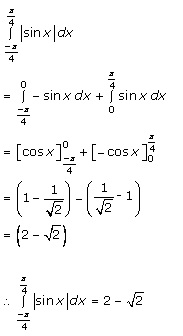
Solution 14

Solution 15
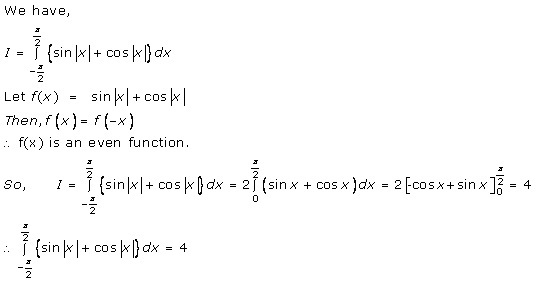
Solution 16
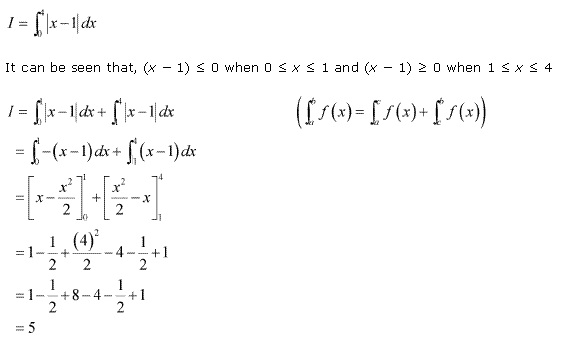
Solution 17

Solution 18
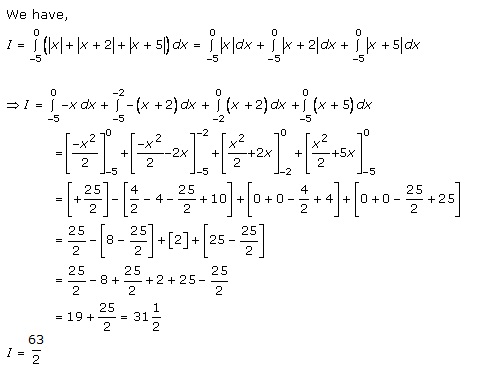
Solution 19

Solution 20
![]()
![]()

![]()
![]()
![]()
Solution 21
![]()
For
![]()
Using Integration By parts
![]()
![]()
![]()
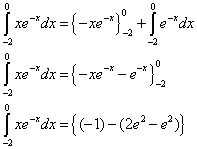
![]()
For
![]()
Using Integration By parts
![]()
![]()
![]()

Solution 22
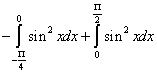
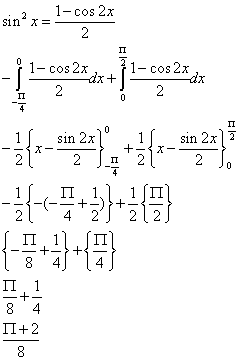
Solution 23
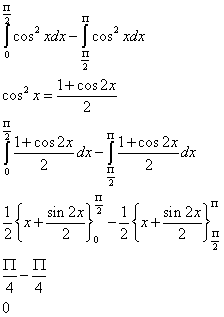
Solution 24
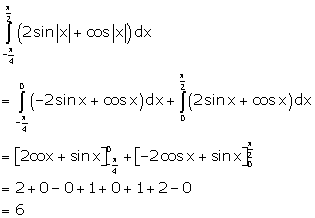
Solution 25
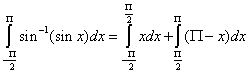
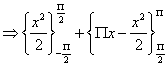
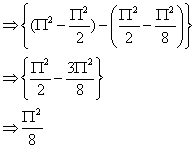
Solution 27
[x]=0 for 0 < x
and [x]=1 for 1< x < 2
Hence

Solution 28
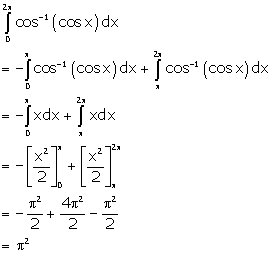
Solution 26
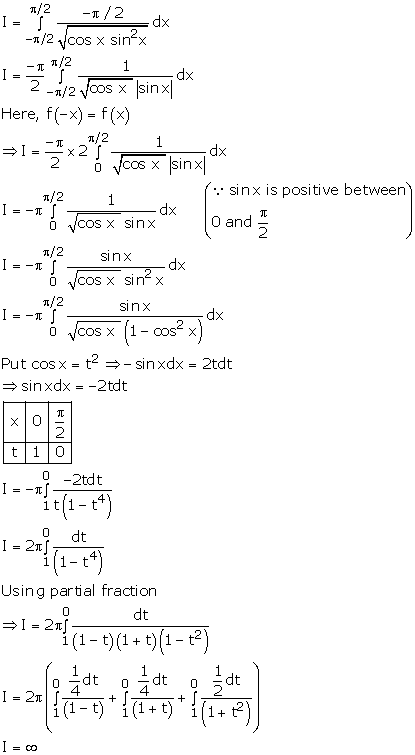
NOTE: Answer not matching with back answer.
Definite Integrals Exercise Ex. 20.4A
Solution 1
We know
![]()
Hence
![]()
We know
![]()
![]()
If
![]()
Then also
![]()
Hence
![]()
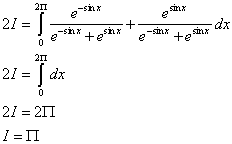
Solution 2
We know
![]()
Hence
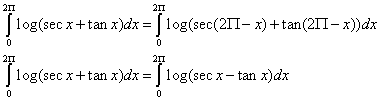
If
![]()
Then
![]()
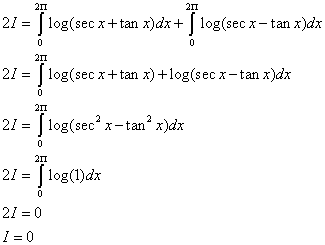
Solution 3
We know
![]()
Hence
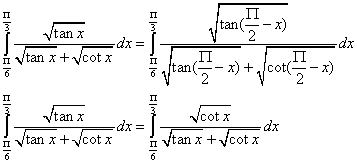
If
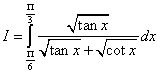
Then
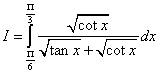
So
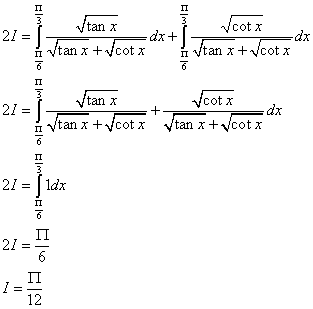
Solution 4
We know
![]()
Hence
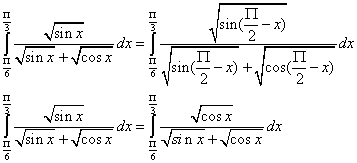
If
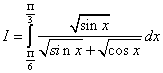
Then
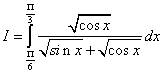
Hence
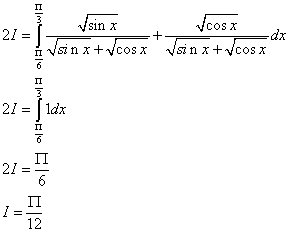
Solution 5
We know
![]()
Hence
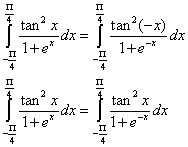
If
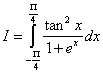
Then
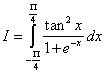
So
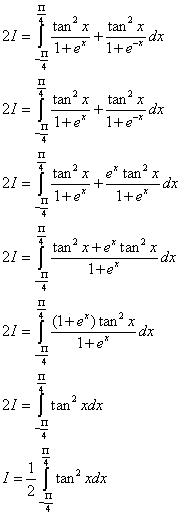
We know
If f(x) is even
![]()
If f(x) is odd
![]()
Here
![]()
f(x) is even, hence
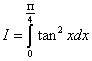
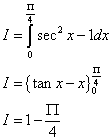
Note: Answer given in the book is incorrect.
Solution 6
We know
![]()
Hence
![]()
If
![]()
Then
![]()
So
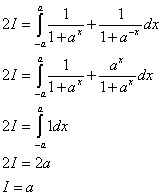
Solution 7
We know
![]()
Hence
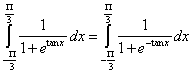
If
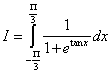
Then
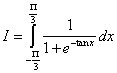
So
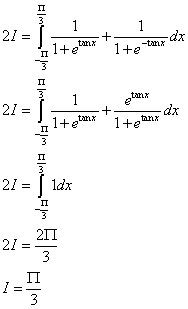
Solution 8
We know
![]()
Hence
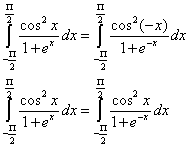
If
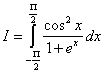
Then
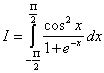
So
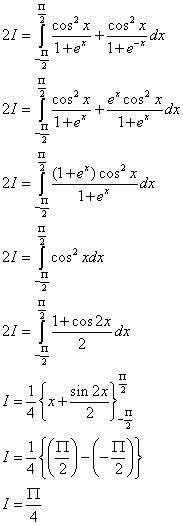
Note: Answer given in the book is incorrect.
Solution 9

If f(x) is even
![]()
If f(x) is odd
![]()
Here
![]() is odd and
is odd and
![]() is
even. Hence
is
even. Hence
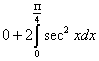
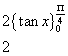
Solution 10

Solution 11

Solution 12
Solution 13

Solution 14
Solution 15
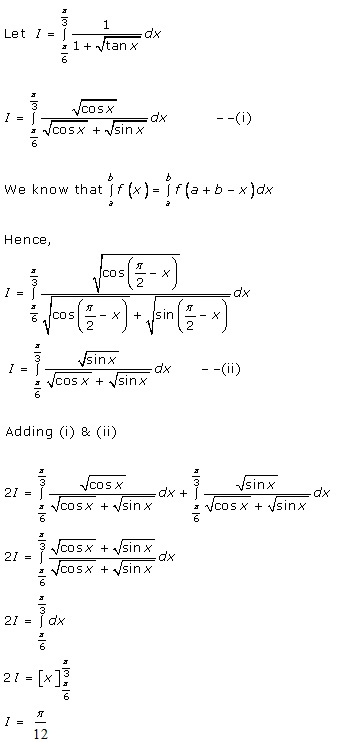
Solution 16
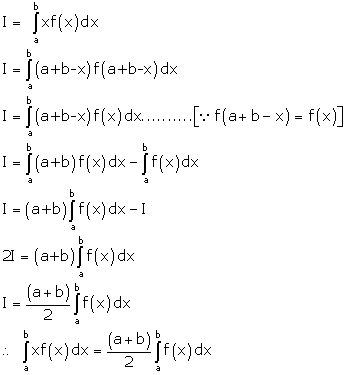
Definite Integrals Exercise Ex. 20.4B
Solution 1
Solution 2
Solution 3
B
Solution 4
Solution 5
Solution 6
Solution 7

Solution 8

Solution 9

Solution 10
Solution 11

Solution 12

Solution 13
Solution 14

Solution 15
Solution 16

Solution 17
Solution 18
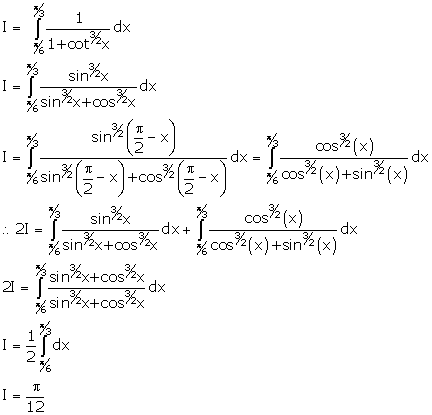
Solution 19
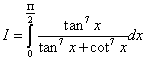
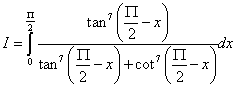
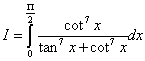
Hence
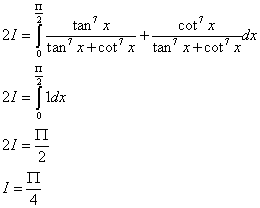
Solution 20
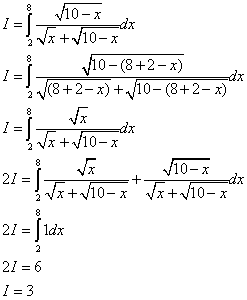
Solution 21
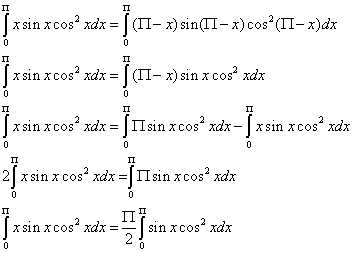
Now
![]()
Let cosx=t
![]()
![]()
![]()
![]()
![]()
![]()
Solution 22

Solution 23
Solution 24

Solution 25 (i)

Solution 26
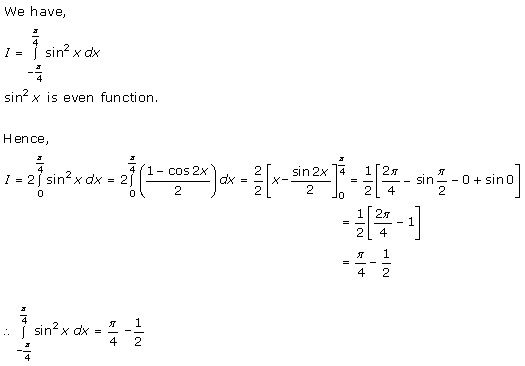
Solution 27

Solution 28

Solution 29

Solution 30
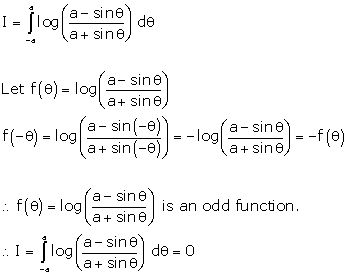
Solution 31
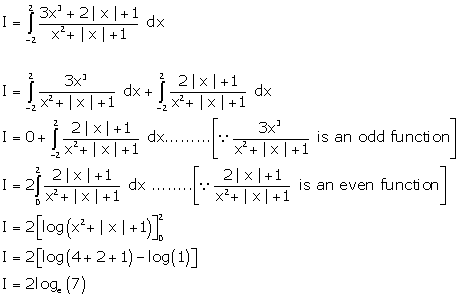
Solution 32 (i)
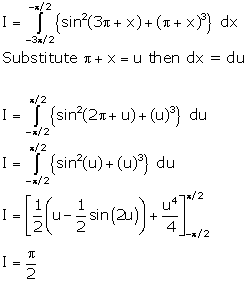
Solution 33
Solution 34

Solution 35
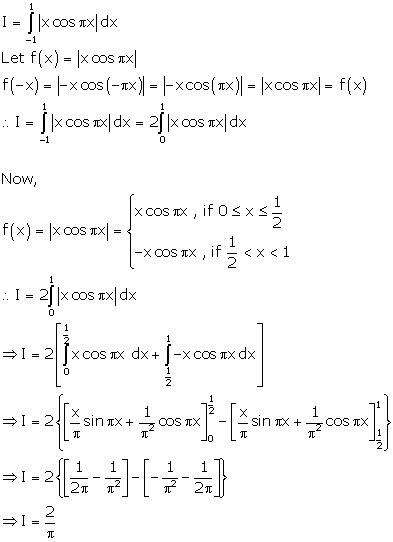
Solution 36
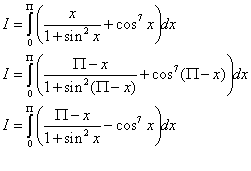
![]()
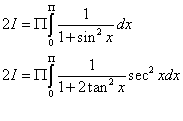
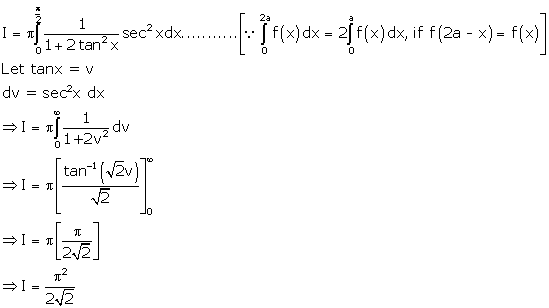
Solution 37
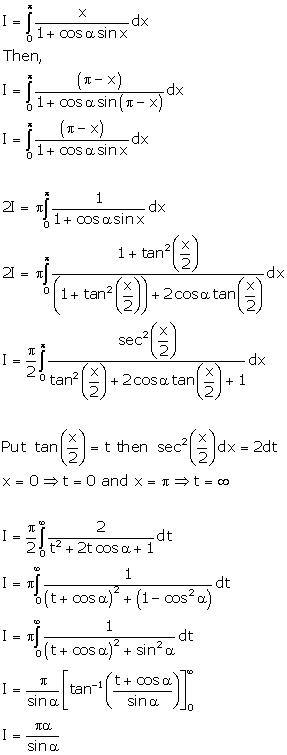
Solution 38
We know
![]()
Also here
![]()
So
![]()
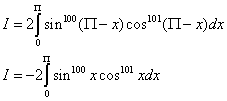
Hence
![]()
Solution 39
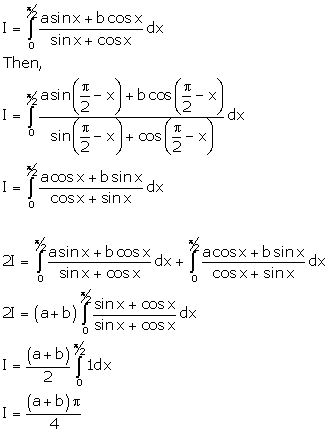
Solution 43

Solution 44

Solution 45


Solution 46

Solution 47
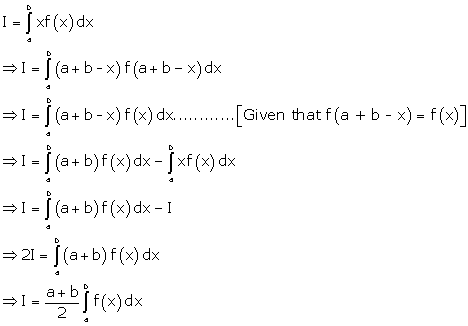
Solution 48

Solution 49
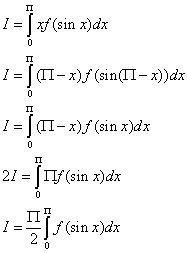
Definite Integrals Exercise Ex. 20RE
Solution 1

Solution 2
Solution 3
Solution 4

Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
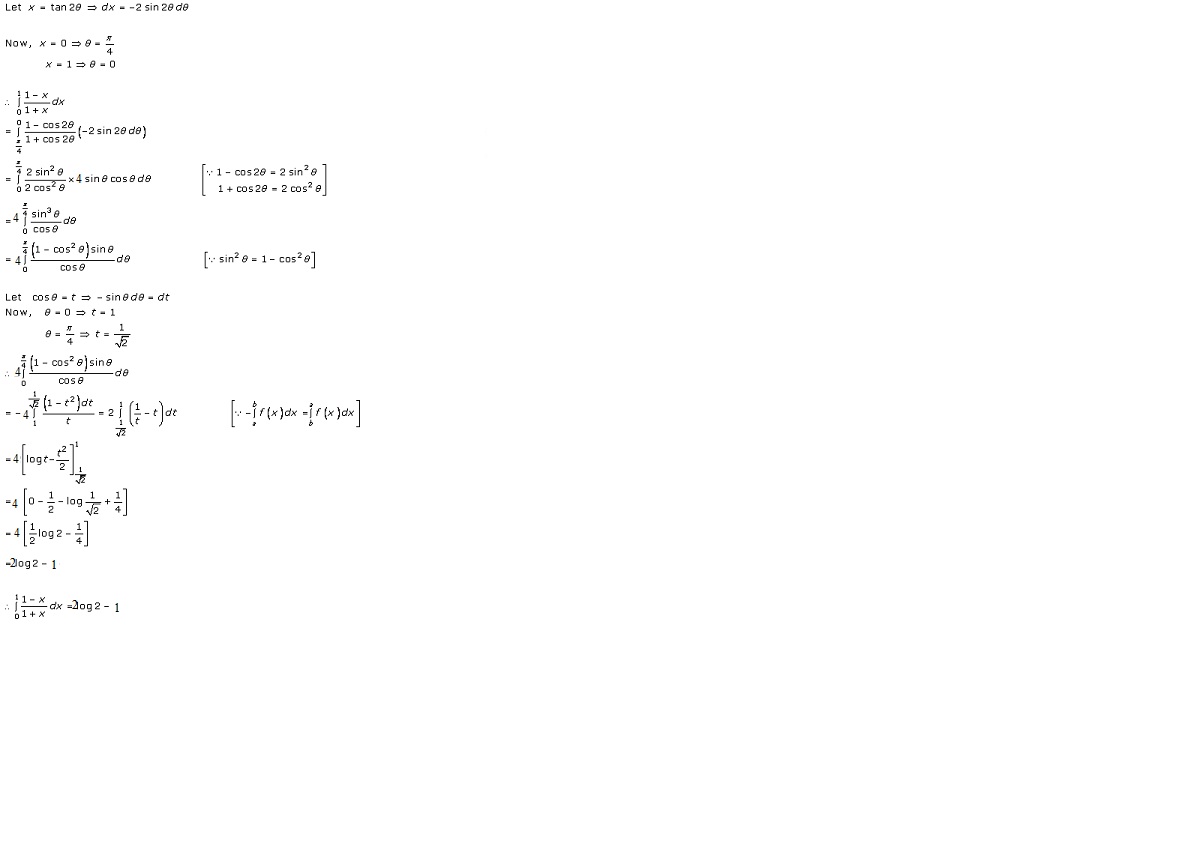
Solution 10
Solution 11
Solution 12

Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
Solution 20
Solution 21

Solution 22

Solution 23
Solution 24
Solution 25
Solution 26
Solution 27
Solution 28

Solution 29
Solution 30
Solution 31
Solution 32
Solution 33
Solution 34
Solution 35
Solution 36
Solution 37
Solution 38

Solution 39

Solution 40
Solution 41
Solution 42

Solution 43
Solution 44

Solution 45
Solution 46
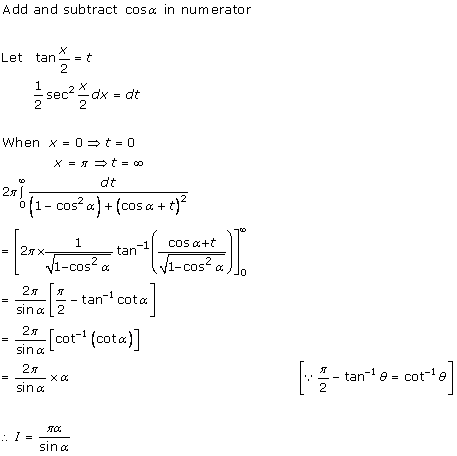
Solution 47
Solution 48
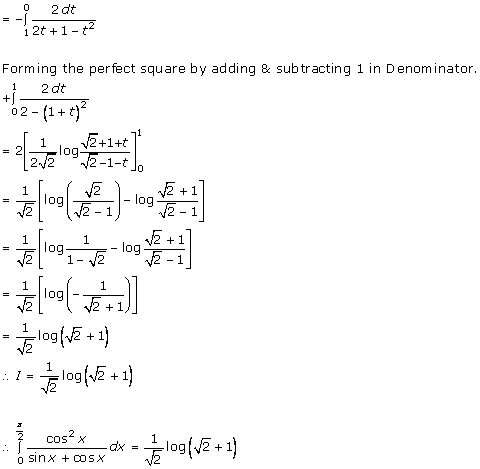
Solution 49
Solution 50
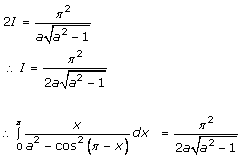
Solution 51
Solution 52
Solution 53

Solution 54
Solution 55
Solution 56
Solution 57
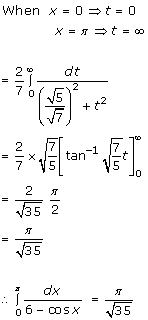
Solution 58
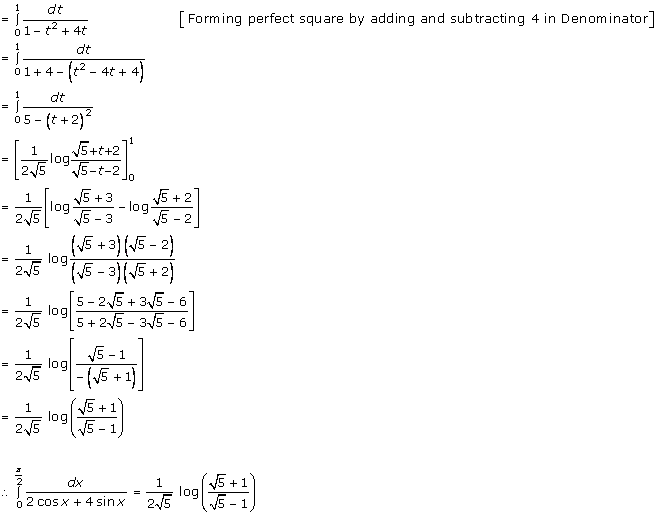
Solution 59
Solution 60

Solution 61

Solution 62

Solution 63
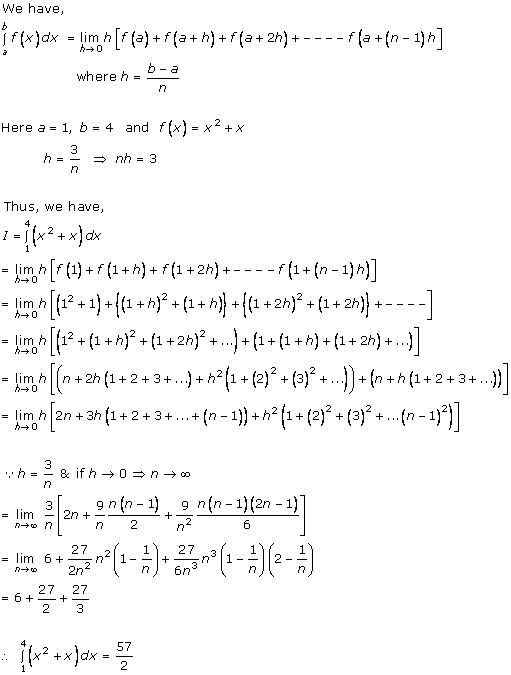
Solution 64

Solution 65

Solution 66

Solution 67
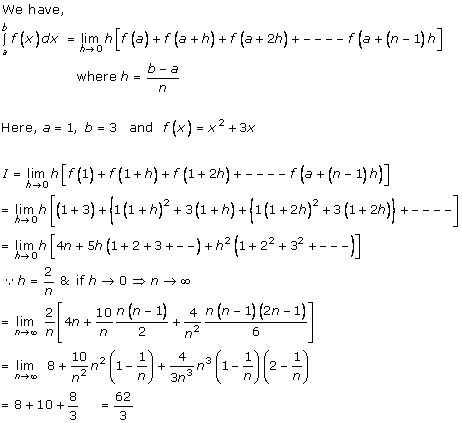
Solution 68
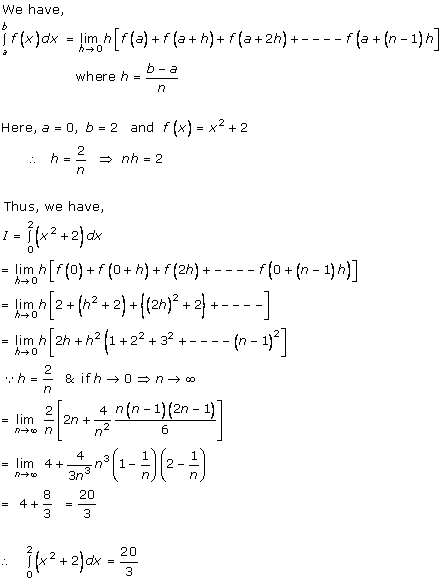
Solution 69

Definite Integrals Exercise Ex. 20.5
Solution 1
Solution 2
Solution 3


Solution 4
Solution 5

Solution 6

Solution 7
Solution 8
Solution 9

Solution 10
Solution 11
Solution 12

Solution 13
Solution 14
Solution 15

Solution 16
Solution 17

Solution 18
Solution 19

Solution 20
Solution 21
Solution 22
Solution 23

Solution 24

Solution 25
Solution 26
Solution 27
Solution 28
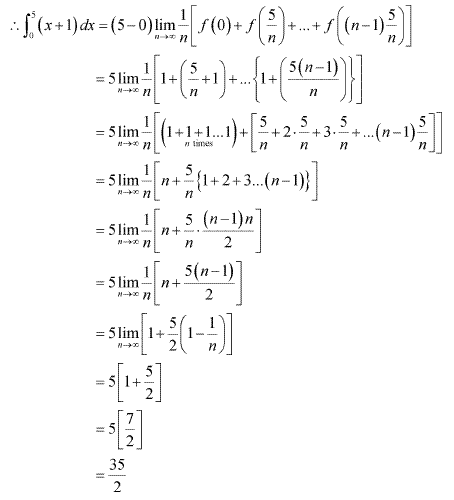
Solution 29
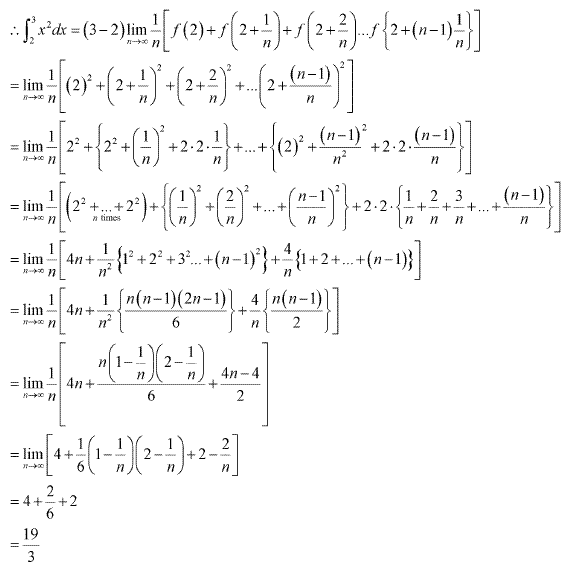
Solution 30

Solution 31

Solution 32


Solution 33

Definite Integrals Exercise MCQ
Solution 1
Correct option: (d)

Solution 2
Correct option: (c)
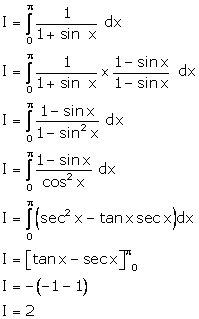
Solution 3
Correct option: (a)

Solution 4
Correct option: (c)

Note: Answer not matching with back answer.
Solution 5
Correct option:(c)

Solution 6
Correct option: (b)

Solution 7
Correct option: (a)
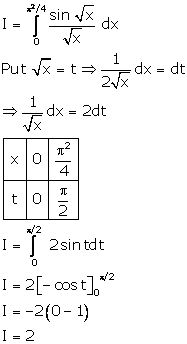
Solution 8
Correct option: (d)

Solution 9
Correct option: (b)

Solution 10

Note: Answer not matching with back answer.
Solution 11
Correct option: (a)

Solution 12
Correct option:(c)

Solution 13
Correct option: (a)
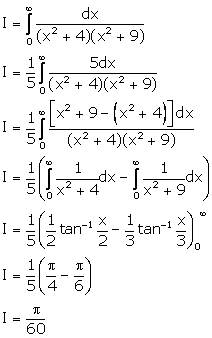
Solution 14
Correct option: (a)

Solution 15
Correct option:(a)
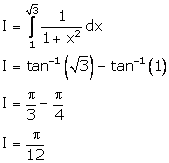
Solution 16
Correct option:(a)
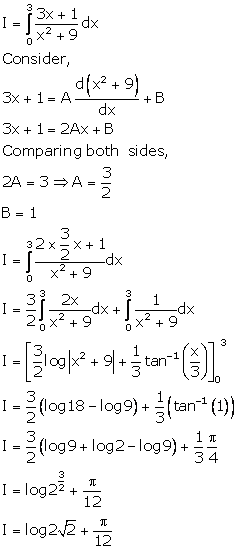
Solution 17
Correct option:(b)

Solution 18
Correct option: (b)
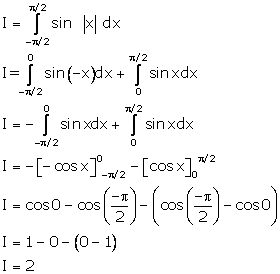
Solution 19
Correct option: (a)

Solution 20
Correct option: (b)

Solution 21
Correct option:(b)

Solution 22
Correct option: (b)

Solution 23
Correct option: (c)

Solution 24
Correct option: (b)

Solution 25
Correct option: (b)
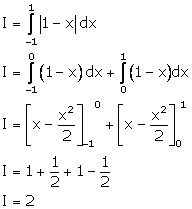
Solution 26
Correct option:(c)

Solution 27
Correct option: (b)

Solution 28
Correct option: (d)
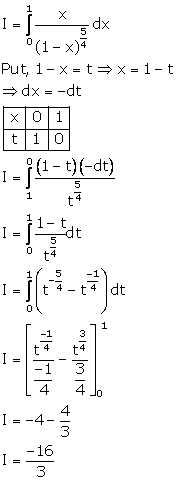
Note: Question is modified.
Solution 29
Correct option: (c)
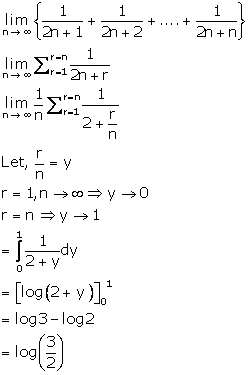
Solution 30
Correct option:(a)

Solution 31
Correct option:(d)

Solution 32
Correct option: (d)
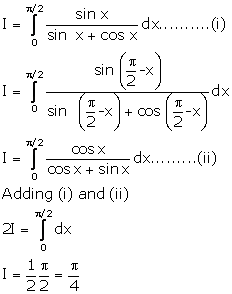
Solution 33
Correct option:(c)

Note: Answer not matching with back answer.
Solution 34
Correct option:(d)

Note: Answer not matching with back answer.
Solution 35
Correct option: (c)

Solution 36
Correct option: (a)

Solution 37
Correct option:(d)
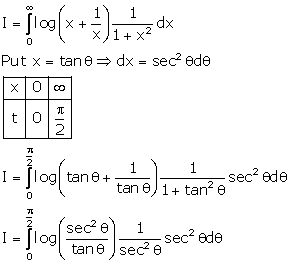


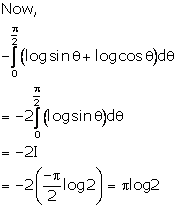
NOTE: Answer is not matching with back answer.
Solution 38
Correct option: (c)
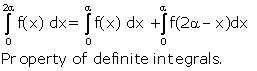
Solution 39
Correct option: (d)

Solution 40
Correct option: (b)

Solution 41
Correct option: (c)
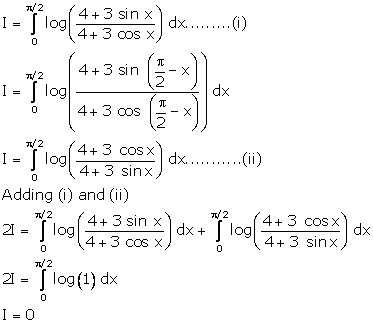
Solution 42
Correct option: (c)

Definite Integrals Exercise Ex. 20VSAQ
Solution 1

Solution 2
Solution 3
Solution 4
Solution 5
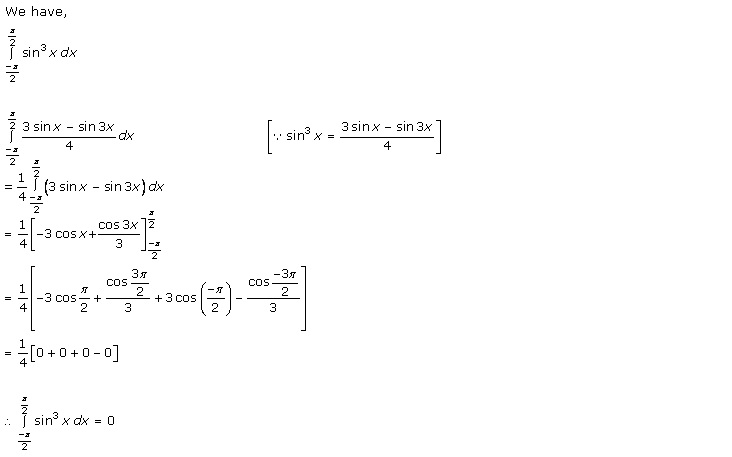
Solution 6
Solution 7
Solution 8
Solution 9
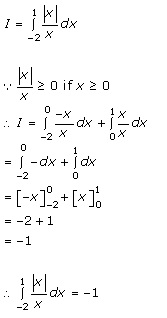
Solution 10
Solution 11
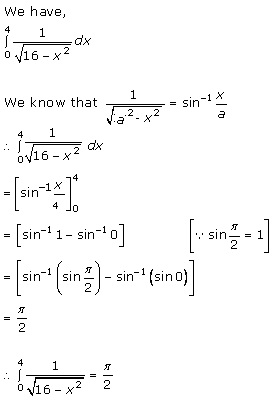
Solution 12
Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
Solution 37
Solution 38
Solution 39

Solution 40
Solution 41
Solution 42
Solution 18
Solution 19
Solution 43
Solution 20
Solution 21
Solution 23
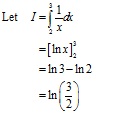
Solution 24
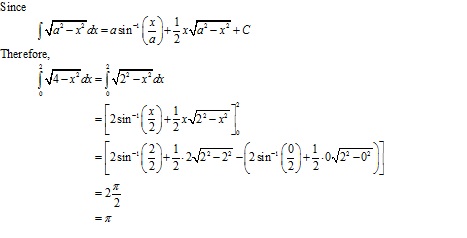
Solution 25

Solution 44
Solution 31
Solution 45
Solution 35

Solution 32

Solution 33

Solution 30

Solution 29
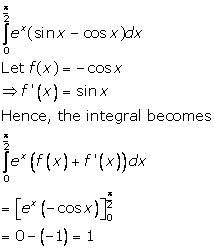
Solution 28

Solution 34

Solution 27
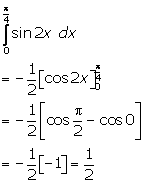
Solution 26

Solution 22